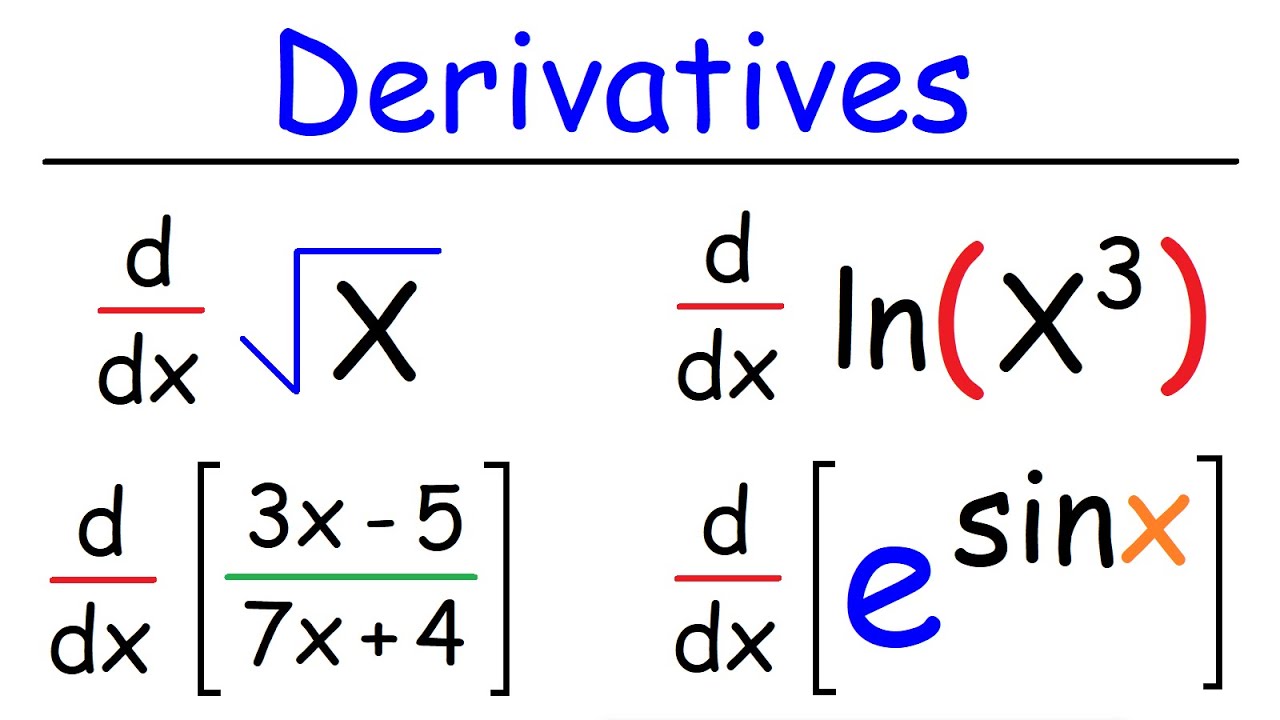Calculus 1 Final Review (Part 1) || Limits, Related Rates, Limit Definition of Derivative, Implicit
TLDRThis video script is a comprehensive review for a Calculus 1 final, covering key topics such as limits, derivatives, implicit differentiation, exponential growth and decay, related rates, linear approximations, and differentials. The review is structured to address specific problems, providing detailed explanations and solutions, with an emphasis on understanding the underlying concepts and applying them to various scenarios. The script also includes a brief introduction to Calculus 2 topics, promising a follow-up video that delves deeper into optimization and integrals.
Takeaways
- 📚 The video is a comprehensive review for a Calculus 1 final exam, covering key topics such as limits, derivatives, integrals, and applications.
- 🔢 The review begins with an explanation of non-infinite limits and progresses to more complex concepts like the definition of a derivative and implicit differentiation.
- 📈 The video emphasizes the importance of understanding the graphical representation of functions and the application of the squeeze theorem in evaluating certain limits.
- 🌐 The concept of continuity and different types of discontinuities are discussed, with examples demonstrating how to identify and handle them in functions.
- 🔄 The topic of horizontal and vertical asymptotes is introduced, with methods for determining their presence in functions explained.
- 🏋️♂️ A physics-based problem involving Newton's law of cooling is solved to illustrate the application of derivatives in real-world scenarios.
- 📚 The power rule, chain rule, and product rule for derivatives are reviewed, with an example provided to test understanding of these fundamental calculus rules.
- 🤝 The concept of implicit differentiation is explained, with a focus on solving for dy/dx when the relationship between x and y is not explicitly given.
- 📊 The video also tackles exponential growth and decay, specifically Newton's law of cooling, and provides a step-by-step guide to solving related problems.
- 📐 Linear approximations and differentials are introduced, with an explanation of their purposes and how to calculate them for given functions.
- 🎯 The video concludes with a summary of the topics covered and a预告 for part 2 of the review, which will delve into further applications of derivatives and integrals.
Q & A
What is the main topic of the video?
-The main topic of the video is a comprehensive review of Calculus 1 concepts, including limits, derivatives, integrals, and their applications.
What are the specific topics covered in the video?
-The video covers evaluating non-infinite limits, continuity, infinite limits, the limit definition of a derivative, implicit differentiation, exponential growth and decay rates, linear approximations, and differentials.
How does the video approach the concept of limits?
-The video approaches the concept of limits by starting with evaluating non-infinite limits and then moving on to continuity and infinite limits. It emphasizes the importance of one-sided limits and the conditions under which the limit from both sides might not match, leading to the limit not existing.
What is the significance of the squeeze theorem mentioned in the video?
-The squeeze theorem is significant as it provides a method to evaluate limits when the direct approach is not obvious. It involves finding two functions that bound the function of interest, and if the limits of these bounding functions as x approaches a certain value are equal, then the limit of the original function also equals that value.
How does the video explain the concept of discontinuities?
-The video explains discontinuities by discussing the behavior of functions at certain points. It differentiates between removable discontinuities, where the function can be modified to make it continuous at that point, and other types of discontinuities like jump discontinuities.
What is the role of the limit definition of a derivative in the video?
-The limit definition of a derivative is used in the video to calculate the derivative of a function at a specific point. It is particularly useful when the derivative cannot be found using standard rules like the power rule or chain rule.
How does the video address the concept of horizontal and vertical asymptotes?
-The video addresses horizontal and vertical asymptotes by explaining how to find them for a given function. For horizontal asymptotes, it discusses the limit of the function as x approaches infinity or negative infinity. For vertical asymptotes, it talks about the points where the denominator of a fraction equals zero and checks if these points lead to infinite values as x approaches these points.
What is the application of Newton's law of cooling discussed in the video?
-The application of Newton's law of cooling discussed in the video involves a cup of chocolate milk warming up from a refrigerator temperature to room temperature. The law is used to determine the rate at which the milk's temperature changes and to predict the temperature of the drink after a certain amount of time has passed.
How does the video handle related rates problems?
-The video handles related rates problems by using derivatives to find the rate of change of certain quantities with respect to time. It emphasizes the importance of identifying the correct quantities that are changing and applying the concept of related rates to find unknown rates.
What is the purpose of linear approximations as explained in the video?
-Linear approximations, as explained in the video, are used to simplify the calculation of square roots or other complex expressions. It involves finding a linear function that approximates the original function near a specific point, which can then be used to estimate values of the function.
How does the video explain the concept of differentials?
-The video explains differentials as a way to determine the change in the y-value of a function when there is a small change in the x-value. It involves taking the derivative of the function and multiplying it by the change in x (dx) to find the differential dy, which represents the approximate change in y.
Outlines
📚 Introduction to Calculus 1 Final Review
The video begins with an introduction to a two-part Calculus 1 final review series. The first part will cover topics such as limits, derivatives, and their applications, while the second part will focus on integrals and related concepts. The reviewer emphasizes the importance of understanding different types of limits, including one-sided and two-sided limits, as well as the definition of a derivative. They also mention that the timestamps for the video are provided for viewers who may want to focus on specific topics.
🔢 Evaluating Non-Infinite Limits
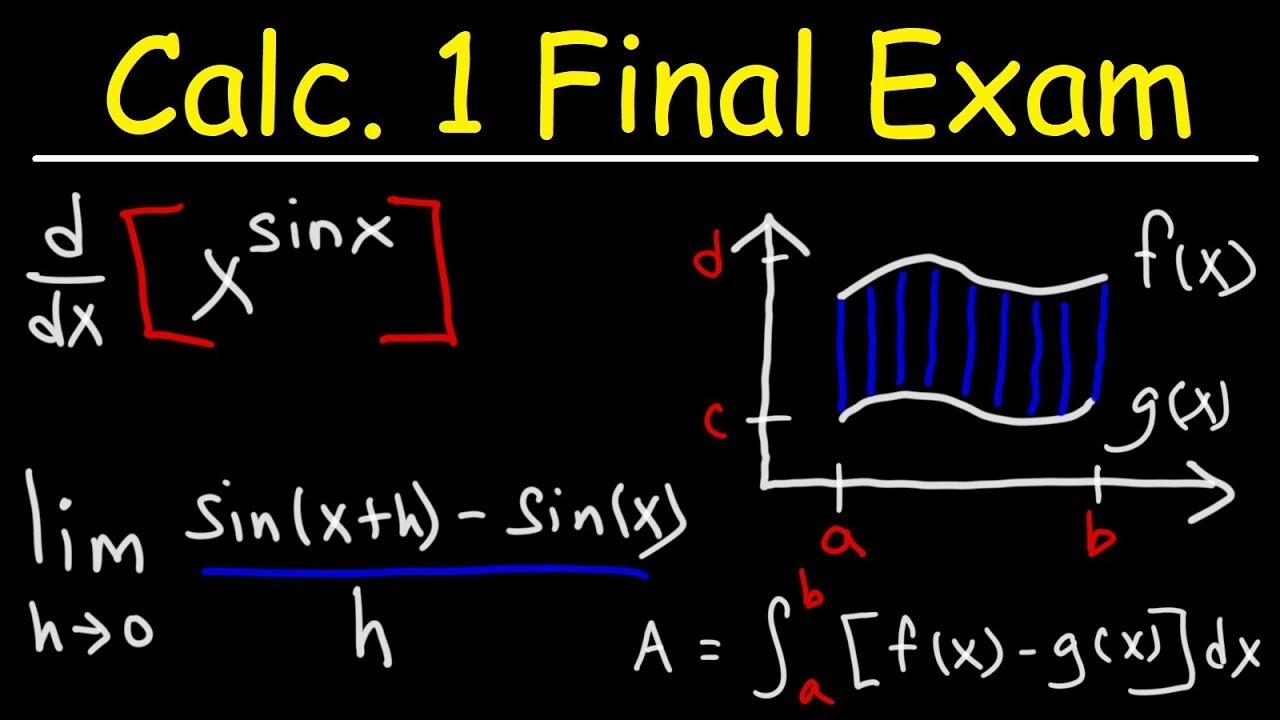
The reviewer discusses the process of evaluating non-infinite limits using a piecewise function as an example. They explain the importance of understanding the function's behavior from both the left and right sides and how to determine if a limit exists. The example given involves finding the limit as X approaches 2 for a function defined by an absolute value expression. The reviewer demonstrates how to calculate the one-sided limits and highlights what happens when the one-sided limits do not match, leading to the conclusion that the limit does not exist.
📈 Solving Trigonometric Limits
In this section, the reviewer addresses a common mistake made when calculating the limit of a trigonometric function as X approaches zero. They use the example of the limit of x squared times the sine of 1/x. The reviewer explains why simply taking the limit of each factor separately can lead to an incorrect conclusion and introduces the squeeze theorem as a method to properly evaluate such limits. They emphasize the importance of understanding the behavior of the sine function as X approaches zero and provide a graphical representation to illustrate the concept.
🔄 Continuity and Discontinuities
The reviewer discusses the concept of continuity in functions and how to identify different types of discontinuities. They use a piecewise function with a defined value at X equals zero to demonstrate how to check for continuity. The reviewer explains the process of finding one-sided limits and comparing them to the function's value at the point of interest to determine if there is a discontinuity. They identify the type of discontinuity as removable based on the function's behavior.
🔧 Modifying Functions for Continuity
The reviewer presents a problem involving a piecewise function that is not continuous at X equals 2 and explains how to modify the function to ensure continuity. They discuss the process of finding the limit from both sides of the discontinuity and using that information to redefine the function at the point of discontinuity. The reviewer demonstrates how to apply this process to a specific function and achieve a continuous function across the defined interval.
🌐 Infinite Limits without L'Hôpital's Rule
The reviewer challenges the viewer to understand and calculate infinite limits without using L'Hôpital's rule. They provide two examples involving polynomial functions and the square root of a polynomial function. The reviewer emphasizes the importance of developing an intuition for the behavior of these functions as X approaches infinity and understanding how the terms in the numerator and denominator interact to determine the limit. They explain how the higher-degree terms dominate the behavior of the function at infinity.
📊 Identifying Asymptotes
The reviewer explains how to find horizontal and vertical asymptotes of a function. They discuss the process of determining the horizontal asymptote by examining the behavior of the function as X approaches positive and negative infinity. For vertical asymptotes, the reviewer explains how to identify values of X that make the denominator zero and how to determine if these points result in infinite values for the function. They provide a step-by-step method for finding and verifying vertical asymptotes, emphasizing the importance of this concept for calculus exams.
🔢 Limit Definition of Derivative
The reviewer presents a problem that involves using the limit definition of a derivative to find the derivative of a function at a specific point. They explain the process of plugging in the point and taking the limit as h approaches zero. The reviewer demonstrates how to handle a radical in the numerator by multiplying by the conjugate and simplifying the expression to find the derivative. They also mention that this method can be used to verify the results obtained from other derivative rules.
🚀 Physics Problem: Newton's Law of Cooling
The reviewer provides a physics problem involving Newton's law of cooling. They explain the given function that describes the height of an object as a function of time and how to use the limit definition of a derivative to find the velocity of the object at a specific time. The reviewer also discusses how to find the time when the object will hit the ground and its final velocity. They emphasize the importance of understanding the concepts of initial and final velocities in the context of Newton's law of cooling.
📝 Derivatives and Implicit Differentiation
The reviewer presents a problem that involves taking the derivative of a product of functions using the product and chain rules. They provide a detailed explanation of the process, including handling the cube of the sine function and the exponential function. The reviewer emphasizes the importance of understanding the derivative rules and being able to apply them to more complex functions. They also introduce the concept of implicit differentiation, explaining how to solve for dy/dx when y is part of the equation.
📊 Exponential Growth and Decay
The reviewer discusses Newton's law of cooling in the context of an object heating up in a room, which is a slight variation of the typical cooling problem. They provide the formula for Newton's law of cooling and explain how to apply it to find the temperature of an object after a certain amount of time. The reviewer emphasizes the importance of understanding the formula and being able to apply it to solve problems involving exponential growth and decay.
🏃♂️ Related Rates
The reviewer presents a related rates problem involving two people moving apart from each other. They explain how to use Pythagorean theorem to relate the rates of change of different quantities and how to set up the equation to solve for the rate at which they are moving apart. The reviewer emphasizes the importance of drawing a diagram to visualize the problem and understanding the relationship between the rates of change of different quantities.
📊 Linear Approximations
The reviewer discusses the concept of linear approximations and how they can be used to approximate the value of a function. They provide a step-by-step explanation of how to find the linearization of a function and use it to estimate the value of the function at a specific point. The reviewer emphasizes the importance of understanding the formula for linear approximations and being able to apply it to solve problems.
📝 Differentials
The reviewer explains the concept of differentials and how they can be used to determine the change in a function's value as the input changes. They provide an example of finding the differential of a function and using it to estimate the change in the function's value. The reviewer emphasizes the importance of understanding the relationship between differentials and derivatives, and how differentials can be used to approximate function values.
🎓 Final Thoughts and Upcoming Content
The reviewer concludes the first part of the Calculus 1 final review by summarizing the topics covered and expressing hope that the video was helpful. They also provide a preview of the topics that will be covered in the second part of the review, including optimization, L'Hôpital's rule, and integral concepts. The reviewer encourages viewers to subscribe for more content and expresses well-wishes for their upcoming finals.
Mindmap
Keywords
💡Calculus
💡Limits
💡Derivatives
💡Integrals
💡Piecewise Functions
💡Optimization
💡Newton's Law of Cooling
💡Related Rates
💡Implicit Differentiation
💡Differentials
Highlights
The video is a comprehensive review for a Calculus 1 final exam, covering a wide range of topics including limits, derivatives, integrals, and applications.
The review begins with an explanation of evaluating non-infinite limits, emphasizing the importance of understanding one-sided limits and their implications on the existence of a limit.
A detailed discussion on the limit definition of a derivative is provided, highlighting the process of using the limit definition to find derivatives of functions.
The video explains the concept of horizontal and vertical asymptotes, and how to determine them for a given function, which is crucial for understanding the long-term behavior of functions.
The application of the squeeze theorem is demonstrated to evaluate limits that may not be easily computed through traditional methods, showcasing an alternative approach to problem-solving.
Implicit differentiation is discussed, with an example provided to illustrate how to solve for unknowns when the relationship between variables is not explicitly given.
Exponential growth and decay problems are tackled, including Newton's law of cooling, which has practical applications in various fields such as physics and engineering.
Related rates problems are explored, which involve calculating the rate of change of certain quantities based on given information and other changing variables.
The video also covers linear approximations, providing a method to approximate the behavior of functions near a specific point using linear equations.
Differentials are introduced, explaining how they can be used to estimate changes in the y-value of a function based on changes in the x-value.
The second part of the review will cover additional topics such as optimization, mean value theorem, L'Hopital's rule, and integration techniques up to substitution.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: