Logarithms explained Bob Ross style

Tibees
16 Sept 201908:56
EducationalLearning
32 Likes 10 Comments

TLDRIn this episode of 'The Joy of Mathematics,' Toby explains logarithms using the analogy of growing trees, simplifying the concept with examples of trees doubling or tripling in size each year. He emphasizes that while logarithms may seem intimidating, they are simply a different way to express exponential growth. Toby also discusses the restrictions of logarithms, noting they are undefined for bases of zero or one, and covers special types of logs like the common log (log base 10) and the natural log (ln). The episode encourages viewers to embrace logarithms as valuable tools for understanding both large and small numbers.

Takeaways
  • 🌳 The concept of logarithms is introduced using the analogy of trees growing in a forest, which can help to visualize and understand the concept.
  • πŸ“ˆ Logarithms can simplify the process of understanding exponential growth, such as a tree doubling in size each year.
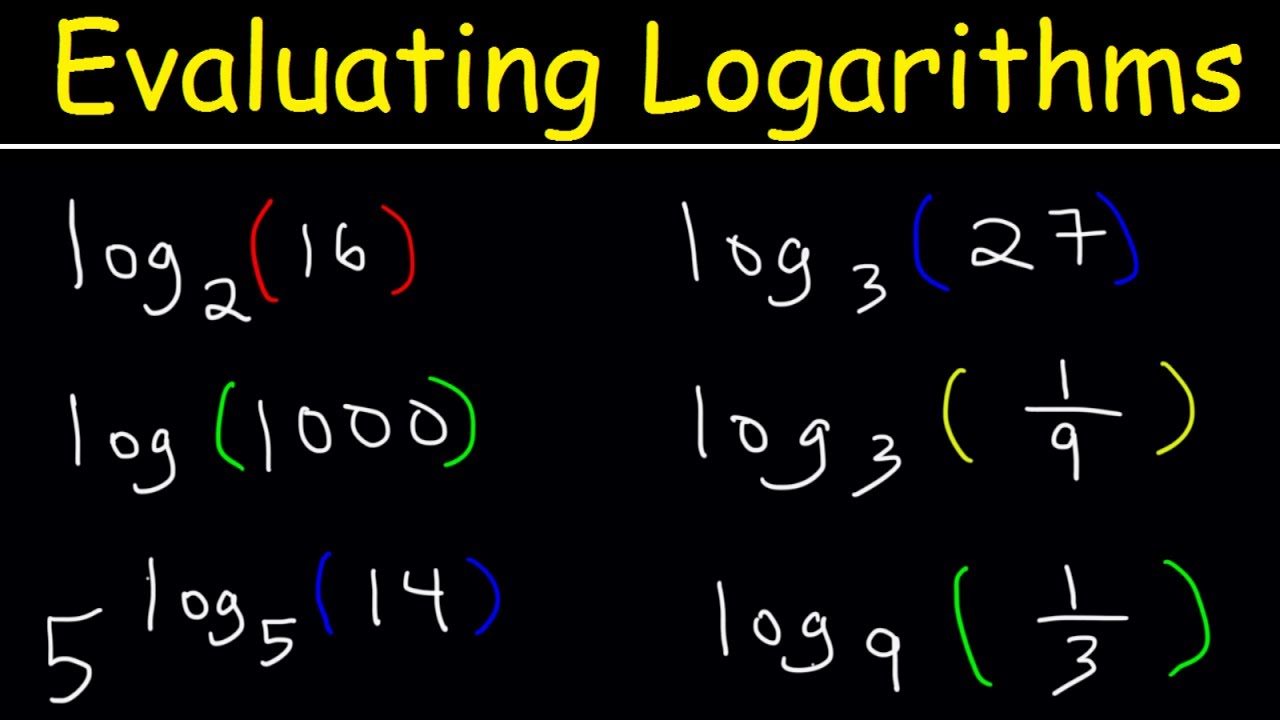
  • πŸ”’ The mathematical representation of exponential growth using logarithms is demonstrated, e.g., a tree doubling in size can be written as log base 2 of 16 equals 4.
  • 🌳 Trees are used as a metaphor for logarithms, where their growth represents the base, exponent, and argument of the logarithmic function.
  • πŸ“š Logarithms are often intimidating to students due to their unfamiliar notation, but with practice, they become easier to understand.
  • 🚫 The logarithm function is only defined for bases greater than zero and not equal to one, as certain base values lead to undefined results or involve complex numbers.
  • πŸ”„ The script emphasizes the importance of understanding the order of base, exponent, and argument in logarithmic expressions.
  • 🌲 A tree that is half its size every year can be represented using a negative exponent in logarithmic form, showing a reverse growth pattern.
  • ⏱️ The value of a logarithm can indicate the time parameter, such as a tree being half its size after one year, represented as log base 2 of 0.5 equals -1.
  • πŸ“ˆ Special logarithms like the natural logarithm (Ln) and common logarithm (log base 10) are mentioned, highlighting their applications in different mathematical contexts.
  • 🌳 The video concludes with an encouragement to not be intimidated by logarithms and to approach them with a positive attitude towards learning.
Q & A

  • What is the main topic of discussion in this episode of The Joy of Mathematics?

    -The main topic of discussion in this episode is logarithms, their understanding, and their application in different scenarios.

  • How does the speaker introduce the concept of logarithms in the script?

    -The speaker introduces the concept of logarithms by using the analogy of trees growing in size every year, demonstrating how logarithms can be used to calculate the growth rate and time.

  • What is the mathematical representation of a tree doubling in size every year for four years?

    -The mathematical representation is 2 to the power of 4 (2^4), which equals 16.

  • How can the concept of logarithms be written in a different form?

    -The concept of logarithms can be written in the form of log base 2 of 16 equals 4, showing the equivalent logarithmic form of the exponential expression.

  • What are the three components of a logarithmic expression?

    -The three components of a logarithmic expression are the base, the exponent, and the argument.

  • Why is the base of a logarithm function never equal to one?

    -The base of a logarithm function is never equal to one because any number to the power of one would result in the number itself, not the argument, making the logarithm undefined.

  • What happens if the base of a logarithm is zero or negative?

    -If the base of a logarithm is zero or negative, it results in undefined or imaginary numbers, which are not considered reliable and thus not included in the function.

  • How does the speaker explain the negative exponent in the context of the tree example?

    -The speaker explains that a negative exponent indicates a reduction in size. In the tree example, if a tree that doubles every year is half its size, it would be represented as 2 to the power of negative 1, which equals 0.5, so log base 2 of 0.5 is negative 1.

  • What is the significance of the natural logarithm (Ln) in mathematics?

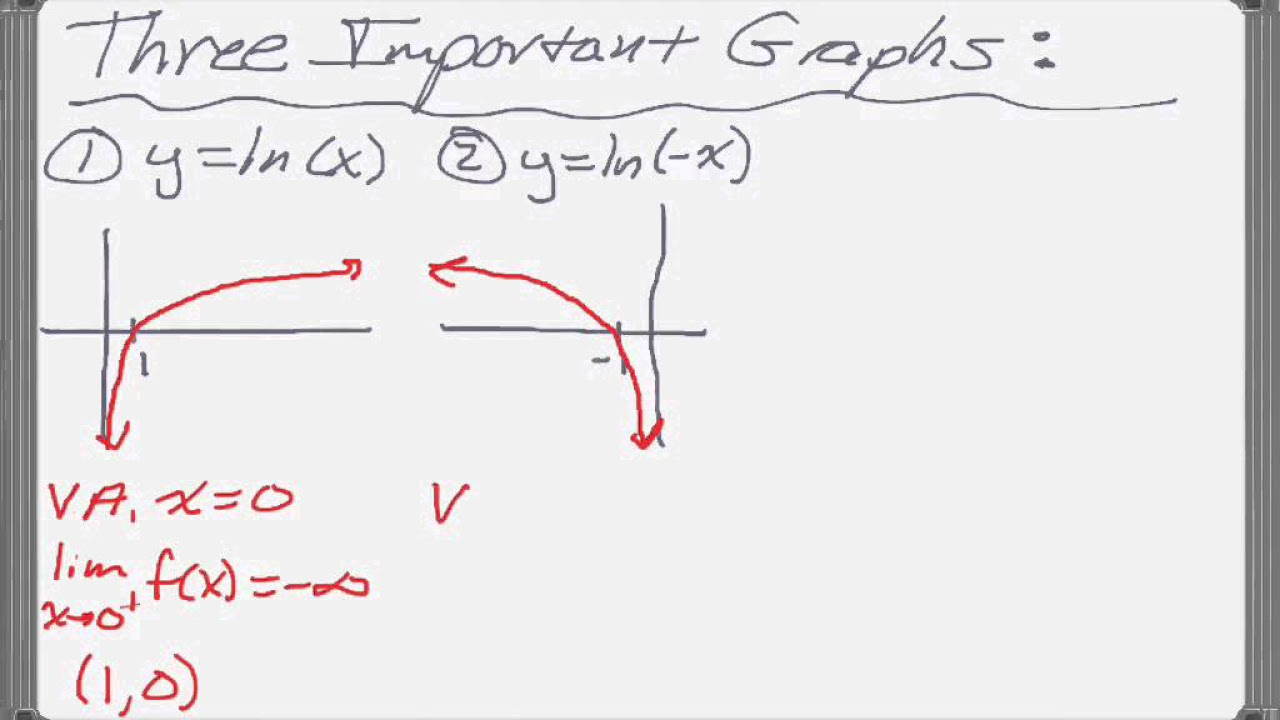
    -The natural logarithm (Ln) is the logarithm in base e, Euler's number, and it is significant because it appears in many cases of exponential growth and decay.

  • What is the default base of logarithm used in most calculators and why is it commonly used?

    -The default base of logarithm used in most calculators is 10, known as the common logarithm. It is commonly used because it aligns with the decimal system and is convenient for many practical calculations.

  • How does the speaker aim to change the viewer's perception of logarithms?

    -The speaker aims to change the viewer's perception of logarithms by demystifying them through relatable examples and analogies, encouraging viewers to see logs as useful tools rather than intimidating concepts.

Outlines
00:00
🌳 Introduction to Logarithms and Stress Relief

This paragraph introduces the topic of logarithms in a light-hearted manner, using the analogy of growing trees to explain the concept. The host, Toby, welcomes viewers to an episode of 'The Joy of Mathematics' and acknowledges that studying logarithms might be stressful. He uses the example of a tree doubling in size each year to illustrate exponential growth and introduces the logarithmic form of expressing this growth (log base 2 of 16 equals 4). Toby emphasizes that while logarithms may seem intimidating, they become easier with practice and are crucial for understanding large or small numbers.

05:01
πŸ“ˆ Understanding Logarithmic Functions and Their Limitations

The second paragraph delves deeper into the nature of logarithmic functions, discussing their limitations and special cases. Toby explains that logarithms are only defined when the base is greater than zero and not equal to one, as a base of one or zero would not yield a valid result. He uses the forest analogy to explore negative and fractional exponents, leading to imaginary numbers. The paragraph also touches on special logarithms like the common log (log base 10) and the natural log (ln of X), highlighting their relevance in exponential growth and decay scenarios. Toby encourages viewers to embrace logarithms and not let them be a source of intimidation.

Mindmap
Keywords
πŸ’‘Logarithms
Logarithms are mathematical operations that are the inverse of exponentiation, used to solve equations where the variable is an exponent. In the context of the video, logarithms are introduced as a way to understand the growth of trees, where the base of the logarithm corresponds to the rate of growth (e.g., doubling or tripling each year). The video simplifies the concept by using trees as a relatable example, making logarithms more approachable for the viewer.
πŸ’‘Stress
Stress is a state of mental or emotional strain resulting from adverse or demanding circumstances. In the video, stress is mentioned as a common feeling students experience when learning complex mathematical concepts like logarithms. The host aims to alleviate this stress by using a relaxed and friendly approach to explain the topic, using the metaphor of trees and forests to create a calming and relatable learning environment.
πŸ’‘Exponential Growth
Exponential growth refers to a pattern of growth where a variable increases at a rate that is proportional to its current value. In the video, this concept is illustrated through the metaphor of trees growing in size over time. The rate of growth (doubling or tripling) is used to explain how quickly the tree's size increases each year, demonstrating the principle of exponential growth in a tangible way.
πŸ’‘Base
In the context of logarithms and exponential growth, the base is the number that is used as the foundation for the multiplication in the repeated multiplication process. The base is a crucial element in understanding how fast something grows or changes. In the video, the base is represented by the rate at which the tree grows (e.g., doubling or tripling each year), which is used to calculate the logarithm.
πŸ’‘Exponent
An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. In the context of the video, the exponent represents the multiplication factor or the power to which the base is raised to achieve a certain growth. The exponent in logarithms is the unknown variable that we solve for to find out how many times the base must be multiplied by itself to reach a certain value.
πŸ’‘Argument
In the context of logarithms, the argument is the value that the logarithm is evaluated to. It is the result of the exponentiation process. The argument is the number that, when the base is raised to its power, equals the result of the logarithmic expression. In the video, the argument is used to represent the final size or growth factor of the tree after a certain number of years.
πŸ’‘Natural Logarithm
The natural logarithm, denoted as ln or log_e, is a logarithm with the base of the mathematical constant e (approximately 2.71828). It is called 'natural' because it is closely related to the concept of growth and decay in nature. The natural logarithm is a fundamental tool in various fields of mathematics, science, and engineering, and it is often used in calculations involving exponential functions.
πŸ’‘Logarithmic Form
Logarithmic form is a way of expressing an equation where the variable is the exponent in an exponential equation. It is the inverse form of writing an exponential equation, which makes it useful for solving problems where the result of an exponential operation is known, but the exponent (or the power) is what needs to be found.
πŸ’‘Time Parameter
The time parameter in the context of the video refers to the number of years it takes for a tree to grow to a certain size based on a specific growth rate. It is the unknown variable in the logarithmic equation that, when solved, gives the duration of growth. The time parameter is a crucial concept in understanding how logarithms can be used to model real-world situations, such as the growth of trees over time.
πŸ’‘Negative Exponent
A negative exponent indicates the reciprocal of the base raised to the absolute value of the exponent. It is used in the context of the video to explain how a tree that is half its size each year can be modeled mathematically. Negative exponents are a way to represent division in the context of exponential expressions.
πŸ’‘Common Logarithm
The common logarithm, often denoted as log or log_10, is a logarithm with a base of 10. It is widely used in many scientific and mathematical calculations, especially when dealing with orders of magnitude or when the data is best represented on a logarithmic scale. The common logarithm is a standard function on most calculators and is frequently used in engineering, physics, and other scientific disciplines.
Highlights

The Joy of Mathematics podcast introduces logarithms in a relatable way, using the example of growing trees.

Stress relief is suggested through visualization, imagining stress melting away into tree leaves.

A logarithm is explained as a simpler way to express exponential growth, using the example of a tree doubling in size each year.

Mathematically, the doubling tree example is shown as 2^4 = 16, demonstrating the concept of exponentiation.

The logarithmic form of the doubling tree example is introduced as log base 2 of 16 equals 4.

The podcast emphasizes that logarithms can seem intimidating but become easier with familiarity.

A second tree example is given where the tree triples in size each year, and it would take 3 years to become 27 times larger.

The concept of base, exponent, and argument in logarithms is explained using the tree example.

A mnemonic is provided to remember the order of base, exponent, and argument in logarithms using a log with an anti-clockwise swirl.

The limitations of logarithms are discussed, such as being undefined with a base of 1 or dealing with negative numbers or fractions.

The concept of a 'time-traveling tree' is introduced to explain negative exponents in logarithms.

The natural logarithm (Ln of X) and its relation to Euler's number is briefly mentioned as another form of logarithm.

The default common logarithm (log base 10) is noted as a frequently used form in mathematics.

The podcast concludes by encouraging listeners to not be intimidated by logarithms and to enjoy their study of mathematics.

Transcripts

Browse More Related Video

Calc AB & Calc BC Natural Logs Review

Using Logarithms and Natural Logarithms in Chemistry

Deriving Natural Logarithms!

Logarithms - The Easy Way!

How to Change the Base of a Logarithm (Precalculus - College Algebra 61)

Derivative of log_x (for any positive base a­1) | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Math EducationLogarithm ConceptsStress ReliefForest MetaphorToby's TeachingExponential GrowthMath AnxietyLogarithmic FunctionsEuler's NumberStudy Tips