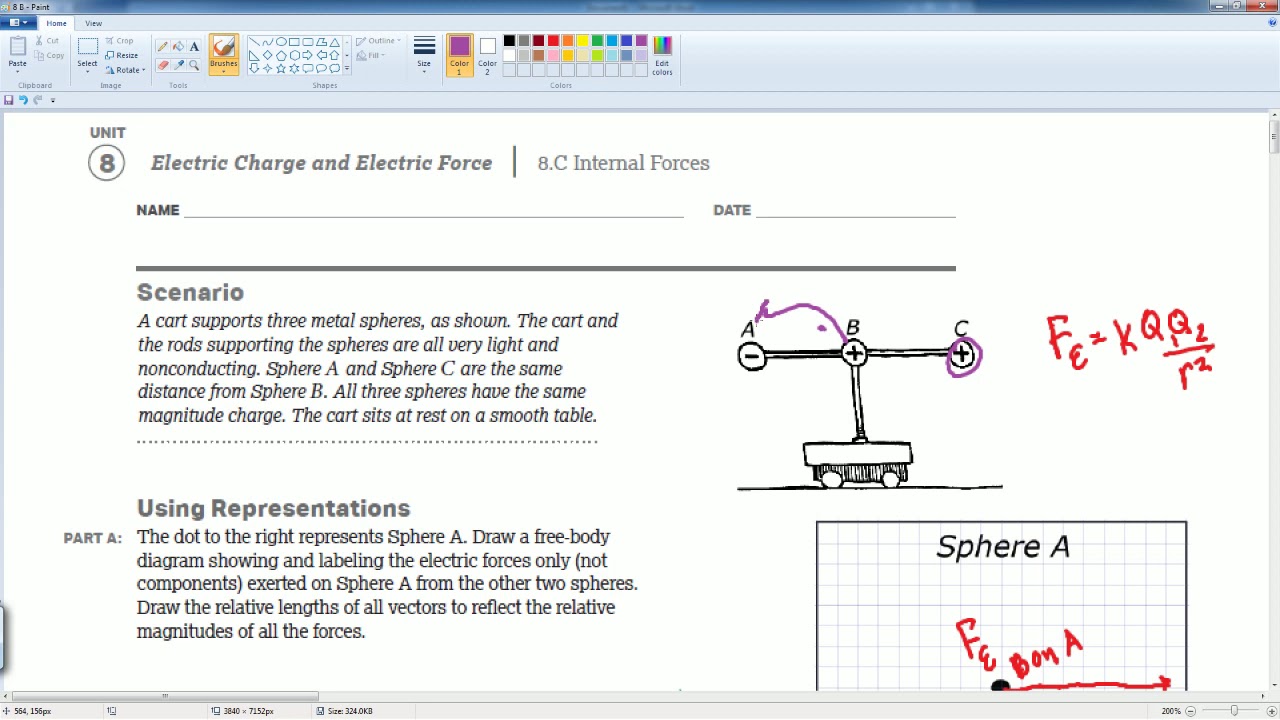
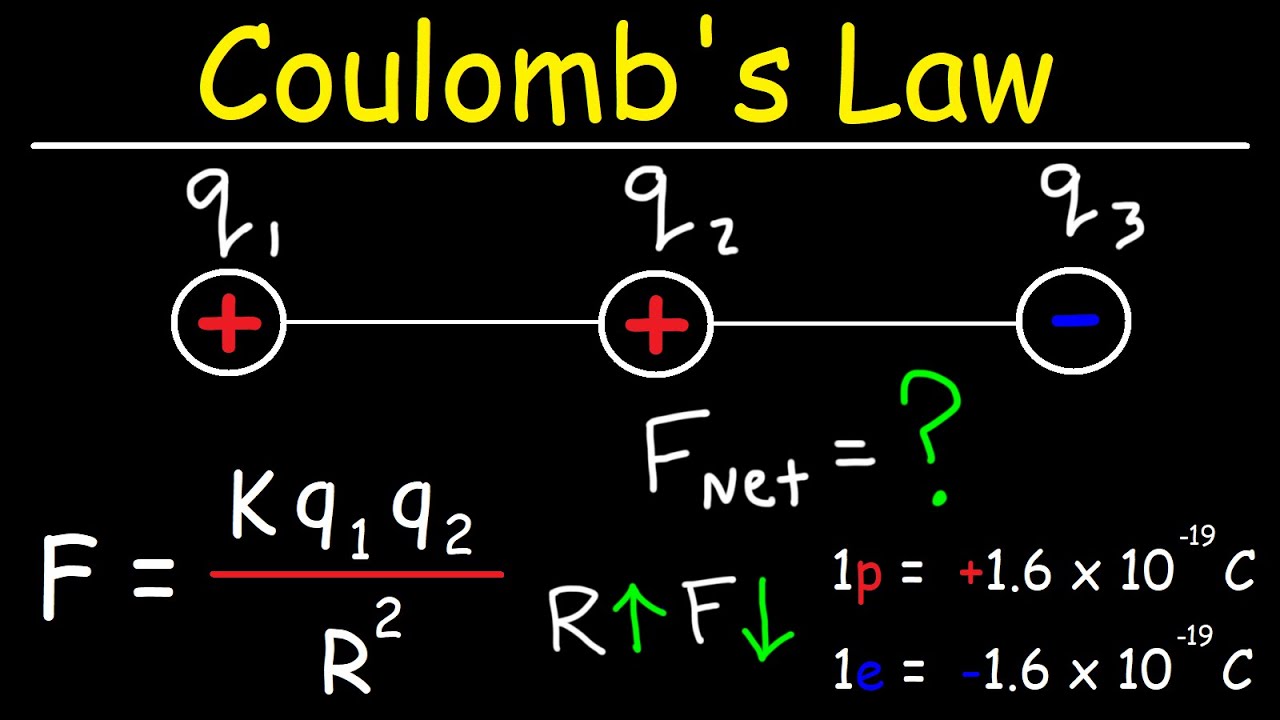
Coulomb's Law Problems

Physics Ninja
15 Feb 202019:18
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging video, the host, Physics Ninja, demonstrates the application of Coulomb's Law to two distinct problems involving point charges. The first problem involves calculating the net force on a negatively charged particle (Q3) due to its interactions with two other charges (Q1 positive and Q2 negative) on the x-axis. The second problem extends the analysis to a two-dimensional scenario in the XY plane. Through step-by-step explanations and clear illustrations, the video effectively shows how to determine the magnitude and direction of the forces, and ultimately, the net force acting on Q3 in both cases.

Takeaways
  • 📚 The video discusses the application of Coulomb's Law to find the net force acting on charges in two different scenarios.
  • 🔋 Coulomb's Law is given as K * Q_A * Q_B / R^2, where K is Coulomb's constant, Q_A and Q_B are the charges, and R is the distance between them.
  • 🎯 In the first scenario, three point charges are placed on the x-axis, and the goal is to find the net force on Q_3, which is a negative charge.
  • 🤔 To solve the problem, calculate the force between each pair of charges and then determine the net force by considering the direction and magnitude of each force.
  • 🚦 The direction of the force between positive and negative charges is attractive, while between like charges it is repulsive.
  • 📐 For the second problem, the charges are placed in a two-dimensional configuration, requiring the use of vector components to find the net force.
  • 📍 The magnitude of the force is calculated using Coulomb's Law, and the direction is determined by the nature of the charges involved.
  • 🔄 When dealing with multiple charges, the net force is found by adding the individual forces acting on the charge of interest.
  • 📊 The video provides a step-by-step demonstration of how to calculate the forces and net force in both one-dimensional and two-dimensional scenarios.
  • 🎓 The importance of understanding the direction of forces and how to break down vector quantities into their components is emphasized.
  • 🌟 The video also explains how to calculate the magnitude and direction of the net force using trigonometric functions and the Pythagorean theorem.
  • 💡 The video concludes by summarizing the process of applying Coulomb's Law to find the net force on a charge in complex configurations.
Q & A

  • What is the main goal of the first problem discussed in the transcript?

    -The main goal of the first problem is to find the net force acting on charge Q3, given three point charges Q1, Q2, and Q3 on the x-axis, where Q1 is positive, Q2 is negative, and Q3 is also negative.

  • What is Coulomb's law and how is it applied to find the force between two charges?

    -Coulomb's law is a formula that describes the electrostatic force between two point charges. It is given by the equation F = k * (QA * QB) / R^2, where F is the force between the charges, k is Coulomb's constant, QA and QB are the magnitudes of the charges, and R is the distance between the centers of the two charges. It is applied by substituting the known values of the charges and distance into the formula to calculate the force.

  • How does the direction of the force between two charges depend on the signs of the charges?

    -The direction of the force between two charges depends on whether the charges are of the same or opposite signs. If the charges are of the same sign (both positive or both negative), they repel each other, and the force is directed away from the other charge. If the charges are of opposite signs (one positive and one negative), they attract each other, and the force is directed towards the other charge.

  • What are the values of the charges Q1, Q2, and Q3 in the first problem?

    -In the first problem, Q1 is a positive charge with a value of 5 microcoulombs (5 * 10^-6 C), Q2 is a negative charge with a value of -3 microcoulombs (-3 * 10^-6 C), and Q3 is a negative charge with a value of -2 microcoulombs (-2 * 10^-6 C).

  • How is the net force on Q3 calculated in the first problem?

    -The net force on Q3 is calculated by first determining the individual forces acting on Q3 due to its interactions with Q1 and Q2 using Coulomb's law. Then, these forces are added vectorially, taking into account their directions and magnitudes, to find the resultant net force.

  • What is the result of the net force acting on Q3 in the first problem?

    -In the first problem, the net force acting on Q3 is found to be 1.1 Newtons in the direction to the right, after considering the attractive force from Q1 to the left and the repulsive force from Q2 to the right.

  • How does the second problem differ from the first one?

    -The second problem differs from the first in that the charges are placed in a two-dimensional configuration (XY plane) instead of along the x-axis. This requires the application of vector components and trigonometry to find the net force acting on Q3.

  • What is the distance calculation for the force F1 in the second problem?

    -The distance for force F1 in the second problem is calculated using the Pythagorean theorem, with one side being 0.4 meters and the other side being 0.2 meters, resulting in a distance d1 of approximately 0.45 meters.

  • How are the forces broken down into components in the second problem?

    -In the second problem, each force is broken down into its X and Y components using trigonometry. The X component is calculated as the magnitude of the force times the cosine of the angle, and the Y component is the magnitude of the force times the sine of the angle.

  • What is the net force on Q3 in the second problem, and in which direction is it acting?

    -The net force on Q3 in the second problem has a magnitude of approximately 1.2 Newtons and is acting at an angle of approximately 71.2 degrees from the horizontal axis, in the direction where the component forces add up to the greatest effect.

  • What is the significance of the angle theta in the second problem?

    -The angle theta in the second problem is the angle between the force F1 and the positive X-axis. It is used to determine the X and Y components of the force F1, which are essential for calculating the net force acting on Q3 in the two-dimensional configuration.

  • How can the angle alpha be determined in the second problem?

    -The angle alpha, which is the direction of the net force, can be determined using the components of the net force. It is found by taking the inverse tangent (arctangent) of the ratio of the Y component to the X component of the net force.

Outlines
00:00
🔬 Applying Coulomb's Law to Charges on the X-axis

This paragraph introduces the application of Coulomb's law to solve for the net force acting on a charge, Q3, located among other charges, Q1 and Q2, on the x-axis. The speaker aims to find the force on Q3 considering the interactions between the charges. Coulomb's law is expressed as K * Q_A * Q_B / R^2, where K is Coulomb's constant, Q_A and Q_B are the charges, and R is the distance between them. The speaker explains the concept of attractive and repulsive forces between like and unlike charges and sets up the problem to calculate the magnitude and direction of the forces using the law.

05:01
📐 Calculating the Magnitude of Forces in a 2D Configuration

In this paragraph, the speaker transitions from a 1D scenario to a 2D one, maintaining the focus on the net force acting on charge Q3. The speaker labels the forces acting on Q3 and applies Coulomb's law to calculate the magnitude of the forces between the charges. The calculation involves using the Pythagorean theorem to find the distance between the charges in the 2D plane. The speaker emphasizes the need to consider the direction of the forces, as this will be crucial for determining the net force in the upcoming steps.

10:04
📊 Breaking Down Forces into Components and Calculating Net Force

The speaker now focuses on breaking down the force F1 into its X and Y components using trigonometry, specifically tangent, to find the angle between the forces and the axes. The calculation involves finding the magnitude of F1 and F2 and their respective components. The speaker then proceeds to find the net force by adding up the components in their respective directions. The process involves using the Pythagorean theorem to find the magnitude of the net force and trigonometric functions to determine its direction.

15:05
🎯 Finalizing the Net Force Calculation and Visual Representation

In the final paragraph, the speaker completes the net force calculation by summing up the components of the forces in both the X and Y directions. The magnitude and direction of the net force are determined using the Pythagorean theorem and trigonometric tangent function. The speaker also provides a clear visual representation of the net force, its magnitude, and direction, which concludes the problem-solving process. The explanation is aimed at giving the audience a comprehensive understanding of how to apply Coulomb's law in both 1D and 2D scenarios and how to calculate and represent the net force acting on a charge due to interactions with other charges.

Mindmap
Keywords
💡Coulomb's Law
Coulomb's Law is a fundamental principle in physics that describes the electrostatic force between two point charges. It states that the force is directly proportional to the product of the magnitudes of the charges and inversely proportional to the square of the distance between them. In the video, the presenter applies Coulomb's Law to calculate the net force acting on a charge in two different scenarios, first on the x-axis and then in a two-dimensional plane. The law is used to determine the magnitude and direction of the forces between charges, which is crucial for solving the problems presented.
💡Point Charges
Point charges are an idealized model in physics where a charge is considered to be concentrated at a single point in space. This simplification allows for easier calculation of electric fields and forces. In the video, the charges Q1, Q2, and Q3 are treated as point charges, and their interactions are analyzed based on their positions and magnitudes. The concept of point charges is essential for applying Coulomb's Law in the given problems.
💡Electrostatic Force
Electrostatic force is the force that arises from the interaction between charged particles. It can be either attractive or repulsive, depending on the nature of the charges involved. In the video, the presenter calculates the electrostatic forces between different pairs of point charges using Coulomb's Law. Understanding this force is key to determining the net force acting on a charge in an electrostatic field.
💡Net Force
Net force, also known as the resultant force, is the vector sum of all the individual forces acting on an object. It is a crucial concept in understanding the overall effect of different forces. In the video, the net force acting on charge Q3 is calculated by considering the individual forces exerted by Q1 and Q2. The net force determines the overall force experienced by the charge and its direction.
💡Vector Quantities
Vector quantities are physical quantities that have both magnitude and direction. In the context of the video, forces are vector quantities, and their components can be represented in a coordinate system. The presenter breaks down the forces into their x and y components to find the net force acting on Q3 in a two-dimensional scenario. Understanding vector quantities is essential for solving problems involving direction and magnitude of forces.
💡Distance
Distance is a fundamental concept in physics, often used to describe the separation between two points. In the context of the video, the distance between charges is critical for applying Coulomb's Law, as the electrostatic force is inversely proportional to the square of the distance. Accurate calculation of distances is necessary to determine the magnitude of the forces between charges.
💡Direction
Direction refers to the course along which a force is acting or a vector is pointing. In the video, the direction of the forces is important for determining the net force on a charge. The direction is determined based on the nature of the charges (positive or negative) and is used to calculate the components of the forces in a two-dimensional plane.
💡Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. In the video, trigonometry is used to break down the forces into their x and y components, which allows the presenter to calculate the net force in a two-dimensional scenario. The use of trigonometric functions like cosine and sine is essential for determining the direction and magnitude of the forces.
💡Components
In physics, components refer to the individual parts of a vector quantity that can be represented along the axes of a coordinate system. The components of a force determine its effect in each direction. In the video, the presenter calculates the x and y components of the forces acting on Q3, which are then used to find the net force. Understanding components is crucial for analyzing forces in two or three dimensions.
💡Pythagorean Theorem
The Pythagorean Theorem is a fundamental principle in geometry that relates the lengths of the sides of a right-angled triangle. It states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In the video, the Pythagorean Theorem is used to calculate the distance between two charges in a two-dimensional scenario, which is necessary for applying Coulomb's Law.
💡Newton's Laws of Motion
Newton's Laws of Motion are three fundamental principles that describe the relationship between a body and the forces acting upon it, and its motion in response to those forces. While not explicitly mentioned in the video, the concept of force, as discussed, is central to Newton's Second Law, which states that the force acting on an object is equal to the mass of the object multiplied by its acceleration. The net force calculated in the video is an example of how forces can influence the motion of an object, in accordance with Newton's Laws.
Highlights

Introduction to applying Coulomb's law to find the net force on a charge.

Explanation of the first problem involving three point charges on the x-axis.

Discussion on the interaction between positive and negative charges and their attraction.

Step-by-step illustration of calculating the force between two charges using Coulomb's law.

Clarification on the units required for calculating force using Coulomb's law.

Description of the forces acting on charge Q3 due to its interactions with Q1 and Q2.

Methodology for finding the magnitude of force F1 using the given charges and distance.

Explanation of how to calculate the net force on charge Q3 in a two-dimensional scenario.

Use of Pythagorean theorem to determine the distance between charges in a two-dimensional problem.

Breakdown of a vector force into its X and Y components using trigonometry.

Process of finding the net force by adding vector components in the X and Y directions.

Calculation of the magnitude and direction of the net force using the components.

Visual representation of the net force and its components on a vector diagram.

Explanation of the second problem with charges in a different configuration in the XY plane.

Demonstration of how the same principles apply when calculating forces in two dimensions.

Conclusion summarizing the application of Coulomb's law to multiple charges and the method for finding net forces.

Transcripts

Browse More Related Video

Coulomb's Law (7 of 7) Force on Three Charges Arranged in a Right Triangle

AP Physics Workbook 8.B Electric Force

AP Physics Workbook 8.C Internal Force

Introduction to Coulomb's Law or the Electric Force

Calculating the Electric Force

Coulomb's Law - Net Electric Force & Point Charges

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Coulomb's LawPhysics TutorialElectric ChargesForce CalculationVector QuantitiesProblem SolvingElectrostaticsAttraction and RepulsionTwo-Dimensional ForcesPhysics Education