Introduction to Coulomb's Law or the Electric Force
TLDRIn this educational transcript, students learn about Coulomb's Law, which describes the electric force between two charged particles. The discussion includes comparisons to Newton's universal law of gravitation, the significance of the Coulomb constant, and the concept of point charges. The class also delves into the calculation of electric force, understanding the difference between attractive and repulsive forces, and the application of vector concepts in determining net forces on charged objects.
Takeaways
- 📚 Coulomb's Law, named after French physicist Charles Augustin de Coulomb, quantifies the electric force between two charged particles.
- 🔧 The formula for the electric force is F_e = k * q1 * q2 / r^2, where F_e is the force, k is the Coulomb constant, q1 and q2 are the charges, and r is the distance between the centers of charge.
- 🌐 The structure of Coulomb's Law is similar to Newton's universal law of gravitation, with both forces being inversely proportional to the square of the distance between two objects.
- 🔄 The Coulomb constant (8.99 × 10^9 N m^2/C^2) is significantly larger than the universal gravitational constant (6.67 × 10^-11 N m^2/kg^2), indicating that electric forces are generally stronger than gravitational forces.
- 💡 The magnitude of the electric force between two point charges can be either attractive or repulsive, depending on the nature of the charges (opposite charges attract, like charges repel).
- 📌 Point charges are theoretical constructs with zero size and a specific electric charge, useful for simplifying calculations in electrostatics.
- 🔢 The negative sign in the electric force calculation indicates the direction of the force (attractive or repulsive) rather than a physical direction like left or down.
- 🔧 When calculating electric forces, it's crucial to ensure that the units of charge are consistent with the units used in the Coulomb constant (Newtons, meters, and Coulombs).
- 📐 The net force on a charge is determined by considering all individual forces acting on it and summing them vectorially.
- 🔍 The concept of electric force and its calculation is fundamental to understanding electrostatics and the behavior of charged particles in various settings.
Q & A
What is Coulomb's Law and who is it named after?
-Coulomb's Law is an equation that describes the magnitude of the electric force between two charged particles. It is named after Charles Augustin de Coulomb, a French physicist who published several papers detailing his experimental determination of this law in 1785.
What are the similarities between Coulomb's Law and Newton's universal law of gravitation?
-Both Coulomb's Law and Newton's law of universal gravitation share a similar equation form, where the force between two objects is proportional to the product of their respective properties (charges or masses) and inversely proportional to the square of the distance between them.
What does the distance 'r' represent in Coulomb's Law?
-In Coulomb's Law, 'r' represents the distance between the centers of charge of the two charged objects, not their centers of mass.
What are the units of the Coulomb constant and the universal gravitational constant?
-The Coulomb constant is measured in Newtons times meters squared per Coulomb squared, while the universal gravitational constant is measured in Newtons times meters squared per kilogram squared.
How does the magnitude of the Coulomb constant compare to the universal gravitational constant?
-The Coulomb constant is significantly larger than the universal gravitational constant. Specifically, it is about 1.35 times 10^20 times larger.
What is the significance of the negative sign in the electric force calculated using Coulomb's Law?
-The negative sign in the electric force calculation indicates that the force is an attractive force when the charges have opposite signs. A positive sign would indicate a repulsive force when the charges are the same.
What is a point charge and how does it relate to the concept of a point mass?
-A point charge is an idealized object that has zero size and carries an electric charge. It is analogous to a point mass, which is an idealized object with mass but no size, used in physics to simplify problems involving gravitational forces.
What are microcoulombs, nanocoulombs, and picocoulombs, and how do they relate to coulombs?
-Microcoulombs, nanocoulombs, and picocoulombs are units of electric charge. One microcoulomb is 10^-6 coulombs, one nanocoulomb is 10^-9 coulombs, and one picocoulomb is 10^-12 coulombs.
How is the net force on a positive charge in the middle of two like charges determined?
-The net force on a positive charge in the middle is the vector sum of the forces exerted on it by the two like charges. Since both forces are repulsive and in the same direction, their magnitudes add up algebraically.
What is the significance of the direction in the context of electric forces?
-The direction is crucial in determining whether the electric force is attractive or repulsive. An attractive force is indicated by a negative value, while a repulsive force is indicated by a positive value in the calculations.
How does the presence of a third charge affect the electric forces on the original two charges?
-The presence of a third charge adds an additional force to the system. The net force on one of the original charges will be the vector sum of the forces from the other two charges, taking into account both magnitude and direction.
What is the relationship between the electric force and Newton's third law in the context of the charges?
-According to Newton's third law, for every action, there is an equal and opposite reaction. This means that for every force exerted by charge one on charge two, there is an equal but opposite force exerted by charge two on charge one.
Outlines
📚 Introduction to Coulomb's Law and Electric Force
This paragraph introduces the concept of Coulomb's Law, which governs the magnitude of electric force between two charged particles. It draws a comparison with Newton's universal law of gravitation, highlighting the similarities in their mathematical forms. The Coulomb constant and universal gravitational constant are introduced, with a discussion on their relative magnitudes. The concept of point charges and the difference between the forces of gravity and electric forces are also explained. Additionally, the paragraph clarifies the units of electric charge, such as microcoulombs, and their conversion to coulombs for calculations.
🔧 Solving Electric Force Problems and Understanding Negative Results
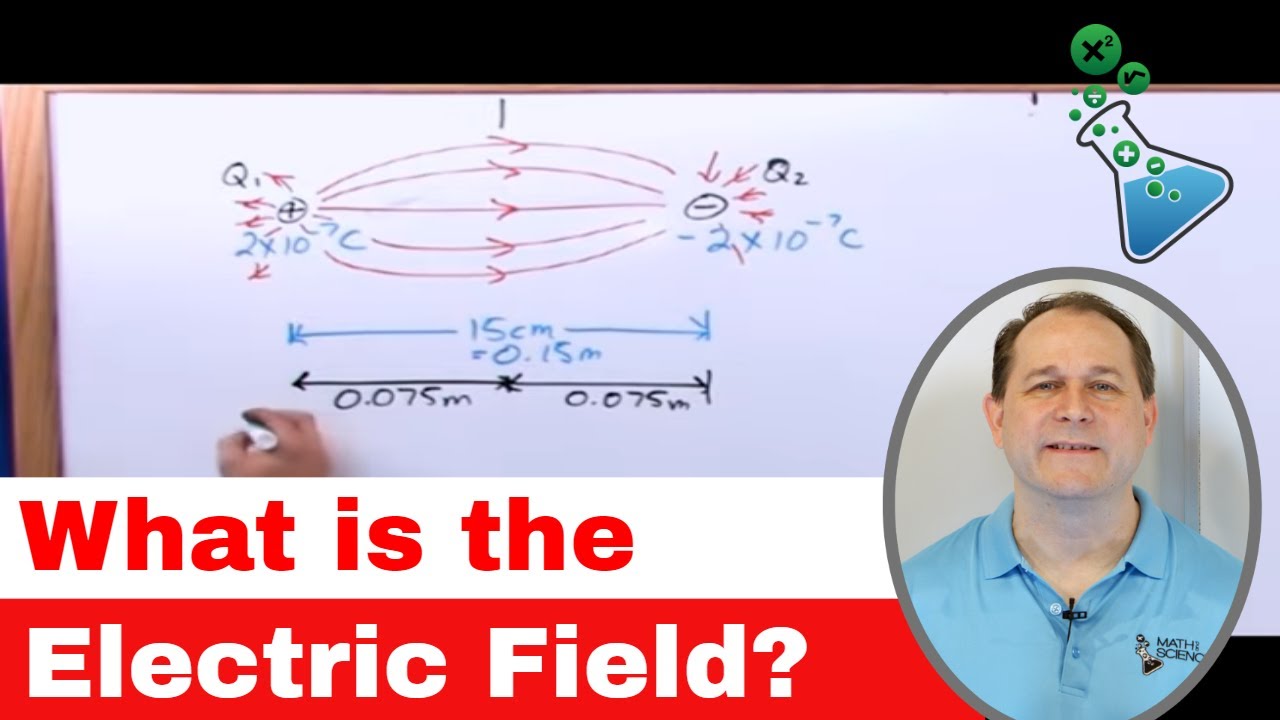
The second paragraph focuses on solving a basic example problem involving the calculation of electric force between two equal magnitude point charges with opposite signs. It emphasizes the importance of unit consistency when using the Coulomb constant. The paragraph also addresses the concept of negative results in electric force calculations, explaining that a negative force indicates an attractive force, while a positive force indicates a repulsive force. The discussion extends to the vector nature of electric forces and the application of Coulomb's law in determining the net force on a charge due to multiple charges.
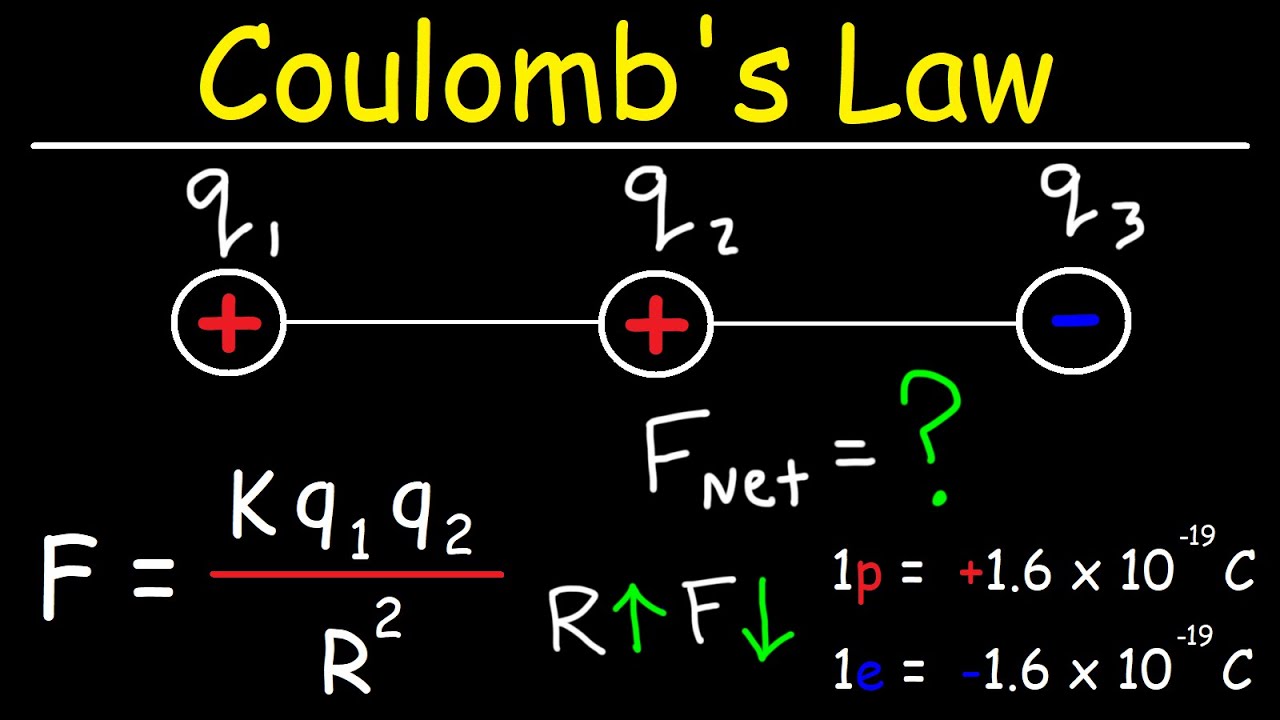
🔄 Analyzing the Net Force with Multiple Charges
This paragraph delves into a scenario where a third charge is introduced, and the net force acting on one of the original charges is calculated. It explains how the introduction of a third charge with the same sign as one of the original charges affects the net force. The calculation demonstrates how to determine the individual forces from each charge and combine them to find the resultant force. The explanation reinforces the understanding of how electric forces can be either attractive or repulsive, depending on the charges involved.
Mindmap
Keywords
💡Coulomb's Law
💡Electric Force
💡Charges
💡Coulomb's Constant
💡Distance (r)
💡Newton's Universal Law of Gravitation
💡Point Charges
💡Microcoulombs (μC)
💡Unit Vector
💡Net Force
💡Attractive and Repulsive Forces
Highlights
Introduction to Coulomb's Law and its significance in physics.
Historical context of Coulomb's Law, named after French physicist Charles Augustin de Coulomb.
Equation of Coulomb's Law and its components: electric force (F_e), charges (q1, q2), and distance (r).
Comparison of Coulomb's Law to Newton's universal law of gravitation in terms of mathematical form.
Explanation of the distance r as the distance between the centers of charge, not mass.
Discussion of the Coulomb constant (k) and its magnitude relative to the universal gravitational constant (G).
Example of how electric force can overcome gravitational force, as demonstrated with a balloon and hair.
Clarification on the concept of point charges and their idealization in physics.
Explanation of the prefixes micro (μ), nano (n), and pico (p) and their relation to Coulombs.
Solution of an example problem involving two equal magnitude point charges and the calculation of electric force.
Discussion on the importance of unit consistency when applying Coulomb's Law.
Explanation of the negative sign in Coulomb's Law indicating attractive or repulsive forces.
Illustration of Newton's third law in the context of electric forces between charges.
Analysis of the net force on a charge when additional like charges are introduced.
Conclusion emphasizing the understanding of electrostatic force and its practical implications.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: