HOW TO CHOOSE CHAIN RULE vs. PRODUCT RULE | Calculus Derivatives

INeedToPassCalculus
14 Jun 201806:33
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial focuses on differentiating between the chain rule and product rule when calculating derivatives in calculus. It emphasizes the importance of recognizing whether the components of a function can stand alone or are part of a composition, which is key to selecting the correct rule. The video walks through eight problems, illustrating how to identify the 'glue' that holds the function pieces together – either multiplication for the product rule or nesting for the chain rule. The clear examples provided make it easier for viewers to grasp the concept and apply it to various derivative problems.

Takeaways
  • 📚 The video focuses on practicing the decision-making process between the chain rule and product rule in calculus for derivatives.
  • 🤔 It emphasizes the challenge of choosing the correct rule when multiple rules are involved in a test setting.
  • 🔍 The script outlines a method for determining the rule: assessing if the components of a function can stand alone.
  • 📈 For problem one, the function involves x squared and sine x, which are standalone functions, indicating the use of the product rule.
  • 🧩 In problem two, the combination of cosine and e to the X is a composition of functions, not standalone, thus suggesting the chain rule.
  • 🔢 Problem three involves three X to the fourth and Ellen, where the latter is not standalone, pointing to a nested function and the chain rule.
  • 🌟 Problem four has twelve X and e to the X, both standalone, which means they are multiplied together, indicating the product rule.
  • 📝 Problem five's notation, with parentheses, doesn't change the nature of the function, which is a nesting (chain rule).
  • 📊 In problem six, x squared and Ln X are both standalone, but their multiplication indicates the product rule.
  • 🚫 Problem seven warns against being tricked by a number in front of the function; the main function is e to the 12x, which is a chain rule case.
  • 🔍 The final problem, number eight, involves Ln and minus Ln X, where the latter is standalone, and the former is not, indicating the chain rule.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is to practice choosing between the chain rule and the product rule for calculus derivatives.

  • Why can it be difficult to decide which rule to use in a derivatives test?

    -It can be difficult because the test may combine a variety of rules, unlike quizzes that focus on a single topic, making it challenging to determine the correct rule to apply.

  • How can you determine if the product rule should be used for a given function?

    -You should check if the two main pieces of the function can stand alone as their own functions. If they can, and they are glued together with multiplication, then the product rule should be used.

  • In what scenario would you use the chain rule according to the video?

    -You would use the chain rule when both parts of the function cannot stand alone as their own functions, indicating a composition of functions or nested functions.

  • What is the first problem's function in the video?

    -The first problem's function is x squared multiplied by sine x (x^2 * sin(x)), where the product rule should be applied.

  • How is the second problem's function structured in the video?

    -The second problem's function is cosine multiplied by e to the power of x (cos(x) * e^x), and the chain rule should be applied here.

  • What is the hint that a problem requires the chain rule in the video?

    -The hint is when neither part of the function can stand alone as their own function, indicating a composition or nesting of functions.

  • How does the video suggest approaching problem three?

    -Problem three should be approached by recognizing that the main parts are (3x^4) and (e^(xln(x))) indicating a chain rule due to the nested functions.

  • What is the significance of the number 7 in problem seven?

    -In problem seven, the number 7 is a constant multiplier and does not affect which derivative rule to use. It simply multiplies the result of the derivative of the function e^(12x).

  • How does the video describe the function for the last problem, problem eight?

    -The function for problem eight is ln(x) - ln(x) * ln(x), where the chain rule should be applied because ln(x) without any variables is not a standalone function, indicating nesting.

  • What is a key takeaway from the video for identifying the correct rule?

    -A key takeaway is to check if the parts of the function can stand alone and how they are combined. If they are glued by multiplication and can stand alone, use the product rule. If not, and there is a composition or nesting, use the chain rule.

Outlines
00:00
📚 Understanding Derivative Rules: Chain vs. Product

This paragraph introduces the challenge of selecting between the chain rule and the product rule in calculus when dealing with derivatives. It emphasizes the difficulty in identifying the correct rule during tests where multiple rules are applied. The speaker guides the audience through eight practice problems, encouraging them to determine whether the chain rule or product rule should be used. The explanation begins with problem one, which involves a product of two functions, x squared and sine x, and proceeds to problem two, which is a composition of functions, cosine and e to the x, indicating the use of the chain rule. Each problem is dissected to reveal the nature of the functions involved and how they interact, ultimately guiding the decision on which rule to apply.

05:01
🔍 Analyzing Function Composition and Derivative Rules

The second paragraph delves deeper into the analysis of function composition and how it affects the choice of derivative rules. It explains how to identify standalone functions and how their combination indicates the use of either the chain rule or product rule. The paragraph covers problems three to eight, each with unique scenarios that test the understanding of function composition and the application of derivative rules. It highlights the importance of recognizing when functions are nested (chain rule) or simply multiplied (product rule). The explanation is enriched with examples, such as the treatment of constants in function differentiation and the differentiation of functions involving natural logarithms. The paragraph concludes with a clear guideline on differentiating between the chain and product rules, reinforcing the concepts learned.

Mindmap
Keywords
💡Chain Rule
The Chain Rule is a fundamental concept in calculus used to find the derivative of a composite function. It states that the derivative of a function composed of two or more functions is the product of the derivative of the outer function evaluated at the inner function, and the derivative of the inner function. In the video, the Chain Rule is used when the function is a composition of other functions, such as in problem two where the function involves the cosine function and an exponential function e to the X.
💡Product Rule
The Product Rule is a calculus formula used to find the derivative of a product of two functions. It states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. In the video, the Product Rule is applied when the given function is a multiplication of two standalone functions, as seen in problem one with x squared and sine X.
💡Derivatives
Derivatives are a core concept in calculus that represent the rate of change or the slope of a function at a particular point. They are used to analyze the behavior of functions, such as determining maximum and minimum values, rates of change, and the shape of curves. In the video, the focus is on practicing the calculation of derivatives using the Chain Rule and Product Rule.
💡Standalone Functions
A standalone function is a function that is complete and can exist independently. In the context of the video, determining whether the parts of a given function are standalone is crucial for deciding whether to use the Chain Rule or the Product Rule. If the parts can exist on their own, the Product Rule is often applicable; if not, the Chain Rule may be needed.
💡Composition of Functions
Composition of functions refers to the process of combining two or more functions to create a new function. In the context of the video, identifying when a function is composed of other functions is essential for applying the correct derivative rule. The Chain Rule is typically used when dealing with composite functions.
💡Nesting of Functions
Nesting of functions occurs when one function is used as an input for another function, creating a hierarchical structure. This concept is critical for recognizing when to use the Chain Rule, as it often signifies that the functions are nested within each other.
💡Quizzes and Tests
Quizzes and tests are assessment tools used to evaluate knowledge and understanding. In the context of the video, they refer to the challenges faced when applying different rules for calculating derivatives, such as the Chain Rule and Product Rule.
💡Autopilot
The term 'autopilot' in the context of the video refers to a state of mind where one can automatically apply a rule or concept without much thought, often due to repetitive practice. It is used to describe the comfort level students might feel when dealing with problems that only require one type of rule.
💡Decision Making
Decision making is the process of choosing between alternatives, often based on certain criteria or rules. In the video, it refers to the process of determining whether to use the Chain Rule or the Product Rule when calculating derivatives.
💡Practice
Practice involves repeating or engaging in an activity to improve or maintain skills and knowledge. In the video, practice is encouraged as a means to become proficient in distinguishing between the Chain Rule and the Product Rule for calculating derivatives.
💡Mathematical Notation
Mathematical notation is the system of symbols and rules used to write mathematics. It includes various ways of representing functions, equations, and operations. In the video, different notations are used to express the same mathematical concepts, such as parentheses to denote the Chain Rule.
Highlights

The video focuses on practicing the decision-making process between the chain rule and product rule in calculus derivatives.

It's common to know which rule to use when problems are repetitive, but tests combining different rules can be challenging.

For problem one, if the function pieces can stand alone, it's a product rule scenario.

Problem two indicates that if both parts of a function can't stand alone, it hints at using the chain rule.

In problem three, the presence of non-standalone functions suggests a chain rule due to nested functions.

Problem four is a product rule derivative because both parts are standalone functions multiplied together.

Problem five shows that the chain rule applies when there's a nesting of functions, even with parentheses altering the appearance.

For problem six, the presence of a full ln function alongside a standalone function indicates a product rule.

In problem seven, a number in front of a function doesn't affect the derivative rule, focusing on the function's core.

Problem eight highlights that a non-standalone part in a function suggests the use of the chain rule.

The video provides a methodical approach to identifying the correct derivative rule by analyzing the structure of the function.

The chain rule is typically used for compositions of functions, whereas the product rule is for multiplication of functions.

Standalone functions are key in determining whether to use the product rule or the chain rule.

The video emphasizes the importance of understanding the composition and structure of functions to apply the correct calculus rule.

The chain rule is identified by nested functions, while the product rule is indicated by standalone functions in multiplication.

The video serves as a practical guide for students to improve their calculus problem-solving skills.

The ability to discern between the chain rule and product rule is crucial for success in higher-level calculus.

The video's examples illustrate the application of calculus rules in a clear and understandable manner.

By practicing with various problems, students can develop an intuitive sense for choosing the right derivative rule.

Transcripts

Browse More Related Video

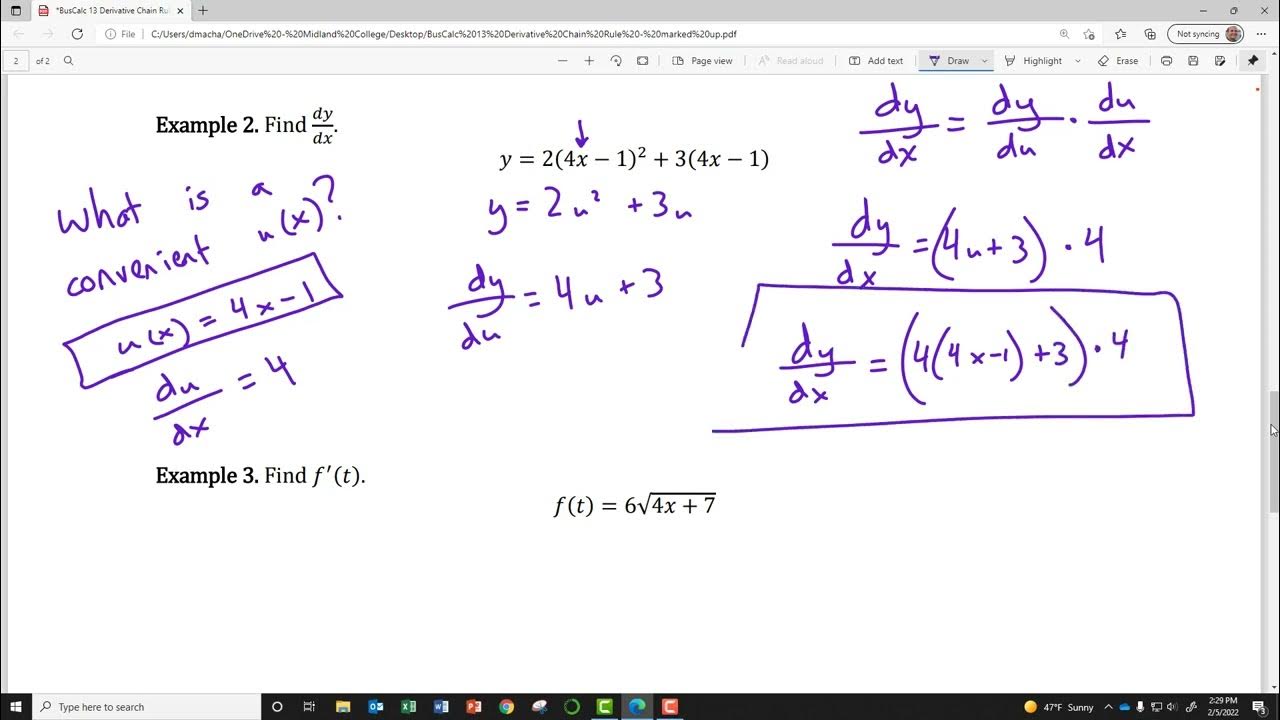
BusCalc 13 Derivative Chain Rule

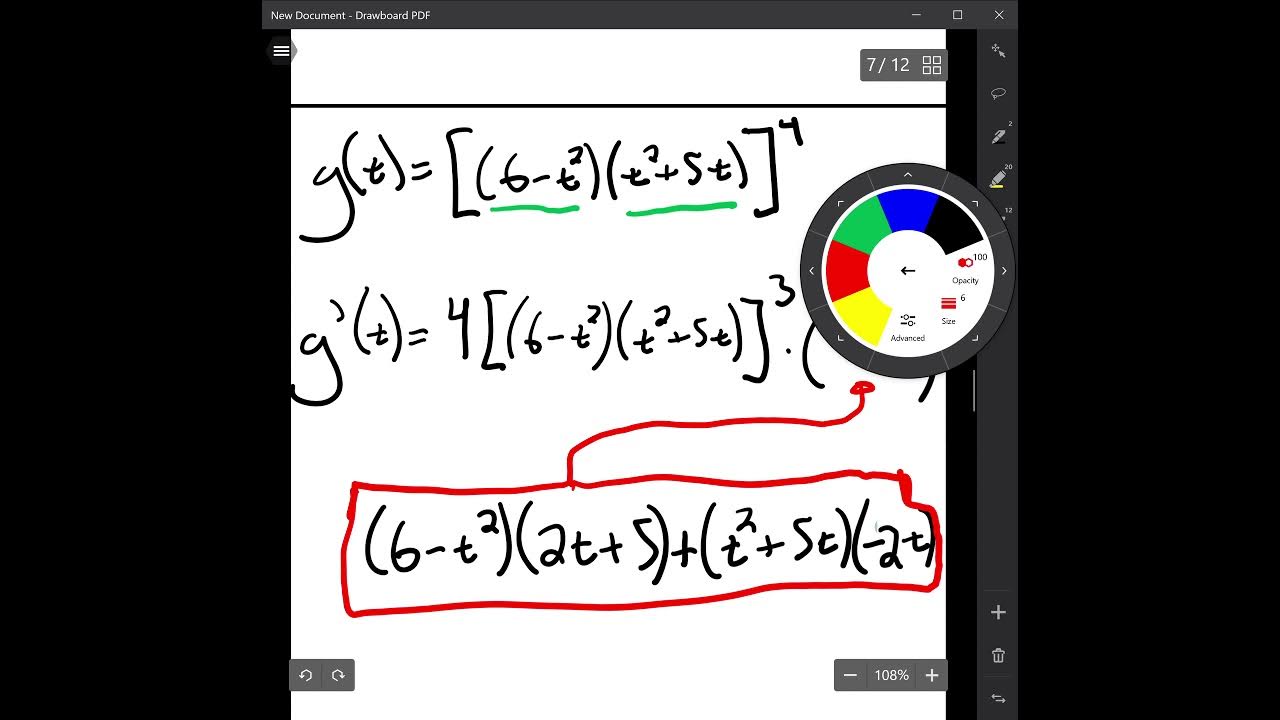
Derivatives with Multiple Rules

More Chain Rule (NancyPi)

Derivatives of Composite Functions - Chain Rule, Product & Quotient Rule

Manipulating functions before differentiation | Derivative rules | AP Calculus AB | Khan Academy

Business Calculus - Math 1329 - Section 2.4 - Chain Rule

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusTipsDerivativeRulesMathematicsEducationChainRuleProductRuleQuizzesExamPreparationFunctionAnalysisComposingFunctionsStandaloneFunctions