Second derivative test | Using derivatives to analyze functions | AP Calculus AB | Khan Academy

Khan Academy
26 Jul 201606:12
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the second derivative test, a method for determining the nature of a critical point on a function's graph. It explains that when a function's first derivative is zero and the second derivative is negative, the function has a relative maximum at that point. Conversely, if the second derivative is positive, the function has a relative minimum. The script uses visual examples to build intuition and concludes with an example to demonstrate how the test is applied.

Takeaways
  • 📈 The second derivative test is used to determine the nature of a critical point of a function, such as whether it is a maximum, minimum, or inconclusive.
  • 🔍 At a local maximum, the first derivative (slope of the tangent line) is zero, and the second derivative is negative, indicating concave down.
  • 🔍 At a local minimum, the first derivative is zero, and the second derivative is positive, indicating concave up.
  • 🤔 If the second derivative is zero, the test is inconclusive, and we cannot determine if the critical point is a maximum, minimum, or neither.
  • 📊 The second derivative test applies to twice differentiable functions, where both the first and second derivatives are defined in the neighborhood of the critical point.
  • 🌐 The test provides an intuitive understanding of the function's concavity around the critical point, which helps in identifying the nature of the point.
  • 📌 The slope of the tangent line at a critical point is horizontal (zero slope) as it is the point where the function changes from increasing to decreasing or vice versa.
  • 📉 A negative second derivative indicates that the function is concave down and has a relative maximum at that point.
  • 📈 A positive second derivative indicates that the function is concave up and has a relative minimum at that point.
  • 🌟 The second derivative test is a powerful tool in calculus that enhances our ability to analyze the behavior of functions and their critical points.
  • 📚 Understanding the second derivative test is crucial for anyone studying calculus as it builds upon the first derivative test and further deepens the understanding of function properties.
Q & A

  • What is the main purpose of the second derivative test?

    -The main purpose of the second derivative test is to determine whether a critical point of a function is a relative maximum, relative minimum, or neither.

  • What is the intuitive understanding of the second derivative test?

    -The intuitive understanding of the second derivative test is that it uses the concavity of a function around a critical point to determine if the point is a maximum, minimum, or neither. If the second derivative is less than zero, the function is concave down and has a relative maximum. If the second derivative is greater than zero, the function is concave up and has a relative minimum.

  • What conditions must a function meet to use the second derivative test?

    -For the second derivative test to be applicable, the function must be twice differentiable over the interval of interest, and the first derivative must be zero at the critical point.

  • What does a positive second derivative at a critical point indicate?

    -A positive second derivative at a critical point indicates that the function is concave up at that point, suggesting a relative minimum value.

  • What does a negative second derivative at a critical point indicate?

    -A negative second derivative at a critical point indicates that the function is concave down at that point, suggesting a relative maximum value.

  • What does a zero second derivative at a critical point mean?

    -A zero second derivative at a critical point is inconclusive; it does not provide enough information to determine whether the point is a maximum, minimum, or neither.

  • How does the second derivative test relate to the slope of the tangent line?

    -The second derivative test relates to the slope of the tangent line in that the first derivative being zero indicates the slope of the tangent line is zero at the critical point. The second derivative then provides information about the rate of change of the slope, which helps determine the concavity and nature of the critical point.

  • In the example given, what is the value of H(8)?

    -In the example, the value of H(8) is 5.

  • What is the first derivative of the function H at x=8?

    -The first derivative of the function H at x=8 is 0.

  • What is the second derivative of the function H at x=8?

    -The second derivative of the function H at x=8 is -4.

  • Based on the given information, what type of critical point does the function H have at x=8?

    -Based on the given information, the function H has a relative maximum point at x=8.

Outlines
00:00
📈 Introduction to the Second Derivative Test

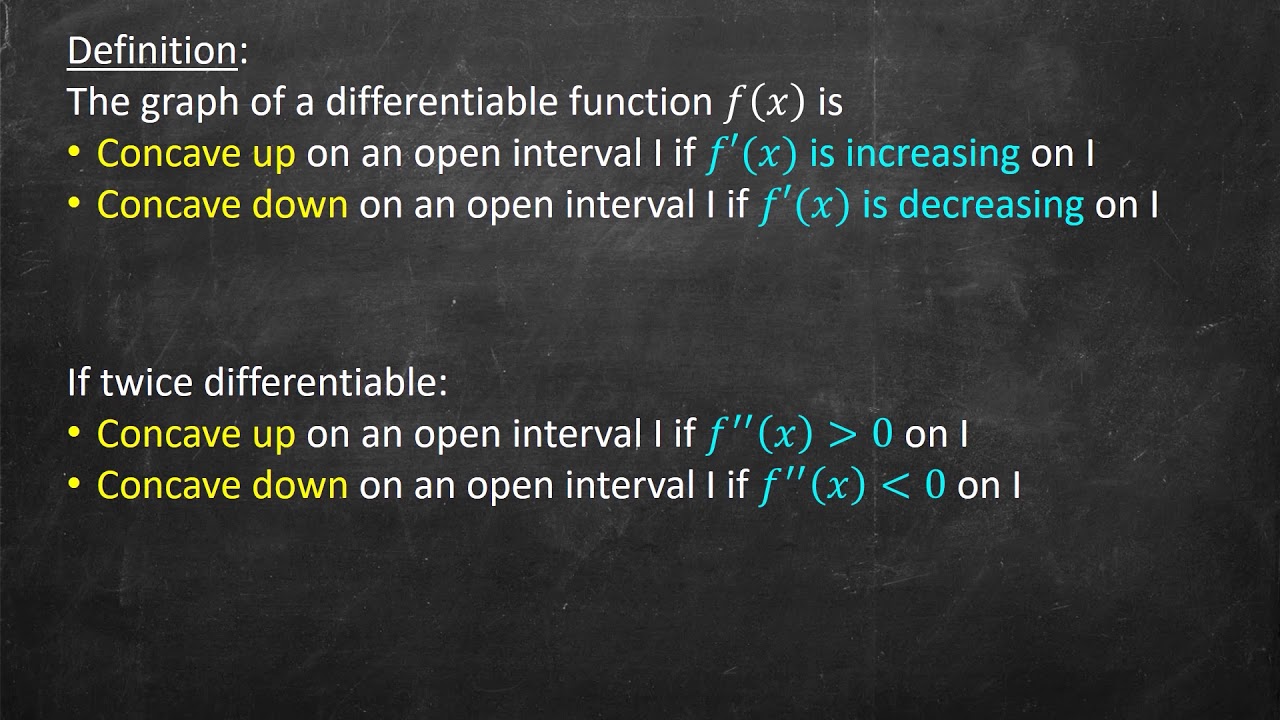
This paragraph introduces the concept of the second derivative test, aiming to provide an intuitive understanding rather than a rigorous mathematical proof. It begins by visually representing a function with a relative maximum at point C, where the first derivative (slope of the tangent line) is zero and the function is concave downward, indicating a negative second derivative. The explanation then contrasts this with a situation where the function has a relative minimum at point C, where again the first derivative is zero, but the function is concave upward, suggesting a positive second derivative. The paragraph establishes the basic intuition that the sign of the second derivative can indicate the nature of a critical point: negative for a relative maximum, positive for a relative minimum, and zero leading to an inconclusive result. The explanation is supported with a visual representation of the function and its derivatives.

05:05
🧠 Applying the Second Derivative Test to a Given Function

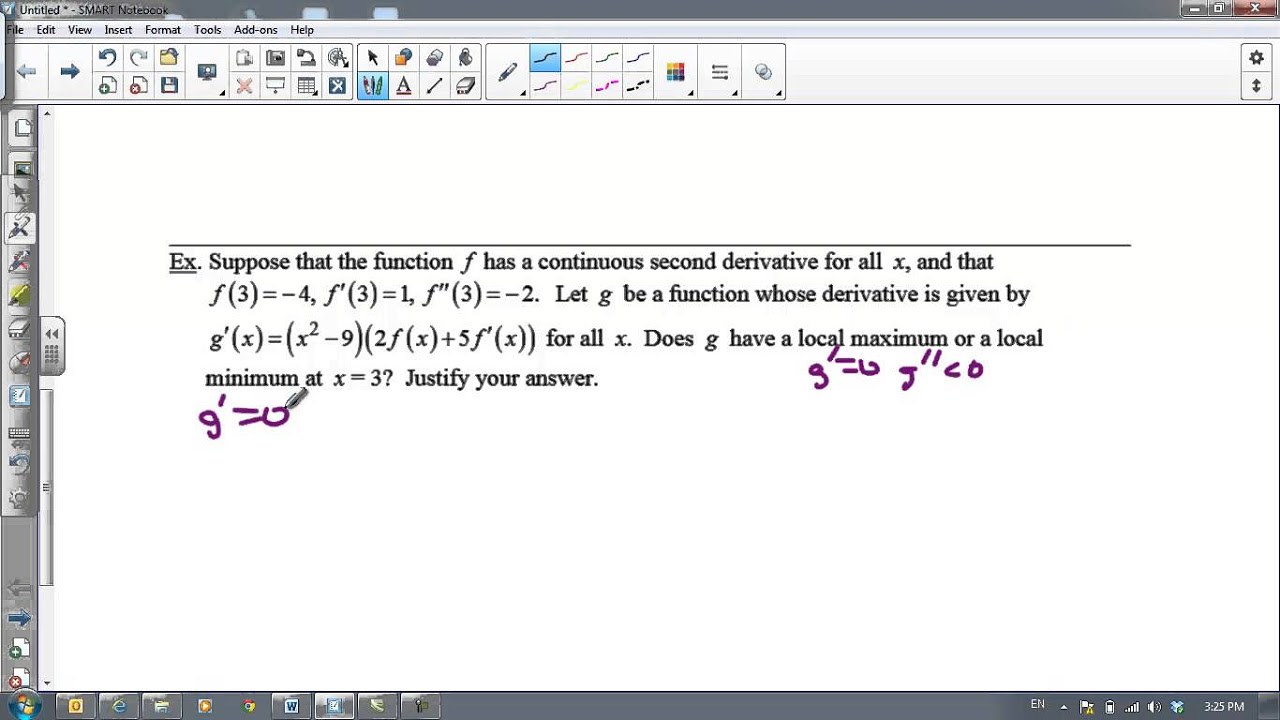
This paragraph applies the second derivative test to a specific example involving a twice differentiable function H. The example provides values for the function, its first derivative, and second derivative at a point where the first derivative is zero. The paragraph guides the viewer through the process of determining whether the point is a relative maximum, minimum, or if the information is inconclusive based on the second derivative's value. It reinforces the concept that a negative second derivative at a critical point indicates a relative maximum, while a positive second derivative indicates a relative minimum. The paragraph concludes by emphasizing the importance of the second derivative in identifying the nature of critical points and encourages the viewer to pause and reflect on the example to solidify their understanding.

Mindmap
Keywords
💡Second Derivative Test
The Second Derivative Test is a method used in calculus to determine the nature of a critical point of a function. It involves analyzing the second derivative of the function to ascertain whether the critical point is a local maximum, local minimum, or neither. In the video, this concept is central to understanding how the shape of a function's graph can reveal its behavior at certain points. For instance, if the second derivative at a critical point is less than zero, the function has a local maximum at that point, as illustrated by the example where the second derivative of function H at x=8 is negative four, indicating a relative maximum at the point (8,5).
💡Critical Point
A critical point in calculus is a point on the graph of a function where the derivative is either zero or undefined. These points are significant because they often represent potential local maximum or minimum values of the function. In the context of the video, the speaker is examining critical points to determine their nature using the second derivative test. For example, the function F has a critical point at X=C, where F'(C) is zero and the graph shows a local maximum.
💡Tangent Line
In the context of the video, a tangent line is a straight line that touches the graph of a function at a specific point without crossing it. The slope of the tangent line at any point on a curve is equal to the derivative of the function at that point. The video uses the concept of the tangent line to discuss the slope at critical points and how it relates to the concavity of the function, which in turn helps determine whether the critical point is a maximum or minimum.
💡Concavity
Concavity is a property of a function that describes the curvature of its graph. A function is said to be concave up if the graph curves above the tangent lines at all points, and concave down if it curves below the tangent lines. In the video, the speaker uses the concept of concavity to help determine the nature of critical points using the second derivative test. If a function is concave up around a critical point, it indicates a local minimum, while concave down suggests a local maximum.
💡Local Maximum/Minimum
A local maximum or minimum is a point on the graph of a function where the function reaches a highest or lowest value in its neighborhood, respectively. These are important concepts in calculus as they help identify the behavior of a function's graph. In the video, the speaker is particularly interested in using the second derivative test to determine whether a critical point is a local maximum or minimum. For example, a local maximum is indicated when the second derivative is less than zero at the critical point, as seen with the function H at x=8.
💡Slope
The slope of a line is a measure of its steepness, and in the context of the video, it is used to describe the rate of change of a function at a particular point. The slope is calculated as the derivative of the function, and it provides insight into whether the function is increasing or decreasing at that point. The video uses the concept of slope to discuss the first derivative test and how it relates to the second derivative test in determining the nature of critical points.
💡Derivative
A derivative in calculus is a measure of how a function changes as its input changes. It is the slope of the function at any given point and gives information about the rate at which the function is increasing or decreasing at that point. The first derivative is used to find critical points, which are then further analyzed using the second derivative to determine if they are local maxima or minima. The video emphasizes the importance of understanding both the first and second derivatives in using the second derivative test.
💡Twice Differentiable
A function is said to be twice differentiable if it can be differentiated twice, meaning that both the first and second derivatives of the function exist. This is an important property for the second derivative test because it requires the existence of both the first and second derivatives to determine the nature of a critical point. In the video, the speaker assumes that the function under consideration is twice differentiable, which allows for the application of the second derivative test.
💡Relative Maximum/Minimum
A relative maximum or minimum refers to a point on the graph of a function where the function reaches a maximum or minimum value compared to its neighboring points, but not necessarily across the entire domain of the function. In the video, the speaker uses the second derivative test to determine if a critical point is a relative maximum or minimum. For example, a relative maximum is indicated when the second derivative at a critical point is less than zero.
💡Inconclusive
In the context of the second derivative test, a result is considered inconclusive when the second derivative at a critical point is zero. This means that the test does not provide enough information to determine whether the critical point is a local maximum, local minimum, or neither. The video emphasizes the importance of understanding that an inconclusive result does not allow for a definitive conclusion about the nature of the critical point.
💡Graph
A graph is a visual representation of the relationship between variables, typically used to illustrate the behavior of a function. In the video, the speaker uses graphs to visually demonstrate the concepts of local maxima and minima, as well as the concavity of a function. The graph helps viewers understand how the shape of the curve relates to the function's derivatives and critical points.
Highlights

The video aims to familiarize viewers with the second derivative test.

The second derivative test provides an intuitive understanding of a function's critical points.

A local maximum point occurs when the first derivative (slope) is zero and the function is concave downward.

At a local maximum, the second derivative, F prime prime of C, is less than zero.

A local minimum point occurs when the first derivative is zero and the function is concave upward.

At a local minimum, the second derivative, F prime prime of C, is greater than zero.

The second derivative test can determine if a critical point is a maximum, minimum, or inconclusive.

A function must be twice differentiable for the second derivative test to be applicable.

The second derivative test is a calculus tool for analyzing the concavity of a function at a critical point.

If the second derivative is zero, the test is inconclusive, and the nature of the critical point cannot be determined.

The video provides an example to illustrate the application of the second derivative test.

In the example, H of eight equals five, H prime of eight equals zero, and the second derivative at X equals eight is negative four.

Given the second derivative is negative, the point eight comma five is a relative maximum.

The second derivative test is a method for classifying critical points of differentiable functions.

The test is particularly useful for determining the nature of local extrema in a function.

The video emphasizes the importance of visual intuition in understanding the second derivative test.

Transcripts

Browse More Related Video

Concavity and the 2nd Derivative Test

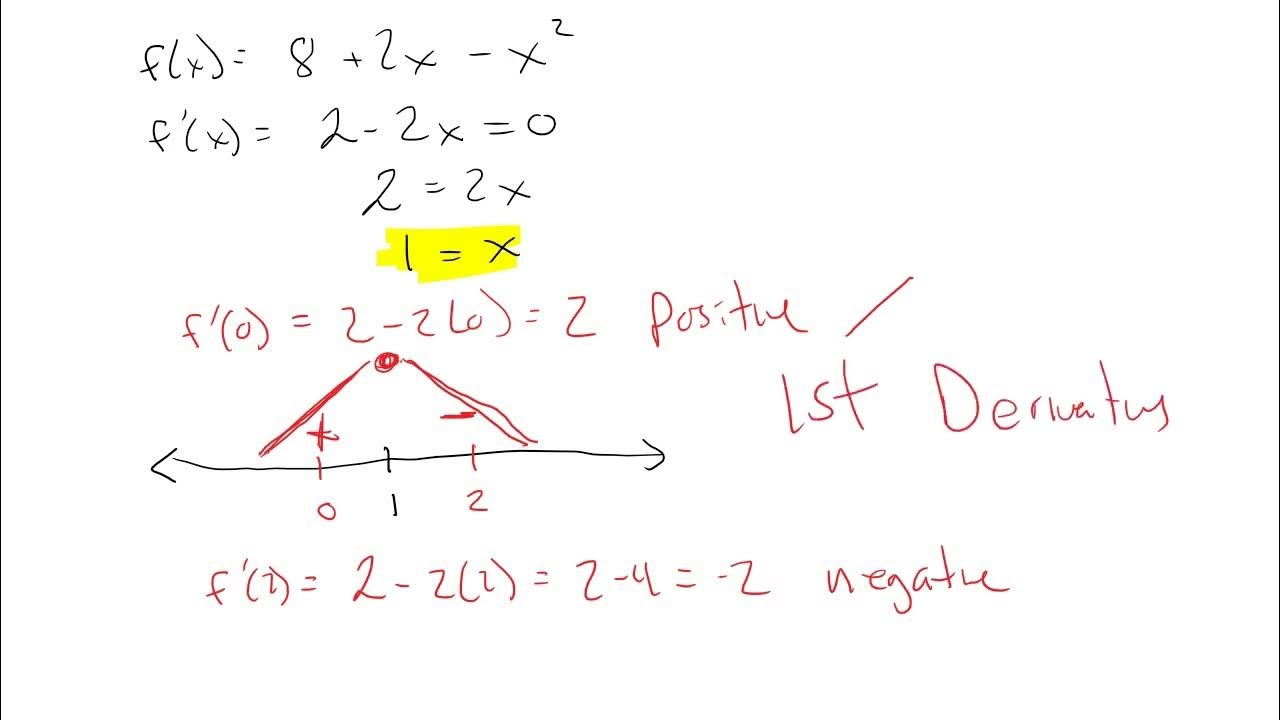
The First Derivative Test

Second Derivative Test to find relative maximums and minimums (extrema)

Second Derivative Test

3.2 - Using Second Derivatives to Classify Max and Min Values

Concavity introduction | Using derivatives to analyze functions | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TechniquesSecond DerivativeLocal ExtremaVisual LearningMathematical AnalysisFunction GraphsCritical PointsTangent SlopesConcavity RulesEducational Content