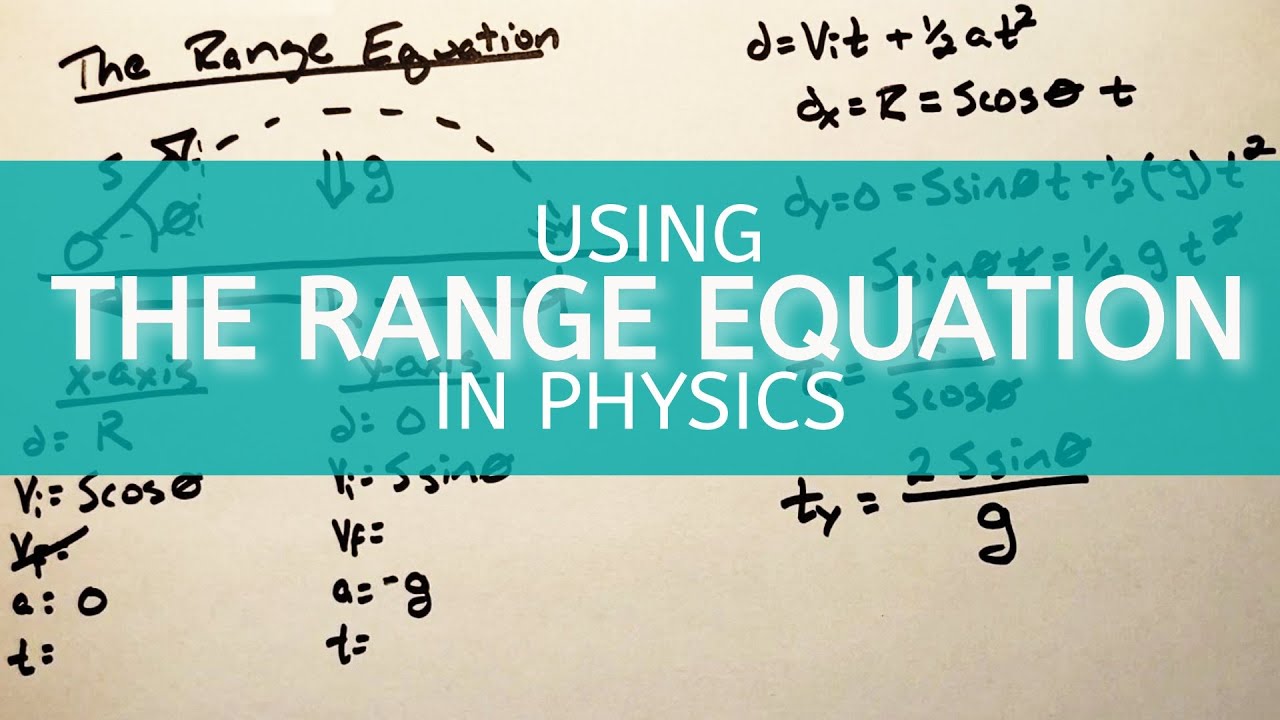AP Physics - Projectile Motion
TLDRThis transcript delves into the principles of projectile motion, emphasizing the independence of horizontal and vertical movements. It explains that a projectile, influenced solely by gravity, follows a parabolic arc and maintains its horizontal velocity while experiencing accelerated vertical motion. The discussion includes practical examples, such as a baseball throw and a stunt car, to illustrate how to calculate the time of flight and horizontal range. The importance of launch angle for maximizing distance is highlighted, with a 45° angle being optimal. The transcript also guides through solving problems involving projectile motion by breaking down complex scenarios into manageable parts, using horizontal and vertical motion tables and kinematic equations.
Takeaways
- 🚀 Projectile motion is influenced only by gravity, neglecting air resistance for simplification.
- 🏐 A projectile follows a parabolic path when launched at an angle and exhibits symmetry in its motion.
- 🔄 The time taken to ascend is equal to the time taken to descend in projectile motion.
- 📈 The horizontal and vertical motions of a projectile are independent and can be analyzed separately.
- 🌐 Horizontal motion has constant velocity with zero acceleration, while vertical motion is influenced by gravity.
- 🔢 To solve projectile motion problems, keep the horizontal and vertical components separate, except for the time of flight.
- 👏 The maximum horizontal range of a projectile launched from ground to ground is achieved at a 45° angle.
- 🚗 For objects launched at angles, their horizontal velocity remains constant throughout the motion.
- 📊 To find the horizontal range, use the horizontal velocity and the total time of flight calculated from the vertical motion.
- 📐 The initial velocity of a projectile can be decomposed into horizontal and vertical components for analysis.
- 🧪 A home experiment can be conducted to observe and calculate the projectile motion using a ball, stopwatch, and ruler.
Q & A
What is the primary force acting on a projectile?
-The primary force acting on a projectile is gravity.
How does air resistance affect projectile motion in the context of this discussion?
-In this discussion, the effect of air resistance is neglected to simplify the calculations and focus on the basic principles of projectile motion under the influence of gravity alone.
What type of motion does a projectile exhibit when launched at an angle?
-A projectile launched at an angle exhibits parabolic motion, with the path showing symmetry in its up and down journey.
What is the relationship between the time it takes for a projectile to ascend and descend?
-The time it takes for a projectile to ascend is equal to the time it takes to descend, assuming no air resistance and launched from and returning to the same height.
How does the horizontal motion of a projectile differ from its vertical motion?
-The horizontal motion of a projectile has zero acceleration since there is no force acting in the horizontal direction, while the vertical motion is influenced by gravity, causing a constant acceleration downwards at 9.8 m/s^2.
What is the key to solving projectile motion problems?
-The key to solving projectile motion problems is to keep the horizontal and vertical components of motion separate and understand that the only transferable quantity between them is the time of flight.
What is the maximum range a projectile launched from flat ground can achieve, and at what angle?
-A projectile launched from flat ground can achieve maximum range when launched at an angle of 45 degrees.
How can you find the horizontal component of a projectile's initial velocity if given the total initial velocity and launch angle?
-The horizontal component of a projectile's initial velocity can be found by multiplying the total initial velocity by the cosine of the launch angle.
In the context of this script, what is the significance of the independence of horizontal and vertical motion in projectile motion?
-The independence of horizontal and vertical motion allows for the separation of the two components, simplifying the calculations and making it possible to analyze and solve problems by considering each component separately.
What happens to the vertical velocity of a projectile at its highest point?
-At the highest point, the vertical velocity of a projectile momentarily becomes zero before it starts descending.
How can you experimentally determine the height from which a ball is dropped, based on its flight time and horizontal displacement?
-By using the principles of projectile motion, you can calculate the height from which a ball is dropped by analyzing the time it takes to fall (using its vertical motion) and its horizontal displacement (using its horizontal motion).
Outlines
🚀 Introduction to Projectile Motion
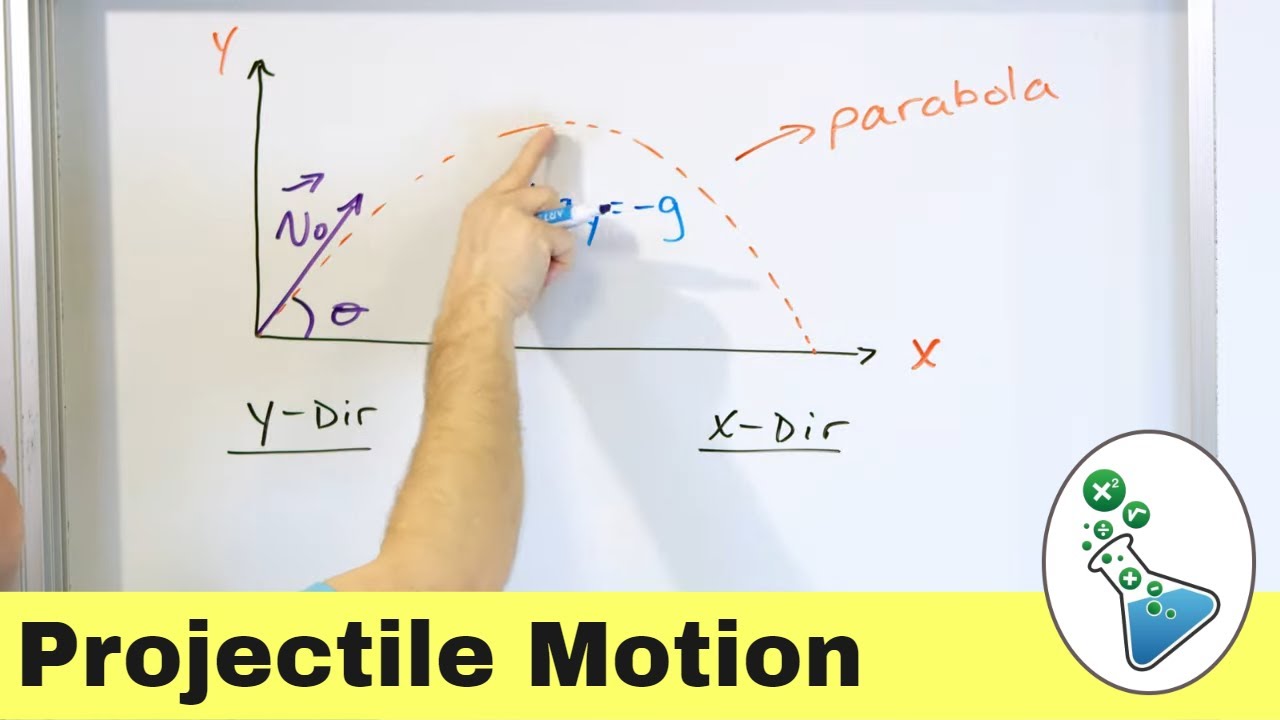
This paragraph introduces the concept of projectile motion, emphasizing that a projectile is an object acted upon solely by gravity. It outlines the objectives of the discussion, which include sketching the theoretical path of a projectile, recognizing the independence of horizontal and vertical motion, and solving problems involving projectiles launched at various angles and speeds. The paragraph also mentions the neglect of air resistance for simplicity, and the parabolic trajectory typically followed by projectiles launched at an angle.
📐 Horizontal and Vertical Motion Analysis
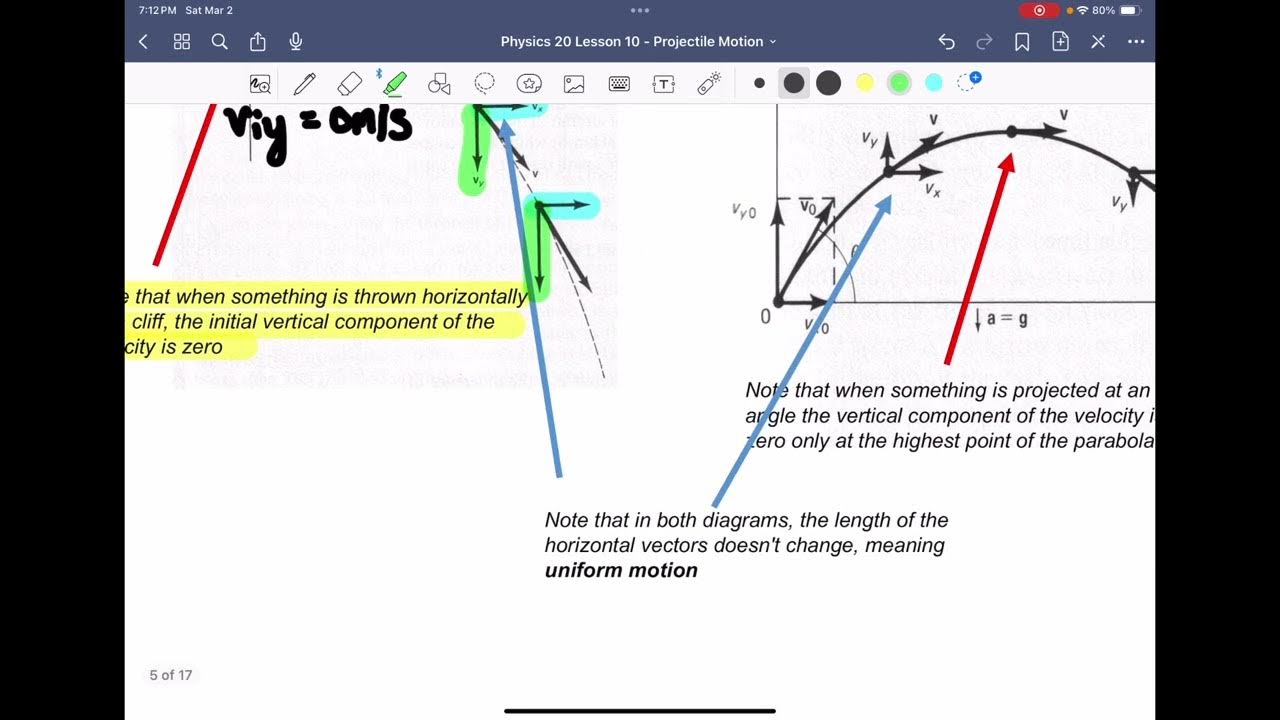
The second paragraph delves into the analysis of horizontal and vertical motion in projectile motion. It explains that horizontal motion is uniform, with no acceleration, while vertical motion is similar to freefall, accelerating at 9.8 m/s². The paragraph highlights the independence of these two motions and how they can be solved separately. It also introduces the concept of time being the same for both motions, which is crucial for solving projectile problems. A practical example is given, where a baseball is thrown horizontally from a height of 2 meters, and the problem of determining how far it travels before hitting the ground is discussed.
🤔 Solving Projectile Motion Problems
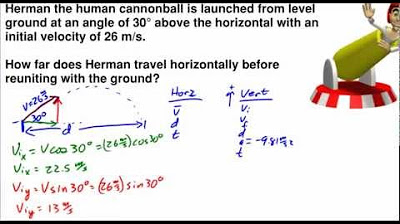
This paragraph focuses on the methodology of solving projectile motion problems. It explains the process of breaking down the initial velocity into horizontal and vertical components and using these components in motion tables for analysis. The paragraph also touches on the maximum range of a projectile, which is achieved when launched at a 45° angle, assuming no air resistance. A sample problem involving Herman the Human Cannonball is presented, illustrating how to calculate the horizontal distance traveled before returning to the ground, given an initial velocity and launch angle.
🧪 Hands-on Experiment with Projectile Motion
The final paragraph encourages a hands-on approach to understanding projectile motion by suggesting a home experiment. It invites the user to roll a ball off a table and measure the time it stays in the air and the horizontal distance it travels. The paragraph then challenges the user to apply their knowledge of physics to calculate the height of the table and the horizontal velocity of the ball, based on the collected data. It concludes with an invitation to share the findings and a recommendation to visit A+ Physics for further assistance if needed.
Mindmap
Keywords
💡Projectile Motion
💡Gravity
💡Horizontal and Vertical Motion
💡Parabolic Arc
💡Symmetry
💡Initial Velocity
💡Acceleration
💡Range
💡Time of Flight
💡Components of Motion
💡Lab Activity
Highlights
Projectile motion is a type of motion where an object is acted upon only by gravity.
Air resistance is neglected in the model of projectile motion for simplicity.
The path of a projectile launched at an angle tends to be parabolic.
The time it takes for a projectile to go up is equal to the time it takes to come down.
The velocity magnitude of a projectile remains the same in the vertical direction throughout its motion, only the direction changes.
The horizontal and vertical motions of a projectile are independent of each other.
In horizontal projectile motion, there is no acceleration; the initial velocity is the same as the final velocity.
Projectile motion equations can only be used when the only force acting on the object is gravity.
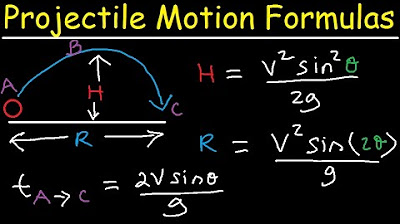
To calculate the horizontal range of a projectile, one must first calculate the time it is in the air using its vertical motion.
For a projectile launched horizontally, the horizontal displacement can be found using the formula Delta X = v average * t.
The maximum horizontal range of a projectile launched from flat ground to flat ground is achieved at a launch angle of 45°.
The horizontal component of a projectile's velocity remains constant throughout its flight.
To solve problems involving projectiles launched at an angle, one must break down the initial velocity into horizontal and vertical components.
The initial velocity vector can be decomposed into its horizontal (Vx) and vertical (Vy) components using trigonometric functions.
For a projectile launched at an angle, the horizontal motion table uses the horizontal component of the initial velocity and zero acceleration.
The vertical motion table helps determine the time a projectile is in the air by analyzing its upward and downward paths.
A practical application of projectile motion can be found in the lab activity where one can measure the time a ball is in the air and its horizontal displacement to calculate the height and horizontal velocity.
The lab activity encourages the application of physics concepts to real-world scenarios, enhancing understanding through hands-on experience.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: