AP Physics Workbook 7.L Angular Momentum

Mr.S ClassRoom
21 Apr 202015:55
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript from an AP Physics workbook discusses concepts of torque and rotation, focusing on a planet's energy and motion around a star. It explains the law of areas by Kepler, which states that a line connecting the planet to the star sweeps out equal areas in equal times, leading to variations in the planet's velocity based on its proximity to the star. The transcript also covers the conservation of angular momentum, highlighting that the planet's angular momentum remains constant throughout its orbit due to zero net torque. The discussion includes graphical representations of kinetic and potential energy, velocity, and force vectors, providing a comprehensive understanding of planetary motion and the underlying physics principles.

Takeaways
  • 🌍 The total energy of a planet is depicted with kinetic energy shown as a solid line above and total energy below on a graph.
  • 🔄 It takes 1.25 years for a planet to travel from point A to point B, completing two full cycles around its orbit.
  • 📊 Based on the graph, the time represented is 2.5 years for a full orbit, derived from the given 1.25-year half-orbit period.
  • 🚀 Kepler's law of areas states that a line connecting a planet to the Sun sweeps out equal areas in equal times, which is a consequence of the conservation of angular momentum.
  • 🌞 When a planet is closer to the Sun, it moves faster due to the conservation of angular momentum, thus covering the area quicker.
  • 📉 The potential energy graph of a planet-star system should be a reflection over the x-axis of the kinetic energy graph, balancing out to a flat line representing the total energy.
  • 🪂 At Point C on the orbit, the planet's velocity vector is outward, and the net force (gravity) vector is inward, illustrating the dynamics of elliptical orbit motion.
  • 🔄 The velocity of the planet is at its highest when it is closest to the star (perihelion) and lowest when it is farthest away (aphelion).
  • 📌 The net force vector and velocity vector at Point C form an obtuse angle, indicating the planet is moving away from the star and losing velocity.
  • 🌐 Angular momentum is conserved in a system with no net torque, meaning the total angular momentum remains constant as the planet orbits the star.
Q & A

  • What is the total energy of the start planet shown on the grid?

    -The total energy of the start planet is depicted on the grid with the kinetic energy represented by a solid line above it, while the total energy is indicated downwards.

  • How long does it take for the planet to travel from point A to point B?

    -It takes 1.25 years for the planet to travel from point A to point B, which corresponds to two full cycles of the planet's orbit.

  • What is Kepler's law of areas and how does it relate to the planet's velocity at different points in its orbit?

    -Kepler's law of areas states that a line connecting the planet to the Sun sweeps out equal areas in equal times. This means that when the planet is closer to the Sun, it moves faster, sweeping out a longer path in the same amount of time, resulting in a greater velocity compared to when it is farther from the Sun.

  • How long does it take for the planet to go from point B to point C, and why does this time differ from the expected 0.25 years?

    -The time it takes for the planet to go from point B to point C should be less than 0.625 years. This is because point B to point C is closer to the Sun, and according to Kepler's law of areas and conservation of angular momentum, the planet will move faster in this part of the orbit, completing the distance in a shorter time.

  • How should the graph of the potential energy of the planet-star system be drawn over the same interval?

    -The graph of the potential energy should be a reflection of the kinetic energy graph over the x-axis. This means that if the kinetic energy graph shows an increase followed by a decrease, the potential energy graph would show a decrease followed by an increase, mirroring the kinetic energy graph.

  • What do the vectors V and f represent on the orbit diagram at Point C?

    -Vector V represents the velocity of the planet at Point C, and vector f represents the net force acting on the planet. The direction of these vectors provides insights into the planet's motion and the forces acting upon it.

  • How does the planet's motion around the star influence the direction of the net force and velocity vectors?

    -As the planet moves in an elliptical orbit, the net force vector points inwards towards the star, and the velocity vector is tangent to the path of the orbit. The relative directions of these vectors change as the planet moves, with the force vector always pointing towards the star and the velocity vector changing direction along the tangent of the orbit.

  • What is the relationship between the planet's angular momentum and the net torque acting on it?

    -According to the law of conservation of angular momentum, the total angular momentum of a rotating object remains constant if the net torque acting on it is zero. In the case of the planet orbiting the star, the net force is directed towards the star and does not create a torque since it is not perpendicular to the planet's vector pointing towards the star. Therefore, the angular momentum remains constant throughout the orbit.

  • How does the planet's kinetic energy change as it moves from Point B to Point C?

    -As the planet moves from Point B to Point C, its kinetic energy decreases. This is because the planet is moving away from the star and the gravitational force is pulling it back in, causing it to lose velocity and thus kinetic energy.

  • What is the significance of the obtuse angle between the velocity vector (V) and the net force vector (f) at Point C?

    -The obtuse angle between the velocity vector and the net force vector at Point C indicates that the planet is losing kinetic energy. This is because the angle between the velocity and force vectors determines whether energy is being added to (acute angle) or subtracted from (obtuse angle) the system.

  • How does the law of conservation of angular momentum apply to the planet's motion around the star?

    -The law of conservation of angular momentum ensures that the planet's angular momentum remains constant as it orbits the star. Since the net force acting on the planet is directed towards the star and does not create a torque, the planet's angular momentum does not change throughout its orbit.

Outlines
00:00
🌌 Orbital Period and Energy Analysis

This paragraph discusses the concept of a planet's total energy and its orbital period. It begins by presenting a scenario where the total energy of a start planet is depicted on a grid, showing both kinetic and total energy. The first question revolves around calculating the time it takes for a planet to travel from point A to point B, which is half an orbit. By analyzing the graph, the video explains how to determine the full orbital period and the number of orbits completed. It then delves into Kepler's law of areas and the conservation of angular momentum, highlighting that when a planet is closer to the Sun, it moves faster, sweeping out equal areas in equal times. The paragraph clarifies a mistake regarding the time it should take for a planet to travel from point B to point C, emphasizing that due to the conservation of angular momentum, the time should be less than initially stated.

05:07
📊 Potential Energy and Vector Analysis

The second paragraph focuses on sketching the graph of a planet's potential energy over a specific interval and understanding the relationship between kinetic and potential energy in an elliptical orbit. It explains that at the end of the orbit, the sum of kinetic and potential energy should average out. The paragraph then introduces the concept of drawing vectors to represent the velocity and net force of a planet at a specific point (Point C) in its orbit. It discusses the direction of the force (inwards towards the star) and the velocity (tangential to the orbit), and how these vectors change as the planet moves closer to and farther from the star. The paragraph also touches on the planet's acceleration and velocity changes as it follows an elliptical orbit.

10:18
🔄 Direction of Planetary Motion and Angular Momentum

This paragraph corrects the direction of the planet's motion, which should be counterclockwise. It then moves on to discuss the energy changes at Point C, explaining that as the planet moves farther from the star, its connecting energy decreases. The paragraph uses vector analysis to explain why the planet slows down at this point in its orbit, attributing it to the force of gravity pulling it back in. It also explains the relationship between the angle of the velocity and net force vectors and how they affect the planet's energy. The paragraph concludes with an explanation of the conservation of angular momentum, stating that because there is no net torque on the planet as it orbits, its angular momentum remains constant.

15:19
📚 Summary of Kepler's Laws and Torque

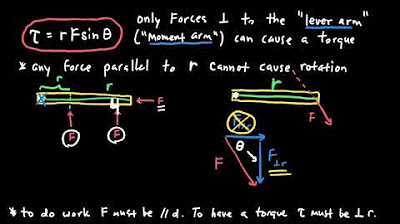
The final paragraph provides a summary of the key concepts discussed in the tutorial, including the law of conservation of momentum and torque. It reiterates the importance of understanding Kepler's law of areas, which is the second law of planetary motion, and how it relates to the planet's motion around the star. The paragraph emphasizes the need to review these concepts if they are not fully understood, ensuring a comprehensive grasp of the principles governing planetary motion.

Mindmap
Keywords
💡Torque
Torque is a measure of the force's tendency to cause or change the rotational motion of an object. In the context of the video, it's a fundamental concept in understanding how the net force acting on a planet creates rotational motion around a star. The video explains that the net torque acting on the planet is zero, which is why the angular momentum of the planet remains constant as it orbits the star, illustrating the conservation of angular momentum principle.
💡Angular Momentum
Angular momentum is a measure of the rotational motion of an object. It is a vector quantity that depends on the object's moment of inertia and its angular velocity. In the video, the conservation of angular momentum is a key principle, stating that if no net torque acts on an object, its angular momentum will remain constant. This concept is crucial in understanding the stable orbits of planets around a star, as the video explains that the planet's angular momentum remains constant throughout its orbit due to the absence of net torque.
💡Kepler's Law of Areas
Kepler's Law of Areas, also known as the second law of planetary motion, states that a line connecting a planet to the Sun sweeps out equal areas in equal times. This law is a direct consequence of the conservation of angular momentum and describes how a planet moves faster when it is closer to the Sun, thus sweeping out larger areas in the same amount of time. The video uses this law to explain why the planet moves faster in certain parts of its orbit and why it takes less time to travel from one point to another when closer to the Sun.
💡Potential Energy
Potential energy is the stored energy an object has due to its position in a force field, such as the gravitational field of a star. In the context of the video, the potential energy of a planet in its orbit around a star is related to its distance from the star. The video explains that the potential energy is higher when the planet is farther from the star and lower when it is closer, reflecting the gravitational potential energy. The combination of potential and kinetic energy over the orbit results in the total energy of the system, which remains constant.
💡Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. It is directly proportional to the mass of the object and the square of its velocity. In the video, kinetic energy is contrasted with potential energy to explain the energy changes of a planet as it orbits a star. The kinetic energy is highest when the planet is closest to the star and lowest when it is farthest away, reflecting the conservation of mechanical energy in the system.
💡Orbit
An orbit is the path that an object takes around another object in space, typically under the influence of gravity. In the video, the orbit of a planet around a star is described as elliptical, and the concepts of potential and kinetic energy, as well as angular momentum, are used to explain the planet's motion within this orbit. The video also discusses how the planet's velocity and the net force acting on it change as it moves along its orbit.
💡Velocity
Velocity is a vector quantity that describes the speed of an object in a specific direction. In the context of the video, the velocity of a planet in its orbit is a critical factor in understanding its motion. The video explains that the planet's velocity varies as it moves around the star, being greatest at the closest point to the star (perihelion) and smallest at the farthest point (aphelion). This variation in velocity is related to the conservation of angular momentum and Kepler's laws.
💡Net Force
Net force is the vector sum of all the individual forces acting on an object. In the video, the net force acting on a planet in its orbit is directed towards the star, causing the planet to follow an elliptical path. The video explains that even though there is a net force, it does not create a torque because it is not perpendicular to the planet's moment of inertia, leading to the conservation of angular momentum.
💡Conservation of Mechanical Energy
The conservation of mechanical energy states that the total mechanical energy (the sum of kinetic and potential energy) in a closed system remains constant if no external forces do work on the system. In the video, this principle is illustrated by the planet's orbit, where the sum of its kinetic and potential energy remains constant as it moves around the star, reflecting the conservation of mechanical energy.
💡Elliptical Orbit
An elliptical orbit is a type of planetary motion where a celestial body, such as a planet, moves around a central point, typically a star, in an elongated circular path or ellipse. In the video, the elliptical orbit is used to explain the varying distance between the planet and the star, which in turn affects the planet's velocity and the net force acting on it. The video uses the concept of an elliptical orbit to discuss Kepler's laws and the conservation of angular momentum.
💡Mechanical Energy
Mechanical energy is the sum of the kinetic and potential energies in a system. It is a measure of the total energy that can be used to do work. In the context of the video, the mechanical energy of a planet in its orbit is constant, demonstrating the conservation of mechanical energy. The video explains that as the planet moves closer to the star, its potential energy decreases while its kinetic energy increases, and vice versa when it moves farther away, maintaining a constant total mechanical energy.
Highlights

The total energy of the start planet is depicted in the given scenario, with kinetic energy represented by a solid line.

The planet takes 1.25 years to travel from point A to point B, completing two cycles as shown on the graph.

According to Kepler's law of areas, a line connecting the planet to the Sun sweeps out equal areas in equal time, arising from the conservation of angular momentum.

When the planet is closer to the Sun, it moves faster due to the conservation of angular momentum, thus taking less time to travel a certain path.

The potential energy graph should be mirrored over the x-axis to reflect the conservation of energy throughout the planet's orbit.

At Point C on the orbit diagram, a vector V represents the planet's velocity, and a vector f represents the net force.

The planet's motion around an elliptical orbit is influenced by a force directed towards the star, with the velocity vector tangential to the path.

As the planet moves from point B to Point C, it is farther from the star, resulting in a decrease in velocity and an obtuse angle between the velocity and net force vectors.

The kinetic energy of the planet decreases as it moves away from the star due to the force of gravity pulling it back in.

The angular momentum of the planet around the star is conserved, as there is no net torque acting on the planet during its orbit.

The net force on the planet does not create a torque because it is directed towards the star, not perpendicular to the planet's vector.

The conservation of angular momentum is a key principle in understanding the constant nature of the planet's orbit.

Kepler's law of areas is a fundamental concept in explaining the elliptical orbit of a planet and its velocity changes.

The tutorial provides a comprehensive understanding of torque and rotation in the context of planetary motion.

The scenario and subsequent analysis demonstrate the intricate relationship between energy, force, and motion in physics.

Transcripts

Browse More Related Video

AP Physics 1 - Kepler's Laws

Kepler's Laws

2022 Live Review 7 | AP Physics 1 | Understanding Torque and Rotational Motion

AP Physics 1 Torque and Rotational Motion Review

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy

AP Physics Workbook 7.I Collisions "Updated and Fixed"

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsConceptsTorqueAnalysisRotationalDynamicsKeplerLawsAngularMomentumOrbitalMechanicsAPPhysicsEducationalContentWorkbookSolutionsPlanetaryEnergy