L'Hôpital's rule example 1 | Derivative applications | Differential Calculus | Khan Academy

Khan Academy
7 Jun 201007:42
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of evaluating a limit as x approaches 0 for a given trigonometric function using L'Hopital's rule. The presenter first attempts to directly evaluate the function at x=0, resulting in an indeterminate form. They then apply L'Hopital's rule multiple times, taking derivatives of the numerator and denominator until a determinate form is reached. The final result of the limit is found to be 6, demonstrating the utility of L'Hopital's rule in solving complex limit problems.

Takeaways
  • 📌 The problem at hand involves evaluating a limit as x approaches 0 for a given trigonometric function.
  • 🔍 The initial approach is to directly substitute x with 0, resulting in an indeterminate form (0/0).
  • 📚 L'Hopital's rule is suggested as a method to tackle the indeterminate form by finding the limit of the ratio of the derivatives.
  • 🌟 The first derivative of the numerator is 2cos(x) - 2cos(2x), and the first derivative of the denominator is 1 - cos(x).
  • 🔄 Upon applying L'Hopital's rule, another indeterminate form (0/0) is encountered, indicating the need for a second application.
  • 📈 The second derivative of the numerator is -2sin(x) + 4sin(2x), and the second derivative of the denominator is sin(x).
  • 🎯 The limit of the second application of L'Hopital's rule is found to be 6, as the derivatives of the numerator and denominator do not result in an indeterminate form.
  • 💡 The final result of the limit, as determined through L'Hopital's rule, is 6.
  • 📝 The process demonstrates the importance of systematically applying derivatives and evaluating limits when direct substitution leads to an indeterminate form.
  • 🌐 The method can be generalized for similar limit problems involving trigonometric functions and indeterminate forms.
Q & A

  • What is the initial limit problem presented in the transcript?

    -The initial limit problem is to evaluate the limit as x approaches 0 of (2 sine of x minus sine of 2x) divided by (x minus sine of x).

  • What is the first approach taken to solve the limit problem?

    -The first approach is to directly evaluate the function at x equals 0.

  • What is the result of plugging x equals 0 into the function?

    -The result is an indeterminate form of 0/0, as both the numerator and denominator become zero.

  • How does the video suggest proceeding when faced with an indeterminate form like 0/0?

    -The video suggests using L'Hopital's rule, which involves taking the derivatives of the numerator and denominator and evaluating the limit of the new expression.

  • What are the derivatives of the numerator and the denominator?

    -The derivative of the numerator is (2 cosine of x minus 2 cosine of 2x), and the derivative of the denominator is (1 minus cosine of x).

  • What happens when the derivatives are evaluated at x equals 0?

    -Evaluating the derivatives at x equals 0 results in another indeterminate form of 0/0.

  • How many times can L'Hopital's rule be applied in this scenario?

    -L'Hopital's rule can be applied multiple times until a determinate form is reached or until it's no longer valid to apply the rule.

  • What is the final result of the limit after applying L'Hopital's rule multiple times?

    -The final result of the limit is 6, after several applications of L'Hopital's rule resolve the indeterminate forms.

  • What does the video imply about the use of L'Hopital's rule in limit problems?

    -The video implies that L'Hopital's rule is a powerful tool in limit problems, especially when dealing with indeterminate forms, but it should be applied carefully and correctly to ensure valid results.

  • What is the significance of the result in the context of the limit problem?

    -The significance of the result is that it provides a determinate value for the limit, which helps in understanding the behavior of the function as x approaches 0.

Outlines
00:00
📚 Introduction to Limit Evaluation

This paragraph introduces the process of evaluating a limit as x approaches 0 for a given trigonometric function. The speaker begins by suggesting to first check the function at x=0, which results in an indeterminate form (0/0). The concept of L'Hopital's rule is then introduced as a potential solution to this indeterminate form. The speaker explains that to apply L'Hopital's rule, the derivatives of the numerator and denominator must exist at x=0. The paragraph concludes with the application of L'Hopital's rule and the calculation of the derivatives of the given function.

05:00
🔢 Application of L'Hopital's Rule and Further Analysis

In this paragraph, the speaker continues the process of evaluating the limit using L'Hopital's rule. The derivatives of the numerator and denominator are calculated, leading to another indeterminate form (0/0). The speaker emphasizes that L'Hopital's rule can still be applied, and the process is repeated to find the next set of derivatives. The new derivatives are evaluated at x=0, resulting in a definitive answer for the limit, which is found to be 6. The paragraph concludes by reinforcing the successful application of L'Hopital's rule in solving the limit problem.

Mindmap
Keywords
💡limit
In the context of the video, a limit refers to a mathematical concept used to describe the behavior of a function as the input (or argument) approaches a particular value. The video focuses on evaluating the limit as x approaches 0 for a given trigonometric function. This concept is crucial in calculus and is used to understand the behavior of functions at certain points, such as when the input approaches infinity or a specific value.
💡L'Hopital's rule
L'Hopital's rule is a method in calculus that allows us to evaluate limits of the form 0/0 or ∞/∞ by taking the derivatives of the numerator and the denominator. The video script illustrates the application of L'Hopital's rule multiple times to find the limit of a function when direct substitution leads to an indeterminate form. This rule is particularly useful when dealing with limits at points where the function is not defined or does not exist.
💡trigonometric functions
Trigonometric functions, such as sine and cosine, are mathematical functions that relate angles to real numbers. In the video, these functions are used in the expression for which the limit is being evaluated. The sine function, in particular, is used extensively in the numerator and denominator of the given expression, and understanding its behavior near x=0 is key to solving the problem.
💡indeterminate form
An indeterminate form is a type of expression that cannot be evaluated directly because it involves an undefined operation, such as 0/0 or ∞/∞. In the video, the initial attempt to evaluate the limit results in an indeterminate form, which prompts the use of L'Hopital's rule to find a determinate value. Identifying when an expression is in an indeterminate form is crucial for applying the appropriate techniques to find a solution.
💡derivative
The derivative of a function is a measure of how the function changes as its input changes. It is a fundamental concept in calculus, representing the rate of change or slope of a function at a given point. In the video, derivatives are used to find the limit of the given function by applying L'Hopital's rule, which involves taking the derivative of both the numerator and the denominator.
💡chain rule
The chain rule is a technique used in calculus to find the derivative of a composite function. It states that the derivative of a function composed of multiple functions is the derivative of the outer function times the derivative of the inner function. In the video, the chain rule is used to find the derivative of sin(2x), which involves taking the derivative of the outer function sin and applying it to the inner function 2x.
💡cosine
The cosine function is one of the six trigonometric functions and is closely related to the sine function. It is used to calculate the ratio of the adjacent side to the hypotenuse in a right-angled triangle, as well as in various mathematical and physical applications. In the video, the cosine function appears in the derivative calculations and in the final evaluation of the limit.
💡sine
The sine function is one of the six trigonometric functions, used to calculate the ratio of the opposite side to the hypotenuse in a right-angled triangle. It is a periodic function that oscillates between -1 and 1 and is fundamental in solving problems in mathematics, physics, and engineering. In the video, the sine function is central to the function whose limit is being evaluated, with its value at x=0 being 0.
💡evaluation
Evaluation in mathematics refers to the process of determining the value of an expression by substituting values for the variables. In the context of the video, evaluation is the act of finding the limit of a function as x approaches a certain value. The video demonstrates the evaluation process by substituting x=0 into the function and then using L'Hopital's rule to find the limit when direct substitution leads to an indeterminate form.
💡substitution
Substitution is a mathematical technique where one expression is replaced with another in order to simplify or solve an equation or function. In the video, substitution is used to replace x with 0 in the function to see if a direct evaluation of the limit is possible. However, when substitution leads to an indeterminate form, other methods like L'Hopital's rule are employed.
💡rate of change
The rate of change of a function describes how quickly the function's output changes as the input varies. It is a central concept in calculus and is represented by the derivative of the function. In the video, the rate of change is discussed in the context of finding the derivative of the numerator and the denominator to apply L'Hopital's rule and evaluate the limit.
Highlights

The problem involves evaluating a limit as x approaches 0 of a trigonometric function.

The initial attempt is to directly evaluate the function at x equals 0, resulting in an indeterminate form of 0/0.

L'Hopital's rule is suggested as a method to deal with the indeterminate form.

The first derivative of the numerator is 2 cosine of x minus 2 cosine of 2x, using the chain rule.

The first derivative of the denominator is 1 minus cosine of x.

Upon applying L'Hopital's rule, another indeterminate form of 0/0 is encountered.

L'Hopital's rule is applied a second time, leading to a more complex derivative calculation.

The second derivative of the numerator results in negative 2 cosine of x plus 4 sine of 2x.

The second derivative of the denominator is sine of x.

After evaluating the third application of L'Hopital's rule, a determinate result is obtained.

The limit, if it exists, is found to be 6 through the application of L'Hopital's rule.

The process demonstrates the importance of persistence when dealing with indeterminate forms.

The use of L'Hopital's rule is emphasized as a powerful tool in calculus for solving limits of the form 0/0 or infinity/infinity.

The example serves as a clear illustration of the step-by-step application of L'Hopital's rule in limit calculations.

Trigonometric functions and their derivatives play a crucial role in the problem-solving process.

The chain rule is fundamental in finding the derivatives of composite functions.

The problem showcases the interplay between algebraic manipulation and calculus concepts.

The solution路径 involves a combination of direct substitution, derivative calculation, and repeated application of L'Hopital's rule.

Transcripts

Browse More Related Video

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy

Limits of Logarithmic Functions | Calculus

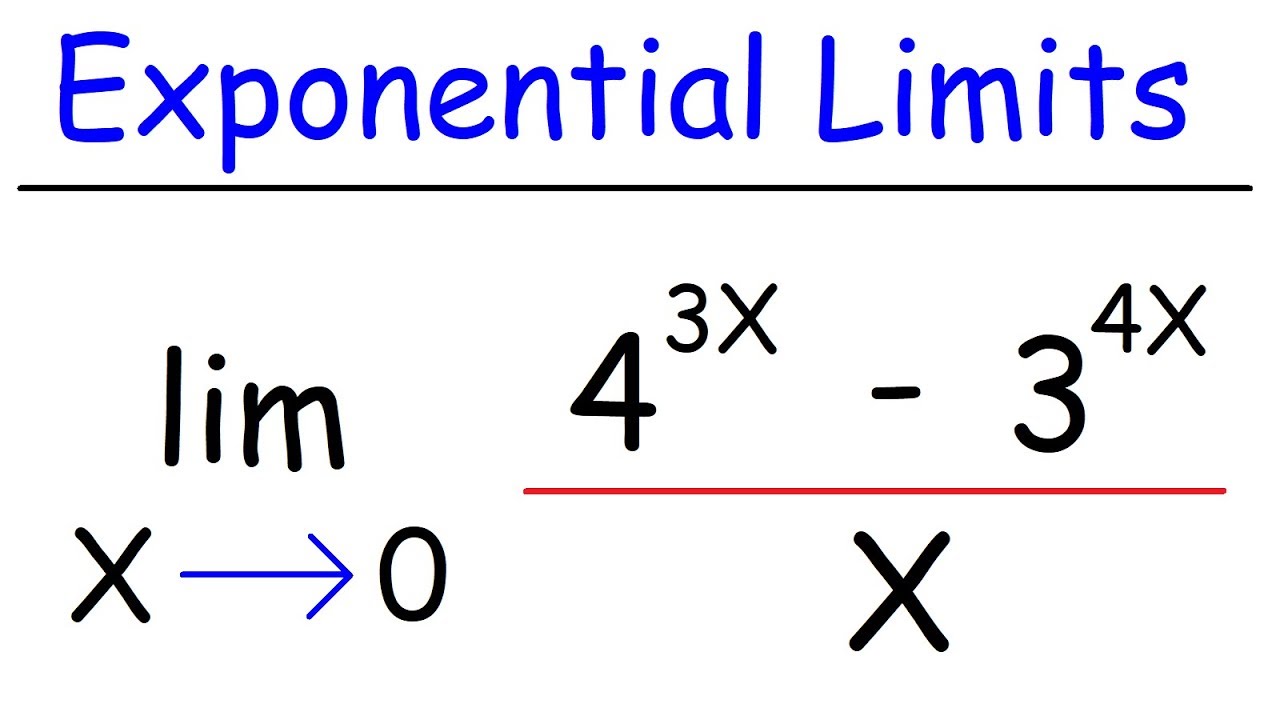
Limits of Exponential Functions | Calculus

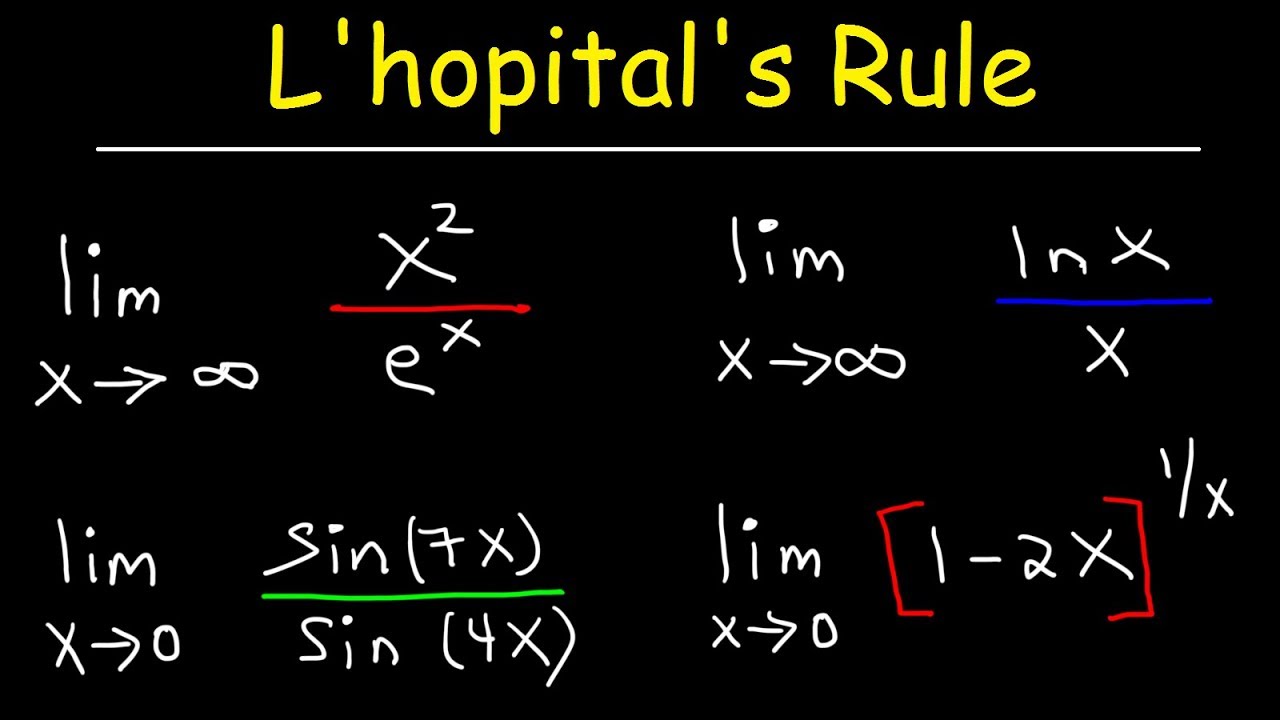
L'hopital's rule

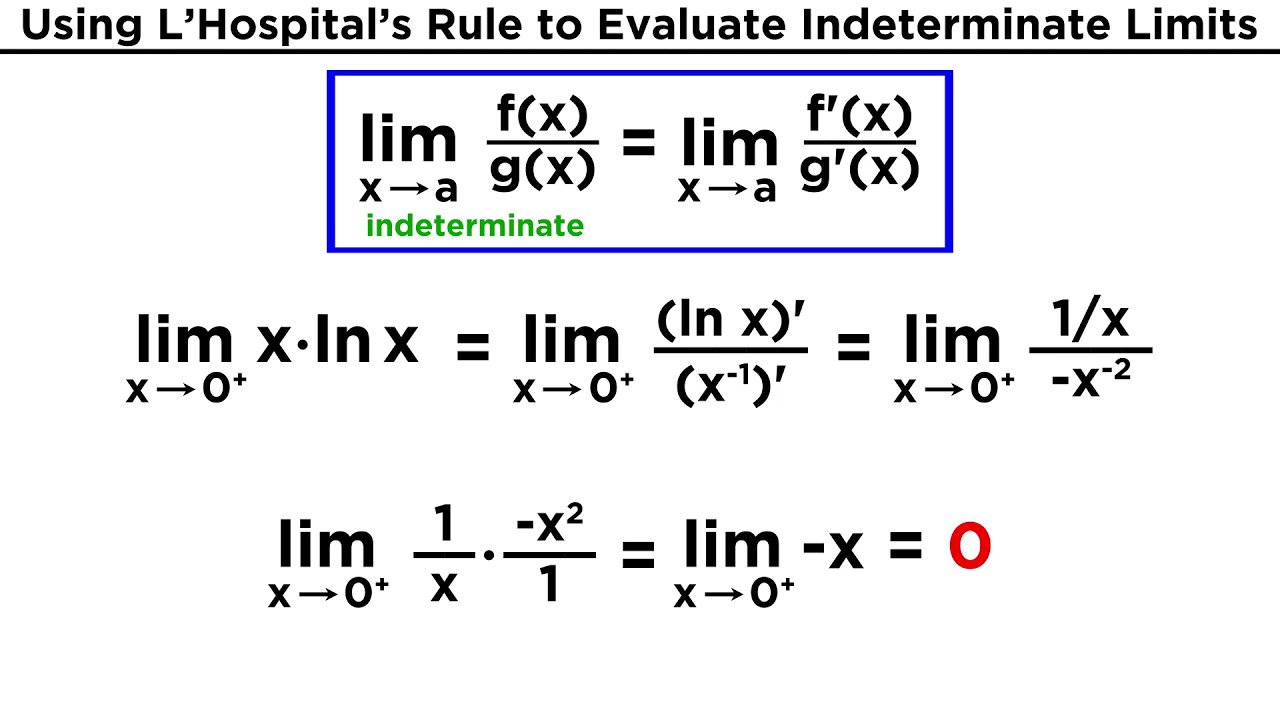
Understanding Limits and L'Hospital's Rule

L'Hôpital's rule example 3 | Derivative applications | Differential Calculus | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusLimitsL'Hopital's RuleTrigonometryIndeterminate FormsDerivativesMathematicsEducational ContentProblem SolvingMath Tutorial