Trigonometry Law of Sines / Sine Rule

tecmath
23 Oct 201909:35
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, the presenter introduces the sign rule, a method for solving non-right angle triangles to find unknown sides and angles. The rule is explained with the formula S/a = S/b = sign(C)/C, where S is the hypotenuse and a, b, and C are the angles and opposite sides. Two examples are worked out, demonstrating how to use the rule to find both sides and angles, with a focus on practical application and a helpful tip for solving equations. The video concludes with a call to action for viewers to support the channel and a preview of the next topic, the cosign rule.

Takeaways
  • 😃 Welcome to the Tech Math Channel, where today's focus is on understanding the sine rule, a method applicable to non-right angle triangles.
  • 👍 A shoutout was given to the latest Patron, Zoya, highlighting the value of support for the channel.
  • 📌 The sine rule relates the sides of a triangle to the sines of its angles, essential for calculating unknown sides and angles in non-right angle triangles.
  • 🖥 The formula for the sine rule can be presented in two formats, either involving sines over sides or sides over sines, providing flexibility in applications.
  • 📚 Triangles are labeled with capital letters for angles and matching lowercase letters for their opposite sides, facilitating the use of the sine rule.
  • 📝 To apply the sine rule effectively, knowing at least one complete set of an angle and its corresponding side, plus part of another set, is necessary.
  • 👨‍💻 An example illustrated the process of using the sine rule to find an unknown side, employing a simplified version of the rule for efficiency.
  • 📈 Another example demonstrated finding an unknown angle using the sine rule, introducing an extra step involving trigonometric calculations.
  • ✏️ Tips were offered on handling specific types of questions, such as calculating angles indirectly through the use of complementary angles.
  • 📱 The importance of supporting the Tech Math Channel through Patreon was emphasized, with a look ahead to covering the cosine rule in future videos.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the sign rule, a method used with non-right angle triangles to find unknown sides and angles.

  • How can you express the sign rule in its simplest form?

    -The sign rule can be expressed in its simplest form as: S of angle A / side a = S of angle B / side b = S of angle C / side c.

  • What is the significance of the sign rule in trigonometry?

    -The sign rule is significant in trigonometry as it helps in solving non-right angle triangles by relating the sides and angles of the triangle.

  • What are the specific letters assigned to the sides and angles in the triangle?

    -The specific letters assigned to the sides are 'a', 'b', and 'c', corresponding to the sides opposite angles A, B, and C, respectively.

  • How does the video demonstrate the application of the sign rule?

    -The video demonstrates the application of the sign rule through two examples: one where a side length is calculated using known angles and another where an angle is calculated using known side lengths.

  • What is the first example given in the video involving the sign rule?

    -The first example involves a triangle with an angle of 37° opposite side length 'a' and an angle of 97° opposite the unknown side length 'b'. The sign rule is used to find the length of side 'b'.

  • What is the second example provided in the video, and how is it solved?

    -The second example involves a triangle with an angle of 44° opposite side length 'a' (12 units) and an unknown angle 'B' opposite side length 'b' (14 units). The sign rule is used to find the measure of angle 'B'.

  • How does the video suggest solving for an unknown value using the sign rule?

    -The video suggests using a proportion method, where known values are used to form a ratio, which is then cross-multiplied to solve for the unknown value.

  • What is the additional trick mentioned in the video for solving problems with the sign rule?

    -The additional trick mentioned is to use the relationship between angles in a triangle (total 180°) to find the third angle when given two angles and one side length.

  • How can you find the length of an unknown side using the sign rule?

    -To find the length of an unknown side using the sign rule, you can use the known values of the angles and their opposite sides to form a proportion and solve for the unknown side length.

  • How can you find the measure of an unknown angle using the sign rule?

    -To find the measure of an unknown angle using the sign rule, you can use the known values of the sides and their opposite angles to calculate the sine of the unknown angle and then find its measure using the inverse sine function.

Outlines
00:00
📏 Introduction to the Sine Rule in Non-Right Angle Triangles

This segment introduces the concept of the sine rule, a fundamental principle used to calculate unknown sides and angles in non-right angle triangles. The presenter begins by expressing gratitude towards the latest Patron, Zoya, for supporting the TechMath channel and encourages viewers to consider becoming patrons. The sine rule is described as a method for dealing with triangles that do not have a right angle, where the ratio of the length of a side over the sine of its opposite angle is constant across all sides and angles of the triangle. This rule can be applied in two forms, either by comparing the sides to the sines of their respective angles or inversely. The video illustrates how to label the sides and angles of a triangle with capital and lowercase letters to apply the sine rule effectively. Through simplification of the rule for clarity, it's shown how knowing one complete set of an angle and its corresponding side length, along with part of another set, allows for the calculation of unknown elements within the triangle. The explanation is grounded with a practical example, demonstrating the application of the sine rule to find a missing side length.

05:01
🧮 Applying the Sine Rule: Examples and Techniques

In this section, the presenter delves into practical applications of the sine rule through detailed examples, emphasizing its utility in solving for unknown sides and angles in non-right angle triangles. The first example showcases how to determine an unknown side length by applying a condensed version of the sine rule, using known angles and their corresponding sides. The presenter employs a step-by-step approach, including labeling sides, substituting known values into the sine rule equation, and performing calculations to find the unknown side. The second example introduces a more complex scenario aimed at finding an unknown angle. This involves a similar process of labeling, substituting values, and calculating, but with the addition of an extra step to solve for the angle using the inverse sine function. The video concludes with strategic advice on handling various problem types, such as calculating angles indirectly by knowing the total sum of angles in a triangle. The presenter encourages viewers to support the channel as a patron and hints at covering the cosine rule in future videos.

Mindmap
Keywords
💡Sign Rule
The Sign Rule is a method used for solving problems related to non-right angle triangles. It is a formula that allows us to find unknown sides or angles by using the known sides and angles of the triangle. In the video, the Sign Rule is expressed as S of capital A over little a equals S of capital B over little B, which equals the sign of capital C over little C. This rule is crucial for solving the examples provided, where unknown side lengths and angles are calculated based on given information.
💡Non-Right Angle Triangles
Non-right angle triangles are triangles in which none of the angles are 90 degrees. These triangles are more complex to solve than right-angled triangles because they require the use of trigonometric functions like the sine function to determine the relationships between their sides and angles. The video focuses on using the Sign Rule specifically for these types of triangles, as it is an essential tool for solving problems where right-angle trigonometry is not applicable.
💡Sine Function (S)
The sine function, denoted as 'S' or 'sin', is a fundamental trigonometric function that relates the ratio of the length of the side opposite an angle to the length of the hypotenuse in a right-angled triangle. In the context of the video, the sine function is used to calculate the values of angles and sides in non-right angle triangles using the Sign Rule. The sine function is integral to solving the problems presented in the video, as it allows for the conversion of angle measurements into ratios that can be used in the Sign Rule formula.
💡Patron
A patron, in the context of the video, refers to an individual who supports the Tech Math Channel through a subscription service, providing financial assistance to help create and maintain the content. Patrons are acknowledged and appreciated by the creator for their contributions, which enable the production of educational material. This concept is important as it highlights the community aspect of learning and the support network that exists between content creators and their audience.
💡Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. It is particularly useful in solving problems involving non-right angle triangles, as it provides methods for finding unknown angles and sides when some information is already known. The Sign Rule discussed in the video is a part of trigonometry and is essential for solving problems in non-right angle triangles.
💡Angle Labeling
In the context of the video, angle labeling refers to the process of assigning specific letters (capital and lowercase) to the angles and sides of a triangle, respectively. This labeling system is crucial for applying trigonometric rules and formulas, as it allows for a clear and organized approach to problem-solving. Capital letters are used for angles, while lowercase letters correspond to the sides opposite those angles.
💡Unknowns
In mathematical problems, unknowns are the values that are not yet known and need to be solved for. In the context of the video, unknowns refer to the sides or angles of a triangle that the viewer is trying to find using the Sign Rule. The process of solving for unknowns involves using known values and mathematical relationships to determine the values of these unknown quantities.
💡Condensed Version of the Sign Rule
The condensed version of the Sign Rule is a simplified form of the full Sign Rule formula, which is used when only certain parts of the triangle's information are known. This version focuses on the specific relationships between the known and unknown elements, making it easier to apply to particular problems. In the video, the condensed Sign Rule is used to solve for either an unknown side or an unknown angle, depending on the given information.
💡Inverse Sine Function
The inverse sine function, often denoted as 'sin^-1' or 'arcsin', is the inverse operation of the sine function. It is used to find the angle whose sine is a given value. In the context of the video, the inverse sine function is crucial for finding the angle when the sine value (resulting from the application of the Sign Rule) is known.
💡Tech Math Channel
The Tech Math Channel is the name of the educational platform or channel from which the video script is derived. It is likely a YouTube channel or similar platform dedicated to teaching math, specifically focusing on topics such as trigonometry and problem-solving techniques. The channel is mentioned in the context of seeking support from patrons and viewers, indicating its role as an educational resource.
💡Cosine Rule
The Cosine Rule is a formula used in trigonometry to find a side length or an angle in any triangle when two sides and the included angle, or two angles and the side between them, are known. It is an extension of the Pythagorean theorem and is particularly useful for non-right angle triangles. In the video, the Cosine Rule is mentioned as a topic for a future video, indicating its importance in solving triangle problems.
Highlights

Introduction to the sign rule in non-right angle triangles.

Shoutout to latest Patron Zoya for supporting the TechMath channel.

Explanation of the sine rule: a/sin(A) = b/sin(B) = c/sin(C).

Alternative representation of the sine rule: sin(A)/a = sin(B)/b = sin(C)/c.

Labelling triangle sides and angles with letters for easier understanding.

Using matching sets to work out unknown sides and angles.

Example of solving for a side using the sine rule.

Substituting known values into the sine rule for calculation.

Calculating side lengths using sine values and ratios.

Example of solving for an angle using the sine rule.

Substituting known values into the sine rule to solve for angles.

Using the inverse sine function to find unknown angles.

Calculating remaining angles in a triangle using sum of angles.

Tips for dealing with questions involving finding specific angles.

Announcement of upcoming video on the cosine rule.

Transcripts

Browse More Related Video

The Law of Sines

Law of Cosines, Finding Angles & Sides, SSS & SAS Triangles - Trigonometry

Trigonometry: Finding missing sides and angles

How to Use the Law of Sines in Trigonometry (Precalculus - Trigonometry 32)

Trigonometry - How To Solve Right Triangles

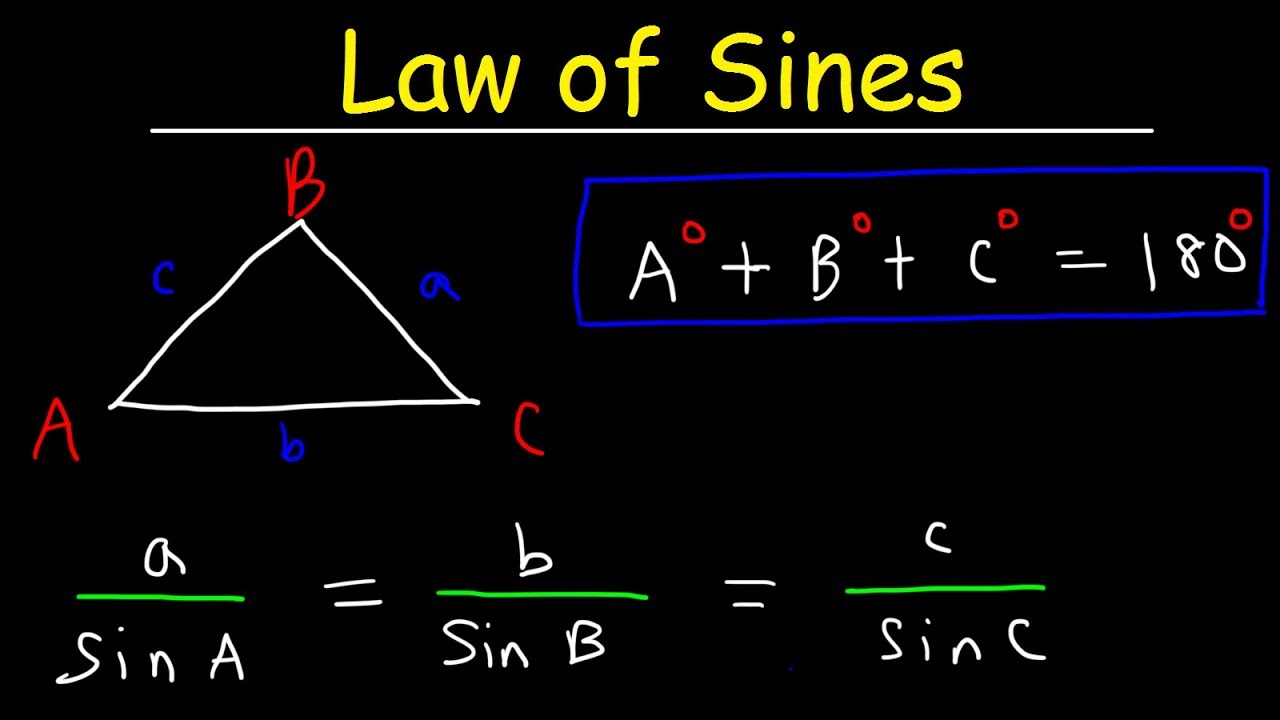
Law of Sines, Basic Introduction, AAS & SSA - One Solution, Two Solutions vs No Solution, Trigonomet

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsSine RuleGeometryNon-Right AnglesTutorialEducationalTechMathAngle CalculationTriangle SidesLearning