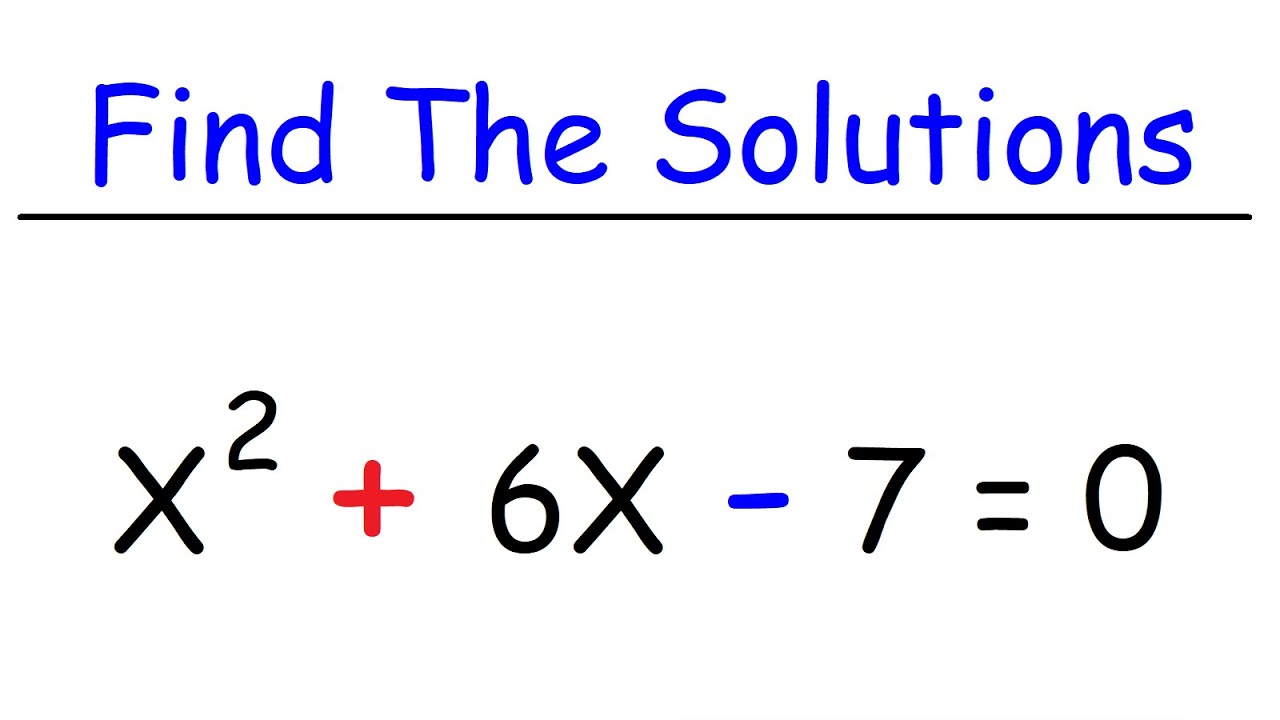How Imaginary Numbers Were Invented
TLDRThe script explores the historical evolution of mathematics, particularly focusing on the cubic equation and the development of complex numbers. It narrates the journey from ancient civilizations unable to solve cubic equations, through the breakthroughs by mathematicians like Luca Pacioli, Scipione del Ferro, Antonio Fior, Niccolo Fontana Tartaglia, and Gerolamo Cardano, to the eventual understanding of imaginary numbers. The story highlights the separation of math from the real world as a key to unlocking deeper truths about reality, exemplified by the use of imaginary numbers in Schrödinger's equation, foundational to quantum physics.
Takeaways
- 📏 Mathematics originated as a tool for practical purposes like measuring land and tracking commerce.
- 🚀 The concept of imaginary numbers, once thought impossible, became integral to our understanding of the universe's physical theories.
- 📚 Luca Pacioli's 'Summa de Arithmetica' from 1494 concluded that solving the cubic equation was impossible, despite earlier civilizations' progress with simpler equations.
- 🌐 Ancient mathematicians visualized problems using geometry, representing equations with shapes and areas.
- 🔢 Negative numbers were not initially recognized as valid solutions due to their incompatibility with the tangible realities of length, area, and volume.
- 🤼♂️ The competitive nature of 16th-century mathematics led to the secrecy surrounding the solutions to the cubic equation, as revealing them could jeopardize a mathematician's position.
- 🎓 Scipione del Ferro discovered a method to solve depressed cubics but kept it secret until his deathbed, when he shared it with his student Antonio Fior.
- 📈 Niccolo Fontana Tartaglia, known as Tartaglia, developed an algorithm to solve the depressed cubic and presented it as a poem to protect his discovery.
- 🔐 Gerolamo Cardano, after much persuasion, learned Tartaglia's method and expanded upon it to solve the general cubic equation, despite promising secrecy.
- 📖 Cardano's 'Ars Magna' introduced a geometric proof for each arrangement of the cubic equation and laid the groundwork for modern algebraic notation.
- 🌀 Imaginary numbers, first encountered in solving cubic equations, re-emerged in quantum physics, showing their fundamental role in describing the behavior of atoms.
Q & A
What was the initial purpose of mathematics according to the transcript?
-The initial purpose of mathematics was to quantify our world, measure land, predict the motions of planets, and keep track of commerce.
What problem in mathematics was considered impossible and how was it approached differently?
-The cubic equation was considered impossible. The secret to solving it involved separating math from the real world, splitting algebra from geometry, and inventing new numbers called imaginary numbers.
Who published 'Summa de Arithmetica' and what was its significance?
-Luca Pacioli, Leonardo da Vinci's math teacher, published 'Summa de Arithmetica' in 1494. It was a comprehensive summary of all mathematics known in Renaissance Italy at the time.
What was the conclusion of Luca Pacioli regarding the cubic equation?
-Luca Pacioli concluded that a solution to the cubic equation was impossible.
How did ancient mathematicians visualize and solve quadratic equations?
-Ancient mathematicians visualized quadratic equations using words and pictures, thinking of the x squared term as a literal square and other terms as rectangles, and used geometric methods to solve them.
What was the significance of the negative numbers to ancient mathematicians?
-Negative numbers were not considered real or valid by ancient mathematicians because they were dealing with tangible quantities like lengths, areas, and volumes, and the concept of a negative length or area did not make sense in their real-world applications.
Who were the key figures in the development of solving cubic equations and what were their contributions?
-Key figures in the development of solving cubic equations include Omar Khayyam, who identified 19 different cubic equations; Scipione del Ferro, who found a method to solve depressed cubics; Antonio Fior, who learned the secret from del Ferro; and Niccolo Fontana Tartaglia, who developed an algorithm to solve the depressed cubic and later contributed to solving the general cubic equation.
How did Gerolamo Cardano contribute to the solution of the cubic equation?
-Gerolamo Cardano discovered a method to solve the general cubic equation, including the x squared term, by transforming it into a depressed cubic using a substitution, which could then be solved using Tartaglia's formula.
What was the significance of Rafael Bombelli's work on cubic equations?
-Rafael Bombelli made a significant contribution by finding a way to work with the square roots of negative numbers, which he treated as a new type of number. This allowed him to solve cubic equations that had previously led to geometric paradoxes.
How did the concept of imaginary numbers evolve and become fundamental in mathematics and physics?
-The concept of imaginary numbers, initially seen as a quirky intermediate step in solving cubic equations, evolved to become fundamental in mathematics and physics. They were popularized by Descartes as 'imaginary numbers' and later by Euler with the introduction of the letter 'i'. The unique properties of imaginary numbers, such as their role in rotation in the complex plane, made them essential in describing wave functions in quantum mechanics through the Schrödinger equation.
What is the role of imaginary numbers in the Schrödinger equation?
-In the Schrödinger equation, imaginary numbers play a crucial role as they allow the equation to describe wave functions that correspond to the quantized orbits in the Bohr model of the atom, forming the basis of our understanding of atomic behavior and much of chemistry and physics.
How does the Brilliant platform enhance learning in STEM fields?
-The Brilliant platform enhances learning in STEM fields by using interactivity and visual manipulation of concepts. It offers a variety of courses that increase in difficulty and provide learners with the opportunity to engage with the material directly, supported by helpful hints when needed.
Outlines
📚 The Birth of Mathematics and the Conundrum of the Cubic Equation
This paragraph delves into the origins of mathematics as a tool for quantifying the world, from measuring land to predicting celestial motions. It highlights the historical challenge of solving cubic equations, which baffled ancient civilizations. The narrative pivots on the revolutionary idea of detaching math from reality, leading to the invention of imaginary numbers. It introduces Luca Pacioli's "Summa de Arithmetica" and the cubic equation's long-standing unsolved status until the Renaissance.
🔍 The Secret Solution to the Cubic Equation
The paragraph narrates the secretive discovery of a method to solve depressed cubic equations by Scipione del Ferro, a mathematics professor at the University of Bologna. It discusses the competitive and often perilous nature of being a mathematician in the 1500s, where job security depended on solving problems that others could not. The story unfolds with del Ferro's student, Antonio Fior, who inadvertently reveals the secret to Niccolo Fontana Tartaglia, setting the stage for a dramatic mathematical duel.
🏆 Tartaglia's Triumph and the Quest for a General Solution
This section details Tartaglia's victory over Fior in a mathematical challenge, showcasing his mastery over solving depressed cubic equations. Tartaglia's innovative approach of extending the concept of completing the square into three dimensions is highlighted. The narrative then follows Gerolamo Cardano's relentless pursuit to learn Tartaglia's method, leading to the development of a general solution for all cubic equations, despite the oath he made to Tartaglia.
🤯 The Emergence of Imaginary Numbers and Complex Plane
The paragraph explores the introduction of imaginary numbers and their role in solving cubic equations. It recounts the initial skepticism and the eventual acceptance of these numbers as an essential part of mathematics. The story of Rafael Bombelli, who first embraced the concept of negative areas and square roots of negatives, is highlighted. The paragraph also touches on the evolution of algebra and geometry, leading to the modern understanding of complex numbers and their significance in physics, particularly in Schrödinger's equation.
🌀 The Role of Complex Numbers in Quantum Mechanics
This final paragraph discusses the surprising application of imaginary numbers in quantum mechanics, specifically in Schrödinger's wave equation. It explains how the complex numbers, initially seen as an abstract mathematical tool, are fundamental to our understanding of atomic behavior. The narrative concludes by reflecting on the transformative journey of mathematics from its concrete origins to the abstract yet powerful realm of complex numbers, which have become indispensable in describing the universe.
Mindmap
Keywords
💡Mathematics
💡Algebra
💡Geometry
💡Imaginary Numbers
💡Cubic Equations
💡Scipione del Ferro
💡Niccolo Fontana Tartaglia
💡Gerolamo Cardano
💡Completing the Square
💡Complex Numbers
💡Schrödinger Equation
Highlights
Mathematics originated as a tool for quantifying the world, including measuring land and predicting celestial motions.
The solution to an impossible problem in mathematics was to disconnect it from reality, leading to the invention of imaginary numbers.
Luca Pacioli, Leonardo da Vinci's math teacher, published 'Summa de Arithmetica' in 1494, summarizing all known mathematics in Renaissance Italy.
Ancient civilizations, including Babylonians, Greeks, Chinese, Indians, Egyptians, and Persians, could not solve the cubic equation.
Omar Khayyam, a Persian mathematician, identified 19 different cubic equations but did not find a general solution.
Scipione del Ferro discovered a method to solve depressed cubics but kept it a secret to secure his job.
Antonio Fior, del Ferro's student, revealed the secret of solving depressed cubics on del Ferro's deathbed.
Niccolo Fontana Tartaglia solved all 30 depressed cubics given by Fior in two hours, securing his position as a respected mathematician.
Tartaglia developed an algorithm to solve the depressed cubic, which he wrote as a poem to avoid revealing the method.
Gerolamo Cardano managed to extract Tartaglia's method and discovered a solution to the full cubic equation.
Cardano published 'Ars Magna,' an updated compendium of mathematics, including a chapter on solving the cubic equation.
Cardano encountered cubic equations that led to the concept of square roots of negative numbers, which he initially dismissed as useless.
Rafael Bombelli, an Italian engineer, embraced the square roots of negatives and developed a method to solve cubic equations involving them.
The development of complex numbers liberated algebra from geometry and allowed for the solution of real-world problems.
Imaginary numbers, initially seen as a quirk of mathematics, turned out to be fundamental to the description of reality, particularly in quantum physics.
The Schrödinger equation, which includes the square root of negative one, is fundamental to quantum mechanics and the understanding of atomic behavior.
The use of complex numbers in physics was a surprise discovery, showing that nature operates with complex numbers rather than just real numbers.
Brilliant, the sponsor of the video, is a platform that teaches STEM concepts through interactive learning, allowing for a deeper understanding of subjects like calculus and complex numbers.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: