Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (8 of 92) Meaning of the Wave Eqn.

Michel van Biezen
4 Feb 201704:24
EducationalLearning
32 Likes 10 Comments

TLDRThe video delves into the physical meaning of the wave equation and its role in quantum mechanics. It explains that the wave equation, which is not a real quantity, allows for the calculation of the probability of finding a particle at a specific location. The script clarifies that while the wave equation does not represent a physical wave or a real function, it is a crucial tool in quantum mechanics for understanding particle behavior and probabilities.

Takeaways
  • 🌊 The wave equation is a fundamental concept in quantum mechanics, representing the physical behavior of particles under certain constraints.
  • 📐 The script begins by discussing the Schrödinger equation, which is a differential equation that operates on the wave function.
  • 🔄 The wave equation should satisfy the Schrödinger equation, just like any solution must satisfy its corresponding differential equation.
  • 🎲 The wave equation is initially introduced in the context of a particle with mass M in a single dimension, subject to various physical forces and energies.
  • 🌀 The wave equation is written as a function of position and time, and when plugged into the Schrödinger equation, it should satisfy it.
  • 📈 The script presents a specific form of the wave equation involving a constant, e to the power of (I KX - Omega T), where I is the imaginary unit.
  • 🚫 Despite its appearance, the wave equation does not represent a real, measurable quantity or a classic wave function in quantum mechanics.
  • 🎱 The wave equation is crucial for calculating the probability of finding a particle in a particular location, rather than representing the particle's position or movement directly.
  • 📊 The script clarifies that the wave equation, in its complex form, is a vehicle for calculating probabilities in a one-dimensional case.
  • 🔑 The technique for using the wave equation to calculate probabilities will be explained in subsequent videos.
  • 🤔 The concept that the wave equation does not represent a real wave or quantity is a challenging aspect of quantum mechanics, but it is essential for understanding particle behavior.
Q & A

  • What is the primary purpose of the wave equation in quantum mechanics?

    -The primary purpose of the wave equation in quantum mechanics is to provide a means to calculate the probability of finding a particle in a particular location, rather than representing a measurable classical wave function.

  • How is the wave equation related to the Schrödinger equation?

    -The wave equation satisfies the Schrödinger equation, which is a fundamental differential equation in quantum mechanics that describes how the quantum state of a physical system changes with time.

  • What does the wave equation represent in the context of a single particle in a one-dimensional environment?

    -In the context of a single particle in a one-dimensional environment, the wave equation represents the quantum state of the particle, which is a complex-valued function of position and time.

  • Why does the wave equation contain the imaginary unit 'i'?

    -The imaginary unit 'i' is present in the wave equation because it allows for the representation of complex numbers, which are necessary for describing quantum states that cannot be represented by real numbers alone.

  • What happens to the wave equation when there are no forces acting on the particle and the potential energy is constant?

    -When there are no forces acting on the particle and the potential energy is constant, the Schrödinger equation reduces to a simpler form, and the wave equation can be used to calculate the probability distribution of the particle's location.

  • How does the wave equation differ from classical wave equations?

    -The wave equation in quantum mechanics is not a real quantity and does not represent a physical wave like classical wave equations do. Instead, it provides a way to calculate probabilities in quantum systems.

  • What is the significance of the probability aspect in the wave equation?

    -The probability aspect is significant because it allows us to predict the likelihood of finding a particle at a specific location, which is a core concept in understanding quantum behavior and is different from classical physics where the exact position and momentum of a particle can be precisely known.

  • What does the wave equation look like for a particle with no net force acting on it?

    -For a particle with no net force acting on it, the wave equation takes the form of a constant times e to the power of (I KX - Omega T), where I is the imaginary unit, K is the wave number, X is the position, Omega is the angular frequency, and T is time.

  • How can the wave equation be expressed in terms of cosine and sine functions?

    -The wave equation can be expressed in terms of cosine and sine functions by using Euler's formula, which allows the transformation of the exponential form (e^(I KX - Omega T)) into a combination of cosine and sine functions, representing the wave's amplitude and phase.

  • What is the role of the potential energy (V) in the context of the wave equation?

    -In the context of the wave equation, the potential energy (V) is a term that represents the energy of the system due to its position in a force field. When V is constant, it simplifies the Schrödinger equation, allowing the wave equation to be used for calculating probabilities.

  • How does the total energy of a particle in the wave equation relate to its kinetic and potential energy?

    -In the wave equation, the total energy of a particle is the sum of its kinetic and potential energy. This is reflected in the Schrödinger equation, where the kinetic energy term and the potential energy term together determine the form of the wave equation.

Outlines
00:00
🌊 Introduction to Wave Equation in Quantum Mechanics

This paragraph introduces the concept of the wave equation in the context of quantum mechanics. It begins by discussing the physical meaning of the wave equation and its representation of a particle subjected to various physical constraints such as forces, potential energy, and kinetic energy. The paragraph then connects the wave equation to the Schrödinger equation, highlighting the role of the wave equation in describing the probability of finding a particle at a particular location rather than representing a physical wave or a measurable classical wave function. The importance of understanding the wave equation's role in quantum mechanics is emphasized, as it provides a means to calculate probabilities in a quantum system.

Mindmap
Keywords
💡Wave Equation
The wave equation in the context of this video is a mathematical function that describes the behavior of a wave as a function of space and time. It is fundamental to understanding the dynamics of waves in various physical contexts, including quantum mechanics. The video explains that the wave equation, when applied to a particle in quantum mechanics, does not directly represent the physical wave of the particle but is used to derive probabilities of finding the particle at a certain position and time.
💡Schrodinger Equation
The Schrodinger Equation is a cornerstone of quantum mechanics, described in the video as a differential equation that governs the wave function of a quantum system. It's used to determine how quantum states evolve over time. The video clarifies that for a wave function to be meaningful in quantum mechanics, it must satisfy the Schrodinger Equation, which in turn provides insights into the physical properties and behavior of quantum particles.
💡Differential Equation
A differential equation is a mathematical equation that relates some function with its derivatives. In the context of the video, the Schrodinger Equation is a type of differential equation applied to the wave function of a particle. This relationship is pivotal in quantum mechanics for analyzing the dynamics of particles under various forces and potentials, illustrating how changes in the system can be predicted over time.
💡Particle
In the video, a 'particle' refers to a small object that can be described by quantum mechanics. The discussion revolves around understanding the properties and behavior of such particles when subjected to different physical conditions. The wave equation is used to calculate probabilities concerning the particle's location, highlighting quantum mechanics' probabilistic nature in predicting particle behavior.
💡Potential Energy
Potential energy, denoted as V in the video, represents the energy held by an object due to its position relative to other objects, stresses within itself, its electric charge, or other factors. The video discusses how the potential energy influences the Schrodinger Equation and, consequently, the behavior of particles in a quantum system. A constant potential energy scenario simplifies the equation, allowing for more straightforward analysis of the particle's quantum state.
💡Kinetic Energy
Kinetic energy in the context of this video refers to the energy that a particle possesses due to its motion. The video relates this concept to classical mechanics, where kinetic and potential energies together define the total energy of a system. In quantum mechanics, the Schrodinger Equation incorporates kinetic energy to describe how the quantum state of a particle changes with time.
💡Real Solution
A 'real solution' in mathematics and physics refers to a solution that does not involve imaginary numbers. The video points out that the wave equation for a particle in quantum mechanics often involves complex numbers (involving the imaginary unit 'i'), which means its solutions are not 'real' in the typical mathematical sense. This characteristic emphasizes the probabilistic interpretation of quantum mechanics, as the wave function itself isn't directly observable but relates to the likelihood of finding a particle in a specific state.
💡Probability
Probability in this video is used in the context of finding a quantum particle at a particular location and time. It explains that while the wave equation does not directly represent the probability, it facilitates calculating the probability of a particle's position. This aspect underscores quantum mechanics' probabilistic nature, distinguishing it from classical mechanics' deterministic approach.
💡Complex Numbers
Complex numbers are a type of number that include an imaginary component (sqrt(-1), denoted as 'i'). The video discusses how the wave equation for a quantum particle involves complex numbers, making the solution non-real. This incorporation of complex numbers is crucial for accurately describing quantum phenomena, even though it complicates the physical interpretation of the wave function.
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the physical properties of nature at the scale of atoms and subatomic particles. The video focuses on how quantum mechanics uses the wave equation and the Schrodinger Equation to predict the behavior of particles. Unlike classical mechanics, quantum mechanics relies on probabilities to describe how particles behave, reflecting the inherent uncertainties in measuring quantum systems.
Highlights

Exploring the physical meaning of the wave equation.

The wave equation's representation of a particle under physical constraints.

The connection between the wave equation and the Schrödinger equation.

The role of the wave equation in satisfying the Schrödinger equation.

The wave equation's non-real nature and its implications for quantum mechanics.

The use of complex numbers (I) in the wave equation.

The reduction of the Schrödinger equation to a constant potential scenario.

The transition from the Schrödinger equation to a classical mechanics equation.

The wave equation's ability to calculate the probability of finding a particle at a location.

The distinction between the wave equation and a measurable classical wave function.

The importance of the wave equation in quantum mechanics for probability calculation.

The non-intuitive aspect of the wave equation in quantum mechanics.

The upcoming explanation of the technique to calculate probability using the wave equation.

The fundamental role of the wave equation in understanding quantum mechanics.

The challenge of imagining a wave equation that doesn't represent a real wave in quantum mechanics.

Transcripts

Browse More Related Video

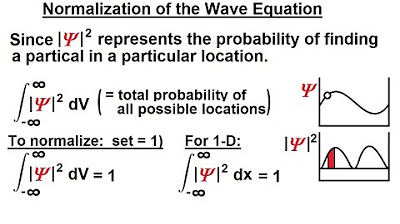
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (2 of 92) Normalization of the Wave Equation

The Quantum Wavefunction Explained

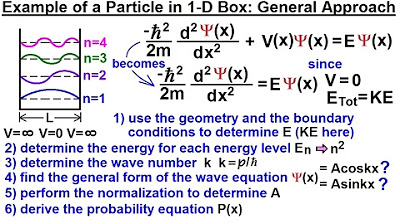
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

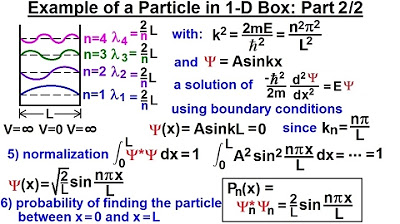
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

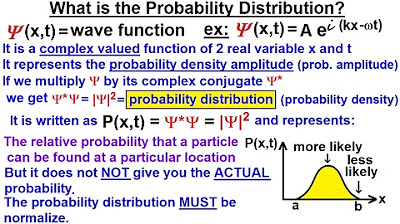
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (9 of 92) What is the Prob Distribution?

Lecture 3: The Wave Function

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsWaveEquationSchrodingerEquationProbabilityCalculationPhysicalConstraintsParticlePhysicsNonRealSolutionQuantumBehaviorOneDimensionalPhysicsEducation