AP Calculus BC Lesson 9.2

Elizabeth Fein
14 Apr 202312:49
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript delves into the concept of second derivatives in the context of parametric equations. It explains the mathematical process of obtaining the second derivative of a curve defined by parametric equations, using the formula involving differentiation with respect to the parameter 't'. The transcript provides step-by-step examples of how to calculate the second derivative, including finding the first derivative and applying the chain rule. It also discusses how to determine the concavity of a curve by analyzing the sign of the second derivative, with specific examples illustrating the process and the points of inflection.

Takeaways
  • πŸ“š The second derivative of a function is calculated by taking the derivative of the first derivative.
  • πŸ”„ For parametric equations, the second derivative with respect to X is given by the formula: D(D(y)/D(t))/D(x)/D(t).
  • 🌐 When finding the second derivative of a parametric curve, include the parameter 't' in the calculations.
  • πŸ“ To find the first derivative 'dy/dx' for parametric equations, use the relationship dy/dt = (dy/dx) / (dx/dt).
  • πŸ”’ Practice the formula with examples to solidify understanding, such as finding the second derivative of y with respect to X for given parametric equations.
  • πŸŽ“ Memorize the formula for the second derivative of a parametric curve for use in exams like the AP exam.
  • πŸ“Œ Substitute specific values of the parameter 't' to evaluate the second derivative at particular points.
  • πŸ“Š Determine the concavity of a parametric curve by analyzing the sign of the second derivative.
  • πŸ“ˆ Use a sign chart to identify intervals of concavity and points of inflection for a parametric curve.
  • 🌟 Remember that a positive second derivative indicates concavity up (convex down), while a negative second derivative indicates concavity down (convex up).
Q & A

  • What is the mathematical formula for the second derivative of a function with respect to X?

    -The second derivative of a function with respect to X is given by the formula dΒ²y/dXΒ² = d/dx(dy/dx), which means you take the derivative of the first derivative with respect to X.

  • How does the process of finding the second derivative change when dealing with parametric equations?

    -When dealing with parametric equations, the second derivative of a curve is found using the formula D(dy/dx)/DT, where DT represents the derivative with respect to the parameter T, and DX is the derivative with respect to X.

  • What is the first step in finding the second derivative of a parametric curve?

    -The first step is to find the first derivative dy/dx using the parametric equations by taking the derivative of Y with respect to T (dy/dt) and dividing it by the derivative of X with respect to T (dx/dt).

  • How do you find the first derivative dy/dx for the parametric equations X(T) = 2T^3 + 6 and Y(T) = T^4?

    -For X(T) = 2T^3 + 6, the derivative dx/dt is 6T^2. For Y(T) = T^4, the derivative dy/dt is 4T^3. Then, dy/dx is calculated as dy/dt divided by dx/dt, which gives (4T^3) / (6T^2), simplifying to 2/(3T).

  • What is the second derivative of y with respect to X for the parametric equations X(T) = 2T^3 + 6 and Y(T) = T^4?

    -The second derivative is found using the formula D(dy/dx)/DT. After calculating the first derivative dy/dx = 2/(3T) and its derivative with respect to T, the second derivative is (2/3) * (-2/T^2) / (6T^2), which simplifies to -1/(9T^2).

  • How can you determine the concavity of a parametric curve?

    -To determine the concavity, you find the second derivative of the curve. If the second derivative is positive at a point, the curve is concave up at that point. If it's negative, the curve is concave down.

  • What is the second derivative of y with respect to X for the parametric equations X(T) = T^3 + 4 and Y(T) = ln(T)?

    -The first derivative dy/dx is (1/T) / (3T^2). The second derivative is then D(dy/dx)/DT = (1/3T^3) / (3T^2), which simplifies to -1/(3T^5).

  • How do you find the second derivative of y with respect to X at a specific value of T?

    -You first find the general form of the second derivative, and then substitute the specific value of T into that formula to calculate the second derivative at that point.

  • What is the second derivative of y with respect to X for the parametric equations X(T) = 4cos(T) and Y(T) = T at T = Ο€/3?

    -The first derivative dy/dx is -4sin(T)/5. The second derivative is D(dy/dx)/DT = (4cos(T))/(-4sin(T)^3). At T = Ο€/3, the second derivative is (4cos(Ο€/3))/(-4sin(Ο€/3)^3), which simplifies to -1/√3.

  • How do you determine the intervals of T on which a parametric curve is concave up or down?

    -You find the second derivative of the curve, set it equal to zero to find potential points of inflection, and then test values within the intervals to determine where the second derivative is positive (concave up) or negative (concave down).

  • For the curve C defined by X(T) = 5T and Y(T) = T^3 - 3T^2 - 1, what are the intervals of T where C is concave up and down?

    -The second derivative is 6(T^2 - 1)/25. Setting this equal to zero gives T = Β±1 as points of inflection. Testing values shows C is concave up on (1, ∞) and concave down on (-∞, -1) βˆͺ (1, ∞).

Outlines
00:00
πŸ“š Understanding Second Derivatives in Parametric Equations

This paragraph introduces the concept of finding the second derivative of a function, particularly when dealing with parametric equations. It explains the mathematical formula for the second derivative and how to apply it to parametric equations defined by X(t) and Y(t). The paragraph also includes a step-by-step example of calculating the second derivative of a parametric curve, emphasizing the importance of including the parameter 't' in the differentiation process. The explanation is geared towards students preparing for the AP exam, highlighting the need to memorize the formula for the second derivative in parametric form.

05:02
πŸ”’ Calculating Second Derivatives of Parametric Equations with Examples

This section delves into the practical application of calculating second derivatives of parametric equations. It provides a clear explanation of the process, starting with finding the first derivative and then using it to find the second derivative. The paragraph includes two examples: one where the parametric equations are given in terms of 't' and another where the 't' values are implicitly present in the equations. The examples demonstrate how to find the first derivative (dy/dx), set up the formula for the second derivative (d^2y/dx^2), and evaluate it at a specific 't' value. The paragraph emphasizes the importance of understanding the concavity of the curve, which is determined by the sign of the second derivative.

10:04
πŸ“ˆ Determining Concavity of Parametric Curves Using Second Derivatives

This paragraph focuses on using the second derivative to determine the concavity of parametric curves. It explains the process of finding the second derivative in terms of the parameter 't' and then using it to identify intervals where the curve is concave up or concave down. The paragraph provides a detailed example with parametric equations X(t) = 5t and Y(t) = t^3 - 3t^2 - 1, showing how to calculate the first derivative (dy/dx), set up and solve for the second derivative (d^2y/dx^2), and create a sign chart to determine the intervals of concavity. The explanation includes finding points of inflection and testing values within these intervals to determine the sign of the second derivative, ultimately concluding the intervals where the curve is concave up and down.

Mindmap
Keywords
πŸ’‘Second Derivative
The second derivative is a mathematical concept used to analyze the concavity of a function. It is the derivative of the first derivative. In the context of the video, the second derivative is used to determine the concavity of a parametric curve, which is crucial for understanding the shape and direction of the curve's tangents and normal lines.
πŸ’‘Parametric Equations
Parametric equations are a set of equations that define a curve in the coordinate plane by providing the coordinates of each point on the curve as a function of a third variable, known as the parameter. These equations are essential in the video for describing the shape of a curve and for finding its derivatives with respect to another variable.
πŸ’‘Derivatives
Derivatives are a fundamental concept in calculus that represent the rate of change of a function with respect to its independent variable. They are used to analyze various properties of functions, such as slopes, tangents, and inflection points. In the video, derivatives are used to find the rate of change of parametric equations and to determine the concavity of the curve.
πŸ’‘Concavity
Concavity is a geometric property of a curve that describes whether it bends upwards (concave up) or downwards (concave down). It is determined by the sign of the second derivative of a function. In the video, the concavity of parametric curves is analyzed by examining the sign of their second derivatives.
πŸ’‘Points of Inflection
A point of inflection is a point on a curve where the concavity changes, meaning the curve switches from concave up to concave down or vice versa. These points are significant in understanding the shape of a curve and are found where the second derivative of a function is zero or undefined.
πŸ’‘Chain Rule
The chain rule is a fundamental calculus technique used to find the derivative of a composite function. It states that the derivative of a function composed of two or more functions is the derivative of the outer function times the derivative of the inner function. In the video, the chain rule is applied when differentiating the first derivative to find the second derivative.
πŸ’‘Quotient Rule
The quotient rule is a calculus formula used to find the derivative of a quotient of two functions. It states that the derivative of a function f(t) divided by a function g(t) is the derivative of f(t) times g(t) minus f(t) times the derivative of g(t), all divided by the square of g(t). The video mentions the quotient rule in the context of differentiating parametric equations.
πŸ’‘Derivative with Respect to Parameter
In the context of parametric equations, taking a derivative with respect to a parameter means finding the rate of change of the function's output with respect to that parameter. This is crucial for analyzing the behavior of the curve described by the parametric equations and is the foundation for finding higher-order derivatives like the second derivative.
πŸ’‘Rate of Change
The rate of change is a fundamental concept in calculus that describes how a quantity changes with respect to another quantity. It is the derivative of a function at a particular point and represents the instantaneous rate of change or the slope of the tangent line at that point. In the video, the rate of change is used to analyze the behavior of parametric curves.
πŸ’‘Instantaneous Slope
Instantaneous slope is the slope of the tangent line to a curve at a specific point. It represents the steepness of the curve at that point and is found by taking the derivative of the function that defines the curve. In the context of the video, the instantaneous slope is used to describe the tangent to a parametric curve.
Highlights

The definition of the second derivative is the derivative of the first derivative.

The mathematical formula for the second derivative of y with respect to X is D^2y/Dx^2 = d^2y/dx^2.

For parametric equations, the second derivative of a curve is found using the formula D^2y/Dx^2 = (d/dt(dy/dx)) / (dx/dt).

When applying the second derivative formula to parametric equations, the parameter t must be included in the differentiation process.

The first derivative dy/dx is calculated by dividing dy/dt by dx/dt for parametric equations.

An example is provided where the second derivative of y with respect to X is calculated for the parametric equations X(t) = 2t^3 + 6 and Y(t) = t^4.

The second derivative can be found by first determining the first derivative and then applying the chain rule and quotient rule as necessary.

The concavity of a curve defined by parametric equations can be analyzed by evaluating the second derivative at specific values.

A second example is given where the second derivative of y with respect to X is found in terms of T for the parametric equations x = t^3 + 4 and y = ln(t).

The second derivative is used to determine the intervals of concavity for a curve defined by parametric equations.

The process of finding the intervals of concavity involves setting the second derivative equal to zero to find points of inflection.

The sign of the second derivative indicates whether the curve is concave up or down.

A third example involves finding the second derivative of y with respect to X at T = Ο€/3 for the parametric equations X(t) = 4cos(t) and Y(t) = t.

The second derivative is used to determine the intervals of t where the curve is concave up or down.

The final example involves finding the intervals of t where the curve C, defined by X(t) = 5t and Y(t) = t^3 - 3t^2 - 1, is concave up or down.

A sign chart is used to test the intervals of t and determine the concavity of the curve C.

The curve C is found to be concave up on the interval (1, ∞) and concave down on the interval (-∞, 1) based on the second derivative.

Transcripts

Browse More Related Video

Second Derivative of Parametric Equations with Example

Business Calculus - Math 1329 - Section 3.2 - Concavity and Points of Inflection

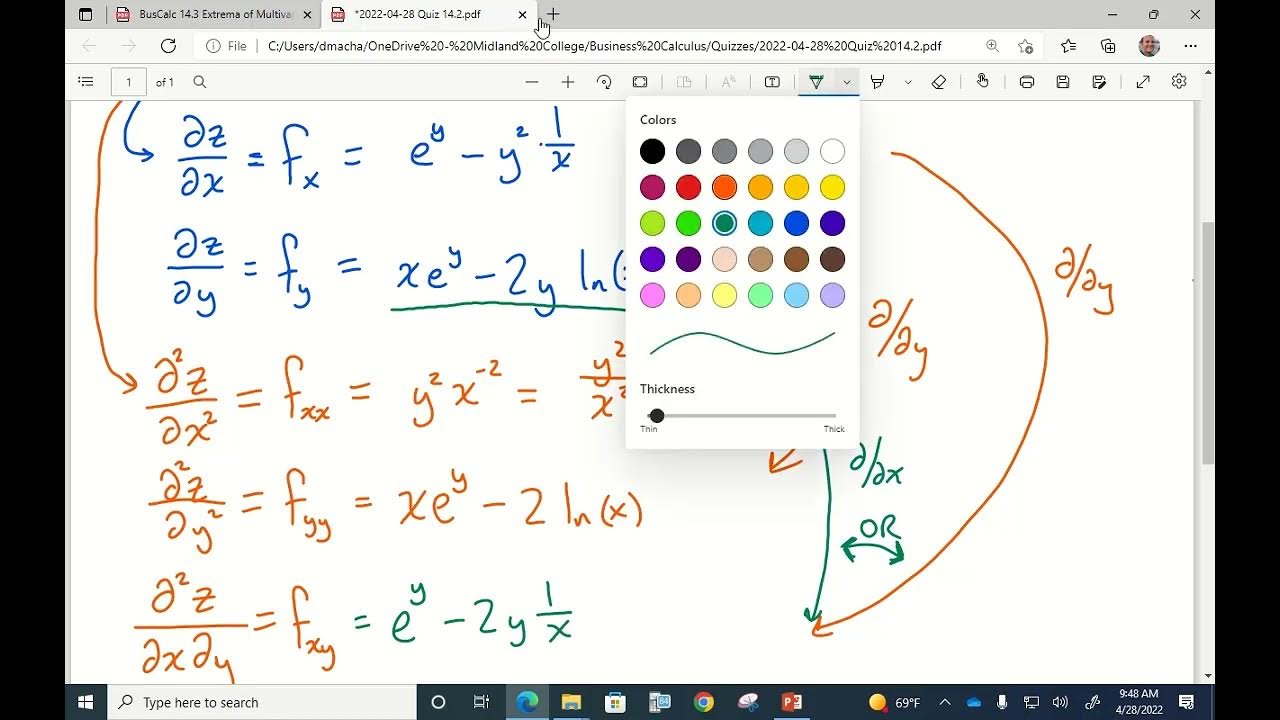
BusCalc 14.3 Extrema of Multivariable Functions

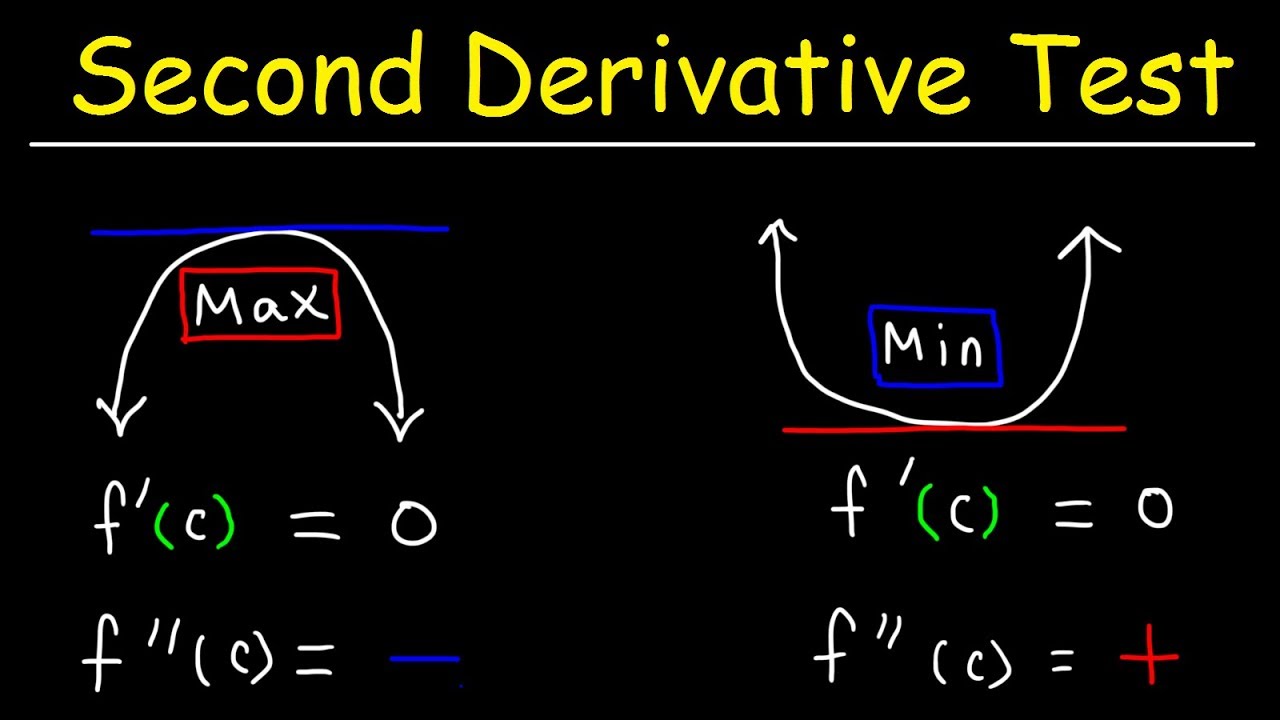
Second Derivative Test

Calculus 1 Lecture 3.4: The Second Derivative Test for Concavity of Functions

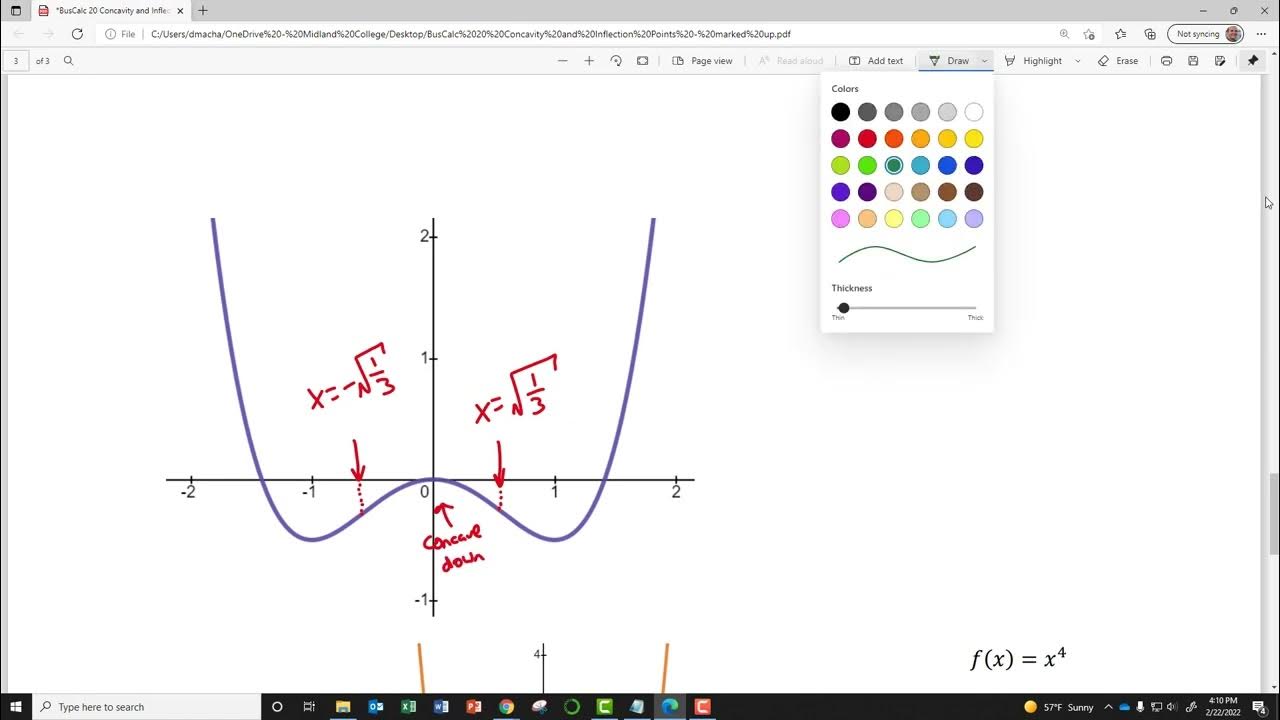
BusCalc 20 Concavity and Inflection Points

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ConceptsSecond DerivativesParametric EquationsConcavity AnalysisAdvanced MathCurve AnalysisMathematical FormulasAP Exam PrepEducational ContentOnline Learning