6 to the (3x + 5) = 1, many don’t know where to start
TLDRIn this informative video, John, a seasoned math teacher, introduces viewers to solving exponential equations using logarithms. He starts by posing a challenging problem involving exponents and logarithms, then proceeds to explain the concept of logarithms and their inverse relationship with exponential functions. John uses the 'bacon and eggs' mnemonic to help remember the relationship between the base, answer, and exponent in logarithmic expressions. He demonstrates the steps to solve a basic exponential equation and emphasizes the importance of practice and comprehensive instruction in mastering math. The video concludes with John solving the initial problem, showing that X equals -53, and encourages viewers to embrace the use of calculators for solving such equations.
Takeaways
- 🔢 The key skill discussed is solving algebraic equations, particularly exponential equations involving variables in the exponent.
- 💡 The problem presented is 6^(3x + 5) = 1, and the goal is to solve for the variable x.
- 🎓 John, an experienced math teacher, introduces himself and offers assistance for learning math through his program at TCM Academy.
- 📌 The correct answer to the problem is x = -53, which is derived using logarithms.
- 📚 Logarithms are introduced as the tool for solving exponential equations, with the concept explained through the 'bacon and eggs' analogy (base, answer, exponent).
- 🔍 The script provides a brief overview of the properties of logarithms and how they can be used to transform exponential equations into linear ones for easier solving.
- 🧠 The importance of understanding both exponential and logarithmic functions is emphasized, as they are inverse functions of each other.
- 📈 The use of a calculator is highlighted as crucial for solving exponential equations, especially when dealing with logarithms.
- 📖 The script touches on the historical context of solving such problems without calculators, using tables of logarithm values.
- 🚀 The video encourages viewers to subscribe to the channel for more comprehensive math instruction and to embrace the power of modern calculators in learning math.
- 🌟 The overarching message is that with dedication and the right instruction, anyone can overcome challenges in mathematics.
Q & A
What is the main topic of the video?
-The main topic of the video is solving exponential equations using logarithms in algebra.
What is the equation presented in the video?
-The equation presented in the video is 6^(3x + 5) = 1.
What is the solution to the presented equation?
-The solution to the equation is x = -53.
Who is the instructor in the video?
-The instructor in the video is John, who has been teaching middle and high school math for decades.
What is the significance of logarithms in solving exponential equations?
-Logarithms are significant in solving exponential equations because they are the inverse functions of exponentials, allowing for the conversion of an exponential equation into a linear equation that can be easily solved.
What is the 'bacon and eggs' concept mentioned in the video?
-The 'bacon and eggs' concept is a mnemonic for remembering the relationship between logarithms and exponents, where 'B' stands for base, 'A' stands for answer, and 'E' stands for exponent, helping to visualize the process of converting between exponential and logarithmic forms.
How does the instructor suggest solving the equation 4^Y = 10?
-The instructor suggests using logarithms to solve the equation 4^Y = 10. By taking the logarithm of both sides and applying the properties of logarithms, the variable Y can be isolated and solved for.
What is the role of a calculator in solving exponential equations?
-A calculator plays a crucial role in solving exponential equations by providing the necessary logarithmic values, which would otherwise require looking up in tables or performing complex calculations by hand.
Why is it important to understand logarithms and exponential functions?
-Understanding logarithms and exponential functions is important because they are fundamental concepts in algebra and higher levels of mathematics, often used to solve complex problems in various fields.
What is the final result when dividing both sides of the equation by log(6) in the example given?
-When dividing both sides of the equation by log(6), the result is 3x + 5 = 0, leading to the solution x = -53 after further simplification.
What advice does the instructor give for success in mathematics?
-The instructor advises that success in mathematics requires consistent work and comprehensive instruction. There are no shortcuts, and understanding the material requires time and effort, along with access to a good teacher or educational resources.
Outlines
📚 Introduction to Solving Exponential Equations
The speaker, John, introduces the topic of solving exponential equations in algebra. He presents a specific equation, 6^(3x + 5) = 1, and challenges the audience to solve it without a calculator. John emphasizes the importance of understanding how to solve such equations and offers a brief overview of his experience teaching math. He also promotes his math program at TCM academy and encourages the audience to engage with the content by liking and subscribing.
📈 Understanding Exponential Equations and Logarithms
John delves deeper into the concept of exponential equations, using the example 4^Y = 10. He explains the need to use logarithms to solve these equations and introduces the concept of logarithms, mentioning the log button on calculators. He uses a mnemonic, 'bacon and eggs', to help remember the relationship between logarithms and exponents. John simplifies the concept by explaining the parts of a power and how to convert an exponential equation into a logarithmic form using logarithms.
🔢 Solving Exponential Equations with Logarithms
The speaker provides a step-by-step guide on how to solve the exponential equation 4^Y = 10 using logarithms. He explains the process of taking the logarithm of both sides of the equation and using the properties of logarithms to isolate the variable. John also discusses the importance of having a solid understanding of logarithms and exponential functions, and he encourages viewers to subscribe to his YouTube channel for more comprehensive math instruction.
📖 Simplifying Logarithmic Expressions
John continues the discussion on logarithms by explaining how to simplify logarithmic expressions. He uses the example of log₆ = log₁ and demonstrates how to divide both sides of the equation by log₆ to isolate the variable. He emphasizes the importance of knowing certain logarithmic values, such as log₁ = 0, and explains the concept of common logarithms (base 10). The speaker also reflects on the historical context of solving logarithmic problems without calculators.
🎓 Final Steps in Solving Exponential Equations
In the final part of the video script, John concludes the process of solving the exponential equation 6^(3x + 5) = 1. He explains that log₁ = 0 simplifies the equation to 3x + 5 = 0, leading to the solution x = -5/3. The speaker highlights the ease of using calculators in modern times compared to the past when students had to use tables of logarithmic values. He wraps up by encouraging viewers to appreciate the convenience of calculators and to continue learning math with his help.
Mindmap
Keywords
💡Algebra
💡Exponential Equations
💡Logarithms
💡Solving Equations
💡Variables
💡Exponents
💡Logarithmic Properties
💡Calculator
💡Mathematics Adventures
💡Teaching Mathematics
Highlights
The key skill discussed is solving equations in algebra, particularly exponential equations involving variables in the exponent.
The problem presented is solving the equation 6^(3x + 5) equals 1 for the variable x.
The solution to the problem is x equals -53, which is a straightforward calculation using logarithms.
The video introduces John, a middle and high school math teacher with decades of experience.
John emphasizes the importance of understanding logarithms to solve exponential equations.
A brief introduction to logarithms is provided, explaining their relationship with exponential functions.
The concept of 'bacon and eggs' is introduced as a mnemonic for understanding logarithms, where B^A = E stands for log base B of A equals E.
The video explains how to convert an exponential equation into a logarithmic form using logarithms.
John provides a simple example of solving an exponential equation (4^Y = 10) using logarithms.
The properties of logarithms are discussed, such as the ability to move the exponent in front of the log when taking the log of a power.
The video emphasizes the importance of having comprehensive instruction and practice in mathematics to be successful.
John invites viewers to subscribe to his YouTube channel for more math content and assistance.
The process of solving the given exponential equation (6^(3x + 5) = 1) is walked through step by step.
The video highlights the historical context of solving logarithmic and exponential problems without calculators.
The importance of understanding logarithms and exponential functions as inverse functions is stressed.
The video concludes with a reminder to appreciate the convenience of modern calculators when studying mathematics.
Transcripts
Browse More Related Video

2 to the x = 9, many don’t know where to start

Solving Exponential Equations with Common Bases (Precalculus - College Algebra 54)
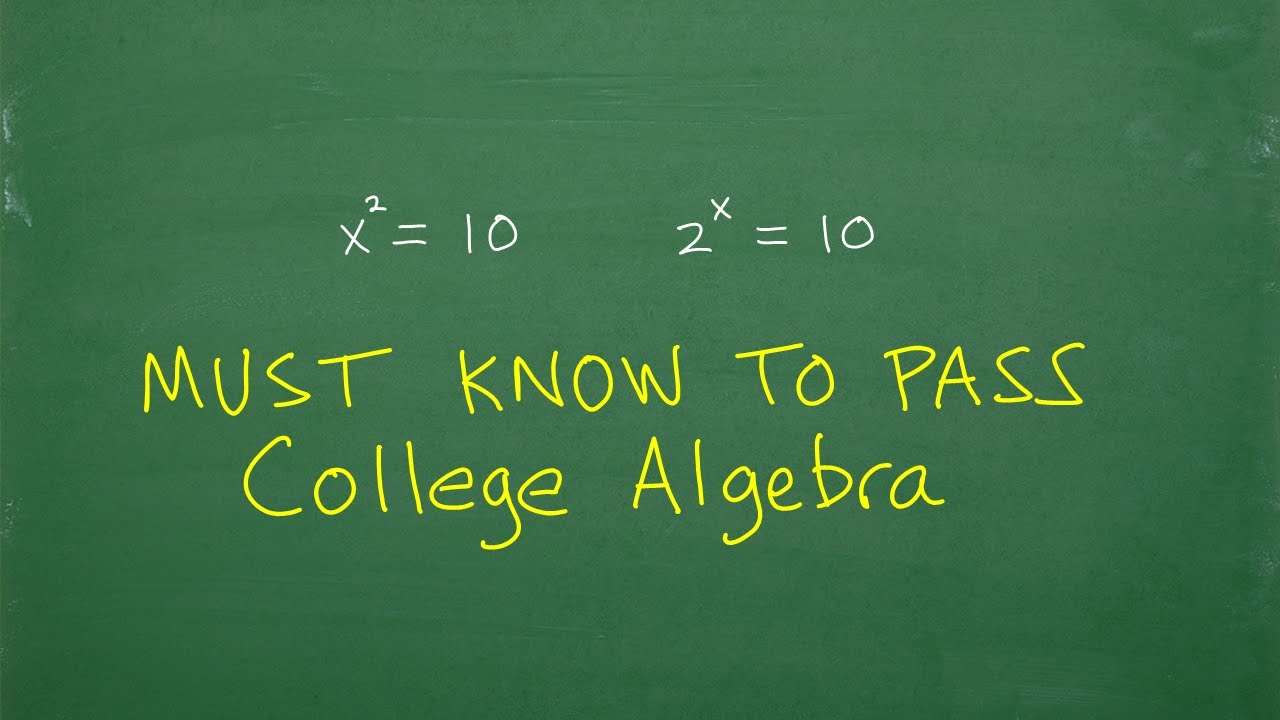
Want to PASS College Algebra? Absolutely, better understand this…

Using Logarithms and Natural Logarithms in Chemistry
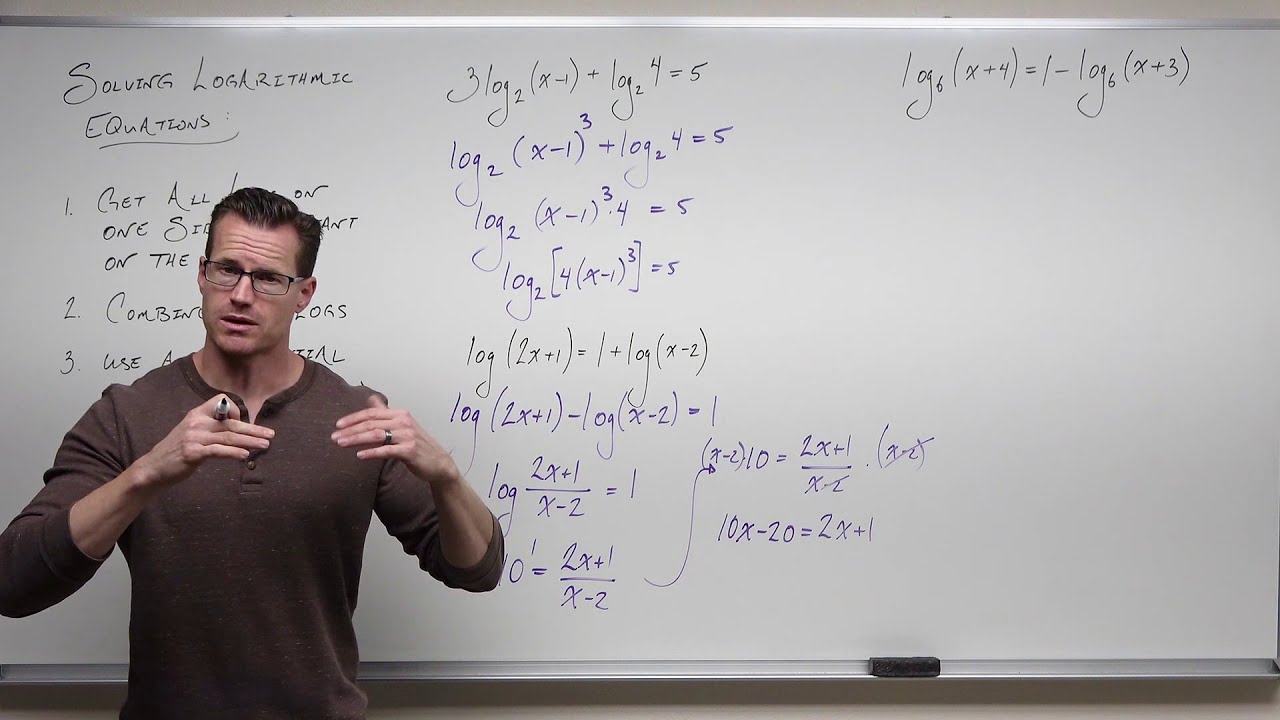
Solving Logarithmic Equations with Exponentials (Precalculus - College Algebra 63)

Introduction to Solving Logarithms and Exponentials (Precalculus - College Algebra 57)
5.0 / 5 (0 votes)
Thanks for rating: