Solving Logarithmic Equations with Exponentials (Precalculus - College Algebra 63)
TLDRIn this comprehensive video, the presenter delves into the intricacies of solving logarithmic equations, building on from a previous video that focused on a special case involving logarithms of the same base. The video is structured to first address equations with at least one non-logarithmic term, emphasizing the need to isolate all logarithmic terms on one side of the equation and constants on the other. The presenter then transitions into more challenging examples, highlighting the utility of the change of base formula to simplify complex problems. Throughout the video, the importance of converting logarithmic equations into exponential form is underscored, as exponentials are the inverse function that can solve logarithms. The narrative is interspersed with practical examples, demonstrating how to combine logarithms, check for domain issues, and apply algebraic techniques to find solutions. The video concludes with a teaser for the next segment, which will explore solving exponential equations, promising a continuation of the same problem-solving strategies.
Takeaways
- 📚 When solving logarithmic equations, if all terms are logarithms with the same base, you can combine them and set the insides equal to each other.
- 🔍 If there is at least one term that is not a logarithm, combine all logarithmic terms on one side of the equation and use exponential notation to rewrite and solve.
- 🧮 The change of base formula is crucial when dealing with logarithms of different bases; it allows you to convert them into a common base for easier solving.
- ✅ Always check for domain issues when plugging solutions back into the original equation to ensure that the solutions are valid and do not result in negative or zero values inside logarithms.
- 📈 Exponential notation is the inverse operation to logarithms and is used to solve logarithmic equations by setting the base to the power of the exponent equal to the argument of the logarithm.
- 🤔 In complex logarithmic equations, it's often helpful to factor or simplify terms before distributing to avoid unnecessary complications.
- 📊 When faced with logarithms of different bases that cannot be easily converted to a common base, consider using the natural logarithm (ln) as an intermediate step to combine terms.
- 🧷 Identifying and using the properties of logarithms, such as the product rule (log(a) + log(b) = log(a*b)), is essential for solving more challenging logarithmic equations.
- 🔗 The change of base formula is not only for converting between different bases but can also be used strategically to select a base that simplifies the equation.
- ⚖️ When combining logarithms, remember that coefficients become exponents, and you must respect the order of operations to avoid mathematical errors.
- 🔑 The concept of logarithms and exponents being inverse operations is fundamental to solving equations involving both; understanding this relationship is key to finding solutions.
Q & A
What is the general approach when solving logarithmic equations with at least one term that is not a logarithm?
-When solving logarithmic equations with at least one term that is not a logarithm, the approach is to combine all the logarithmic terms into one term on one side of the equation and leave the constant term on the other side. Then, use exponential notation to rewrite it and solve using algebraic techniques.
How can the change of base formula be used to simplify logarithms with different bases?
-The change of base formula can be used to convert logarithms with different bases into a common base that simplifies the equation. This is particularly useful when the original bases do not share a common factor or are not easily reducible to a simpler form.
What is the first step in solving a logarithmic equation that does not have all terms with the same logarithmic base?
-The first step is to group all logarithmic terms on one side of the equation and isolate any constant terms on the other side. This prepares the equation for the use of exponential notation to solve for the variable.
Why is it advised not to distribute logarithms with addition until absolutely necessary?
-Distributing logarithms with addition until absolutely necessary is advised against because it can sometimes lead to unnecessary extra work. It's often possible to simplify the equation or find a shortcut by first combining the logarithms and then, if necessary, distributing them.
How does the process of solving logarithmic equations relate to the process of solving exponential equations?
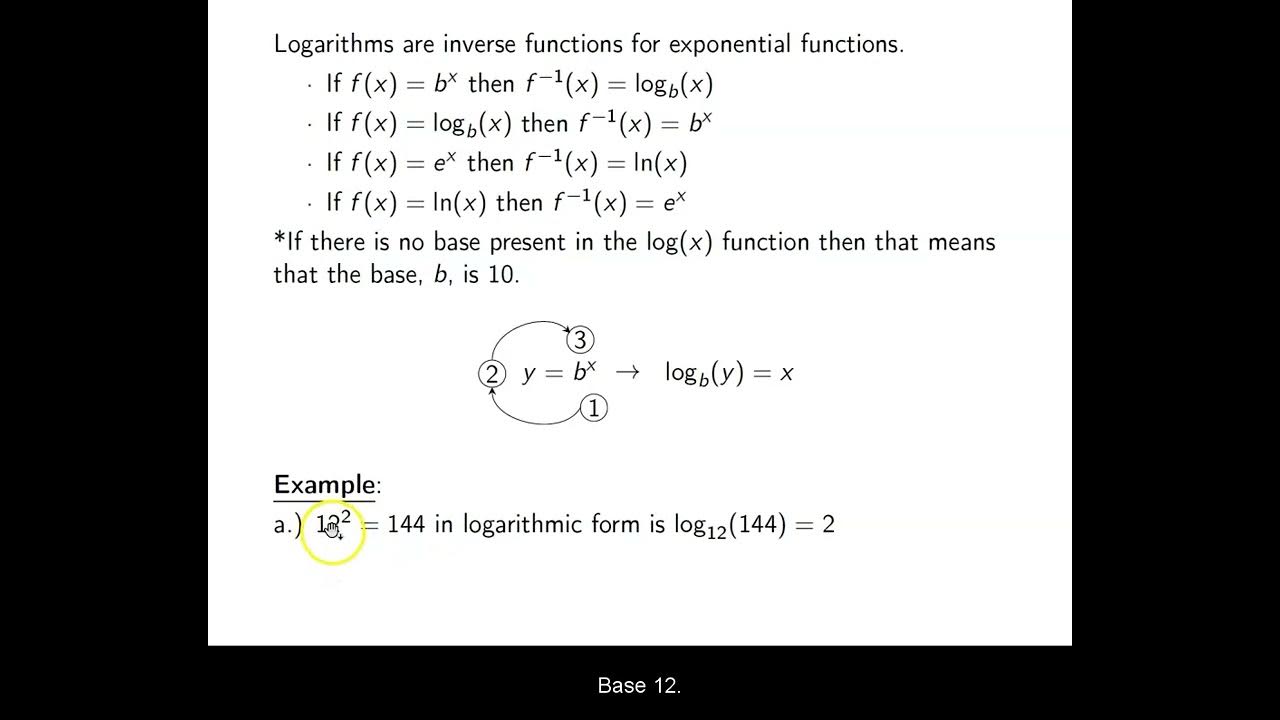
-Solving logarithmic equations is closely related to solving exponential equations because logarithms are the inverse of exponentiation. When solving logarithmic equations, one often needs to convert the equation into exponential form to use the properties of exponents and solve for the variable.
What is the zero product property, and how is it used in solving logarithmic equations?
-The zero product property states that if a product of factors equals zero, then at least one of the factors must be zero. In the context of solving logarithmic equations, this property is used to find solutions when an equation is factorable, allowing for the isolation of variable terms set equal to zero.
What is the importance of checking for domain issues when solving logarithmic equations?
-Checking for domain issues is important because logarithms are only defined for positive arguments. When solving logarithmic equations, one must ensure that the solutions do not result in negative or zero values inside the logarithms, as these would be outside the domain of the logarithmic function.
How can one determine if a logarithmic equation is solvable using common bases?
-A logarithmic equation is solvable using common bases if all the logarithmic terms share the same base. If this is the case, one can combine the logarithmic terms and set the insides equal to each other to solve the equation.
What is the role of the change of base formula in solving logarithmic equations with different bases?
-The change of base formula allows one to convert logarithms with different bases into a single, common base, which can then be more easily manipulated and solved. This is particularly useful when the original bases do not allow for straightforward simplification or combination of terms.
Why is it beneficial to factor logarithms, especially when dealing with fractions, in logarithmic equations?
-Factoring logarithms, especially when they involve fractions, can simplify the equation and make it easier to solve without affecting the final solution. This process can eliminate the need for complex algebraic manipulations and reduce the chances of making errors during the solution process.
How does the concept of exponentiation help in solving logarithmic equations?
-Exponentiation helps in solving logarithmic equations because it is the inverse operation of logarithms. By converting a logarithmic equation into an equivalent exponential equation, one can leverage the properties of exponents to isolate and solve for the variable.
Outlines
📚 Introduction to Solving Logarithmic Equations
This paragraph introduces the topic of solving logarithmic equations, emphasizing that the video will cover various methods, including handling cases where not all terms are logarithms. It sets the stage for a deeper dive into more challenging examples later in the video and outlines the general approach of combining logarithmic terms and using exponential notation to solve equations.
🔍 Simplifying Logarithmic Expressions with Exponential Notation
The second paragraph focuses on translating logarithmic expressions into exponential notation for easier solving. It discusses the process of combining multiple logarithms with the same base and then isolating the variable to a power, which can be solved using known algebraic techniques. The importance of waiting to distribute until necessary is highlighted to avoid unnecessary complexity.
🧩 Combining Logarithms and Using Exponentials
This section delves into the process of combining logarithms with the same base and then using exponential notation to express and solve the equation. It advises on when to distribute terms within logarithms and emphasizes the utility of the change of base formula, especially when dealing with more complex logarithmic equations.
🔑 The Role of Exponentials in Solving Logarithmic Equations
The fourth paragraph underscores the necessity of using exponentials to solve logarithmic equations. It explains that when logarithms cannot be combined due to different bases or the presence of non-logarithmic terms, the approach involves isolating logarithms on one side and constants on the other. The paragraph also discusses the importance of checking for domain issues when plugging solutions back into the original equation.
🌟 Advanced Techniques for Solving Complex Logarithmic Equations
The fifth paragraph introduces more advanced techniques for solving complex logarithmic equations, particularly those involving different bases. It discusses the use of the change of base formula to transform logarithms into a more manageable form and the application of factoring and distribution to simplify the equations before solving.
📝 Grouping and Factoring in Logarithmic Equations
This paragraph emphasizes the importance of grouping and factoring logarithmic terms, especially when dealing with fractions. It provides an example where simplifying the argument of a logarithm before algebraic manipulation can make the problem easier to solve. The paragraph also illustrates different methods to handle logarithms with the same base and how to approach equations with non-logarithmic terms.
🧠 Advanced Problem Solving with Logarithms and Exponentials
The sixth paragraph presents a more complex scenario involving logarithms with different bases that cannot be easily converted to a common base. It outlines a method for handling such cases by using the change of base formula to express all logarithms with a single, preferred base, allowing for their combination and subsequent solution using exponential notation.
🔗 The Change of Base Formula in Logarithmic Equations
The seventh paragraph discusses the application of the change of base formula in scenarios where logarithms have different bases. It provides a detailed example of how to transform each term to a common base, simplify the resulting expressions, and then combine the logarithms to solve the equation using exponential notation.
📉 Dealing with Logarithms of Different Bases
The eighth paragraph addresses the challenge of solving logarithmic equations with different bases that do not simplify easily. It demonstrates the process of using the change of base formula to a base that allows for simplification, and then solving the equation using techniques similar to those used for exponential equations.
🎯 Final Thoughts on Solving Logarithmic Equations
The final paragraph wraps up the discussion on solving logarithmic equations. It reiterates the importance of using exponentials to solve logarithms and hints at the next topic of solving exponential equations, which will build on the techniques learned from working with logarithms.
Mindmap
Keywords
💡Logarithmic Equations
💡Change of Base Formula
💡Exponential Notation
💡Combining Logarithms
💡Zero Product Property
💡Domain Issues
💡Distributing Logarithms
💡Logarithmic Arguments
💡Natural Logarithm
💡Approximate Solutions
💡Logarithmic Simplification
Highlights
The video discusses solving logarithmic equations, focusing on cases where not all terms are logarithms.
It is shown that when all terms are logarithms with the same base, they can be combined and set equal to the inside of one logarithm.
For equations with at least one non-logarithmic term, logarithms are combined into one term on one side of the equation.
Exponential notation is used to rewrite and solve the equation once logarithms are isolated on one side.
The video emphasizes the importance of using the inverse operation, exponentiation, to solve logarithmic equations.
An example demonstrates how to combine logarithms with the same base and a non-zero constant into a single logarithmic term.
The process of changing the base of logarithms using the change of base formula is explained, which is crucial when bases are not the same.
The video illustrates how to handle logarithmic equations with multiple bases by converting them into a common base for easier solving.
A detailed example shows the simplification process of logarithms with different bases by converting them to a base of 2.
The use of substitution methods, such as setting u = log base 2 of x, is introduced to solve more complex logarithmic equations.
The video covers the concept of domain issues in logarithms, emphasizing that the argument of a logarithm cannot be zero or negative.
It is shown how to simplify logarithmic expressions by factoring and combining like terms before applying algebraic techniques.
The transcript includes an example of solving a logarithmic equation with a base of e, using multiplication and division of logarithms.
The video explains how to deal with logarithms of different bases that cannot be simplified to a common base, using the natural logarithm (ln).
An advanced example demonstrates the use of the change of base formula in a more complex context, involving multiple steps and algebraic manipulation.
The transcript concludes with a reminder that logarithmic equations often require multiple steps and a good understanding of logarithmic properties and algebra.
The video emphasizes the utility of change of base and substitution methods in solving complex logarithmic equations.
Transcripts
Browse More Related Video

Solving Logarithms with Common Bases (Precalculus - College Algebra 62)

Solving Exponential Equations with Common Bases (Precalculus - College Algebra 54)

Introduction to Solving Logarithms and Exponentials (Precalculus - College Algebra 57)
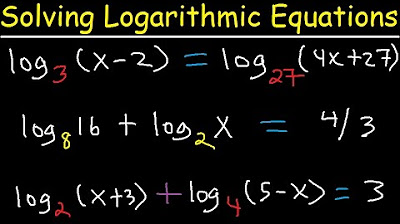
Solving Logarithmic Equations With Different Bases - Algebra 2 & Precalculus
Ch. 4.3 Logarithmic Functions

Business Calculus -- Math 1329 -- Section 4.2 -- Logarithmic Functions
5.0 / 5 (0 votes)
Thanks for rating: