Electrical Formulas - Basic Electricity For Beginners
TLDRThis video script offers an insightful overview of essential electrical formulas used in DC circuits. It begins with the fundamental Ohm's Law (V=IR), explaining how voltage, current, and resistance relate. The script then delves into power calculations, detailing formulas for power dissipated by a resistor and its equivalences. It also covers the concept of electrical work and energy transfer, introducing the relationship between power, charge, and voltage. Moving on to circuit analysis, the script explains series and parallel circuits, including current and voltage behavior, total resistance calculations, and the application of Kirchhoff's laws. The video aims to equip viewers with the necessary formulas and concepts for solving DC circuit problems, making it an invaluable resource for anyone studying or working with electricity.
Takeaways
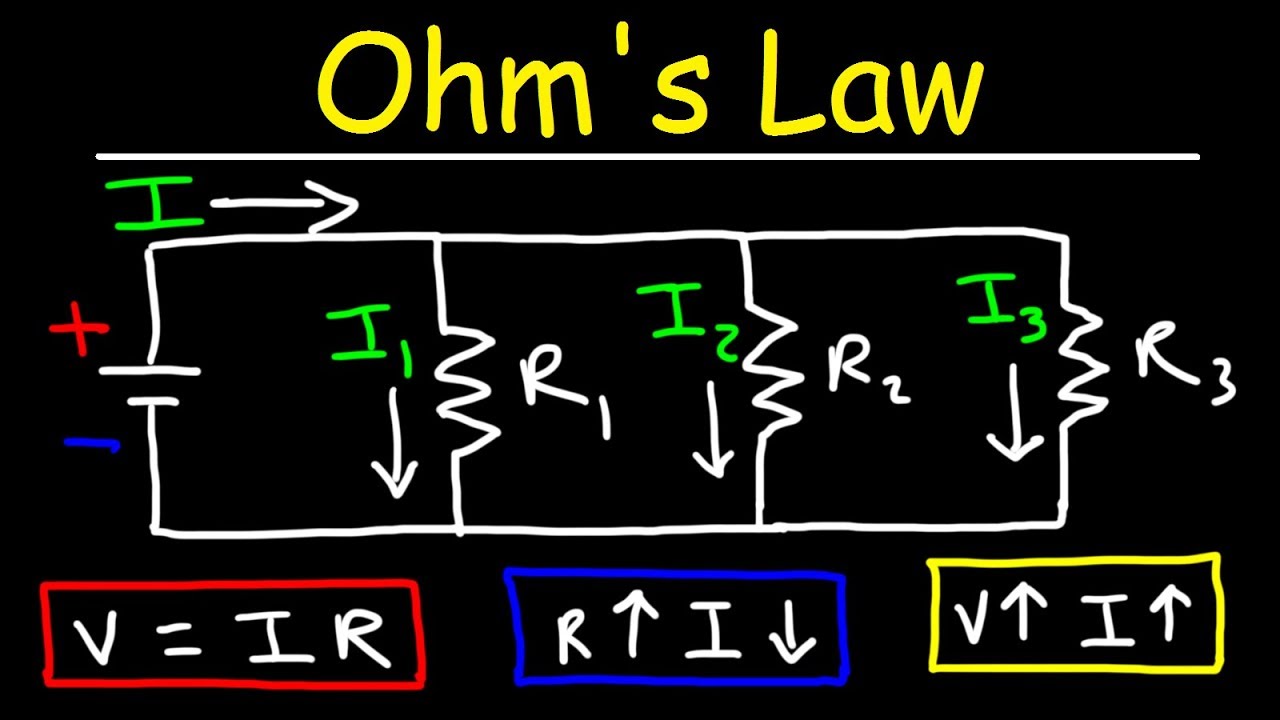
- 🔋 Ohm's Law is fundamental in DC circuits, relating voltage (V), current (I), and resistance (R) as V = IR.
- 💡 Power dissipated by a resistor can be calculated using the formulas P = V*I, P = I^2*R, or P = V^2/R, where P is in watts.
- ⏱️ Electrical work or energy (in joules) is calculated by multiplying power (in watts) by time (in seconds).
- 🔌 The unit for power is the watt, with 1 horsepower equaling 746 watts, and 1 watt being equivalent to 1 joule per second.
- ⚡ The rate of electrical energy transfer is represented by power, which is energy divided by time.
- 🔌 Electrical energy can also be calculated by multiplying the electric charge (in coulombs) by the voltage.
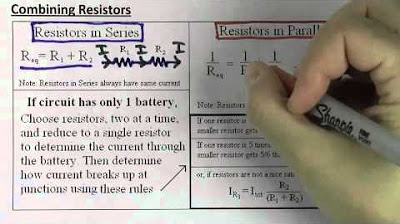
- 🔎 In a series circuit, the current flowing through all components is the same, and the total resistance is the sum of individual resistances.
- 🔄 According to Kirchhoff's Voltage Law, the sum of voltages in a loop equals zero, meaning the battery voltage is the sum of voltage drops across each resistor in series.
- 🔗 In a parallel circuit, the voltage across each resistor is the same as the battery voltage, and the total current is the sum of the currents through each resistor.
- 🔄 The total resistance in a parallel circuit is found by taking the reciprocal of the sum of the reciprocals of each individual resistance.
- 🔌 Kirchhoff's Current Law states that the total current entering a junction equals the total current leaving the junction, which can be used to solve for unknown currents in complex circuits.
Q & A
What is the fundamental relationship between voltage, current, and resistance in a DC circuit?
-The fundamental relationship is described by Ohm's Law, which states that the voltage (V) across a resistor is equal to the current (I) flowing through it multiplied by the resistance (R), or V = IR.
How can you calculate the power dissipated by a resistor in a circuit?
-The power dissipated by a resistor can be calculated using the formula P = V*I, where P is the power in watts, V is the voltage across the resistor in volts, and I is the current flowing through the resistor in amps. Alternatively, P can also be calculated as I^2 * R or V^2 / R.
What is the unit of electrical power and how is it related to energy and time?
-The unit of electrical power is the watt. Power is the rate at which electrical energy is transferred, which is energy divided by time. Therefore, power tells you how fast energy is being transferred to a device, measured in watts (joules per second).
How is electrical energy calculated in a circuit, and what is the relationship between charge, current, and voltage?
-Electrical energy can be calculated using the formula E = Q*V, where E is the energy in joules, Q is the charge in coulombs, and V is the voltage in volts. Since charge (Q) is equal to current (I) multiplied by time (t), the formula can also be expressed as E = I*V*t.
What is the significance of the term 'electric current' and how is it measured?
-Electric current is the rate at which electric charge flows through a conductor. It is measured in amperes (amps), with one ampere being one coulomb of charge flowing per second.
In a series circuit, why is the current the same through all components?
-In a series circuit, there is only one path for the current to flow, so the current (I) is the same through all components because it cannot split or diverge.
How does the total resistance in a series circuit of multiple resistors compare to the individual resistances?
-In a series circuit, the total resistance is the sum of all the individual resistances. If you have resistors R1, R2, and R3 in series, the total resistance (RT) is RT = R1 + R2 + R3.
What is Kirchhoff's Voltage Law and how does it apply to a series circuit?
-Kirchhoff's Voltage Law states that the sum of all the voltages in a closed loop or circuit is zero. In a series circuit, this means that the voltage supplied by the battery (VB) is equal to the sum of the voltage drops across each resistor (V1 + V2 + V3).
In a parallel circuit, how does the voltage across each resistor compare to the voltage supplied by the battery?
-In a parallel circuit, each resistor is connected across the same battery, so the voltage across each resistor (V1, V2, V3) is equal to the voltage supplied by the battery (VB).
How is the total resistance in a parallel circuit calculated when you have multiple resistors?
-In a parallel circuit, the total or equivalent resistance is found using the reciprocal formula: 1/RT = 1/R1 + 1/R2 + 1/R3. This means that the total resistance is the sum of the reciprocals of each individual resistance.
What is Kirchhoff's Current Law and how does it apply to a parallel circuit?
-Kirchhoff's Current Law states that the total current entering a junction or node in a circuit is equal to the total current leaving that junction. In a parallel circuit, this means that the total current (IT) is the sum of the currents through each resistor (I1 + I2 + I3).
Outlines
🔋 Fundamental Electrical Formulas and Ohm's Law
This paragraph introduces the viewer to essential electrical formulas used in DC circuits. It begins with a simple circuit diagram consisting of a battery and a resistor, explaining conventional current flow from the positive to the negative terminal. The first formula discussed is Ohm's Law (V=IR), which links voltage, current, and resistance. The paragraph then covers power calculations, offering three formulas to determine the power dissipated by a resistor or delivered by a battery: P=VI, P=I²R, and P=V²/R. It also explains the concept of electrical work and energy transfer, relating power to energy over time, and introduces the unit conversions for power, such as watts and joules per second. Finally, it touches on the rate of energy transfer and the equivalence of one horsepower to 746 watts.
🔌 Understanding Series and Parallel Circuits
The second paragraph delves into the specifics of series and parallel circuits. In a series circuit, the current (I) remains constant through each component, such as resistors, while the total resistance is the sum of individual resistances. The voltage across each resistor can be calculated using Ohm's Law (V=IR), and the total battery voltage is the sum of the voltage drops across each resistor, aligning with Kirchhoff's Voltage Law. The paragraph then contrasts this with a parallel circuit, where the same voltage is applied across each resistor, but the current divides among the paths. The total resistance in a parallel circuit is found by taking the reciprocal of the sum of the reciprocals of the individual resistances. Kirchhoff's Current Law is introduced, which states that the total current entering a junction equals the total current leaving it, leading to a formula for calculating branch currents in a parallel circuit.
🔄 Kirchhoff's Laws and Circuit Current Analysis
This paragraph focuses on applying Kirchhoff's Current Law to analyze circuits. It provides an example where the total current entering a junction is equal to the sum of the currents leaving it, with the direction of current flow indicated by positive or negative signs. The example demonstrates how to calculate unknown currents in a circuit using the law. The paragraph reinforces the concept that the sum of currents entering and leaving a junction must be equal, ensuring electrical charge conservation. It concludes with a practical demonstration of how altering the current in one branch affects the current in another, emphasizing the interconnected nature of circuit components.
📚 Summary of Electrical Formulas and Further Learning Resources
The final paragraph summarizes the electrical formulas covered in the video and provides guidance for further learning. It reiterates the importance of understanding these formulas for solving DC circuits and working with electricity. The creator encourages viewers to explore additional example problems through links provided in the description, promising more related content and videos to be posted. This paragraph serves as a conclusion, wrapping up the lesson and directing viewers to supplementary materials for a deeper understanding of the topic.
Mindmap
Keywords
💡Ohm's Law
💡Power
💡Electrical Work
💡Electrical Energy
💡Series Circuit
💡Parallel Circuit
💡Kirchhoff's Voltage Law (KVL)
💡Kirchhoff's Current Law (KCL)
💡Voltage Drop
💡Electric Charge
Highlights
Introduction to common electrical formulas for DC circuits.
Explanation of Ohm's Law (V=IR) relating voltage, current, and resistance.
Power dissipated by a resistor calculated using V*I, I^2*R, or V^2/R.
Electrical work done is power multiplied by time, equating to energy transfer.
Units of voltage, current, resistance, and power explained.
Power as the rate of electrical energy transfer, measured in watts.
Conversion of electrical energy to heat by resistors.
Calculation of electrical energy using charge times voltage.
Electric charge defined as the rate of charge flow per unit time.
Explanation of series circuits with resistors and current flow.
Total resistance in series circuits is the sum of individual resistances.
Voltage across components in series circuits and Kirchhoff's Voltage Law.
Introduction to parallel circuits and their voltage characteristics.
Calculation of equivalent resistance in parallel circuits.
Total current in parallel circuits as the sum of branch currents.
Kirchhoff's Current Law and its application to circuit analysis.
Practical example of calculating currents using Kirchhoff's laws.
Conclusion summarizing the importance of understanding electrical formulas.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: