Terminology for the basic operations and writing algebraic expressions from words
TLDRIn this informative lesson from math mth.com, Maria introduces essential mathematical terminology related to the four basic operations: addition, subtraction, multiplication, and division. She explains the terms 'sum' and 'addends' for addition, 'minuend' and 'subtrahend' for subtraction, 'factors' and 'product' for multiplication, and 'dividend', 'divisor', and 'quotient' for division. Maria also provides strategies for solving equations with missing values, using examples to illustrate how to find the missing dividend by multiplying factors and adding sums. The lesson is designed to clarify mathematical concepts and enhance problem-solving skills.
Takeaways
- 📚 In addition, the answer is called the sum, and the numbers being added are called addends.
- 🔢 In subtraction, the number from which another is subtracted is the minuend, and the number being subtracted is the subtrahend, with the result known as the difference.
- 🤔 The expression representing subtraction is also referred to as the difference, such as in the example of 14 - x being the difference of 14 and x.
- 📈 In multiplication, the numbers being multiplied are called factors, and the result is called the product.
- 👉 The multiplication expression is written as 'times' or a dot to avoid confusion with the letter 'x'.
- 🔄 To find a missing dividend in division, use multiplication strategies based on known factors and quotients.
- 📊 The number being divided in division is the dividend, the number that divides it is the divisor, and the result is the quotient.
- 🌟 Division expressions can be represented using a fraction line instead of the division symbol for clarity.
- ✍️ When writing mathematical expressions, ensure clarity by using appropriate symbols and notations to avoid confusion.
- 🧩 For complex expressions, break down the components into sums, differences, products, and quotients as needed.
- 📝 Practice solving for missing values in mathematical expressions by applying the correct operations and understanding the terminology.
Q & A
What is the term used to describe the result of an addition operation?
-The result of an addition operation is called the sum.
What do you call the individual numbers being added in an addition operation?
-The individual numbers being added in an addition operation are called addends.
In subtraction, what is the term for the number from which another number is subtracted?
-In subtraction, the number from which another number is subtracted is called the minuend.
What is the term for the number being subtracted in a subtraction operation?
-The number being subtracted in a subtraction operation is called the subtrahend.
What do you call the result of a subtraction operation?
-The result of a subtraction operation is called the difference.
In multiplication, what are the numbers being multiplied called?
-In multiplication, the numbers being multiplied are called factors.
What is the term used to describe the result of a multiplication operation?
-The result of a multiplication operation is called the product.
In division, what is the term for the number being divided?
-In division, the number being divided is called the dividend.
What is the term for the number that divides the dividend in a division operation?
-The number that divides the dividend in a division operation is called the divisor.
What do you call the result of a division operation?
-The result of a division operation is called the quotient.
How can you find the missing dividend in a division equation?
-To find the missing dividend in a division equation, you can multiply the divisor with the quotient.
What is the expression for the product of five and Z subtracted from 30?
-The expression for the product of five and Z subtracted from 30 is 30 - (5 * Z).
How do you represent the quotient of the sum of 2 and 8 divided by the sum of 1 and 3 using a fraction line?
-The quotient of the sum of 2 and 8 divided by the sum of 1 and 3, represented using a fraction line, is (2 + 8) / (1 + 3) or (10 / 4).
Write an expression for the difference of five and Y divided by the sum of 2 and 4.
-The expression for the difference of five and Y divided by the sum of 2 and 4 is (5 - Y) / (2 + 4).
How can you express the addition of 3S to the quotient of S and 4?
-The addition of 3S to the quotient of S and 4 can be expressed as 3S + (S / 4).
Outlines
📚 Introduction to Arithmetic Operations Terminology
The video introduces Maria from math mth.com, who explains the terminology associated with the four basic arithmetic operations. In addition, the sum, addends, and the process of finding the sum are discussed, using examples like the sum of X and the numbers 5 and 2. Subtraction is covered next, defining the minuend, subtend, and difference, with an example of 14 - X being the difference of 14 and X. The concept of difference is further illustrated with the subtraction of 15 from 7. Multiplication terms such as factors, product, and the multiplication expression are introduced, with examples of multiplying three, four, five numbers, and finding the product. Division is the final operation explained, defining the dividend, divisor, quotient, and the quotient expression, with strategies for finding missing dividends in division equations provided through examples.
🧠 Problem Solving with Arithmetic Operations
Maria continues the lesson by delving into problem-solving strategies involving arithmetic operations. The paragraph focuses on finding missing dividends in division equations, using a step-by-step approach with examples. The process of multiplying factors to find the missing dividend is demonstrated, as well as the use of addition and subtraction to simplify expressions. The explanation includes writing expressions for division and multiplication without using the standard symbols to avoid confusion. The paragraph concludes with a comprehensive example that combines addition, multiplication, and division to solve for a missing value, emphasizing the flexibility in the order of operations.
Mindmap
Keywords
💡terminology
💡addition
💡subtraction
💡multiplication
💡division
💡sum
💡difference
💡product
💡dividend
💡quotient
Highlights
Maria introduces the lesson focusing on mathematical terminology for the four operations.
In addition, the term 'sum' refers to both the operation and the answer.
Addends are the individual numbers involved in an addition operation.
In subtraction, the number being subtracted from is called the minuend.
The number being subtracted is known as the subtrahend.
The result of a subtraction operation is termed the difference.
In multiplication, the numbers being multiplied are called factors.
The outcome of multiplication is named the product.
Division involves a dividend, which is the number being divided.
The divisor is the number by which the dividend is divided.
The result of division is called the quotient.
Maria provides a strategy for finding the missing dividend in a division equation.
An example is given where the missing dividend is found by multiplying the divisor with the quotient.
Maria constructs an expression for the product of five and Z, subtracted from 30.
The quotient of the sum of 2 and 8 divided by the sum of 1 and 3 is explained.
An expression is written for 36 divided by the sum of 2, 3, and X.
The difference of five and Y, divided by the sum of 2 and 4, is presented as an expression.
The final expression involves the quantity 3S added to the quotient of S and four.
Transcripts
Browse More Related Video
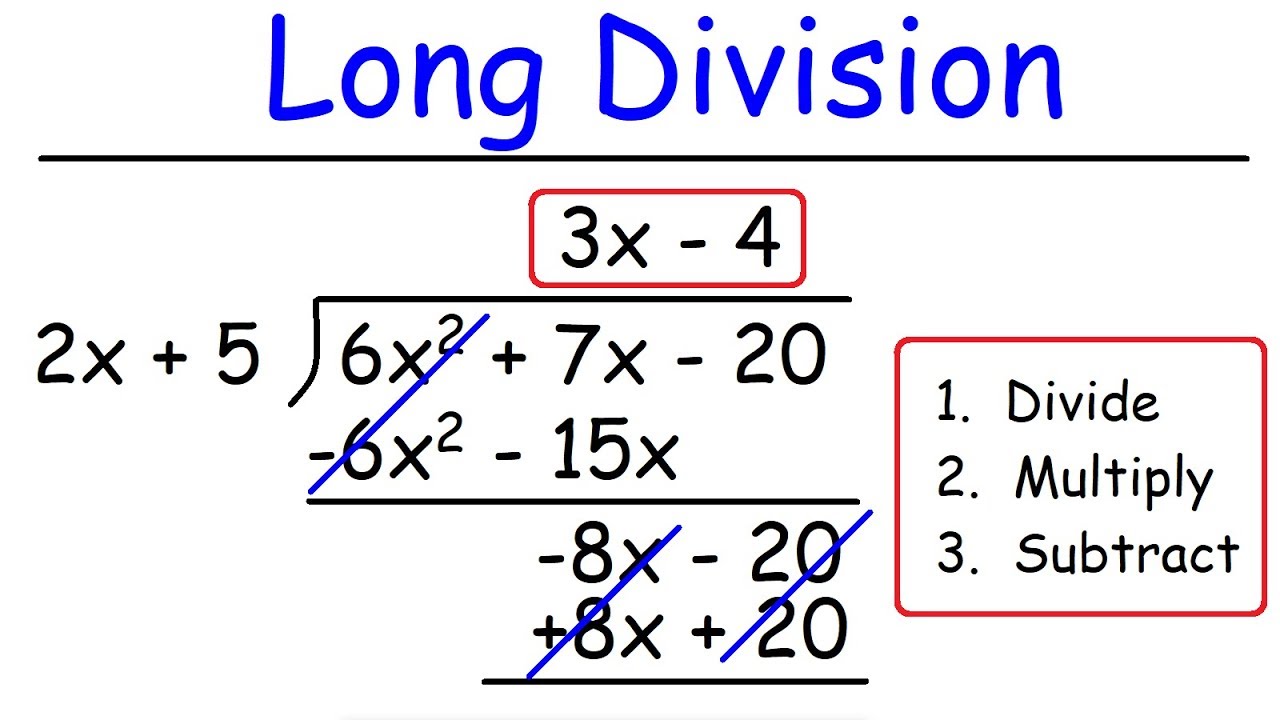
Polynomials - Long Division

How to divide a trinomial into a polynomial using long division

Maths Symbols & Equations - English Vocabulary | Maths Vocabulary | Math or Maths | Basic Math
MATH & GEOMETRY Vocabulary and Terminology in English

Multiplication and Division of Small Numbers
Free English Class! Topic: Math! ➗➕➖ (Lesson Only)
5.0 / 5 (0 votes)
Thanks for rating: