Video 69 - Christoffel Symbol for Surfaces - Part 2

Tensor Calculus

5 Jun 202219:52

EducationalLearning

32 Likes 10 Comments

TLDRIn this video, the presenter delves into the intricacies of the Christoffel symbol in the context of surfaces and curved manifolds. They explore how the symbol's definition varies with covariant and contravariant bases and the necessity of additional terms for surface manifolds to account for vectors pointing in any direction. The video also demonstrates deriving the proper expression for the Christoffel symbol and shows how to evaluate these symbols for surface manifolds using the ambient coordinates and shift tensor. The special case of affine coordinate systems, where Christoffel symbols are zero, is highlighted, offering a direct method for converting ambient to surface space symbols.

Takeaways

📚 The video continues the analysis of the Christoffel symbol in the context of surfaces and curved manifolds, building upon previous discussions.
🔍 The Christoffel symbol is introduced with different index positions, highlighting the distinction between covariant and contravariant bases.
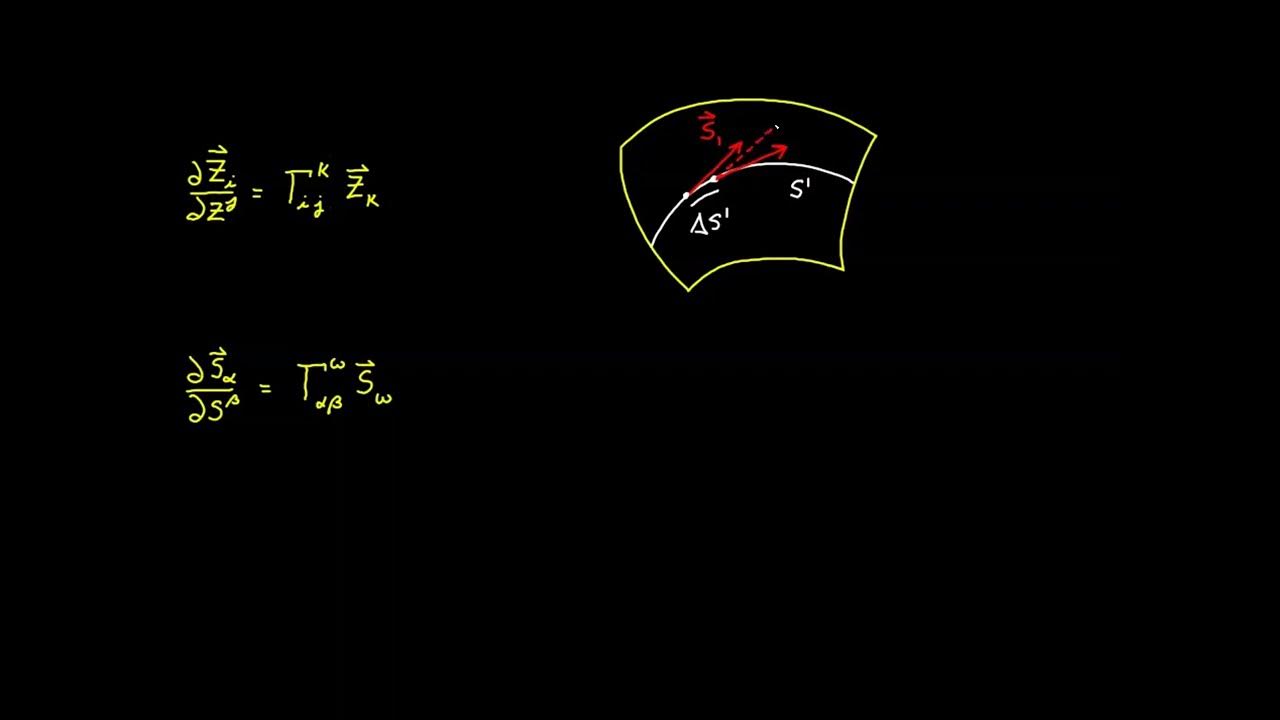
📉 The script explains that for surface manifolds, an additional term is necessary to account for vectors that may point in any direction, including a normal projection.
🔄 Through substitution and application of the product rule, the video derives an expression for the partial derivative of a covariant basis vector in terms of the Christoffel symbol and the metric tensor.
📝 The video demonstrates the process of deriving the proper expression for the Christoffel symbol by manipulating and simplifying the given mathematical expressions.
🧩 The script shows that the Christoffel symbol can be used for both covariant and contravariant basis vectors with adjustments to the sign and dummy indices.
⚖️ The process involves isolating terms and using the properties of the metric tensor and the curvature tensor to simplify the expressions.
🔑 The final derived expression for the Christoffel symbol includes both the surface projection using the Christoffel symbol and the normal projection using the curvature tensor.
🌐 The video also discusses how to evaluate Christoffel symbols for surface manifolds directly from the ambient space using the shift tensor.
🔍 The script concludes with a method to convert Christoffel symbols from an ambient coordinate system to those on a surface manifold using known values and the shift tensor.
📚 The video emphasizes the consistency of using the Christoffel symbol and curvature tensor for both surface and normal projections, with adjustments for index positions.

Q & A

What is the main focus of the video 69 on tensor calculus?
-The main focus of the video is to continue the analysis of the Christoffel symbol in relation to surfaces and curved manifolds, and to explore how it can be used to represent expressions involving covariant and contravariant basis vectors.
What is the significance of the difference between covariant and contravariant basis vectors in the context of the Christoffel symbol?
-The difference between covariant and contravariant basis vectors is significant because it affects the sign of the Christoffel symbol and the direction of the indices, which in turn influences how the symbol is used in expressions for surface manifolds.
Why is the expression involving the Christoffel symbol not sufficient for general surface manifolds?
-The expression is not sufficient for general surface manifolds because it may result in a vector that points in any direction, necessitating both a surface projection and a normal projection, which requires an additional term in the expression.
What is the role of the curvature tensor in the context of the Christoffel symbol and surface manifolds?
-The curvature tensor is used in conjunction with the normal basis vector to represent the normal projection of a vector in surface manifolds, which is necessary when dealing with vectors that may point in any direction.
How does the video script derive the proper expression for the Christoffel symbol involving the contravariant basis vector?
-The script derives the proper expression by starting with the partial derivative of the covariant basis vector, making substitutions using the covariant metric tensor, and then simplifying the expression by using the product rule and the properties of the Christoffel symbol and the curvature tensor.
What is the significance of raising and lowering indices in the expressions involving the Christoffel symbol?
-Raising and lowering indices is significant because it changes the position of the indices, which affects the direction of the vectors involved and the resulting expressions, allowing for the correct representation of vectors in different directions.
How does the script show the consistency between the expressions for covariant and contravariant basis vectors?
-The script demonstrates consistency by showing that both cases involve the use of the Christoffel symbol with the surface basis vectors for the surface projection, and the curvature tensor with the normal basis vector for the normal projection, with the only difference being the position of one of the indices.
What is the purpose of contracting both sides of the expression with the contravariant metric tensor?
-Contracting both sides with the contravariant metric tensor is done to isolate specific terms and simplify the expression, ultimately leading to a clearer representation of the partial derivative of the basis vector.
How can the Christoffel symbols for surface manifolds be evaluated directly from the ambient coordinates?
-The Christoffel symbols for surface manifolds can be evaluated directly from the ambient coordinates by using the shift tensor in conjunction with the known Christoffel symbols in the ambient space, as described in the script.
What is the special case mentioned in the script where the second term in the expression for the Christoffel symbol drops out?
-The special case is when the ambient coordinate system is an affine coordinate system, in which all the Christoffel symbols are zero, and only the first term of the expression remains.

Outlines

00:00

📚 Introduction to Christoffel Symbols in Surface Manifolds

This paragraph delves into the analysis of Christoffel symbols in relation to surfaces and curved manifolds, building upon previous discussions in videos 28 and 32. The speaker explains the use of Christoffel symbols in different expressions, highlighting the distinction between covariant and contravariant bases. The paragraph emphasizes the necessity of additional terms for surface manifolds to account for vectors that may point in any direction, including both surface projection and normal projection. The focus then shifts to deriving the correct expression for these cases, starting with a substitution involving the covariant metric tensor and the contravariant basis vector, and applying the product rule for differentiation.

05:01

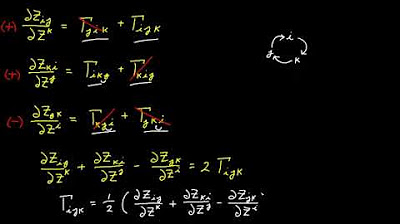
🔍 Deriving Expressions for Surface and Normal Projections

The speaker continues the exploration by deriving expressions for surface and normal projections in curved manifolds. They introduce a substitution to express the partial derivative of the covariant basis vector in terms of the Christoffel symbol and the curvature tensor. By manipulating the indices and using the properties of the metric tensor, the paragraph simplifies the expression to isolate the desired term. The process involves canceling out terms and contracting both sides of the equation with the contravariant metric tensor, leading to a final expression that includes the partial derivative of the basis vector, the Christoffel symbol, and the curvature tensor.

10:02

📐 Consistency in Surface and Normal Projection Expressions

This section emphasizes the consistency of the derived results for both surface and normal projections. The speaker notes that in both cases, the Christoffel symbol is used in conjunction with the surface basis vectors for the surface projection, and the curvature tensor with the normal basis vector for the normal projection. The difference arises from the position of the index, which dictates whether the projection is surface or normal. The paragraph also discusses the general applicability of the Christoffel symbol and curvature tensor, with the only variation being the index position, and concludes with a method to evaluate Christoffel symbols for surface manifolds directly from the ambient coordinates using the shift tensor.

15:04

🔗 Converting Ambient Space Christoffel Symbols to Surface Manifolds

The final paragraph discusses a method to convert Christoffel symbols from the ambient space to the surface manifolds using the shift tensor. The speaker provides a detailed derivation, starting with substitutions for the basis vectors in the surface with their equivalent values using the shift tensor. The process involves applying the product rule and the chain rule for differentiation, leading to an expression that relates the Christoffel symbols in the ambient space to those on the surface. The paragraph concludes by noting that if the ambient coordinate system is affine, the Christoffel symbols are zero, simplifying the conversion process. This method offers a direct way to derive Christoffel symbols for surface manifolds from known values in the ambient coordinate system.

Mindmap

Keywords

💡Christoffel Symbol

The Christoffel symbol is a function that expresses the covariant derivative in differential geometry. It is used to describe the curvature of a surface or a manifold. In the video, the Christoffel symbol is central to the analysis of how vectors behave on curved surfaces, and it is used to derive expressions for the covariant and contravariant basis vectors and their derivatives.

💡Covariant Basis

A covariant basis is a set of vectors that are tangent to a surface at a given point and changes with the surface's curvature. In the script, the covariant basis is used to express the partial derivatives and to derive the Christoffel symbols, which are essential for understanding the behavior of vectors on curved manifolds.

💡Contravariant Basis

The contravariant basis is dual to the covariant basis and is used to raise or lower indices in tensor notation. In the video, the contravariant basis is contrasted with the covariant basis, highlighting the difference in how they are used in expressions involving the Christoffel symbol.

💡Surface Manifolds

A surface manifold is a two-dimensional surface that can be embedded in a higher-dimensional space. The video discusses the application of the Christoffel symbol to surface manifolds, emphasizing the need to account for both surface and normal projections when dealing with vectors that may point in any direction.

💡Curvature Tensor

The curvature tensor is a mathematical object that describes the curvature of a surface or a space. In the context of the video, the curvature tensor is used in conjunction with the normal basis vector to account for the normal projection of vectors on a surface manifold.

💡Partial Derivative

A partial derivative is the derivative of a function with respect to one variable, while the other variables are held constant. In the video, partial derivatives are used extensively in the derivation of expressions involving the Christoffel symbol and the basis vectors.

💡Metric Tensor

The metric tensor is a tensor that provides a way to define distances and angles in a curved space. In the script, the metric tensor is used in the derivation of the Christoffel symbol and is essential for understanding the geometry of the surface manifold.

💡Shift Tensor

The shift tensor is used to relate the basis vectors of a surface manifold to those of the ambient space. In the video, the shift tensor is used to derive the Christoffel symbols for the surface manifold from the known values in the ambient space.

💡Affine Coordinate System

An affine coordinate system is a coordinate system in which the Christoffel symbols vanish, simplifying the analysis of the space. The video mentions that if the ambient space has an affine coordinate system, the derivation of the Christoffel symbols for the surface manifold is simplified.

💡Tensor

In mathematics, a tensor is a generalization of scalars, vectors, and higher-dimensional arrays. In the context of the video, tensors are used to describe the geometric properties of surfaces and manifolds, particularly through the Christoffel symbol and the curvature tensor.

💡Surface Projection

Surface projection refers to the component of a vector that lies within the tangent plane of a surface. The video discusses the need for surface projection when dealing with vectors on a surface manifold, as part of the analysis involving the Christoffel symbol.

💡Normal Projection

Normal projection is the component of a vector that is perpendicular to the surface. The video emphasizes the importance of considering both the surface and normal projections when working with vectors on a surface manifold, particularly when using the Christoffel symbol and the curvature tensor.

Highlights

Introduction of the Christoffel symbol in the context of surfaces and curved manifolds, building on previous discussions.

Differentiation between covariant and contravariant basis in the expression of the Christoffel symbol.

The necessity to modify the expression for surface manifolds to include a normal projection term.

Derivation of the proper expression for the Christoffel symbol with a substitution involving the covariant metric tensor.

Use of the product rule to evaluate the partial derivative of the covariant basis vector.

Identification of the partial derivative of the covariant metric tensor as equivalent to the Christoffel symbol.

Isolation of terms to reveal the relationship between the Christoffel symbol and the curvature tensor.

Contraction of both sides of the expression with the contravariant metric tensor to simplify the equation.

Final expression for the partial derivative of the basis vector incorporating the Christoffel symbol and curvature tensor.

Consistency in using the Christoffel symbol for both surface and normal projections in manifolds.

Demonstration of deriving Christoffel symbols for surface manifolds from ambient coordinates using the shift tensor.

Application of the chain rule to express the partial derivative of the basis vector in terms of the shift tensor.

Simplification of the Christoffel symbol expression when the ambient coordinate system is affine.

Direct conversion from ambient space Christoffel symbols to surface space using the shift tensor.

Review of the derived expressions and their application to sample surfaces in upcoming videos.

Transcripts

Browse More Related Video

Video 70 - Surface Christoffel Examples

Video 68 - Christoffel Symbol for Surfaces - Part 1

Video 30 - Christoffel Symbol Evaluation

Video 88 - Embedded Curves - Part 1

Video 62 - Embedded Curve

Tensor Calculus 8d: The Christoffel Symbol on the Sphere of Radius R