Relating Metric Tensor to Gravity | Tensor Calculus Ep. 16

Andrew Dotson

19 Mar 202119:05

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the realm of tensor calculus for physics, focusing on the Newtonian limit and its relation to the metric tensor and gravitational potential. It explores Poisson's equation and the generalization of the Laplacian of the gravitational potential, aiming to connect classical mechanics with Einstein's field equations. The script simplifies complex concepts by discussing the geodesic equation, the static weak field metric, and the connection between the metric tensor's zero-zero component and the gravitational potential, providing a foundation for understanding the Einstein field equations.

Takeaways

📚 The video discusses the relationship between the metric tensor and the gravitational potential in the Newtonian limit as part of the journey towards the Einstein field equations.
🔍 The script aims to simplify the work needed to derive the Einstein field equations by exploring the reduction of tensor calculus to classical physics concepts in the Newtonian limit.
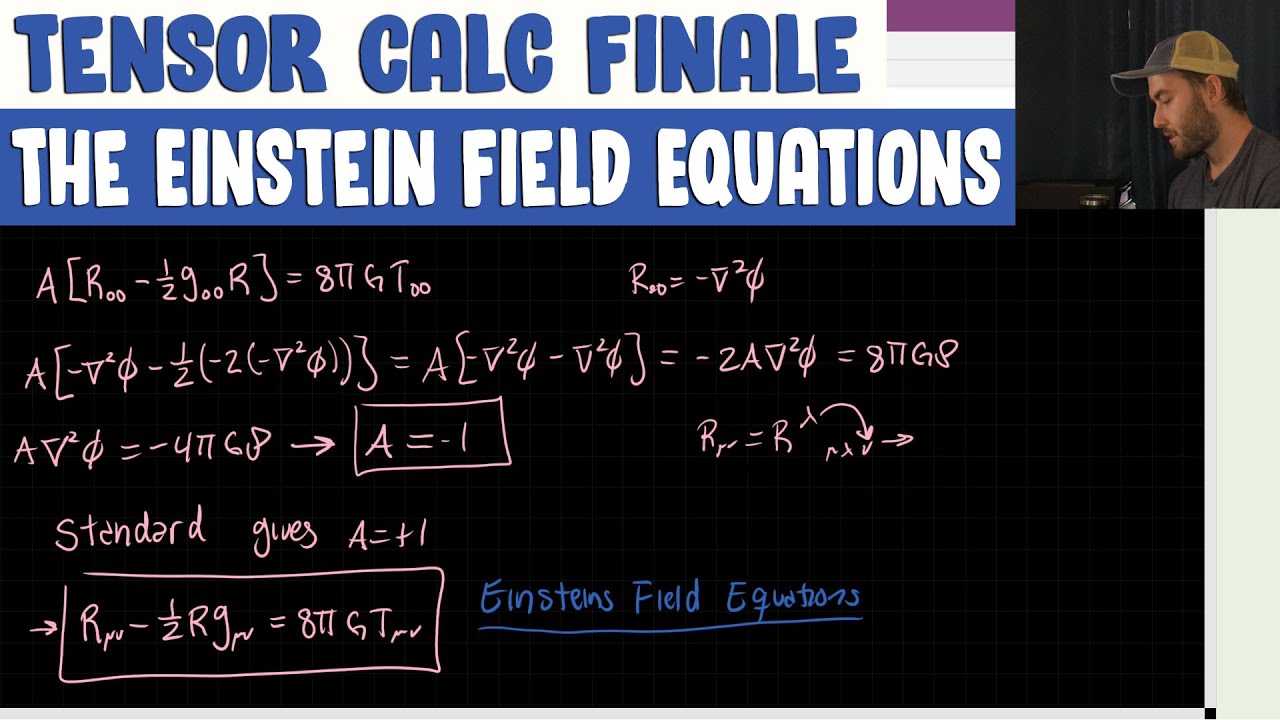
🌌 Poisson's equation, which equates the Laplacian of the gravitational potential to 4π times the gravitational constant times the mass density, is identified as the classical equation that the Einstein field equations should reduce to.
🚀 The energy-momentum tensor's zero-zero component is related to the energy density, which in the classical limit is replaced by the mass density, simplifying the right-hand side of the Einstein field equations.
🔄 The generalization of the potential and Laplacian in the context of tensor calculus involves replacing derivatives with covariant derivatives, a key step in the transition to Einstein's theory of general relativity.
🧭 The geodesic equation, derived in a previous episode, is the generalized version of the free fall equation and is central to understanding the motion of particles in a gravitational field.
🌟 The script introduces the concept of a static weak field metric, which assumes a metric that does not evolve over time and is nearly Minkowskian with a small perturbation.
📉 In the Newtonian limit, the time derivatives of the metric are zero, simplifying the calculation of the Christoffel symbols and their contribution to the geodesic equation.
🔗 The video establishes a connection between the perturbation of the metric tensor (h00) and the gravitational potential (phi), showing that they are proportional in the Newtonian limit.
📐 The Laplacian of the metric tensor's zero-zero component in the Newtonian limit is related to the energy-momentum tensor's zero-zero component, hinting at the structure of the Einstein field equations.
🔮 The script concludes by suggesting that the left-hand side of the Einstein field equations will involve second derivatives of the metric tensor, not covariant derivatives, due to the choice of connection coefficients.

Q & A

What is the main topic of the video?
-The main topic of the video is the exploration of tensor calculus in the context of physics, specifically relating the metric tensor to the gravitational potential in the Newtonian limit and how it could lead to the Einstein field equations.
What is Poisson's equation as mentioned in the script?
-Poisson's equation, as mentioned in the script, is the Laplacian of the gravitational potential being equal to 4 pi times the gravitational constant (big G) times the mass density (rho), which is what the Einstein field equation should reduce to in the Newtonian limit.
What is the significance of the energy-momentum tensor in the context of the video?
-The energy-momentum tensor is significant because its zero-zero component is related to the Hamiltonian density, which in a classical limit is related to the energy density. This is a key step in generalizing the right-hand side of the Einstein field equations.
What is the geodesic equation and why is it important in this context?
-The geodesic equation is a generalization of the free-fall equation and it describes the motion of a particle in a curved spacetime. It is important because it helps to relate the classical equations of motion to the Einstein field equations in the Newtonian limit.
What is the role of the Christoffel symbols in the geodesic equation?
-The Christoffel symbols are used in the geodesic equation to account for the curvature of spacetime. They vanish in a locally Minkowski space, simplifying the equation to a form that describes motion with constant velocity.
What does the term 'static weak field metric' refer to in the video?
-The 'static weak field metric' refers to a metric that does not evolve over time (static) and is almost Minkowski spacetime plus a small perturbation (weak field). It is used to simplify calculations in the Newtonian limit.
How does the script relate the metric tensor to the gravitational potential?
-The script relates the metric tensor to the gravitational potential by showing that in the Newtonian limit, the zero-zero component of the metric tensor can be expressed as 1 plus two times the gravitational potential, which is a direct relation between spacetime geometry and gravitational effects.
What is the significance of the four-velocity in the script?
-The four-velocity is significant because it allows for the specification of velocities in natural units where the speed of light is set to one. It simplifies the geodesic equation in the Newtonian limit, where the velocity is much smaller than the speed of light.
How does the script generalize the potential and Laplacian in the context of the Einstein field equations?
-The script suggests that the potential should be replaced with covariant derivatives, and the Laplacian should be related to the second derivatives of the metric tensor. This is part of the process of generalizing the classical equations to the Einstein field equations.
What is the final equation that connects the metric tensor to the energy-momentum tensor in the script?
-The final equation suggested in the script is that the Laplacian of the metric tensor's zero-zero component in the Newtonian limit should be equal to eight pi times the gravitational constant over c squared times the zero-zero-zero-zero component of the energy-momentum tensor, hinting at the form of the Einstein field equations.

Outlines

00:00

📚 Introduction to Relating Metric Tensor and Gravitational Potential

This paragraph introduces the topic of the video, which is to explore the relationship between the metric tensor and the gravitational potential within the framework of tensor calculus for physics. The speaker aims to prepare for the discussion of Einstein's field equations by examining the Newtonian limit of these equations. The Poisson equation is highlighted as the classical equation of motion for gravity, which the Einstein field equations should reduce to in this context. The focus is on generalizing the left-hand side of the equation, involving the Laplacian of the gravitational potential, to its covariant form, while the right-hand side is more straightforward to generalize due to its relation to the energy density.

05:02

🔍 Exploring the Generalization of the Gravitational Potential

The speaker delves into the generalization of the gravitational potential and its relation to the geodesic equation, which is a generalization of the free fall equation. The geodesic equation is discussed in the context of a locally Minkowski space, where the Christoffel symbols vanish, simplifying the equation to a state of constant velocity motion. The four-velocity is introduced, and its components are analyzed in the Newtonian limit, where the velocity is much smaller than the speed of light. The paragraph concludes with the simplification of the geodesic equation under this limit, focusing on the contributions from the zero-zero component of the Christoffel symbols.

10:04

🌌 Newtonian Limit and Static Weak Field Metric

This paragraph discusses the application of the Newtonian limit to the metric tensor, specifically using the static weak field metric. The static weak field metric is defined as a Minkowski metric plus a small perturbation, which is position-dependent but not time-dependent. The focus is on calculating the Christoffel symbols for the zero-zero component, which simplifies due to the static nature of the metric. The speaker then relates the covariant derivative of the perturbation to the regular gradient, taking into account the Minkowski metric's effect on the derivative.

15:05

🔗 Relating Gravitational Potential to the Metric Tensor in the Newtonian Limit

The final paragraph ties together the concepts discussed in the previous sections by relating the gravitational potential to the zero-zero component of the metric tensor in the Newtonian limit. The speaker explains that the zero-zero component of the metric tensor can be related to the gravitational potential, which is a natural connection given the role of the zero-zero component in the energy-momentum tensor. The Laplacian of the metric tensor's zero-zero component is equated to twice the Laplacian of the gravitational potential, leading to a heuristic understanding of how the Einstein field equations might generalize to include the energy-momentum tensor on the right-hand side and second derivatives of the metric on the left-hand side.

Mindmap

Keywords

💡Tensor Calculus

Tensor calculus is a field of mathematics that deals with tensor fields and their manipulations, particularly in the context of differential geometry and general relativity. In the video, tensor calculus is used to explore the Einstein field equations, which describe the fundamental interaction of gravitation as a result of spacetime being curved by mass and energy. The script mentions tensor calculus as the framework for understanding the relationship between the metric tensor and gravitational potential in the Newtonian limit.

💡Einstein Field Equations

The Einstein field equations are a set of ten interrelated differential equations whose solutions, the metric tensors, describe the curvature of spacetime due to the presence of mass and energy. The video aims to motivate these equations by reducing them to simpler forms that can be more easily understood and calculated. The script discusses the eventual goal of finding coefficients for these equations, which will be 'pretty easy' once the foundational concepts are established.

💡Metric Tensor

The metric tensor is a fundamental concept in differential geometry and general relativity, defining the geometry of spacetime in a given coordinate system. It is used to measure distances and angles between points in spacetime. In the script, the metric tensor is related to the gravitational potential in the Newtonian limit, showing how spacetime geometry is affected by gravitational fields.

💡Newtonian Limit

The Newtonian limit refers to the approximation where the effects of general relativity are negligible, and the physics reduces to the classical Newtonian mechanics. This is typically the case for weak gravitational fields and low velocities compared to the speed of light. The video script discusses how to relate the metric tensor to the gravitational potential within this limit, simplifying the complex equations of general relativity to more familiar Newtonian forms.

💡Poisson's Equation

Poisson's equation is a partial differential equation that describes the potential field generated by a given charge or mass distribution. In the context of the video, Poisson's equation is used to relate the Laplacian of the gravitational potential to the mass density, which is a key step in connecting the Newtonian gravity with the Einstein field equations.

💡Laplacian

The Laplacian is a differential operator, often denoted as 'del squared', which is used in the study of potential fields. It is the divergence of the gradient of a function, providing a measure of how much a function deviates from being a harmonic function. In the script, the Laplacian of the gravitational potential is equated to a multiple of the mass density, setting up the foundation for the generalization to the Einstein field equations.

💡Christoffel Symbols

Christoffel symbols, also known as connection coefficients, are used in differential geometry to describe the change in the basis vectors of a coordinate system under parallel transport. In the video, these symbols appear in the geodesic equation, which is a generalization of the free-fall equation in classical mechanics, and they are instrumental in connecting the motion of particles in spacetime to the curvature induced by the metric tensor.

💡Geodesic Equation

The geodesic equation is a fundamental equation in general relativity that describes the motion of a particle in a gravitational field as a straight line in curved spacetime. The script derives this equation and discusses its simplification in the Newtonian limit, showing how it relates to the classical equation of motion for free fall.

💡Static Weak Field Metric

The static weak field metric is a type of metric tensor used in general relativity to describe spacetimes that are almost flat but have small perturbations, typically in the presence of weak gravitational fields. In the script, this metric is used to simplify the calculations in the Newtonian limit, providing a bridge between the flat spacetime of special relativity and the curved spacetime of general relativity.

💡Covariant Derivative

The covariant derivative is a generalization of the regular derivative that is used in curved spaces, such as spacetime in general relativity. It takes into account the curvature of the space and is essential for defining concepts like parallel transport and geodesics. The script mentions that the covariant derivative of the metric tensor is zero, which is a choice made in defining the connection coefficients to ensure that the metric tensor is covariantly constant.

💡Energy-Momentum Tensor

The energy-momentum tensor, often denoted as Tμν, is a quantity in physics that describes the distribution and flow of energy and momentum in spacetime. In the context of the Einstein field equations, it is the source term that determines how matter and energy influence the curvature of spacetime. The script relates the zero-zero component of this tensor to the Hamiltonian density, which is a measure of energy density in the classical limit.

Highlights

Introduction to the upcoming exploration of Einstein Field Equations in the context of tensor calculus for physics.

Explanation of the Poisson's equation and its relation to the gravitational potential in the Newtonian limit.

Generalization of the energy-momentum tensor and its connection to the Hamiltonian density and energy density.

Discussion on the classical limit where mass density replaces the energy-momentum tensor's zero-zero component.

Introduction to the geodesic equation as a generalization of the free fall equation.

Analysis of the four-velocity in the context of the Newtonian limit and its simplification.

Derivation of the Christoffel symbols and their significance in the geodesic equation.

The use of the static weak field metric to simplify calculations in the Newtonian limit.

Calculation of the Christoffel symbols under the static weak field metric assumption.

Relating the covariant derivative to the regular gradient in the context of the metric perturbation.

Substitution of the geodesic equation components into the Newtonian limit to derive the relationship between the metric tensor and gravitational potential.

Identification of the relationship between the second derivative of position with respect to time and acceleration in the Newtonian framework.

Establishment of the connection between the metric tensor's zero-zero component and the gravitational potential in the Newtonian limit.

Discussion on the energy-momentum tensor's zero-zero component and its relation to the Hamiltonian density.

Heuristic approach to generalize the Newtonian limit equations to the Einstein Field Equations.

Anticipation of the next video's exploration into the options for generalizing the left-hand side of the Einstein Field Equations.

Conclusion summarizing the insights gained and the path forward in understanding tensor calculus in physics.

Transcripts

Browse More Related Video

The Einstein Field Equations | Tensor Calc Finale

Einstein Field Equations - for beginners!

General Relativity Lecture 10

General Relativity Lecture 5

Gravitational Potential and Gravitational Potential Energy

General Relativity Lecture 9