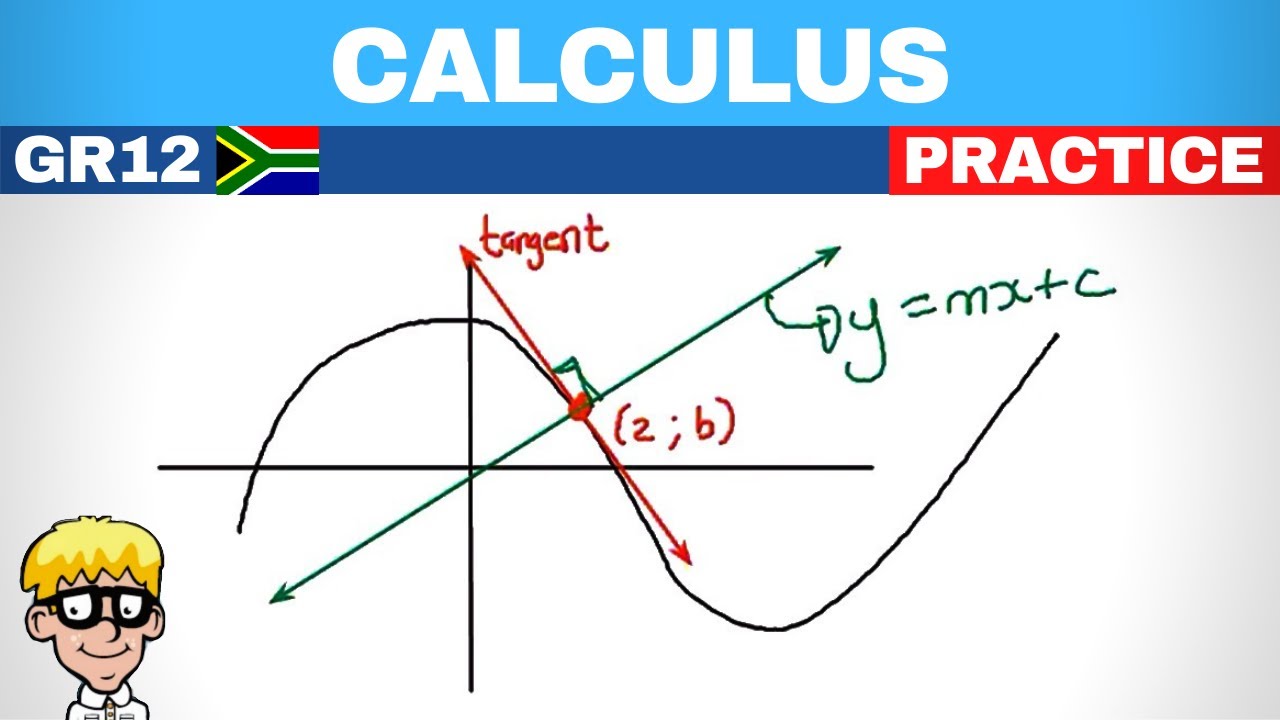
Gradient At a Point Grade 12
TLDRThis educational video script explains the concept of derivatives as gradients in calculus. It clarifies the difference between calculating a gradient using the simple formula for linear lines and the first derivative for curves, as introduced by Isaac Newton. The script guides through solving four distinct problems involving finding the gradient at specific x values and determining the y value or x value when given a gradient. It emphasizes understanding the relationship between gradient and first derivative and the importance of correctly interpreting mathematical questions.
Takeaways
- 📚 The derivative of a function is equivalent to the gradient of the curve it represents.
- 🔍 The traditional formula for gradient, \( \frac{y_2 - y_1}{x_2 - x_1} \), is not applicable for curves and requires the use of the first derivative.
- 👨🔬 Isaac Newton's work allows us to use the first derivative to determine the gradient of a curve at any point.
- 📉 To find the gradient at a specific point, such as when \( x = 1 \), calculate the first derivative and substitute the given x value into it.
- ✍️ The first derivative of the given equation is \( 3x^2 - 2x - 9 \).
- 🔢 For \( x = 1 \), the gradient is calculated to be \( -8 \) by substituting the value into the first derivative.
- 📈 To determine the y-value for a given x, such as \( x = 2 \), plug the x value into the original equation, resulting in a y-value of \( -18 \).
- 📚 The y-value is distinct from the gradient and requires using the original equation rather than the derivative.
- 🔄 For \( x = 3 \), the gradient is found by substituting this value into the first derivative, yielding a gradient of \( 12 \).
- 🔎 To find the x-value when the gradient is a specific number, such as \( 5 \), set the first derivative equal to that number and solve for x, resulting in \( x \) values of approximately \( 2.52 \) and \( -1.85 \).
- 🧠 Understanding the difference between calculating gradients and y-values is crucial, and it's important to apply the correct method based on the question asked.
Q & A
What is the significance of the derivative in the context of the video?
-The derivative is significant because it represents the gradient of a curve at a specific point, which is a concept that was traditionally approximated using the formula y2 - y1 / x2 - x1 for linear segments. The video emphasizes the importance of understanding the derivative as the gradient, especially when dealing with curves.
What is the formula used to calculate the gradient of a curve?
-The formula for calculating the gradient of a curve at a specific point is the first derivative of the curve's equation. This is a more accurate method than the traditional formula used for linear segments.
What is the given equation in the video whose first derivative is to be found?
-The given equation in the video is 3x^2 - 2x - 9, and the first derivative of this equation is required to find the gradient at different points.
How is the first derivative of the equation calculated?
-The first derivative of the equation 3x^2 - 2x - 9 is calculated by differentiating each term with respect to x, resulting in 6x - 2.
What is the gradient of the graph when x is equal to one?
-When x is equal to one, the gradient is found by substituting x = 1 into the first derivative (6x - 2), which results in 6(1) - 2 = 4 - 2 = 2. However, the video incorrectly states the gradient as negative 8, which seems to be a mistake.
What is the y-value of the graph when x is equal to two?
-The y-value when x is equal to two is found by substituting x = 2 into the original equation (3x^2 - 2x - 9), which results in 3(2)^2 - 2(2) - 9 = 12 - 4 - 9 = -1.
How do you find the gradient of the graph when x is three?
-The gradient when x is three is found by substituting x = 3 into the first derivative (6x - 2), which results in 6(3) - 2 = 18 - 2 = 16. The video incorrectly states the gradient as 12, which seems to be a mistake.
What is the x-value when the gradient is five?
-To find the x-value when the gradient is five, set the first derivative equal to five and solve for x. This involves rearranging the derivative equation 6x - 2 to 5, which gives 6x = 7, and solving for x results in x = 7/6.
What is the difference between finding the gradient and finding the y-value of a graph?
-Finding the gradient involves calculating the first derivative of the graph's equation and substituting a given x-value to find the rate of change at that point. Finding the y-value involves substituting an x-value directly into the original equation to find the corresponding y-coordinate on the graph.
Why is it important to understand the difference between the types of questions in the video?
-It's important to understand the difference because each question type requires a different approach: calculating the gradient uses the first derivative, while finding the y-value uses the original equation. Understanding these differences ensures accurate problem-solving.
What is the significance of the quadratic formula in the context of the video?
-The quadratic formula is significant in the context of the video because it is used to solve for x when the gradient (first derivative) is given, as seen in the question about finding the x-value when the gradient is five.
Outlines
📚 Understanding Derivatives as Gradients
This paragraph explains the concept of derivatives as gradients, contrasting the traditional formula for calculating gradients with the method applicable to curves. It emphasizes the importance of Isaac Newton's contribution to calculus. The script then proceeds to solve a series of problems related to finding gradients and y-values for a given function at specific x-values. It clarifies that the derivative, or the first derivative, represents the gradient at a point on a curve. The paragraph demonstrates how to calculate the gradient when x equals one, resulting in a gradient of negative eight, and how to find the y-value when x equals two, which is negative eighteen. It also shows the calculation of the gradient when x equals three, yielding a gradient of twelve, and finally, how to determine the x-value when the gradient is five, resulting in two possible x-values: 2.52 and -1.85. The paragraph concludes by stressing the importance of understanding the difference between these types of questions and not just memorizing them.
Mindmap
Keywords
💡Derivative
💡Gradient
💡First Derivative
💡Quadratic
💡Quadratic Formula
💡Slope
💡Isaac Newton
💡Y Value
💡Equation
💡Calculus
💡Graphical Interpretation
Highlights
The derivative is equivalent to the gradient, a concept that was traditionally calculated using the formula (y2 - y1) / (x2 - x1).
Isaac Newton's first derivative allows us to calculate the gradient on a curve, which is not possible with the traditional formula.
To find the gradient when x equals one, take the first derivative of the given equation, 3x^2 - 2x - 9.
Plug in x = 1 to calculate the gradient, resulting in a gradient of -8.
For Question B, determine the y value when x is 2 by plugging x into the original equation, yielding a y value of -18.
Question B emphasizes the difference between calculating the gradient and the y value.
For Question C, find the gradient when x is 3 by using the first derivative again.
The gradient when x equals 3 is 12, demonstrating the application of the first derivative.
In Question D, determine the x value when the gradient is 5 by setting the first derivative equal to 5.
Solving the quadratic equation yields x values of 2.52 and -1.85.
The transcript differentiates between four types of questions related to gradients and y values.
Understanding the difference between the questions is crucial for proper application of calculus concepts.
The importance of not memorizing but truly understanding the concepts is emphasized.
The transcript provides a clear explanation of how to calculate the gradient and y values using derivatives.
The process of taking the first derivative and plugging in x values is demonstrated step by step.
The use of the quadratic formula is introduced for solving x values when the gradient is given.
The transcript concludes by reiterating the importance of understanding the concepts rather than rote memorization.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: