Directional Derivatives (Calculus 3)

Houston Math Prep

8 Mar 202116:09

EducationalLearning

32 Likes 10 Comments

TLDRThis video from Houston Math Prep delves into the concept of the directional derivative in multivariable calculus. It explains how the gradient of a function, which is perpendicular to level curves, indicates the direction of greatest increase. The video demonstrates how to calculate the rate of change of a function at a specific point in a particular direction using the gradient and a unit vector. Through examples, it illustrates the process of finding directional derivatives for functions of x, y, and even x, y, z, highlighting the importance of understanding the gradient and its application in various mathematical contexts.

Takeaways

📚 The video is part of the 'Houston Math Prep' series, focusing on calculus concepts related to functions in 3D space.
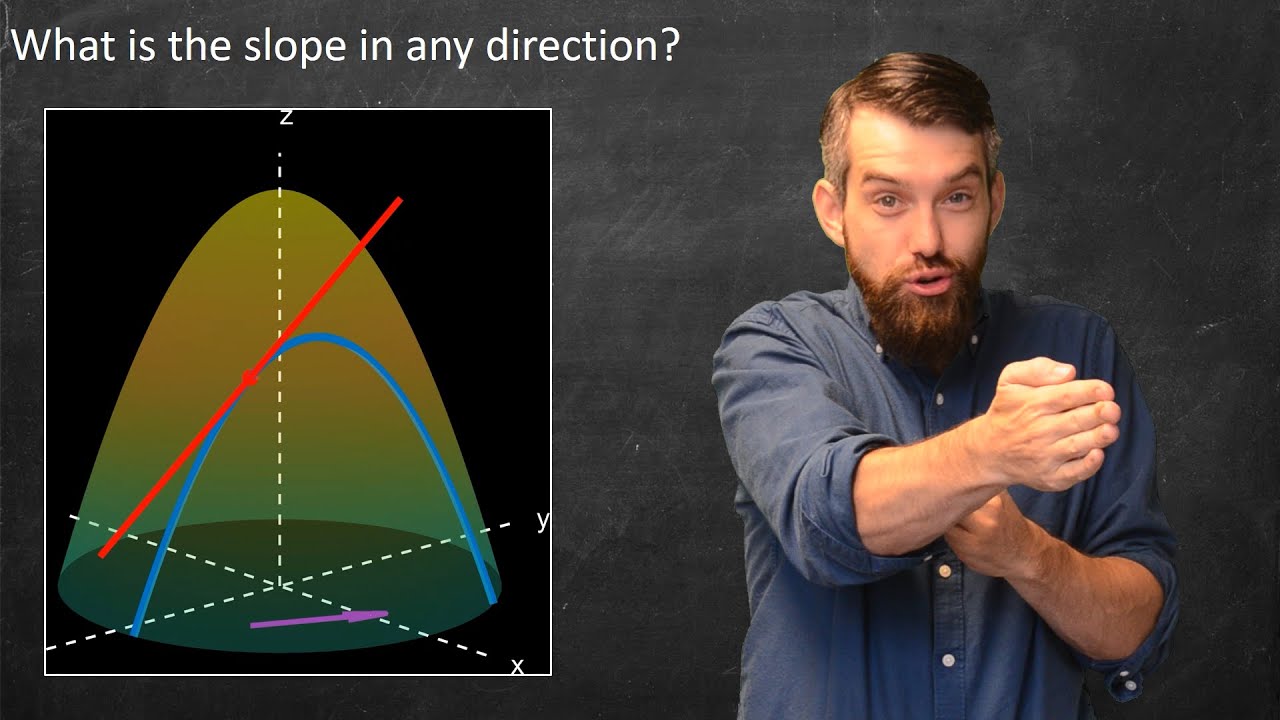
📈 The script introduces the concept of a level curve for a function, which is a surface in 3D space where the value of 'z' is constant.
🧭 It explains the gradient of a function, which is perpendicular to the level curve and indicates the direction of the greatest increase in the function's value.
🚀 The gradient is calculated as the vector of partial derivatives with respect to 'x' and 'y', and its direction is used to find the rate of change of the function.
🌐 The script discusses the concept of a directional derivative, which is the rate of change of a function in a specific direction defined by a vector 'v'.
📝 The notation for the directional derivative is given as \( \mathbf{D}_v f \) evaluated at a point 'p', where 'v' is the direction vector.
🔍 The rate of change in a direction other than the gradient is found using the dot product of the gradient and the unit vector in the direction of 'v'.
📉 The script provides a step-by-step example of calculating the directional derivative for the function \( x^2y^3 \) in the direction of the vector (1, -1) at the point (-2, 1).
📚 Another example is given for the function \( y \cdot e^{x+y} \) in the direction of the vector (3, -2) at the point (0, 2).
📘 The final example involves a function of three variables, \( \sqrt{x \cdot y \cdot z} \), and calculates the directional derivative in the direction of the vector (1, 2, -2) at the point (4, 2, 2).
🔢 The process involves finding the gradient of the function, evaluating it at the given point, normalizing the direction vector to a unit vector, and then performing the dot product to find the directional derivative.

Q & A

What is a level curve in the context of a function of two variables?
-A level curve is a curve along which the function has a constant value. For a function f(x, y), a level curve is defined by f(x, y) = c for some constant c.
How is the gradient of a function related to level curves?
-The gradient of a function is always normal or perpendicular to the level curve at any given point. It points in the direction of the greatest increase of the function's value.
What is a directional derivative?
-A directional derivative is the rate of change of a function at a particular point in the direction of a given vector. It indicates how the function changes as one moves in that specific direction.
How do you calculate the directional derivative of a function?
-The directional derivative is calculated by taking the dot product of the gradient of the function evaluated at a point and a unit vector in the direction of interest. The formula is D_v f(p) = ∇f(p) • v̂, where v̂ is the unit vector in the direction of vector v.
What is the significance of the gradient's magnitude in relation to directional derivatives?
-The magnitude of the gradient represents the maximum rate of change of the function at a given point. When calculating the directional derivative, this magnitude is scaled by the cosine of the angle between the gradient and the direction vector.
How is the unit vector v̂ found from a vector v?
-The unit vector v̂ is found by dividing vector v by its magnitude. If v = (x, y), then v̂ = (x/|v|, y/|v|), where |v| is the magnitude of v, calculated as √(x² + y²).
How can you express the directional derivative without directly calculating the angle between vectors?
-By using the dot product formula, the directional derivative can be expressed as the dot product of the gradient vector and the unit vector in the direction of interest, eliminating the need to directly calculate the angle between them.
What is the gradient of the function f(x, y) = x²y³?
-The gradient of the function f(x, y) = x²y³ is given by ∇f = (2xy³, 3x²y²).
How do you evaluate the gradient at a specific point?
-To evaluate the gradient at a specific point (a, b), substitute x = a and y = b into the gradient function. For example, for f(x, y) = x²y³, at the point (-2, 1), the gradient ∇f is (2(-2)1³, 3(-2)²1²) = (-4, 12).
What is the final value of the directional derivative for the function f(x, y) = x²y³ in the direction of the vector (1, -1) at the point (-2, 1)?
-The final value of the directional derivative is -8√2. This is found by computing the dot product of the gradient (-4, 12) and the unit vector (1/√2, -1/√2), resulting in (-4/√2 - 12/√2) = -16/√2 = -8√2.
How do you find the gradient for a function of three variables, such as f(x, y, z) = √(xyz)?
-For a function of three variables, the gradient is given by the partial derivatives with respect to each variable. For f(x, y, z) = √(xyz), the gradient is ∇f = (1/2 * y*z / √(xyz), 1/2 * x*z / √(xyz), 1/2 * x*y / √(xyz)).
What is the directional derivative for the function f(x, y, z) = √(xyz) in the direction of the vector (1, 2, -2) at the point (4, 2, 2)?
-The directional derivative is 1/6. This is calculated by finding the gradient at (4, 2, 2), resulting in (1/2, 1, 1), then taking the dot product with the unit vector (1/3, 2/3, -2/3), resulting in (1/6 + 2/3 - 2/3) = 1/6.

Outlines

00:00

📚 Introduction to Directional Derivatives

This paragraph introduces the concept of directional derivatives in the context of 3D functions. It explains how the gradient of a function is perpendicular to the level curves and points in the direction of the greatest increase of the function's value at a given point. The paragraph also discusses the rate of change of a function in a specific direction, which is not necessarily the direction of the gradient. The concept of a directional derivative is presented, defined as the rate of change of a function at a particular point in the direction of a given vector, with a common notation involving the gradient and the unit vector in the direction of interest.

05:01

🔍 Calculating Directional Derivatives

The second paragraph delves into the process of calculating directional derivatives. It begins by assuming knowledge of the gradient from a previous video and then explains how to find the rate of change in a given direction by using the dot product of the gradient and the unit vector in that direction. The paragraph simplifies the formula for the directional derivative and provides a step-by-step example using the function f(x, y) = x^2y^3, calculating the directional derivative in the direction of the vector (1, -1) at the point (-2, 1), including finding the gradient, evaluating it at the point, normalizing the direction vector, and performing the dot product to find the rate of change.

10:04

📈 Directional Derivatives of Multivariable Functions

This paragraph extends the concept of directional derivatives to functions of multiple variables, specifically focusing on the function f(x, y) = y * e^(xy). It outlines the process of finding the gradient of the function with respect to both x and y, using the product and chain rules. The paragraph then evaluates the gradient at the point (0, 2) and proceeds to calculate the directional derivative in the direction of the vector (3, -2). It includes the normalization of the direction vector to find the unit vector and the subsequent dot product to determine the rate of change at the specified point.

15:04

🌐 Directional Derivatives in 3D Space

The final paragraph discusses directional derivatives in the context of a function of three variables, f(x, y, z) = √(xy * z). It explains the process of finding the gradient of a function with three variables, which involves taking partial derivatives with respect to each variable. The paragraph evaluates the gradient at the point (4, 2, 2) and then calculates the directional derivative in the direction of the vector (1, 2, -2). This includes finding the unit vector for the given direction and performing the dot product with the gradient at the specified point to determine the rate of change.

Mindmap

Keywords

💡Function of x and y

A function of x and y is a mathematical relationship that assigns a unique output value for each pair of input values (x, y). In the context of the video, it is used to describe a surface in 3D space where the z-value represents the function's output for given x and y values. This concept is central to the video's theme of exploring the surface's properties and how changes in x and y affect the z-value.

💡Level curve

A level curve is a contour line on a graph where all points have the same z-value for a given function. In the video, level curves are used to visualize the constant values of the function across the surface in 3D space, helping to understand the areas where the function's output remains the same.

💡Gradient

The gradient is a vector that points in the direction of the greatest rate of increase of a function and whose magnitude represents the rate of change. In the video, the gradient is discussed as being perpendicular to the level curves and is essential for determining the direction of maximum increase on the surface.

💡Direction of greatest increase

The direction of greatest increase refers to the path along which the value of a function increases most rapidly at a given point. The video explains that moving in the direction of the gradient at point p will yield the fastest rate of increase for the z-value of the function.

💡Directional derivative

A directional derivative is the rate of change of a function at a specific point in a particular direction defined by a vector. The video introduces this concept to find the rate of change in various directions other than the gradient's direction, using the notation \( \nabla f \cdot \hat{v} \) at point p.

💡Unit vector

A unit vector is a vector with a magnitude of one, used to specify direction without regard to scale. In the script, the unit vector \( \hat{v} \) is derived from vector v by dividing it by its magnitude to ensure the directional derivative calculation is normalized and dimensionless.

💡Dot product

The dot product is a mathematical operation that takes two vectors and returns a scalar value, representing the product of the magnitudes of the vectors and the cosine of the angle between them. In the video, the dot product is used to calculate the directional derivative by combining the gradient and the unit vector in the desired direction.

💡Magnitude

The magnitude of a vector is its length, which in the context of the video, is used to normalize the vector to form a unit vector. The magnitude is essential in calculating the directional derivative, as it ensures the rate of change is independent of the vector's scale.

💡Instantaneous rate of change

The instantaneous rate of change is the limit of the rate of change as the time interval approaches zero, representing the derivative of a function at a specific point. The video discusses this concept in relation to the directional derivative, which provides the instantaneous rate of change in a specified direction at a given point.

💡Partial derivative

A partial derivative is the derivative of a function with respect to one variable, treating all other variables as constants. The video script mentions partial derivatives when calculating the gradient of functions of multiple variables, such as \( \frac{\partial f}{\partial x} \) and \( \frac{\partial f}{\partial y} \), which are components of the gradient vector.

💡Chain rule

The chain rule is a fundamental principle in calculus for differentiating composite functions. In the script, it is applied when taking partial derivatives of functions that are themselves composed of other functions, such as \( e^{x \cdot y} \), where the derivative of the inner function with respect to the variable of interest is multiplied by the derivative of the outer function.

Highlights

Introduction to directional derivatives and their significance.

Explanation of level curves and their relationship with the gradient.

The gradient of a function is always normal or perpendicular to the level curve.

The gradient indicates the direction of greatest increase at a point.

Moving in the direction of the level curve results in no change in the function's z value.

Directional derivatives measure the rate of change in a specific direction.

Formula for the directional derivative involving the gradient and a unit vector.

Calculation example using the function f(x, y) = x^2 y^3 and vector (1, -1) at point (-2, 1).

Gradient of f(x, y) = x^2 y^3 is computed as (2xy^3, 3x^2 y^2).

Evaluation of the gradient at point (-2, 1) resulting in (-4, 12).

Conversion of vector (1, -1) to a unit vector (1/√2, -1/√2).

Dot product calculation leading to a directional derivative of -8√2.

Second example with function f(x, y) = y e^(xy) and vector (3, -2) at point (0, 2).

Gradient of f(x, y) = y e^(xy) computed as (y^2 e^(xy), e^(xy)(1 + xy)).

Evaluation of the gradient at point (0, 2) resulting in (4, 1).

Conversion of vector (3, -2) to a unit vector (3/√13, -2/√13).

Dot product calculation leading to a directional derivative of 10/√13.

Third example with function f(x, y, z) = √(xyz) and vector (1, 2, -2) at point (4, 2, 2).

Gradient of f(x, y, z) = √(xyz) computed as (yz/(2√(xyz)), xz/(2√(xyz)), xy/(2√(xyz))).

Evaluation of the gradient at point (4, 2, 2) resulting in (1/2, 1, 1).

Conversion of vector (1, 2, -2) to a unit vector (1/3, 2/3, -2/3).

Dot product calculation leading to a directional derivative of 1/6.

Transcripts

Browse More Related Video

Directional Derivatives | What's the slope in any direction?

Directional derivatives and slope

The Gradient of a Function (Calculus 3)

Why the gradient is the direction of steepest ascent

Gradient and graphs

Directional derivative

Directional Derivatives (Calculus 3)

Takeaways

Q & A

What is a level curve in the context of a function of two variables?

How is the gradient of a function related to level curves?

What is a directional derivative?

How do you calculate the directional derivative of a function?

What is the significance of the gradient's magnitude in relation to directional derivatives?

How is the unit vector v̂ found from a vector v?

How can you express the directional derivative without directly calculating the angle between vectors?

What is the gradient of the function f(x, y) = x²y³?

How do you evaluate the gradient at a specific point?

What is the final value of the directional derivative for the function f(x, y) = x²y³ in the direction of the vector (1, -1) at the point (-2, 1)?

How do you find the gradient for a function of three variables, such as f(x, y, z) = √(xyz)?

What is the directional derivative for the function f(x, y, z) = √(xyz) in the direction of the vector (1, 2, -2) at the point (4, 2, 2)?