First Derivative Calculus Grade 12 | What Is the First Derivative?
TLDRThis video delves into the concept of derivatives in calculus, emphasizing their importance in determining the gradient of curves, as opposed to straight lines. The instructor explains how to calculate the first derivative and use it to find the gradient at any point on a graph. Through various examples, the video illustrates how the derivative helps identify whether the slope is positive, negative, or zero, thereby offering a deeper understanding of the relationship between derivatives and gradients in calculus.
Takeaways
- 📚 The script is an educational video explaining the concept of derivatives in calculus, emphasizing their importance for understanding the rest of grade 12 calculus.
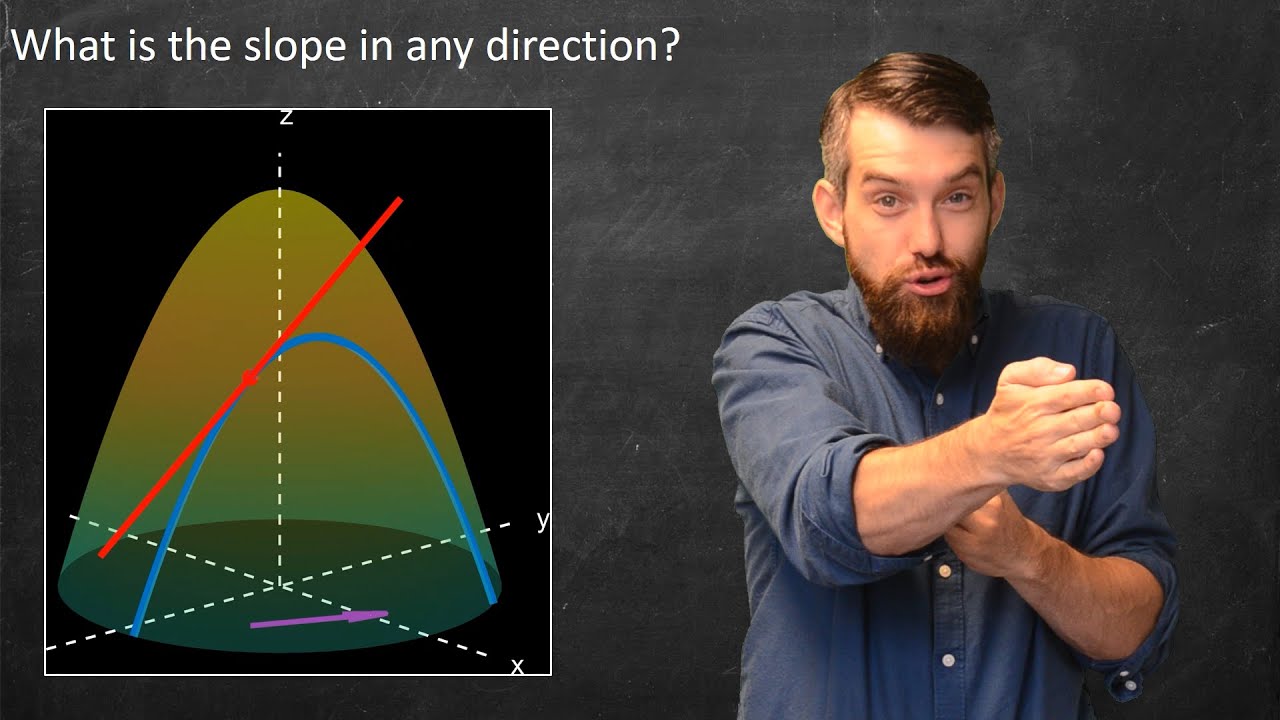
- 🔍 Derivatives represent the gradient or slope of a curve, contrasting with the method used for straight lines, which is not applicable to curves.
- 👨🏫 Isaac Newton and Gottfried Leibniz are credited with developing calculus, which includes the ability to calculate the gradient of a curve.
- 📈 The process of calculating the first derivative of a function is demonstrated, involving basic algebraic operations like multiplication and simplification.
- 📉 The derivative function describes the gradient at any point on the curve, which can be determined by plugging in an x-value into the derivative formula.
- 📊 The script uses the example of a roller coaster to illustrate the concept of a graph going uphill (positive gradient) or downhill (negative gradient).
- 👉 The video provides a practical demonstration of plugging in x-values to find the gradient at specific points on the curve, such as x = 1, x = 3, and x = -1.
- 📌 The gradient at a point where the graph turns or is flat is expected to be zero, which is verified by plugging in the specific x-value into the derivative formula.
- 🔄 The script explains the inverse relationship between knowing the gradient and finding the corresponding x-value on the graph.
- 🧠 The main takeaway is that the first derivative is a powerful tool for determining the gradient at any point on a curve, given the x-value.
- 🔑 Understanding the concept of derivatives is highlighted as crucial for grasping the broader topics within calculus.
Q & A
What is the main concept discussed in the video?
-The main concept discussed in the video is the derivative in calculus, its significance, and how it represents the gradient of a curve at any given point.
Why is the concept of the derivative important for understanding calculus?
-The derivative is important because it allows us to calculate the gradient or slope of a curve at any point, which is a fundamental aspect of understanding changes in functions and is essential for further studies in calculus.
Who are the mathematicians mentioned in the video that contributed to the development of calculus?
-Isaac Newton and Gottfried Leibniz are the mathematicians mentioned in the video who are credited with the development of calculus.
What is the difference between calculating the gradient of a straight line and a curve?
-The gradient of a straight line is calculated using the formula (y2 - y1) / (x2 - x1), whereas for a curve, this formula is not applicable. Calculus provides a method to calculate the gradient of a curve at any point using the derivative.
How is the first derivative of a function calculated in the video?
-The first derivative is calculated by applying the derivative rules to each term of the function. In the video, the function 3x^2 + 4x - 2 - x is differentiated to get 6x - 4 - 1, which simplifies to 6x - 5.
What does the first derivative of a function represent?
-The first derivative of a function represents the gradient or the rate of change of the function at any given point along the curve.
How can you determine if a portion of a graph is going uphill or downhill?
-You can determine if a portion of a graph is going uphill or downhill by looking at the sign of the gradient. A positive gradient indicates an uphill slope, while a negative gradient indicates a downhill slope.
What is the significance of the gradient being zero at a certain point on the graph?
-A gradient of zero at a certain point on the graph indicates that the graph is flat at that point, which means there is no change in the function's value, and it could be a point of inflection or a local maximum or minimum.
How can you find the x-value for a given gradient using the derivative?
-If you know the gradient at a certain point and have the derivative, you can set the derivative equal to the known gradient and solve for x to find the x-value where the gradient is that specific value.
What is the practical application of understanding the derivative in real-world scenarios?
-Understanding the derivative has practical applications in various fields such as physics for calculating velocity and acceleration, economics for finding maximum profit points, and engineering for optimizing designs.
Why might the calculated gradient at a point not be exactly zero even when the graph appears flat?
-The calculated gradient might not be exactly zero due to rounding errors in the x-value used for the calculation. The actual x-value might be slightly different, causing a small discrepancy in the result.
Outlines
📚 Understanding the Derivative in Calculus
This paragraph introduces the concept of the derivative in calculus, emphasizing its importance for understanding the rest of grade 12 calculus. It explains that the derivative represents the gradient, which was previously calculated using the formula for straight lines. The paragraph highlights the work of Isaac Newton and Gottfried Leibniz in developing calculus to calculate the gradient of a curve. The process of finding the first derivative of a given function is demonstrated, and the concept of gradient is illustrated by choosing specific x-values and calculating the corresponding derivative values, showing how the gradient can be negative (downhill) or positive (uphill) depending on the part of the curve being considered.
🔍 Calculating Gradient at Any Point with the First Derivative
The second paragraph delves deeper into the power of the first derivative, which allows for the calculation of the gradient at any point on a graph, given the first derivative and the specific x-value of interest. The instructor uses the example of a graph's turning point to illustrate how the gradient approaches zero, indicating a flat section of the graph. The summary also touches on the inverse problem of determining the x-value for a given gradient by setting the first derivative equal to a specific value and solving for x. This paragraph reinforces the significance of the first derivative as a tool for both understanding the gradient at any point on a graph and for finding x-values corresponding to particular gradients.
Mindmap
Keywords
💡Derivative
💡Gradient
💡Calculus
💡Isaac Newton
💡Gottfried Leibniz
💡First Derivative
💡Function
💡Graph
💡X Value
💡Positive Gradient
💡Negative Gradient
Highlights
The derivative in calculus represents the gradient, a concept previously understood in the context of straight lines.
Isaac Newton and Gottfried Leibniz developed calculus to extend the concept of gradient to curves, not just straight lines.
The formula for calculating the gradient of a straight line is y2 - y1 / x2 - x1, which is not applicable to curves.
Calculus allows for the computation of the gradient of a curve at any point, a significant advancement in mathematical analysis.
The process of finding the first derivative of a function involves basic algebraic manipulations such as multiplying coefficients.
The first derivative of a function describes the gradient at any given point on the curve.
Understanding the derivative makes the rest of grade 12 calculus more comprehensible.
The gradient can be negative, indicating a downward slope on a graph, as demonstrated with an example.
Selecting an x value and plugging it into the derivative formula yields the gradient at that specific point on the curve.
A gradient of minus two indicates a negative slope, which corresponds to a downward movement on the graph.
A positive gradient corresponds to an upward slope, or an increasing portion of the graph.
The gradient at a specific point can be calculated by substituting an x value into the derivative formula.
A gradient of zero indicates a flat or horizontal portion of the graph, suggesting no change in the function's value.
Rounding errors can affect the precision of calculated gradients, especially near zero values.
The first derivative is a powerful tool for determining the gradient at any point on a graph, given the x value.
If the gradient is known, it's possible to work backwards to find the corresponding x value on the graph.
The video emphasizes the importance of understanding the derivative as a fundamental concept in calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: