AP Physics B Kinematics Presentation General Problems #10

The New Jersey Center for Teaching and Learning
28 Jun 201211:43
EducationalLearning
32 Likes 10 Comments

TLDRThis script explores the physics of a horizontally thrown ball from a 75-meter tall building at 4.6 m/s. It covers the time to hit the ground, horizontal distance covered, and final velocity using kinematic equations. The solution finds a 3.9-second fall time, an 18-meter horizontal range, and a final velocity of 38.3 m/s, illustrating projectile motion principles.

Takeaways
  • 📚 The problem involves a horizontally thrown ball from a 75m tall building with an initial speed of 4.6 m/s.
  • 🏗 The initial vertical velocity (v_y) is 0 m/s, indicating a purely horizontal throw.
  • 📉 The horizontal acceleration (ax) is 0 m/s², as there is no influence in the horizontal direction.
  • 📈 The vertical acceleration (ay) is due to gravity, which is 9.8 m/s².
  • 📍 The initial position in the horizontal direction (x) is 0 m, and the initial vertical position (y) is 75 m.
  • ⏱ The goal is to find the time it takes for the ball to hit the ground, disregarding air resistance.
  • 🔍 The third kinematic equation (y = y₀ + v_y*t + 0.5*ay*t²) is chosen to solve for the time of flight.
  • 🔢 The time (T) to hit the ground is calculated to be approximately 3.9 seconds, considering the initial vertical velocity is zero.
  • 📏 To find the horizontal distance from the building where the ball lands, the equation x = x₀ + vx*T is used.
  • 🌐 The horizontal range is calculated to be about 18 meters, based on the time of flight and initial horizontal velocity.
  • 🚀 The final velocity of the ball just before impact is determined using the Pythagorean theorem, resulting in approximately 38.3 m/s.
Q & A

  • What is the initial vertical velocity of the ball when thrown from the roof?

    -The initial vertical velocity (v_y) of the ball is 0 m/s because it is thrown horizontally.

  • What is the acceleration in the horizontal direction for the ball in projectile motion?

    -The horizontal acceleration (ax) is 0 m/s² since there is no acceleration in the horizontal direction for projectile motion.

  • What is the acceleration due to gravity acting on the ball?

    -The acceleration due to gravity (ay) is 9.8 m/s², which is the standard acceleration acting on the ball in the vertical direction.

  • What is the initial height of the ball above the ground when thrown?

    -The initial height (y_initial) of the ball is 75 meters from the ground when thrown from the roof of the building.

  • How do we determine the time it takes for the ball to hit the ground?

    -We determine the time using the kinematic equation y = y_initial + (1/2) * a_y * t², where y is the final height (0 m), y_initial is the initial height, a_y is the acceleration due to gravity, and t is the time.

  • What is the formula used to calculate the horizontal distance the ball travels before hitting the ground?

    -The horizontal distance is calculated using the equation x = x_initial + v_x * t, where x_initial is the initial horizontal position (0 m), v_x is the horizontal velocity, and t is the time taken to hit the ground.

  • How far from the building will the ball land?

    -The ball will land approximately 18 meters away from the building, calculated using the horizontal velocity and the time it takes to hit the ground.

  • What is the final vertical velocity of the ball just before it hits the ground?

    -The final vertical velocity (v_y_final) just before the ball hits the ground is calculated using v_y_final = a_y * t, which is 9.8 m/s² * 3.9 s, resulting in approximately -38 m/s.

  • What is the horizontal velocity of the ball just before it hits the ground?

    -The horizontal velocity (v_x) remains constant at 4.6 m/s because there is no horizontal acceleration.

  • How do we calculate the resultant velocity of the ball just before it hits the ground?

    -The resultant velocity (V) is calculated using the Pythagorean theorem: V = √(v_x² + v_y²), where v_x is the horizontal velocity and v_y is the vertical velocity just before impact.

  • What is the magnitude of the final velocity of the ball just before it hits the ground?

    -The magnitude of the final velocity is approximately 38.3 m/s, calculated using the Pythagorean theorem with the horizontal and vertical velocities.

Outlines
00:00
💥 Projectile Motion Time Calculation

This paragraph discusses the physics of a horizontally thrown ball from a 75-meter tall building at an initial speed of 4.6 m/s. The focus is on calculating the time it takes for the ball to hit the ground, assuming no air resistance. The key variables are identified: initial horizontal velocity (vx), vertical velocity (vy), acceleration due to gravity (g), and initial positions in both x and y directions. The kinematic equation used to solve for time (t) is y = y0 + (1/2)gt^2, with the final height set to 0 m. After substituting the given values, the calculated time is approximately 3.9 seconds, indicating when the ball will impact the ground.

05:00
🏃 Horizontal Distance Calculation

The second paragraph delves into calculating the horizontal distance the ball travels before hitting the ground. The kinematic equations are reviewed, and the third equation, x = x0 + vx*t, is chosen for its simplicity and relevance to the problem. Since there is no horizontal acceleration (ax = 0), the equation simplifies to x = vx*t. Using the previously calculated time of 3.9 seconds and the initial horizontal velocity of 4.6 m/s, the horizontal distance covered is approximately 18 meters.

10:00
🚀 Final Velocity Before Impact

The final paragraph addresses the calculation of the ball's velocity just before it hits the ground. It explains that while the horizontal velocity (vx) remains constant at 4.6 m/s, the vertical velocity (vy) changes due to gravity. The vertical velocity is calculated using vy = g*t, resulting in approximately -38 m/s after 3.9 seconds. The horizontal velocity remains unaffected by gravity. To find the resultant velocity, the Pythagorean theorem is applied: v = √(vx^2 + vy^2). The magnitude of the final velocity is found to be approximately 38.3 m/s, highlighting an increase in speed due to gravity's influence.

Mindmap
Keywords
💡Projectile Motion
Projectile motion refers to the motion of an object thrown or projected into the air, which moves in a curved path under the action of gravity. In the video, the theme revolves around analyzing the projectile motion of a ball thrown horizontally from a building. The script discusses how the ball's motion can be broken down into horizontal and vertical components, which are independent of each other due to the absence of air resistance.
💡Horizontal Velocity (Vx)
Horizontal velocity, denoted as 'Vx', is the speed of an object in the horizontal direction. In the script, the initial horizontal velocity of the ball is given as 4.6 m/s, which remains constant throughout the motion since there is no horizontal acceleration. This concept is crucial for calculating the horizontal distance the ball travels before hitting the ground.
💡Vertical Velocity (Vy)
Vertical velocity, or 'Vy', is the speed of an object in the vertical direction. The script specifies that the initial vertical velocity is 0 m/s because the ball is thrown horizontally. As the ball falls, 'Vy' changes due to gravity, which is a key factor in determining the time it takes for the ball to hit the ground.
💡Acceleration Due to Gravity (G)
Acceleration due to gravity, represented as 'G', is the acceleration that an object experiences due to the Earth's gravitational pull, which is approximately 9.8 m/s². In the video, this value is used to calculate the change in the ball's vertical velocity and its eventual impact on the ground.
💡Initial Position
The initial position is the starting point of an object's motion. In the script, the initial position for both the horizontal (X) and vertical (Y) components is set to 0 m and 75 m above the ground, respectively. These values are essential for setting up the equations to solve for the time and distance of the ball's flight.
💡Kinematic Equations
Kinematic equations are formulas used to describe the motion of an object when forces are acting upon it. The script uses three specific kinematic equations to calculate the time of flight, horizontal distance, and final velocity of the ball. These equations relate displacement, initial velocity, time, acceleration, and final velocity.
💡Time of Flight
Time of flight is the duration an object spends in motion before it lands or reaches its target. The script calculates the time it takes for the ball to hit the ground using the kinematic equation for vertical motion, which involves the initial and final vertical positions and the acceleration due to gravity.
💡Horizontal Range
Horizontal range is the horizontal distance an object travels during its flight. The script calculates this by using the horizontal velocity and the time of flight, demonstrating how far the ball travels horizontally before it hits the ground.
💡Resultant Velocity
Resultant velocity is the overall velocity of an object in motion, considering both its horizontal and vertical components. In the script, the final velocity of the ball just before it hits the ground is calculated using the Pythagorean theorem, combining the horizontal and vertical velocities to find the magnitude of the resultant velocity.
💡Significant Figures
Significant figures are the digits in a number that carry meaningful information about its precision. The script mentions the importance of using significant figures correctly when calculating the final velocity of the ball, ensuring the accuracy of the result without rounding errors.
💡Quadratic Equation
A quadratic equation is a polynomial equation of the second degree, which often appears in physics problems involving motion under constant acceleration. Although the script mentions that a quadratic equation is not necessary for solving the time of flight due to the absence of initial vertical velocity, it is an important mathematical tool for related problems where initial conditions differ.
Highlights

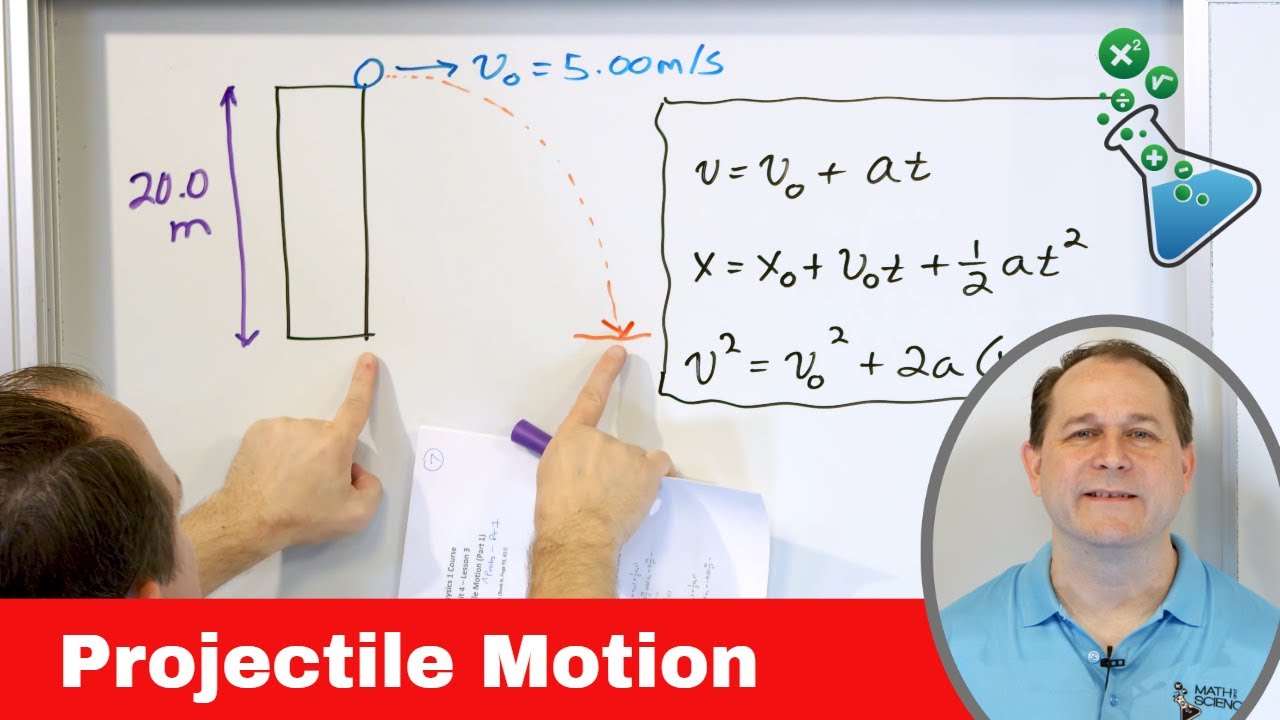
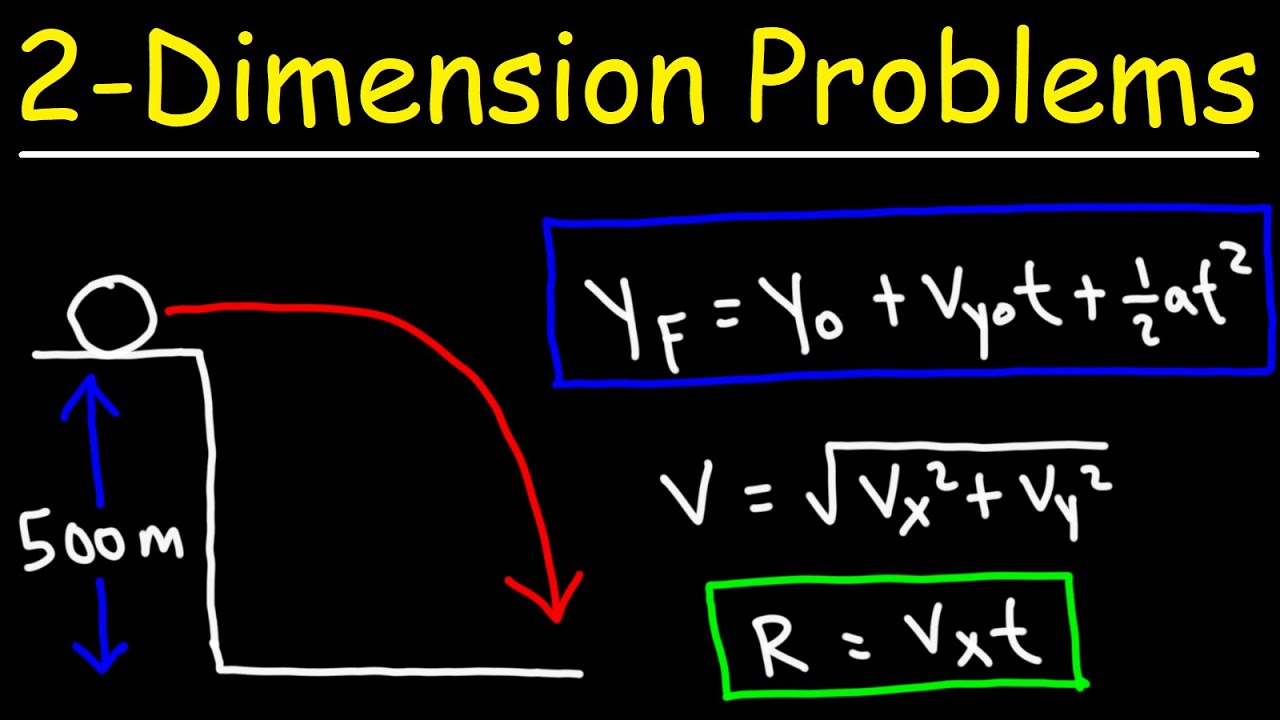
A ball is thrown horizontally from the roof of a 75m tall building at 4.6 m/s.

Initial horizontal velocity (vx) is 4.6 m/s, vertical velocity (vy) is 0 m/s.

Horizontal acceleration (ax) is 0 m/s², vertical acceleration (ay) is 9.8 m/s² due to gravity.

The ball's initial position is x = 0m, y = 75m.

The goal is to find the time it takes for the ball to hit the ground.

The third kinematic equation y = y + vy*t + 1/2*ay*t² is chosen to solve for time.

Since vy = 0, the equation simplifies to y = 1/2*ay*t².

Solving for t² gives t² = 2*(y - y0) / ay.

Taking the square root of both sides yields t = sqrt(2*(y - y0) / ay).

Plugging in values, the time to hit the ground is calculated as 3.9 seconds.

Part B asks for the horizontal distance the ball lands from the building.

The horizontal range is calculated using the equation x = vx*t.

With vx = 4.6 m/s and t = 3.9s, the horizontal distance is 18 meters.

Part C asks for the ball's velocity just before it hits the ground.

The vertical velocity (vy) is calculated as ay*t = 9.8 m/s² * 3.9s = 38 m/s.

The horizontal velocity (vx) remains 4.6 m/s as there is no horizontal acceleration.

The resultant velocity magnitude is found using the Pythagorean theorem: v = sqrt(vx² + vy²).

The final velocity magnitude is calculated as 38.3 m/s.

Significant figures are discussed to ensure the calculated velocity is accurate.

Transcripts

Browse More Related Video

Projectile Launched of a Cliff

Projectile launched off a cliff at an angle

AP Physics B Kinematics Presentation General Problems #12

Solving Projectile Motion Problems in Physics - [1-4-7]

Two Dimensional Motion Problems - Physics

Physics 3.5.4a - Projectile Practice Problem 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Projectile MotionPhysics CalculationHorizontal ThrowImpact TimeVelocity AnalysisFree FallGravity EffectKinematic EquationsMotion StudyEducational Content