Electric Flux

Andrey K
19 Nov 201307:35
EducationalLearning
32 Likes 10 Comments

TLDRThis script discusses the concept of electric fields and charges, focusing on the transition from point charges to more complex shapes. It explains the limitations of Coulomb's law for point charges and introduces Gauss's law as a more general relationship. The script delves into electric flux, illustrating its calculation for both uniform and non-uniform electric fields, and how it's approximated using integral calculus for complex surfaces. It emphasizes electric flux as a measure of electric field lines passing through a surface, setting the stage for Gauss's law.

Takeaways
  • 🔋 The concept of point charges and their electric fields are discussed, with the electric field being calculated using Coulomb's Law for stationary point charges.
  • 📐 Coulomb's Law is expressed as E = k * q / (4 * π * ε₀ * D²), where E is the electric field, q is the charge, D is the distance, ε₀ is the permittivity of free space, and k is Coulomb's constant.
  • 🤔 The limitations of Coulomb's Law are highlighted, noting that it only applies to point charges and not to more complex shapes or distributions of charge.
  • 🌀 Gauss's Law is introduced as a more general relationship between electric charges and electric fields, applicable beyond the point charge scenario.
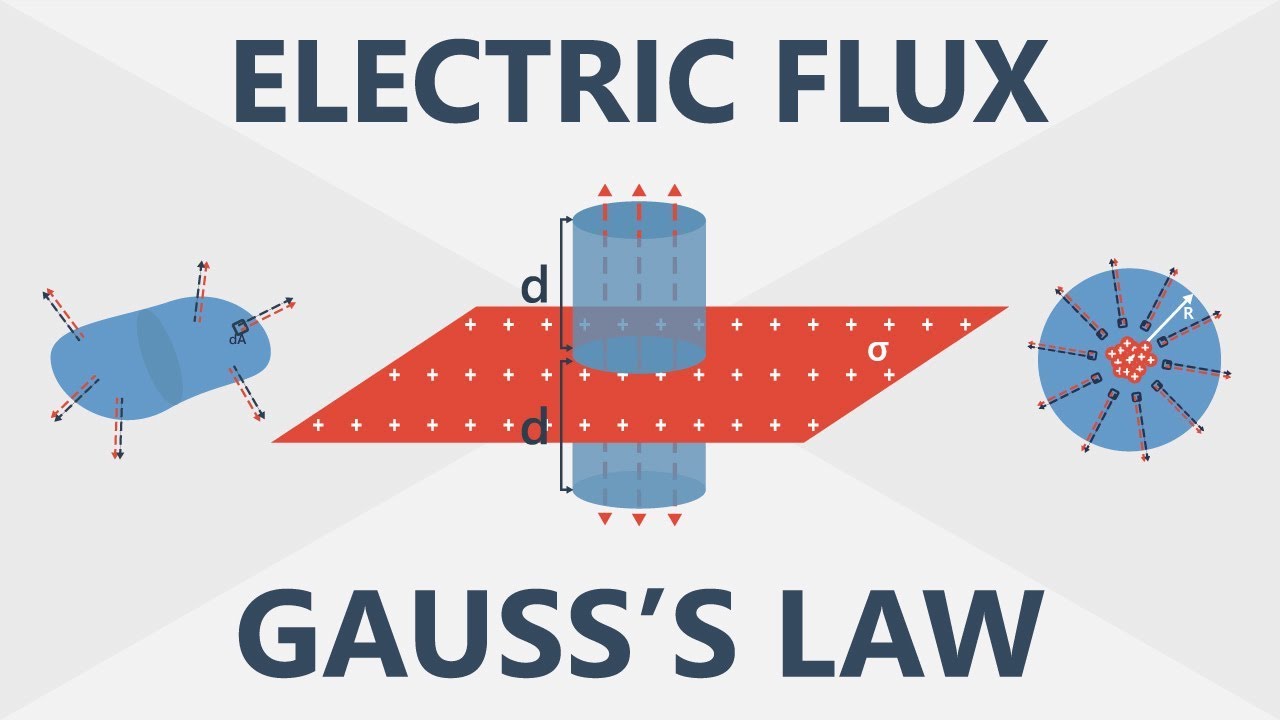
  • 🌐 Electric flux is defined and discussed as the number of electric field lines passing through a given surface, with the formula Φ = E * A * cos(θ), where A is the area and θ is the angle between the field lines and the area vector.
  • 📏 An example is provided to calculate electric flux through a square surface with a side length of 2 m and a constant electric field of 3000 N/C, resulting in an electric flux of 6000 N·m²/C.
  • 📐 The general formula for electric flux in non-uniform fields or non-flat surfaces is given by the integral ∫E * dA, where dA is an infinitesimally small area element.
  • 📚 The script emphasizes the importance of dividing a complex surface into small elements to approximate the electric flux when the field is not uniform or the surface is not flat.
  • 🔍 The concept of taking the limit as the area elements approach zero to convert the sum of dot products into an integral is explained, which is crucial for calculating exact electric flux values.
  • 🌌 The integral form of the electric flux formula is shown to be applicable for closed paths over a surface, assuming a non-uniform electric field and a non-flat area.
  • 🔄 The script concludes by reinforcing the idea that electric flux is related to the number of electric field lines passing through a surface, setting the stage for further discussion on Gauss's Law.
Q & A

  • What is the basic formula for calculating the electric field created by a point charge?

    -The electric field E created by a point charge q at a distance D away is given by the formula E = k * q / D^2, where k is Coulomb's constant.

  • What is the limitation of using Coulomb's law for calculating electric fields?

    -Coulomb's law is only applicable when the electric charge is considered to be a point charge, and it does not account for the shape and size of the charge distribution.

  • What is the concept of electric flux?

    -Electric flux is a measure of the number of electric field lines that pass through a given surface and is defined as the dot product of the electric field E and the area vector A.

  • How is electric flux calculated when the electric field is uniform and the surface is flat?

    -In such a case, the electric flux Φ is calculated as Φ = E * A * cos(θ), where θ is the angle between the area vector and the electric field lines, and when θ is zero, cos(θ) equals one, simplifying to Φ = E * A.

  • What is the formula for electric flux through a square surface with a side length of 2 m when the electric field is constant at 3000 N/C?

    -Assuming the surface is flat and at an angle of 60° to the field lines, the electric flux Φ is calculated using Φ = E * A * cos(60°) = 3000 * (2^2) * 0.5 = 6000 N·m/C.

  • What is the significance of the angle between the area vector and the electric field lines in calculating electric flux?

    -The angle determines the component of the electric field that contributes to the flux through the surface. The cosine of the angle is used in the calculation to find this component.

  • How can we approximate the electric flux through a non-uniform electric field or a non-flat surface?

    -We divide the surface into small elements, assume each element has a constant electric field, and calculate the flux for each element. The total flux is the sum of the fluxes through each element.

  • What is the general formula for electric flux when dealing with non-uniform electric fields or non-flat surfaces?

    -The general formula for electric flux is the integral over the surface of the product of the electric field E and the differential area vector dA, represented as ∫E·dA.

  • What is Gauss's law and how does it relate to electric flux?

    -Gauss's law states that the total electric flux through a closed surface is proportional to the total charge enclosed by the surface. It is a more general relationship between electric charges and electric fields than Coulomb's law.

  • Why is it necessary to use an integral for calculating electric flux in certain situations?

    -An integral is necessary when the electric field is not uniform or the surface is not flat, as it allows for the precise calculation of the flux by considering the contributions from every point on the surface.

  • How does the concept of electric flux relate to Gauss's law?

    -Gauss's law uses the concept of electric flux to state that the electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space (ε₀), expressed as ∫E·dA = Q_enclosed/ε₀.

Outlines
00:00
🔋 Understanding Point Charges and Electric Fields

This paragraph introduces the concept of electric charges, traditionally considered as point charges, and their creation of electric fields. It explains how the electric field is calculated using Coulomb's Law for a stationary point charge 'q' at a distance 'D' away, using the formula E = k * q / (4 * π * ε₀ * D²), where k is Coulomb's constant, ε₀ is the permittivity of free space, and D is the distance squared. The paragraph also raises the question of how to calculate the electric field when the charge distribution is not a simple point charge, such as a 'blob' of charge, and introduces the need for a more general relationship beyond Coulomb's Law.

05:01
🌐 Exploring Electric Flux and Gauss's Law

The second paragraph delves into electric flux, a measure of the electric field lines passing through a given surface. It explains the calculation of electric flux through a flat surface in a uniform electric field using the dot product of the area vector and the electric field vector, simplified when the angle between them is zero. An example is provided to calculate the electric flux through a square surface with a side length of 2 meters under a constant electric field of 3000 Newtons per Coulomb at a 60-degree angle to the field lines, resulting in an electric flux of 6000 Newtons meter squared per Coulomb. The paragraph then discusses the approximation of electric flux for non-uniform electric fields and non-flat surfaces by dividing the surface into small elements, leading to the integral formula for electric flux, ∮E • dA, which is used when discussing Gauss's Law in the context of non-uniform fields and complex charge distributions.

Mindmap
Keywords
💡Point Charge
A point charge refers to an electric charge that is assumed to be located at a single point in space, having no physical size or shape. This concept is fundamental in the script as it simplifies the calculations of electric fields using Coulomb's law. For instance, the script discusses how the electric field at a point 'a' distance 'D' away from a point charge 'q' is calculated using Coulomb's law, which is applicable only under the assumption of point charges.
💡Electric Field
The electric field is a vector field that surrounds electric charges and exerts a force on other charges placed within it. In the script, the electric field is described as being created by stationary point charges and is calculated using the equation derived from Coulomb's law. The script also discusses how the electric field is visualized with field lines and how its magnitude and direction affect the calculation of electric flux.
💡Coulomb's Law
Coulomb's law is the fundamental principle that describes the electrostatic interaction between electrically charged particles. It states that the electric force between two point charges is proportional to the product of the magnitudes of the charges and inversely proportional to the square of the distance between them. The script uses Coulomb's law to derive the equation for the electric field created by a point charge.
💡Electric Flux
Electric flux is a measure of the electric field passing through a given surface. It is a scalar quantity that represents the number of electric field lines passing through a surface. The script introduces the concept of electric flux as a way to quantify the field lines passing through a surface, such as a square area, and how it is calculated in both uniform and non-uniform electric fields.
💡Gauss's Law
Gauss's law, also known as the flux theorem, is a fundamental law of electromagnetism that relates the electric flux through a closed surface to the total charge enclosed by the surface. The script mentions Gauss's law as a more general relationship between electric charges and electric fields, applicable when the charge distribution is not a simple point charge.
💡Dot Product
The dot product is a mathematical operation that takes two vectors and returns a scalar. In the context of the script, the dot product is used to calculate the electric flux through a surface when the electric field is not perpendicular to the surface. The script gives an example where the dot product is used to find the electric flux through a square surface at a 60-degree angle to the field lines.
💡Magnitude
The magnitude of a vector is its length and represents the size of the vector without direction. In the script, the magnitude of the electric field and the area vector are used in the calculation of electric flux, particularly when the angle between the field lines and the surface normal is not zero.
💡Cosine
The cosine function is a trigonometric function that relates the angle of a right triangle to the ratio of the adjacent side to the hypotenuse. In the script, cosine is used in the calculation of electric flux when the angle between the electric field and the surface normal is not zero, as seen in the example with the square surface at a 60-degree angle.
💡Uniform Electric Field
A uniform electric field is an electric field where the magnitude and direction of the field are constant throughout the field. The script discusses the calculation of electric flux in a uniform electric field and contrasts it with the more complex scenario of a non-uniform field.
💡Three-Dimensional Region
A three-dimensional region is a space that extends in length, width, and height. The script mentions dividing a non-flat, three-dimensional region into smaller elements to approximate the electric flux when the electric field is not uniform, illustrating the complexity of such calculations.
💡Integral
An integral is a mathematical concept that represents the area under a curve or the sum of an infinite series of infinitesimally small quantities. In the script, the integral is used to calculate the exact electric flux through a surface when the electric field is not uniform and the surface is not flat, by summing the product of the electric field and the area elements over the entire surface.
Highlights

Point charges create their own electric fields, and the field's quantity is calculated using an equation derived from Coulomb's law.

Coulomb's law is applicable only when the stationary electric charge is assumed to be a point charge.

Gauss's law provides a more general relationship between electric charges and electric fields than Coulomb's law.

Electric flux is a concept that describes the number of electric field lines passing through a given surface.

The electric flux through a flat surface can be calculated using the dot product of the area vector and the electric field.

When the angle between the area vector and electric field lines is zero, the electric flux is the product of the electric field magnitude and area magnitude.

The units of electric flux are Newtons multiplied by meters squared per Coulomb.

For non-uniform electric fields or non-flat surfaces, the electric flux must be approximated by dividing the surface into small elements.

The general formula for electric flux is an integral over the product of the electric field and an infinitesimally small area element.

Gauss's law will utilize the concept of electric flux to define a relationship for non-point charges.

The angle between the electric field lines and the surface affects the calculation of electric flux.

For a constant electric field and flat surface, the electric flux can be directly calculated without approximation.

The electric field's magnitude and the area's magnitude are key factors in calculating electric flux for a flat surface at a specific angle.

An example calculation demonstrates finding the electric flux through a square surface with given dimensions and field strength.

The electric flux calculation must consider the angle between the surface and the electric field lines for accurate results.

In cases of non-uniform electric fields, the approximation of electric flux involves summing the dot products of small surface elements and their respective electric fields.

Transcripts

Browse More Related Video

Ultimate Gauss' Law review

Electric Flux (part 1)

The Electric Flux and It's Applications

Electric Flux, Gauss's Law & Electric Fields, Through a Cube, Sphere, & Disk, Physics Problems

Electric Flux and Gauss’s Law | Electronics Basics #6

Electric flux meaning (& how to calculate it) | Electrostatics | Physics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Electric FieldPoint ChargesCoulomb's LawGauss's LawElectric FluxPhysics ConceptsElectric ChargeField CalculationFlux IntegrationEducational Content