8. Atoms IV

The script begins with an announcer's introduction to MIT OpenCourseWare, highlighting its commitment to providing free educational resources and inviting donations to support its mission. The professor then dives into a discussion on the physics of the Lamb shift, a phenomenon in quantum mechanics that accounts for the coupling of atomic systems with the electromagnetic field. The explanation includes the impact of fluctuating electric fields on the electron's oscillatory motion and the subsequent modification of the Coulomb potential, leading to a change in the electron's binding energy.

This paragraph delves deeper into the Lamb shift, distinguishing between two contributions: the smearing of the Coulomb potential and vacuum polarization. The latter, often misunderstood as the main contribution, is actually only a small part of the total Lamb shift. The professor clarifies that vacuum polarization involves virtual electron-positron pairs that shield the Coulomb field, leading to a complex interplay between shielding and the actual measurement of the charge, ultimately affecting the binding energy.

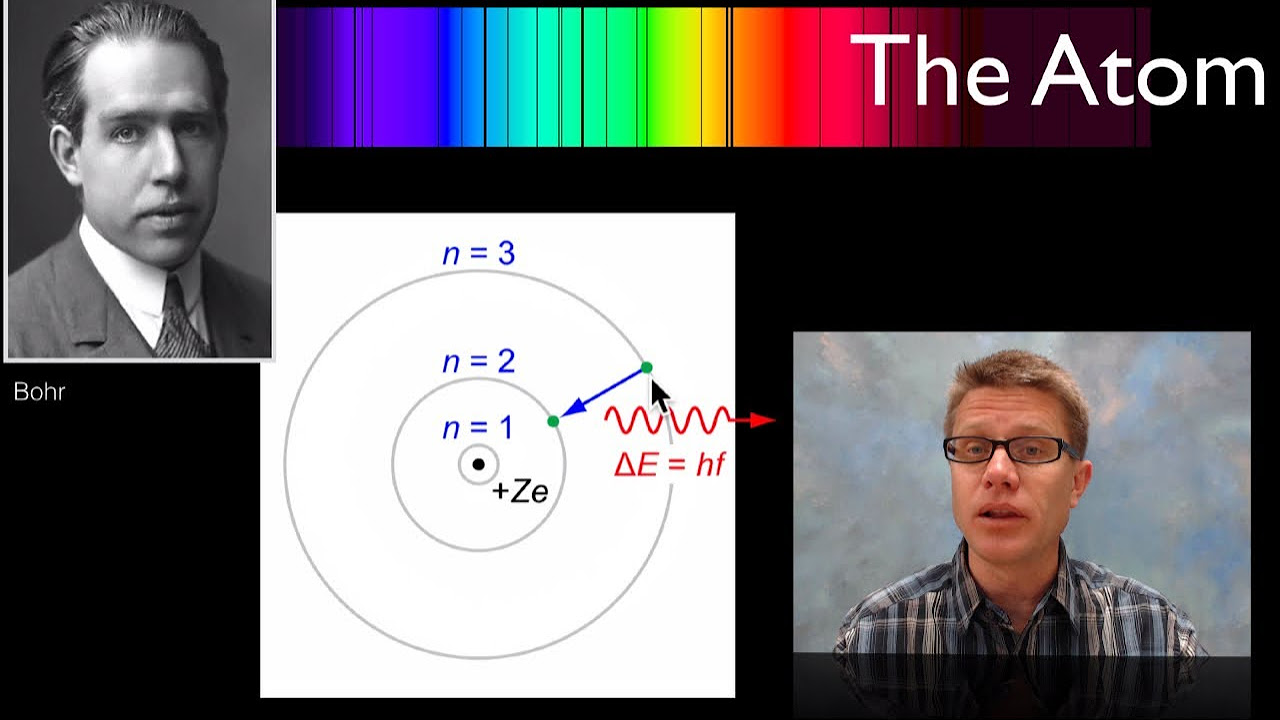
The focus shifts to the hyperfine structure, which arises from the nucleus's structure and its interaction with the electron's magnetic field. The professor outlines the various contributions to hyperfine structure, including the nuclear magnetic moment, quadrupole moment, finite mass, and volume effects. The significance of hyperfine splitting is emphasized, particularly for hydrogen, where it results in multiple ground states with distinct angular momentum quantum numbers. This has practical implications for atomic spectroscopy, magnetic trapping, and the study of nuclear properties.

The professor discusses the broader implications of hyperfine structure, especially its utility in astronomical observations and the determination of nuclear properties. The famous 21-centimeter line of hydrogen, crucial for observing hydrogen in the universe, is highlighted. Furthermore, the use of hyperfine structure in atomic spectroscopy for precise measurements of nuclear characteristics, such as the size of the proton, is underscored. The discussion also touches on the potential for studying unstable nuclei through atomic physics measurements.

The script presents a semi-classical derivation of the internal magnetic field created by an electron at the nucleus's position, considering both the electron's orbital motion and spin. The importance of the delta function contribution for s electrons is emphasized, as it is the dominant effect in many scenarios. The derivation leads to an understanding of the hyperfine interaction, which is described by the Zeeman Hamiltonian of the nucleus in the magnetic field created by the electron.

This paragraph explores the quantum mechanical aspects of hyperfine structure, focusing on the conditions under which the hyperfine interaction occurs. The professor explains that the hyperfine constant involves the nuclear magnetron and the Bohr magneton, with a particular emphasis on the g factors of the nucleus and their differences from the electron's g factor. The calculation of the hyperfine splitting for hydrogen is detailed, leading to the famous 1420 megahertz result.

The script addresses the principles of parity and time reversal symmetry, which restrict the possible electric and magnetic moments of a nucleus. It is argued that odd electric and even magnetic moments would violate these symmetries, leading to the conclusion that certain moments, such as the electric dipole moment, are not possible. The discussion also highlights the recent experimental results that place stringent bounds on the electric dipole moment of the electron.

The professor poses questions about the minimum angular momentum required for a nucleus to have a magnetic dipole or an electric quadrupole moment. Through a series of questions and answers, the script reveals that a nucleus must have at least some angular momentum to exhibit these moments. The argument is made that without the ability to orient an object in space, one cannot determine its moments, and thus, they would not exist.

The script concludes the discussion on the minimum requirements for nuclear moments and introduces the concept of quadrupole moments. It is explained that quadrupole moments are the leading electric moments for a nucleus, and the conditions under which they can exist are discussed. The importance of symmetry in determining the possible moments of a nucleus is reiterated.

The script discusses isotope effects on atomic energy levels, focusing on the mass and volume effects due to differences in isotopes. The mass effect is illustrated by the reduced mass in the Rydberg formula, which affects the energy levels of atoms with different isotopes. The volume effect is explained by the finite size of the nucleus and its impact on the Coulomb potential experienced by the electron. Both effects are shown to influence the binding energy of the electron, with lighter nuclei generally leading to stronger binding.

This paragraph provides a more detailed analysis of isotope effects, including the impact of different isotopes on the magnetic moment and quadrupole deformation. The professor emphasizes that while the mass and volume effects are significant, the nuclear structure also varies between isotopes, leading to different hyperfine structures. The script concludes with a discussion on how these effects can be separated and measured in experiments, providing insight into the properties of different isotopes.