Unit II: Lec 9 | MIT Calculus Revisited: Single Variable Calculus
TLDRThis MIT OpenCourseWare lecture delves into Rolle's Theorem and its implications, illustrating the theorem's intuitive principle that a smooth curve rising and falling must flatten at some point. The professor elucidates the theorem's formal mathematical conditions and its significance in calculus. The lecture further explores the Mean Value Theorem, demonstrating its use in proving geometrically obvious facts analytically, such as the relationship between constant derivatives and parallel curves. The session concludes with insights into the Mean Value Theorem's role in the study of indefinite integrals, setting the stage for the inverse of differentiation.
Takeaways
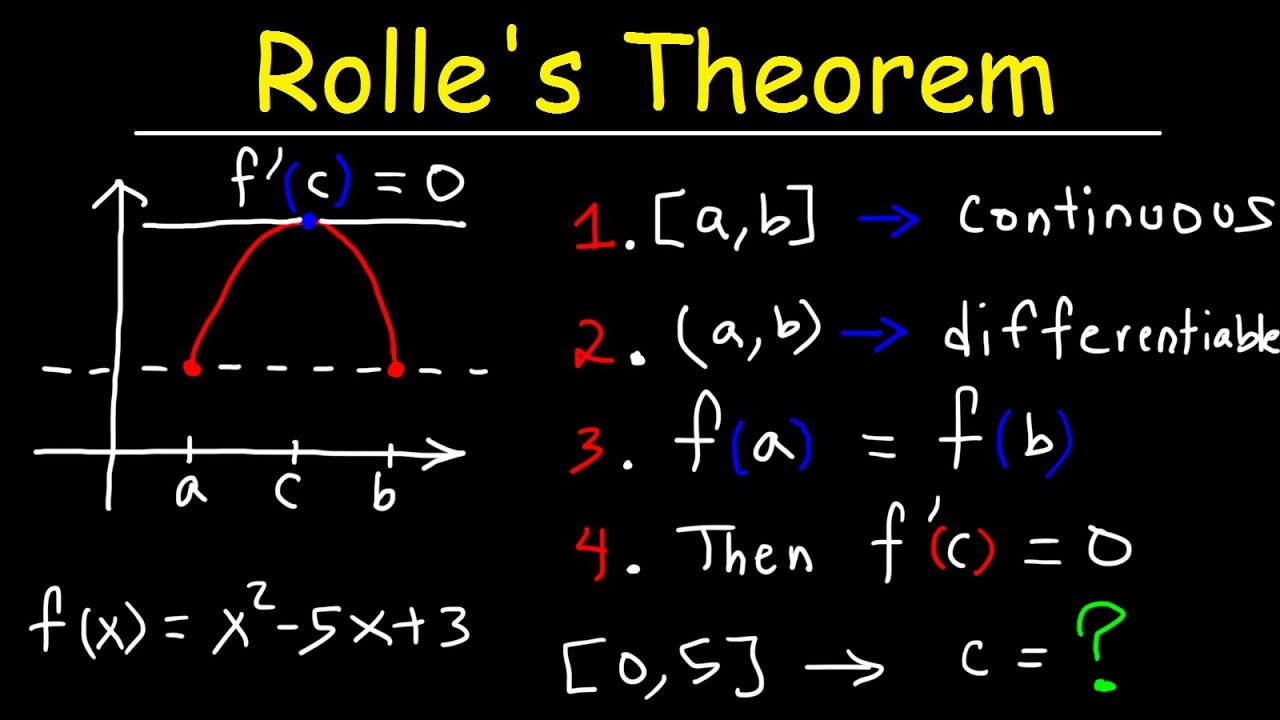
- 📚 The lecture introduces Rolle's Theorem, which states that if a function is continuous on a closed interval and differentiable on an open interval, with both endpoints yielding the same value, there must be at least one point where the derivative is zero.
- 🔍 Rolle's Theorem is an 'existence theorem', meaning it assures the existence of at least one 'c' in the interval where the derivative equals zero, but it does not specify how many such points there are or their exact locations.
- 📉 The importance of Rolle's Theorem lies in its implications and applications, particularly as a foundational concept for the Mean Value Theorem, which is a crucial result in calculus.
- 📈 The Mean Value Theorem extends the idea of Rolle's Theorem, stating that for a continuous and differentiable function on an interval, there exists at least one point where the instantaneous rate of change equals the average rate of change over that interval.
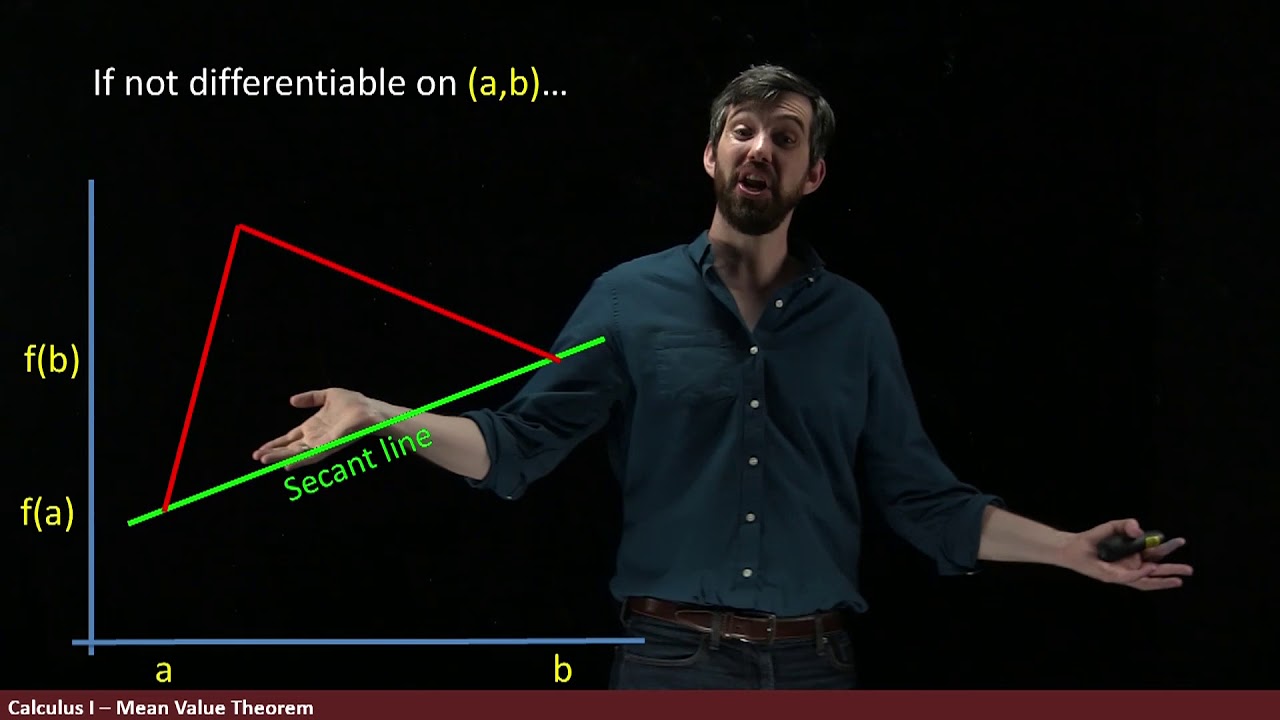
- 🔧 A geometric interpretation of the Mean Value Theorem is that the slope of the tangent to the curve at some point is equal to the slope of the secant line connecting two points on the curve.
- 👀 The script emphasizes the importance of understanding the conditions under which theorems apply, such as the necessity for a function to be single-valued and differentiable for Rolle's and the Mean Value Theorems to hold.
- 📝 The Mean Value Theorem is not just a theoretical concept; it is used to prove many geometrically intuitive facts that have an analytic counterpart, providing a rigorous basis for what might otherwise be assumed true.
- 📉 A corollary of the Mean Value Theorem states that if a function's derivative is identically zero, then the function itself must be constant. This is a direct application of the theorem, showing that the function does not change value over the interval.
- ⏏️ Another corollary discussed is that if two functions have identical derivatives over an interval, the difference between the two functions is a constant. This is a powerful tool for understanding the relationship between functions and their derivatives.
- 🔑 The script uses the Mean Value Theorem to transition into the study of indefinite integrals, setting the stage for exploring the inverse process of differentiation, which is a fundamental concept in calculus.
- 🌐 The lecture concludes with a reminder of the importance of understanding and applying these theorems correctly, as they form the basis for deeper mathematical analysis and comprehension.
Q & A
What is the main topic of the lecture?
-The main topic of the lecture is 'Rolle's Theorem and Its Consequences', which discusses the theorem and its implications in calculus.
What does Rolle's Theorem state in the context of a function?
-Rolle's Theorem states that if a function 'f' is continuous on the closed interval [a, b], differentiable on the open interval (a, b), and f(a) = f(b) = 0, then there exists at least one 'c' in the open interval (a, b) such that the derivative of 'f' at 'c' is 0.
What is the intuitive meaning of Rolle's Theorem?
-The intuitive meaning of Rolle's Theorem is that if a curve is unbroken and smooth, starting and ending at the same point, there must be at least one point where the curve levels off, meaning it has a horizontal tangent.
Why is it important for the function to be continuous on the closed interval and differentiable on the open interval in Rolle's Theorem?
-It is important for the function to be continuous on the closed interval to ensure the curve is unbroken, and differentiable on the open interval to ensure the curve is smooth without sharp corners or discontinuities, which are necessary conditions for Rolle's Theorem to apply.
What is an 'existence theorem' in the context of Rolle's Theorem?
-An 'existence theorem' is a type of theorem that asserts the existence of at least one element or condition that satisfies a certain property, without necessarily providing a method to find or identify that element or condition. Rolle's Theorem is an existence theorem because it guarantees the existence of at least one 'c' where the derivative is 0.
What is the Mean Value Theorem, and how is it related to Rolle's Theorem?
-The Mean Value Theorem states that if a function is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one 'c' in the open interval (a, b) such that the average rate of change of the function over [a, b] equals the instantaneous rate of change at 'c'. It is related to Rolle's Theorem because it extends the idea that if a function starts and ends at the same level, there must be a point where the slope of the tangent equals the average slope.
What is the geometric interpretation of the Mean Value Theorem?
-The geometric interpretation of the Mean Value Theorem is that for a smooth curve representing the function 'f', if you draw a straight line (chord) between two points on the curve, there must be at least one point on the curve where the tangent line is parallel to the chord, indicating that the instantaneous rate of change equals the average rate of change.
Why is the Mean Value Theorem considered more general than Rolle's Theorem?
-The Mean Value Theorem is considered more general because it does not require the function to have the same value at the endpoints (a and b). It only requires that the function is continuous on the closed interval and differentiable on the open interval, making it applicable to a wider range of functions.
What is a potential pitfall in using a less rigorous proof of the Mean Value Theorem?
-A potential pitfall in using a less rigorous proof of the Mean Value Theorem is that it may overlook certain conditions, such as the single-valuedness of the function with respect to different coordinate systems, which could lead to incorrect conclusions.
How does the Mean Value Theorem facilitate the study of indefinite integrals?
-The Mean Value Theorem facilitates the study of indefinite integrals by showing that if the derivative of a function is identically 0, the function itself must be constant. This understanding is crucial when reversing the process of differentiation to find the original function, which is the essence of integration.
What is the significance of the Mean Value Theorem in proving certain geometrically intuitive results?
-The significance of the Mean Value Theorem in proving certain geometrically intuitive results is that it provides an analytical method to confirm whether these intuitive results hold true. For example, it can be used to prove that if two functions have identical derivatives over an interval, their difference must be a constant, which geometrically means the curves are parallel.
Outlines
📚 Introduction to Rolle's Theorem and MIT OCW Support
The script begins with an introduction to MIT OpenCourseWare and a call for donations to support free educational resources. The lecture's focus is on Rolle's Theorem, which is likened to the idea that a smooth curve rising and falling must flatten at some point. The theorem's formal definition is presented, requiring a function 'f' to be continuous on a closed interval and differentiable on an open interval, with 'f(a)' and 'f(b)' equaling zero, ensuring the existence of at least one point 'c' where the derivative is zero. The proof's intuition is explained through the behavior of a curve and its maximum value, and an example is given to illustrate the importance of the curve's continuity at endpoints.
🔍 Deeper Insights into Rolle's Theorem and Its Limitations
This paragraph delves deeper into Rolle's Theorem, highlighting it as an 'existence theorem' that guarantees the presence of at least one critical point but does not specify its number or location. The lecturer cautions that the theorem applies only if the function is differentiable (smooth) and emphasizes the difference between a curve's continuity and differentiability. A contrived example is used to show what can go wrong without these conditions, and the importance of the function being single-valued is discussed, with an example illustrating how a multivalued function might not satisfy the theorem's conditions.
🌟 Rolle's Theorem as a Prelude to the Mean Value Theorem
The importance of Rolle's Theorem is discussed in the context of its role as a stepping stone to more significant results, particularly the Mean Value Theorem. The Mean Value Theorem is introduced with its formal statement, which is an extension of Rolle's Theorem, requiring the function to be continuous on a closed interval and differentiable on an open interval, with the added condition that the average rate of change equals the instantaneous rate of change at some point 'c'. The geometric interpretation of this theorem is provided, likening it to a particle's instantaneous speed equaling its average speed at some point during its journey.
📉 Geometric Interpretation and Proof of the Mean Value Theorem
The geometric interpretation of the Mean Value Theorem is explored, with an analogy to a particle's motion and the relationship between average and instantaneous speed. The lecturer provides a visual explanation involving the slope of a chord and a tangent line, illustrating how the theorem implies the existence of a tangent line parallel to a chord between two points on the curve. The explanation is simplified by considering a rotated coordinate system, applying Rolle's Theorem to the new axes, and highlighting the importance of single-valuedness in the function's behavior.
🔑 The Mean Value Theorem as an Analytical Tool for Geometric Facts
The Mean Value Theorem is presented as a crucial analytical tool for proving geometrically obvious facts. The lecturer emphasizes that while intuitive results are valuable, the theorem provides a rigorous way to confirm their validity. An example is given where a function with a derivative always equal to zero must be constant, with a step-by-step proof using the Mean Value Theorem. The proof leverages the theorem's conditions to show that the function's values must be equal for any two points, thus proving its constancy.
📌 The Mean Value Theorem and Implications for Function Differences
The final paragraph discusses a corollary of the Mean Value Theorem, which states that if two functions have identical derivatives on an interval, their difference must be a constant. This is geometrically interpreted as the curves of the two functions being parallel. The proof involves considering the function formed by the difference of the two functions, showing its derivative to be zero, and thus concluding that the difference is a constant. This result is highlighted as a bridge to the study of indefinite integrals and the inverse of differentiation.
🙌 Closing Remarks and Call for Donations to MIT OCW
The script concludes with a call for donations to support MIT OpenCourseWare, ensuring the continuation of free and open access to MIT courses. The lecturer thanks the Gabriella and Paul Rosenbaum Foundation for funding the video's publication and signs off with a farewell, inviting viewers to join the next lecture for further exploration of calculus concepts.
Mindmap
Keywords
💡Rolle's Theorem
💡Differentiable
💡Continuous
💡Derivative
💡Mean Value Theorem
💡Existence Theorem
💡Single Valued
💡Constant Function
💡Parallel Curves
💡Indefinite Integral
Highlights
Rolle's Theorem states that if a function is continuous on a closed interval and differentiable on an open interval with the same endpoints, and the function values at the endpoints are equal, then there exists at least one point in the interval where the derivative is zero.
Rolle's Theorem is an existence theorem that guarantees the existence of at least one critical point but does not specify its location or quantity.
The theorem's proof is intuitive, suggesting that a function that rises and falls without breaking the x-axis must have a maximum where its derivative is zero.
An example is provided to illustrate the importance of the function being continuous and smooth, without sharp corners or discontinuities.
The importance of the function being single-valued is discussed to ensure that the derivative exists and Rolle's Theorem can be applied.
The Mean Value Theorem is introduced as a direct consequence and extension of Rolle's Theorem, applicable when the function's values at the endpoints are not necessarily zero but equal.
The Mean Value Theorem is described as a fundamental result in calculus, often used to prove geometrically obvious facts with an analytic counterpart.
An intuitive explanation of the Mean Value Theorem is given, comparing it to a particle's instantaneous speed equaling its average speed at some point during its movement.
A geometric interpretation of the Mean Value Theorem is presented, relating the slope of the tangent line to the slope of the chord connecting two points on the curve.
The Mean Value Theorem is used to prove that if the derivative of a function is always zero, then the function itself must be constant.
The concept of parallel curves is introduced, explaining that if two functions have identical derivatives, they are parallel to each other, not necessarily identical.
The Mean Value Theorem is applied to show that if two functions have the same derivative, the difference between them is a constant.
The lecture emphasizes the importance of the Mean Value Theorem in calculus, as it serves as a foundation for proving many intuitively obvious geometric properties.
The Mean Value Theorem's role in the study of indefinite integrals is highlighted, as it allows for the investigation of the original function from its derivative.
The lecture concludes with a teaser for the next session, promising a deeper exploration of the inverse of taking a derivative, or the indefinite integral.
Funding for the publication of the lecture video is acknowledged, with a call to action for donations to support MIT OpenCourseWare.
Transcripts
Browse More Related Video

Lec 21 | MIT 18.01 Single Variable Calculus, Fall 2007

Lec 22: Green's theorem | MIT 18.02 Multivariable Calculus, Fall 2007

Calculus Chapter 3 Lecture 26 The FTIC
Rolle's Theorem
The MEAN Value Theorem is Actually Very Nice
Mean value theorem | Derivative applications | Differential Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: