Mean value theorem | Derivative applications | Differential Calculus | Khan Academy
TLDRThe Mean Value Theorem is a fundamental concept in calculus that asserts the existence of a point 'c' within a closed interval 'a' to 'b' where the derivative (instantaneous rate of change) of a function equals the average rate of change over that interval. This video breaks down the theorem's intuition, explains its conditions of continuity and differentiability, and illustrates it with visual examples and a real-world analogy. It also demonstrates how to apply the theorem analytically to a quadratic function, highlighting its practical implications and clarifying common misconceptions.
Takeaways
- 📌 The Mean Value Theorem (MVT) is an important concept in calculus that might seem complex but has an intuitive underlying principle.
- 📈 The MVT states that if a function f(x) is continuous and differentiable over a closed interval [a, b], there exists at least one point 'c' in the interval where the derivative (f'(c)) equals the average rate of change over [a, b].
- 🔍 To find the average rate of change, you calculate the change in the function's value (f(b) - f(a)) divided by the change in the x-values (b - a).
- 🌟 The theorem can be visualized by drawing the graph of the function and a line representing the average slope between two points on the graph.
- 🛠️ Differentiability means that the function has a derivative at every point within the interval, and if the derivative is graphed, it should also be continuous.
- 🔗 The concept of continuity means that the function's graph is unbroken and connected at every point within the interval.
- 🚗 The MVT can be related to real-world scenarios, such as calculating average velocity where the average speed over a time interval corresponds to the instantaneous speed at some point within that interval.
- 📊 To apply the MVT analytically, one would find the derivative of the function and set it equal to the average slope to solve for the 'c' value.
- 📚 While the MVT is a fundamental concept, its direct application in practical scenarios is limited; it is more commonly used in theoretical mathematics.
- 💡 Understanding the MVT provides insight into the behavior of functions, particularly in relation to their slopes and the concept of differentiability.
Q & A
What is the Mean Value Theorem?
-The Mean Value Theorem states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one point c within the interval (a, b) where the derivative of the function, f'(c), is equal to the average rate of change of the function over the interval [a, b].
What does it mean for a function to be continuous?
-A function is continuous if, at every point within its domain, the function does not have any gaps or breaks. In other words, you can draw the function's graph without lifting your pen from the paper.
What is the definition of differentiability?
-A function is differentiable at a point if the graph of the function has a well-defined tangent line at that point. This means that the function has a derivative, which can be found by calculating the limit of the average rate of change as the interval size approaches zero.
What is the significance of the Mean Value Theorem in calculus?
-The Mean Value Theorem is significant because it establishes a fundamental relationship between the average rate of change of a function over an interval and the instantaneous rate of change (the derivative) at some point within that interval. This relationship is crucial for understanding the behavior of functions and their derivatives.
How does the Mean Value Theorem relate to real-world scenarios, such as velocity and position?
-The Mean Value Theorem can be applied to real-world scenarios like velocity and position. For example, if you have a varying velocity over a time interval, the theorem tells you that there must be a moment within that interval where your instantaneous velocity equals the average velocity. This provides insight into the behavior of objects in motion.
What is the difference between a closed interval and an open interval?
-A closed interval includes both endpoints of the interval, whereas an open interval does not include its endpoints. For example, in a closed interval [a, b], the function is defined at every point between a and b, including a and b, while in an open interval (a, b), the function is defined between a and b but not at a and b themselves.
How do you find the average rate of change of a function over an interval?
-To find the average rate of change of a function over an interval [a, b], you calculate the change in the function's value (f(b) - f(a)) divided by the change in the independent variable (b - a). This gives you the average slope of the function's graph over the interval.
What is the role of the derivative in the Mean Value Theorem?
-The derivative plays a crucial role in the Mean Value Theorem as it represents the instantaneous rate of change (or slope) of the function at a particular point. The theorem states that there exists a point c in the interval (a, b) where the derivative at c (f'(c)) is equal to the average rate of change of the function over the interval [a, b].
How can you determine if a function is differentiable at a point?
-A function is differentiable at a point if there exists a limit as the interval size approaches zero for the average rate of change of the function at that point. This limit is the derivative, which can be calculated using the limit definition of the derivative.
What is an example of a function that is continuous but not differentiable?
-An example of a function that is continuous but not differentiable is the absolute value function. The graph of the absolute value function has a sharp corner at the origin, which means there is no well-defined tangent line at that point, and thus the function is not differentiable at x = 0.
How does the Mean Value Theorem help in understanding the behavior of functions?
-The Mean Value Theorem helps in understanding the behavior of functions by establishing a connection between the average rate of change over an interval and the instantaneous rate of change at a specific point within that interval. This connection provides insights into how the function's slope varies and allows us to predict the existence of points with specific slope characteristics.
What is the analytical process to apply the Mean Value Theorem to a given function?
-To apply the Mean Value Theorem analytically, first ensure the function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b). Then, calculate the average rate of change of the function over the interval. After that, find the derivative of the function and solve for the value of the independent variable that makes the derivative equal to the calculated average rate of change. This value is the point where the instantaneous rate of change equals the average rate of change.
Outlines
📚 Introduction to the Mean Value Theorem
The speaker begins by addressing the audience's requests to learn about the Mean Value Theorem. They express their mixed feelings about the theorem, noting that while the concept is intuitively clear, its presentation in mathematical textbooks can be confusing, particularly for those new to calculus. The speaker aims to clarify the theorem with a visual explanation, starting with a discussion on the necessary conditions for a function f(x) to be applicable to the theorem: it must be continuous and differentiable over a closed interval [a, b]. The explanation includes a brief description of what it means for a function to be continuous and the difference between closed and open intervals.
📈 Visualizing the Mean Value Theorem
In this segment, the speaker continues the explanation by visually representing the Mean Value Theorem. They introduce the concept of average slope between two points a and b on a graph, using rise over run to define the slope. The speaker then explains how the theorem states that for a continuous, differentiable function over a closed interval, there exists at least one point c where the derivative (slope) at that point is equal to the average slope between a and b. The speaker uses an example to illustrate this concept, showing that even if the function is not explicitly known, one can visually identify points where the slope matches the average slope. They also mention that the theorem breaks down for functions that are continuous but not differentiable.
🚗 Real-world Application: Average Velocity
The speaker provides a real-world application of the Mean Value Theorem by relating it to average velocity. They describe a scenario where one's position changes over time, with varying velocity, and how the theorem can be applied to find a point in time where the instantaneous velocity equals the average velocity. The speaker then moves on to an analytical approach, using a specific function (f(x) = x^2 - 4x) over a closed interval [2, 4] to demonstrate the theorem's application. They calculate the average slope of the function over the interval and use the theorem to determine a value of x (in this case, x = 3) where the function's derivative equals the average slope.
📊 Graphing the Mean Value Theorem
The speaker concludes the explanation by graphing the function f(x) = x^2 - 4x and highlighting the key points discussed. They illustrate the graph of the function as a parabola and identify the points corresponding to the interval [2, 4]. The speaker emphasizes that despite the graph's appearance, there indeed exists a point (x = 3) where the slope of the function equals the average slope over the interval. They reiterate that the Mean Value Theorem simply states the existence of such a point with an instantaneous slope equal to the average slope between two given points, and aim to reassure the audience that the theorem, while seemingly complex, is fundamentally about the relationship between slopes at different points on a graph.
Mindmap
Keywords
💡Mean Value Theorem
💡Continuous
💡Differentiable
💡Closed Interval
💡Derivative
💡Average Slope
💡Instantaneous Slope
💡Tangent Line
💡Visual Explanation
💡Mathematical Intuition
Highlights
The Mean Value Theorem is introduced and its intuitive nature is discussed.
The Mean Value Theorem might be confusing for beginners due to abstract mathematical terms.
A visual explanation of the Mean Value Theorem is provided using a graph.
A function must be continuous and differentiable to apply the Mean Value Theorem.
The concept of a closed interval is explained, which is crucial for the theorem's application.
An example of a non-continuous function is given to illustrate the importance of continuity.
Differentiability means the function's derivative is also continuous.
The Mean Value Theorem states that there exists a point where the instantaneous slope equals the average slope over a closed interval.
A real-world analogy is provided by comparing average velocity to the Mean Value Theorem.
The Mean Value Theorem is applied analytically to a specific function and interval.
The average slope of a function over an interval is calculated using the function's values at the interval endpoints.
A point is found where the derivative of the function equals the average slope.
The Mean Value Theorem's significance in higher-level mathematics and its limited application in practical calculus problems is discussed.
The Mean Value Theorem provides insight into the behavior of functions and their slopes.
The transcript ends with a graphical representation of the Mean Value Theorem and its explanation.
Transcripts
Browse More Related Video
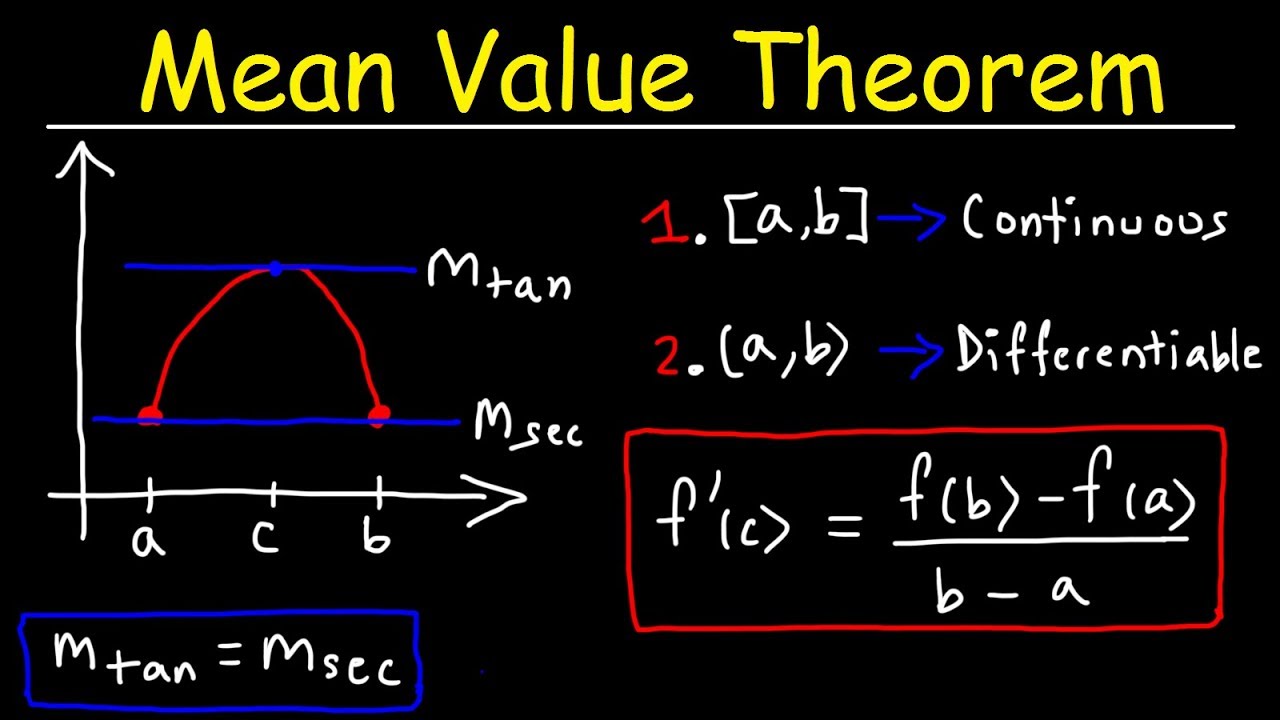
Mean Value Theorem
Mean Value Theorem with Example
Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy

Mean Value Theorem and Rolle's Theorem
Mean value theorem for integrals | AP Calculus AB | Khan Academy

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
5.0 / 5 (0 votes)
Thanks for rating: