Finding Arc Length Using an Integral

turksvids
18 Jan 202006:20
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial guides viewers through calculating the arc length of a function over a specific interval, a common task in calculus. The presenter explains the formula for arc length, involving the derivative of the function and a square root expression. The example given uses the function y = x^(2/3) from x = 1 to x = 8, demonstrating the process of finding the derivative, setting up the integral, and simplifying the expression. The video illustrates the use of u-substitution to evaluate the integral and find the exact arc length, emphasizing that while such problems often require a calculator, the example shown can be evaluated exactly, providing a clear method for students to follow.

Takeaways
  • 📚 The video is about calculating the arc length of a function on a specific interval.
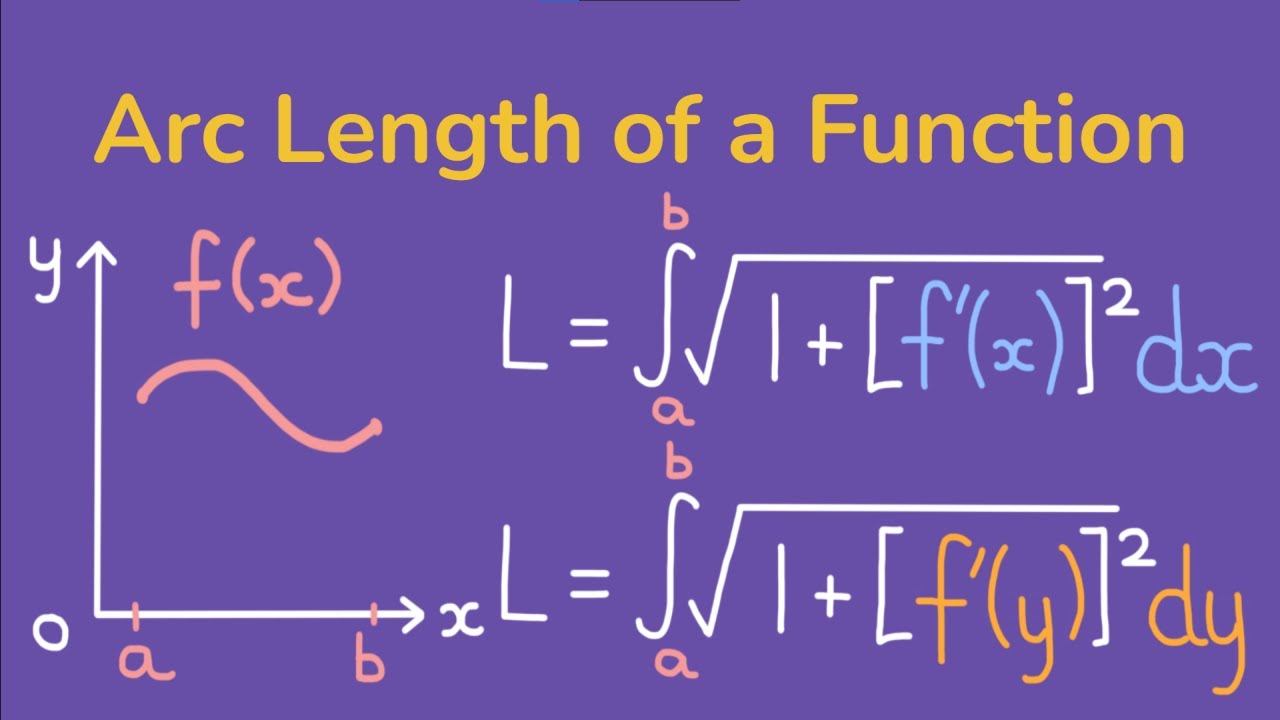
  • 🔍 The formula for arc length from x=a to x=b is √(1 + (dy/dx)^2), where dy/dx is the derivative of y with respect to x.
  • 📝 Derivatives are essential for finding the arc length, and the example given is y = x^(2/3).
  • 📈 The process involves setting up an integral with the square root of 1 plus the derivative squared in the numerator.
  • 🧩 The integral often requires simplification and might end up being a calculator problem or set up without evaluation.
  • 🔑 The example provided is s = ∫ from 1 to 8 of √(1 + (2/3 * x^(-1/3))^2) dx.
  • 📉 The derivative of y = x^(2/3) is found using the power rule, resulting in 2/3 * x^(-1/3).
  • 📝 After simplifying, the integral becomes ∫(1/8 * √(1 + 4/(9x^(2/3))) dx).
  • 🔄 Factoring and finding a common denominator helps in simplifying the integral for u-substitution.
  • 🔄 U-substitution is used with u = 9x^(2/3) + 4, and du is found accordingly.
  • 📊 The bounds of integration are converted from x values to u values, which are 13 and 40 respectively.
  • 📐 The final integral to solve is ∫(1/6 * u^(1/2) du) from 13 to 40, which can be evaluated.
  • 📝 The final result is given as 1/27 * (40^(3/2) - 13^(3/2)), without further simplification.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is calculating the arc length of a function on a specific interval.

  • What formula is used to calculate the arc length from x=a to x=b?

    -The formula used to calculate the arc length is the square root of (1 + (dy/dx)^2).

  • What is the derivative of y = x^(2/3)?

    -The derivative of y = x^(2/3) is (2/3)x^(-1/3) using the power rule.

  • Why might some arc length problems be difficult to evaluate?

    -Some arc length problems are difficult to evaluate because the integral of the square root of 1 plus something squared might not be easily solvable.

  • What is the integral set up for the example given in the video?

    -The integral set up for the example is the integral from 1 to 8 of the square root of (1 + (4/9)x^(-2/3)) dx.

  • How does the video simplify the expression under the square root in the integral?

    -The video simplifies the expression by factoring and finding a common denominator, which leads to recognizing a perfect square under the square root.

  • What substitution is used in the video to simplify the integral?

    -The video uses a u-substitution where u = 9x^(2/3) + 4, and du = (18/3)x^(-1/3) dx.

  • What are the new bounds for the integral after the u-substitution?

    -The new bounds for the integral are u = 13 when x = 1, and u = 40 when x = 8.

  • What technique is used to integrate the simplified expression in terms of u?

    -The technique used is integrating u^(3/2) with respect to u, which is a standard integration formula.

  • What is the final result of the integral after evaluation?

    -The final result is (1/27) times (40^(3/2) - 13^(3/2)), which is not simplified further in the video.

  • What does the video suggest about evaluating arc length integrals in general?

    -The video suggests that while it's common to set up the integral for arc length, it's not always necessary or easy to evaluate it to a numerical value.

Outlines
00:00
📚 Calculating Arc Length of a Function

This paragraph introduces the concept of calculating the arc length of a function over a specific interval, emphasizing the importance of knowing the formula involving the derivative of the function. The formula is the square root of (1 + (dy/dx)^2), where 'dy/dx' is the derivative of 'y' with respect to 'x'. The paragraph also hints at the complexity of evaluating such integrals and sets the stage for an example to demonstrate the process.

05:01
📘 Example Calculation of Arc Length for y = x^(2/3)

The paragraph presents a step-by-step example of calculating the arc length for the function y = x^(2/3) from x = 1 to x = 8. It begins by calculating the derivative, setting up the integral with the correct formula, and then simplifying the expression. The process includes squaring the derivative, simplifying the integrand, and using a common denominator to make the integral more manageable. The paragraph also discusses the potential for using u-substitution to simplify the integral further and sets up the substitution with 'u' representing the expression inside the radical, leading to a new integral in terms of 'u'.

Mindmap
Keywords
💡Arc Length
Arc length is a fundamental concept in calculus that refers to the distance along the curve between two points. In the video, it is the main focus, as the presenter aims to calculate the arc length of a specific function over an interval. The formula for arc length is given as the square root of \(1 + (dy/dx)^2\), which is integral to the video's theme of demonstrating how to calculate it for the function \(y = x^{2/3}\).
💡Derivative
The derivative of a function measures the rate at which the function's output changes with respect to changes in its input. In the context of the video, finding the derivative \(dy/dx\) of the function \(y = x^{2/3}\) is the first step in calculating the arc length, as it is necessary to apply the arc length formula.
💡Integral
An integral is a mathematical concept used to calculate the accumulated quantity of a variable and is the reverse process of differentiation. In the video, after setting up the expression for arc length, the presenter uses integration to find the exact arc length over the interval [1, 8].
💡Square Root
The square root operation is used to find a value that, when multiplied by itself, gives the original number. In the script, the square root is part of the formula for arc length, specifically the square root of \(1 + (dy/dx)^2\), which is crucial for setting up the integral to find the arc length.
💡Power Rule
The power rule is a basic differentiation rule stating that if \(y = x^n\), then \(dy/dx = nx^{n-1}\). In the video, the power rule is applied to find the derivative of \(y = x^{2/3}\), which is essential for the subsequent steps in calculating the arc length.
💡Anti-derivatives
Anti-derivatives, or indefinite integrals, are functions that can differentiate to give the original function. The script mentions that many arc length problems might end up as anti-derivatives, indicating that the process of finding an antiderivative is often part of solving such problems.
💡Set Up But Do Not Evaluate
This phrase refers to the process of preparing an integral for calculation without actually performing the integration. In the video, it is mentioned that many arc length problems are set up but not evaluated, suggesting that the setup is often sufficient to understand the problem or that the integral may be difficult to evaluate.
💡U-Substitution
U-substitution is a technique used in integration to simplify the integral by substituting a new variable \(u\) for a function of the original variable. In the script, the presenter uses u-substitution to transform the integral of the arc length formula, making it easier to evaluate.
💡Bounds
Bounds in the context of integration refer to the limits of integration, which define the interval over which the integral is calculated. In the video, the bounds are the values of \(x = 1\) and \(x = 8\), which are used to evaluate the definite integral for the arc length.
💡Factoring
Factoring is the process of breaking down a complex expression into simpler components. In the script, the presenter uses factoring to simplify the expression inside the square root in the arc length formula, making it possible to apply u-substitution and integrate.
💡Exact Value
An exact value refers to the precise numerical result of a calculation. The video script mentions that if a problem asks for the exact value of the arc length, it implies that there is a known technique to evaluate the definite integral, which is the goal of the video's example.
Highlights

Introduction to calculating the arc length of a function on a specific interval.

Explanation of the formula for arc length from x=a to x=b, involving the square root of 1 plus dy/dx squared.

The importance of finding the derivative (dy/dx) for arc length calculation.

The common occurrence of calculator problems or set up without evaluation in arc length problems.

An example problem provided to find the arc length of y=x^(2/3) from x=1 to x=8.

Application of the power rule to find the derivative of y=x^(2/3).

Setting up the integral for arc length using the derivative and the interval [1, 8].

Simplification of the integrand by squaring the derivative and combining terms.

Factoring to find a common denominator inside the radical for simplification.

Identification of the perfect square in the denominator to facilitate integration.

Use of u-substitution with u as the entire expression inside the radical.

Calculation of du and the transformation of the integral into terms of u.

Conversion of the bounds of integration from x to u values.

Integration of the simplified expression using u-substitution.

Final evaluation of the integral to find the exact arc length from 13 to 40.

Emphasis on the ability to evaluate integrals when finding the exact value of arc length.

Conclusion highlighting the practical steps and techniques used in the example.

Transcripts

Browse More Related Video

Arc Length of y=x^(3/2) | MIT 18.01SC Single Variable Calculus, Fall 2010

Lesson 11 - Arc Length In Parametric Equations (Calculus 2 Tutor)

Arc Length Calculus Problems,

Arc Length (Calculus 3)

Find the Arc Length of a Function with Calculus

Parametric Arclength | MIT 18.01SC Single Variable Calculus, Fall 2010

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Arc LengthCalculusIntegralsMath TutorialDerivativesEducationalMathematicsProblem SolvingX to the 2/3U-Substitution