Efficiency of a Carnot engine | Thermodynamics | Physics | Khan Academy

Khan Academy
18 Sept 200914:03
EducationalLearning
32 Likes 10 Comments

TLDRThis script delves into the concept of engine efficiency, denoted by the Greek letter eta, and its relation to work and energy input. It explains the Carnot cycle's efficiency in terms of heat transfer between two temperature reservoirs, highlighting the work done and the net work as key factors. The script further explores the mathematical relationship between efficiency and temperatures, concluding that the Carnot engine, operating between two given temperatures, is theoretically the most efficient engine possible.

Takeaways
  • 🔧 The efficiency of an engine, denoted by the Greek letter eta, is analogous to the everyday use of the word, measuring the effectiveness of resource use.
  • 🚀 In the context of engines, efficiency is defined as the ratio of work done to the energy provided to do that work.
  • 🔥 The energy provided to an engine, Q1, comes from a heat source, and the efficiency is calculated using the net work done and the heat given.
  • 📊 Efficiency can be expressed as a fraction or a percentage, indicating the proportion of heat energy converted into useful work.
  • ♻️ The Carnot cycle's efficiency is derived from the work done and the heat exchanged with temperature reservoirs.
  • 🔄 The change in internal energy over a complete Carnot cycle is zero, as the system returns to its initial state.
  • ↔️ The net heat applied to the system during a Carnot cycle is the difference between the heat input Q1 and the heat rejected Q2.
  • ∫ The work done by the system is equivalent to the integral of pressure times the change in volume (PdV) during an isothermal expansion.
  • 📚 Using the ideal gas law, the integral for Q1 can be simplified to involve constants and the natural logarithm of the volume ratio.
  • 🔢 The efficiency of a Carnot engine can be related to the temperatures of the heat reservoirs, leading to the formula 1 - T2/T1.
  • 🏆 The Carnot engine is theoretically the most efficient engine possible between two temperature reservoirs, though it may not be practical for all applications.
Q & A

  • What is the Greek letter eta used to represent in the context of engines?

    -In the context of engines, the Greek letter eta (η) is used to represent the efficiency of an engine.

  • How is the efficiency of an engine similar to the everyday use of the word 'efficient'?

    -The efficiency of an engine, like the everyday use of the word 'efficient', refers to how well a resource (time, money, energy) is utilized for a particular task or purpose.

  • What is the definition of efficiency in the context of engines?

    -In the context of engines, efficiency is defined as the ratio of the work done by the engine to the energy provided to do the work, essentially how much useful work is produced from the given energy.

  • What is the significance of the Carnot cycle in the discussion of engine efficiency?

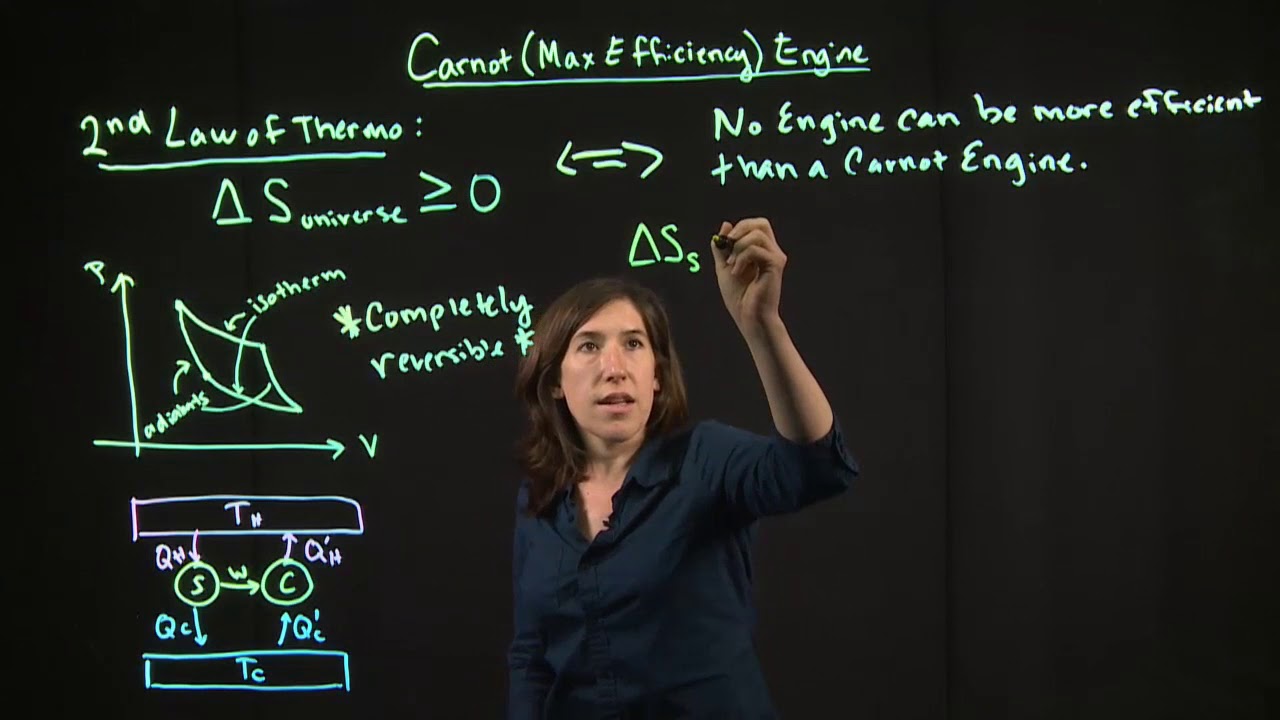
    -The Carnot cycle is significant in the discussion of engine efficiency because it provides a theoretical upper limit on the efficiency that any engine can achieve when operating between two temperature reservoirs.

  • What is the relationship between the work done by an engine and the heat energy provided to it?

    -The work done by an engine is the net work after accounting for the heat energy provided to it and the heat energy that may be released or absorbed during the process.

  • How is the efficiency of a Carnot engine related to the temperatures of the heat reservoirs?

    -The efficiency of a Carnot engine is directly related to the temperatures of the heat reservoirs, and it can be expressed as 1 - (T2/T1), where T1 is the temperature of the hot reservoir and T2 is the temperature of the cold reservoir.

  • What does the area inside the Carnot cycle represent in the PV diagram?

    -In the PV diagram, the area inside the Carnot cycle represents the net work done by the engine, which is the useful work output minus any work input required during the cycle.

  • What is the significance of the internal energy change being zero over a complete Carnot cycle?

    -The significance of the internal energy change being zero over a complete Carnot cycle is that it indicates the system has returned to its initial state, and the cycle is reversible, which is a key assumption in the Carnot cycle.

  • How is the efficiency of an engine calculated using the heat energies Q1 and Q2?

    -The efficiency of an engine can be calculated using the heat energies Q1 and Q2 as efficiency = (Q1 - Q2) / Q1, where Q1 is the heat energy added to the system and Q2 is the heat energy rejected by the system.

  • What is the practical implication of the Carnot engine's theoretical efficiency?

    -The practical implication of the Carnot engine's theoretical efficiency is that it sets a benchmark for the maximum possible efficiency for any heat engine operating between two temperature reservoirs, although it may not be achievable in real-world applications.

  • Why is the Carnot engine considered the most efficient engine theoretically?

    -The Carnot engine is considered the most efficient engine theoretically because it is based on a reversible cycle, which means there is no waste of energy and it operates at the maximum possible efficiency for the given temperature difference.

Outlines
00:00
🔧 Introduction to Engine Efficiency

This paragraph introduces the concept of engine efficiency, denoted by the Greek letter eta, which is analogous to the common use of the word in daily life. Efficiency is defined as the ratio of the work done to the energy provided to do that work. In the context of the Carnot cycle, the efficiency is calculated by considering the heat energy 'Q1' added from a high-temperature reservoir and the net work performed, which is the area inside the cycle diagram. The explanation also touches on the idea of efficiency in terms of time and money, emphasizing the importance of what is achieved with the given resources. The paragraph concludes by setting the stage for further exploration of how efficiency relates to the variables in the Carnot cycle.

05:01
🔄 Understanding Carnot Cycle Efficiency

The second paragraph delves deeper into the Carnot cycle's efficiency, using the concept of internal energy and the work-energy principle. It explains that the change in internal energy over a complete cycle is zero, which leads to the equation W (work done by the system) being equal to Q1 minus Q2. The paragraph then algebraically manipulates this relationship to express efficiency as the net heat applied to the system divided by the total heat input. It also explores the absolute values of Q1 and Q2, highlighting that Q1 is the work done during an isothermal expansion, which is equivalent to the area under the curve on a PV diagram. The explanation uses the ideal gas law to derive the integral expressions for Q1 and Q2, emphasizing the relationship between pressure, volume, and temperature during the isothermal processes of the Carnot cycle.

10:01
📉 Relating Efficiency to Temperature in the Carnot Cycle

The final paragraph establishes a direct relationship between the efficiency of a Carnot engine and the temperatures of the heat reservoirs. It begins by calculating the magnitudes of Q1 and Q2 using integral expressions derived from the ideal gas law, and then substitutes these into the efficiency formula. The paragraph demonstrates a simplification process that leads to the efficiency of a Carnot engine being expressed as 1 - T2/T1, where T1 and T2 are the absolute temperatures of the high and low-temperature reservoirs, respectively. This result is significant as it sets the stage for proving that the Carnot engine is theoretically the most efficient engine possible between two given temperature reservoirs, a point that will be further discussed in subsequent content.

Mindmap
Keywords
💡Efficiency
Efficiency, represented by the Greek letter eta, is a measure of how well an engine performs its intended function relative to the energy input. In the context of the video, it is analogous to how effectively one uses time or money. The script discusses engine efficiency as the ratio of work done to the energy provided, highlighting the importance of this concept in evaluating the performance of engines, especially in the Carnot cycle.
💡Carnot Cycle
The Carnot cycle is a theoretical cycle that represents the most efficient heat engine cycle possible between two temperature reservoirs. It is composed of two isothermal processes and two adiabatic processes. The video script uses the Carnot cycle to illustrate the concept of engine efficiency, showing how the work done and heat exchanged in this idealized cycle relate to the efficiency of an engine.
💡Quasistatic Process
A quasistatic process is one that occurs slowly enough that the system remains in equilibrium at all times. In the script, this concept is used to describe the movement of 'pebbles' as an analogy for the transfer of energy in a Carnot cycle, emphasizing the importance of maintaining equilibrium for reversible processes.
💡Reservoir
In the context of thermodynamics, a reservoir refers to a large energy source that can supply or absorb an infinite amount of heat without changing its temperature. The script mentions a 'first reservoir' as the source of energy (Q1) for the Carnot cycle, and a 'second reservoir' where heat (Q2) is released.
💡Net Work
Net work is the total work done by a system minus the work done on the system. The script explains that in the Carnot cycle, the net work is represented by the shaded area inside the cycle diagram, which is the difference between the heat added to the system and the heat given back to the second reservoir.
💡Internal Energy
Internal energy is the total energy contained within a system, including kinetic and potential energies of its molecules. The script uses the concept of internal energy to explain that over a complete Carnot cycle, the change in internal energy is zero, which is a property of state variables.
💡Entropy
Entropy is a thermodynamic property that measures the degree of randomness or disorder in a system. The script mentions that, like internal energy, entropy is also a state variable, and its change over a complete Carnot cycle is zero.
💡Isothermal Expansion
Isothermal expansion refers to the process where a gas expands at a constant temperature. In the script, this concept is used to describe the first part of the Carnot cycle, where heat (Q1) is added to the system during the expansion process at temperature T1.
💡Ideal Gas Law
The ideal gas law is given by PV = nRT, where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature. The script uses this law to derive the expressions for Q1 and Q2, the heat added and removed during the isothermal processes of the Carnot cycle.
💡Natural Logarithm
The natural logarithm is the logarithm to the base 'e' and is used in various mathematical and scientific contexts, including the calculation of integrals in thermodynamics. The script applies the natural logarithm to calculate the heat exchanged during the isothermal processes of the Carnot cycle, using the formula for Q1 and Q2.
💡Carnot Efficiency
Carnot efficiency is the theoretical maximum efficiency that any engine can achieve when operating between two temperature reservoirs. The script derives the formula for Carnot efficiency as 1 - T2/T1, indicating that the efficiency of a Carnot engine depends only on the temperatures of the heat source and sink.
Highlights

Introduction to the concept of engine efficiency represented by the Greek letter eta.

Efficiency in engines is analogous to everyday efficiency in terms of utilizing resources effectively.

Engine efficiency is defined as the work done with the energy provided.

Explanation of the Carnot cycle and its relevance to engine efficiency.

The energy given to the engine (Q1) is equivalent to the work done over a time period.

Net work done by the engine is represented by the shaded area in the Carnot cycle diagram.

Efficiency is expressed as a fraction, sometimes converted to a percentage.

The ability to transfer heat energy into useful work defines the efficiency of an engine.

Exploration of how efficiency varies with different variables in the Carnot cycle.

Internal energy change in a complete Carnot cycle is zero, as it is a state variable.

The net heat applied to the system is the difference between Q1 and Q2.

Work done by the system (W) equals Q1 minus Q2.

Efficiency can be redefined using the net heat applied to the system and the initial heat input.

Relating efficiency to temperatures in the Carnot cycle through algebraic manipulations.

Magnitude of Q1 and Q2 in terms of work done and heat transferred in the Carnot cycle.

Integral calculus used to determine the work done and heat transferred in isothermal processes.

Efficiency of a Carnot engine can be expressed as 1 minus T2 over T1.

The Carnot engine is theoretically the most efficient engine between two temperature sources.

The practicality and applicability of the Carnot engine in real-world scenarios are discussed.

Transcripts

Browse More Related Video

Carnot efficiency 2: Reversing the cycle | Thermodynamics | Physics | Khan Academy

Carnot efficiency 3: Proving that it is the most efficient | Physics | Khan Academy

Carnot cycle and Carnot engine | Thermodynamics | Physics | Khan Academy

Engines: Crash Course Physics #24

Carnot Engine

24. The Second Law of Thermodynamics (cont.) and Entropy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Engine EfficiencyCarnot CycleThermodynamicsHeat EnginesWork TransferTemperature ReservoirsEnergy ConversionIsothermal ProcessIdeal Gas LawEfficiency Calculation