Ideal gas equation example 4 | Chemistry | Khan Academy
TLDRIn this educational video, the presenter guides viewers through a chemistry problem involving the ideal gas equation, PV=nRT. Using 98 milliliters of an unknown gas with a mass of 0.081 grams, the presenter calculates the molar mass to be 18.5 grams per mole. By comparing this to known molar masses, the mystery substance is deduced to be water, H2O, highlighting the importance of understanding the ideal gas law and molar mass in chemistry.
Takeaways
- 📚 The script discusses the importance of understanding the ideal gas equation, PV = nRT.
- 🔍 It presents a problem involving 98 milliliters of an unknown gas with a mass of 0.081 grams.
- 📏 The script clarifies the difference between mass and weight, emphasizing that mass is measured in grams, not newtons.
- 🧪 The task is to calculate the molar mass of the gas, which is the mass per mole.
- 🌡️ Standard temperature and pressure are defined as 273 degrees Kelvin and 1 atmosphere, respectively.
- 📐 The volume of 1 mole of an ideal gas at standard temperature and pressure is 22.4 liters, a value useful for quick chemistry calculations.
- ⚖️ The script uses a proportional equation to find the number of moles of the gas based on its volume.
- 🔢 It calculates the number of moles (x) as 0.004375 by setting up the equation 22.4x = 0.098 liters.
- ✂️ The molar mass is determined by dividing the mass of the gas (0.081 grams) by the number of moles (0.004375), resulting in 18.5 grams per mole.
- 💧 The molar mass suggests that the mystery gas could be water (H2O), with a molar mass of approximately 18 grams per mole.
- 🧠 The script encourages memorizing the volume of 1 mole of an ideal gas for quick problem-solving in chemistry.
Q & A
What is the ideal gas equation mentioned in the script?
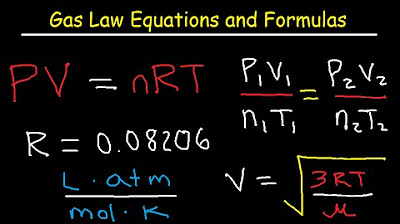
-The ideal gas equation mentioned in the script is PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin.
What is the difference between mass and weight as described in the script?
-In the script, mass is defined as the amount of matter in an object, measured in grams, while weight is the force exerted by gravity on that mass and is measured in newtons in the metric system.
What is the standard temperature and pressure (STP) in the context of the script?
-The standard temperature and pressure (STP) mentioned in the script are 273 degrees Kelvin for temperature and 1 atmosphere for pressure.
How is the molar volume of an ideal gas at STP related to the number of moles?
-At STP, one mole of an ideal gas occupies a volume of 22.4 liters. This relationship is used to calculate the number of moles of a gas given its volume.
What is the molar mass and how is it calculated in the script?
-The molar mass is the mass of one mole of a substance. In the script, it is calculated by dividing the mass of the gas (0.081 grams) by the number of moles (0.004375 moles), resulting in a molar mass of 18.5 grams per mole.
How many moles of gas are in 98 milliliters at STP according to the script?
-The script calculates the number of moles by setting up a proportional equation: 1 mole corresponds to 22.4 liters, so x moles correspond to 0.098 liters (98 milliliters). Solving for x gives 0.004375 moles.
What is the significance of memorizing the molar volume of an ideal gas at STP?
-Memorizing the molar volume of an ideal gas at STP (22.4 liters per mole) is suggested as a handy shortcut for quickly solving problems related to the amount of gas present under these conditions.
What is the relationship between the volume of a gas and the number of moles, as explained in the script?
-The script explains that at STP, the volume of a gas is directly proportional to the number of moles. This relationship is used to find the number of moles when the volume is known.
What method does the script use to determine the molar mass of the unknown gas?
-The script uses the mass of the gas (0.081 grams) and the calculated number of moles (0.004375 moles) to determine the molar mass by dividing the mass by the number of moles.
What is the guessed identity of the mystery substance based on its molar mass in the script?
-Based on the calculated molar mass of 18.5 grams per mole, the script suggests that the mystery substance is likely water (H2O), as the molar mass of water closely matches this value.
How does the script relate the molar mass of hydrogen and oxygen to the molar mass of water?
-The script adds the molar mass of two hydrogen atoms (2 grams) to the molar mass of one oxygen atom (16 grams) to get a total of 18 grams per mole, which is consistent with the molar mass of water.
Outlines
🔍 Calculating Molar Mass of a Gas
The video script begins with an introduction to the importance of understanding the ideal gas equation, PV = nRT, and its application in calculating the molar mass of a gas. The example involves a 98 milliliter sample of an unknown gas with a mass of 0.081 grams. The speaker emphasizes the distinction between mass and weight, clarifying that mass is measured in grams and weight in newtons under standard conditions. The process involves using the volume of the gas at standard temperature and pressure (273 K and 1 atm) and the known volume of one mole of an ideal gas (22.4 liters) to determine the number of moles in the sample. The calculation leads to the discovery that the molar mass of the gas is 18.5 grams per mole, suggesting that the gas might be water (H2O), given its molar mass matches the sum of the atomic masses of two hydrogen atoms (2 grams) and one oxygen atom (16 grams).
Mindmap
Keywords
💡PV=nRT
💡Molar Mass
💡Standard Temperature and Pressure (STP)
💡Moles
💡Volume
💡Mass
💡Ideal Gas
💡Hydrogen
💡Oxygen
💡Mystery Substance
💡Water (H2O)
Highlights
The importance of understanding and applying the ideal gas equation PV=nRT is emphasized for comfort with gas laws.
A problem involving 98 milliliters of an unknown gas with a mass of 0.081 grams is presented.
Clarification on the distinction between mass and weight, especially in the context of the metric system.
The task of calculating the molar mass of the gas is introduced.
Explanation of how to use the ideal gas equation to find the number of moles.
Introduction of standard temperature and pressure values: 273 K and 1 atm.
Mnemonic for remembering the volume occupied by one mole of an ideal gas at STP: 22.4 liters.
Derivation of the relationship between moles and volume for an ideal gas at STP.
Setting up a proportional equation to find the number of moles based on volume.
Calculation of moles for the given volume of 0.098 liters, resulting in 0.004375 moles.
Determination of the molar mass by dividing the mass of the gas by the number of moles.
Performing the calculation 0.081 grams divided by 0.004375 moles to find the molar mass.
Conclusion that the molar mass of the mystery gas is approximately 18.5 grams per mole.
Speculation on the identity of the gas based on its molar mass, suggesting water (H2O) as a candidate.
Explanation of the molar mass calculation for water based on the atomic masses of hydrogen and oxygen.
Final identification of the mystery substance as likely being water based on the calculated molar mass.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: