Conservative Fields & Path Independence (Vector Fields)

Houston Math Prep

7 May 202011:49

EducationalLearning

32 Likes 10 Comments

TLDRThis Houston Math Prep video delves into the concept of gradient fields and their potential functions, illustrating how they can simplify line integrals. It explains that gradient fields, synonymous with conservative fields, are path-independent, meaning the work done by the field along a path is invariant to the path's shape. The video demonstrates how to find a potential function for a given vector field and use it to calculate work along a path or around a closed curve, highlighting the fundamental theorem of line integrals as a powerful tool for these calculations. It concludes with examples, including work along a segment of the unit circle, emphasizing the efficiency of these methods over traditional line integral computation.

Takeaways

📚 The video discusses the concept of a gradient field and its relation to potential functions, which can be used to simplify line integrals.
🔍 A gradient field is synonymous with a conservative field, indicating that the field represents a system where energy is conserved.
📈 The script explains that in a conservative field, the line integral is path-independent, meaning the work done is the same regardless of the path taken between two points.
🔑 The fundamental theorem of line integrals is introduced as a shortcut to calculate work in a gradient field without parameterizing the curve.
📝 To apply the shortcut, one must find the potential function associated with the gradient field and then simply subtract the function's values at the start and end points.
🔄 The video provides an example where the vector field \( F = (y, x) \) is shown to be a gradient field and its potential function is \( f(x, y) = xy \).
🔍 The script demonstrates how to calculate work along a path in a gradient field by plugging endpoints into the potential function, avoiding complex integral calculations.
⭕ When the curve is a simple closed curve in a gradient field, the work done is zero, which is a significant shortcut for calculating line integrals.
📐 The video gives an example of calculating work along a segment of the unit circle using the potential function, resulting in a simplified calculation.
🛑 If the vector field is not a gradient field, traditional methods of parameterizing the curve and calculating line integrals are necessary.
🌐 The script concludes with a mention of Green's theorem as a shortcut for closed curves, which will be covered in a subsequent video.

Q & A

What is the difference between a gradient field and a conservative field?
-There is no difference; a gradient field and a conservative field are synonymous. Both terms refer to a vector field that can be expressed as the gradient of some scalar function, indicating that the field has potential energy properties.
Why is a conservative field also called a gradient field?
-A conservative field is called a gradient field because it represents a system where energy is conserved, and mathematically, it can be expressed as the gradient of a scalar potential function.
What does it mean for a field to be simply connected?
-A simply connected region is one that does not have any holes or isolated points that cannot be encircled without leaving the region. It is a necessary condition for certain mathematical operations, including the application of the fundamental theorem of line integrals for conservative fields.
What is the fundamental theorem of line integrals and how does it simplify calculations in a gradient field?
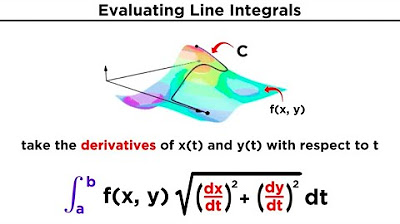
-The fundamental theorem of line integrals allows for the simplification of line integrals in a gradient field by eliminating the need to parameterize the curve. Instead of integrating over the curve, one can simply evaluate the potential function at the endpoints and subtract.
How can you determine if a vector field is a gradient field?
-A vector field is a gradient field if the partial derivative of its component M with respect to y is equal to the partial derivative of its component N with respect to x, ensuring path independence.
What is the significance of a path-independent vector field?
-In a path-independent vector field, the work done or the line integral taken from one point to another is the same regardless of the path taken. This property is crucial for applying the fundamental theorem of line integrals and simplifying calculations.
Why is the work done in a conservative field along a closed curve always zero?
-In a conservative field, the work done along a closed curve is zero because the potential function, which is used to calculate the work, yields the same value at the starting and ending points of the curve, resulting in a net work of zero when subtracted.
Can you provide an example of a potential function for the vector field F = (y, x)?
-Yes, the potential function for the vector field F = (y, x) can be found by integrating each component with respect to its corresponding variable. The potential function is f(x, y) = xy + C, where C is a constant.
How does the fundamental theorem of line integrals apply to a line integral over a portion of the unit circle?
-For a line integral over a portion of the unit circle in a gradient field, the fundamental theorem of line integrals allows you to find the work done by plugging the endpoints of the curve into the potential function and subtracting the results, without needing to parameterize the curve or perform the integral directly.
What is the result of the line integral of F = (y, x) over a quarter of the unit circle from θ=0 to θ=π/4?
-The result of the line integral of F = (y, x) over this path is 1/2. This is found by evaluating the potential function at the endpoints (1,0) and (√2/2, √2/2) and taking the difference.
What is the next topic to be covered after the discussion of gradient fields and the fundamental theorem of line integrals?
-The next topic to be covered is Green's theorem, which provides a shortcut for calculating line integrals over closed curves when the vector field is not a gradient field.

Outlines

00:00

📚 Understanding Gradient Fields and Conservative Vector Fields

This paragraph introduces the concept of gradient fields and conservative vector fields, explaining that they are synonymous. It emphasizes the importance of understanding partial derivatives and the requirement for the field to be defined on a simply connected region. The paragraph clarifies that a conservative field represents a system where energy is conserved, allowing for the assignment of potential functions independent of the path taken between two points. The fundamental theorem of line integrals is introduced as a method to simplify calculations by eliminating the need to parameterize curves, instead using the potential function and its values at endpoints to find work done by the field.

05:03

🔍 Applying Path Independence in Gradient Fields

The second paragraph delves into the application of path independence in gradient fields, illustrating how to calculate work done by a vector field along a curve without the need for complex integrals. It provides an example using the vector field F = <y, x>, showing how to find the potential function and use it to calculate work from one point to another. The paragraph also discusses the implications of a closed curve in a gradient field, where the work done is always zero due to the nature of path independence, offering a shortcut for such cases. Two examples are given: one with a full unit circle resulting in zero work, and another with a quarter of the unit circle where the work is calculated using the potential function and the endpoints.

10:05

🛠 Efficient Methods for Calculating Line Integrals in Gradient Fields

The final paragraph outlines a general approach for efficiently calculating line integrals in gradient fields. It suggests a step-by-step process beginning with checking if the vector field is a gradient field and if the path is a simple closed curve, which simplifies the calculation to zero. For non-closed paths, the fundamental theorem of line integrals is used by finding the potential function and subtracting the function values at the start and end points. The paragraph concludes by noting that if the vector field is not a gradient field, traditional parameterization and line integral methods must be used, with a teaser for the next video on Green's theorem for closed curves.

Mindmap

Keywords

💡Gradient Field

A gradient field is a vector field \( \mathbf{F} \) that can be expressed as the gradient of some scalar function \( f \), denoted as \( \mathbf{F} = \nabla f \). In the context of the video, a gradient field is crucial for simplifying line integrals, as it implies the field is conservative. The script uses the example of a field \( \mathbf{F} = (y, x) \) to illustrate how to determine if a field is a gradient field by checking if the partial derivatives of its components are equal.

💡Conservative Field

A conservative field is synonymous with a gradient field. It is a vector field where the work done by the field on a particle moving between two points is independent of the path taken. The video explains that if a field is conservative, it represents a system where energy is conserved, and the potential function can be used to calculate work without needing to parameterize the path.

💡Path Independence

Path independence refers to the property of a conservative field where the work done by the field on a particle moving between two points does not depend on the specific path taken. The video emphasizes this concept by stating that in a path-independent field, the line integral can be calculated by simply finding the difference in potential values at the endpoints, regardless of the path taken.

💡Potential Function

A potential function is a scalar function \( f \) associated with a conservative vector field, such that the field can be expressed as its gradient. The video demonstrates the process of finding a potential function by integrating the components of the field with respect to their respective variables, and it shows how this function is used to calculate work in a path-independent field.

💡Line Integral

A line integral is a mathematical concept that allows for the calculation of quantities along a curve, such as the work done by a vector field. The video explains how in the case of a gradient field, line integrals can be simplified by using the potential function and the fundamental theorem of line integrals, avoiding the need for parameterization.

💡Fundamental Theorem of Line Integrals

The fundamental theorem of line integrals is a key concept that allows for the simplification of line integrals in gradient fields. The video illustrates that instead of parameterizing the curve and integrating, one can directly calculate the line integral by evaluating the potential function at the endpoints of the path and subtracting.

💡Parameterization

Parameterization is the process of defining a curve by a vector-valued function \( \mathbf{r}(t) \), which is then used to express the components of a vector field in terms of the parameters. The video mentions that in non-gradient fields, one must parameterize the curve to calculate line integrals, which is a more complex process than necessary for gradient fields.

💡Partial Derivatives

Partial derivatives are used to find the rate of change of a function with respect to one variable while keeping the other variables constant. In the context of the video, partial derivatives are essential for determining if a field is a gradient field by comparing the partial derivatives of the field's components with respect to different variables.

💡Simply Connected Region

A simply connected region is a type of region in space that does not contain any holes or punctures. The video mentions this concept to indicate that for the shortcuts involving gradient fields to apply, the field must be defined on a simply connected region, ensuring that there are no complications from the topology of the region.

💡Closed Curve

A closed curve is a curve that starts and ends at the same point, forming a loop. The video explains that for a gradient field, the line integral over a closed curve is always zero, providing a shortcut for calculating work in such cases without needing to find the potential function or endpoints.

💡Green's Theorem

Green's Theorem is a mathematical result that relates a line integral around a simple closed curve to a double integral over the plane region enclosed by the curve. The video hints at this theorem as a shortcut for line integrals in closed curves, which will be covered in a subsequent video.

Highlights

Introduction to the concept of gradient fields and their application in simplifying line integrals.

Explanation of the equivalence between gradient fields and conservative fields.

Assumption of basic knowledge of partial derivatives and simply connected regions for using gradient field shortcuts.

Definition of a conservative field as a system where energy is conserved.

The potential function as a means to assign potential independently of the path taken.

Fundamental theorem of line integrals allows for shortcutting line integrals in gradient fields.

Demonstration of how to calculate work in a gradient field without parameterizing the curve.

Example of calculating work in the vector field F = (y, x) from (0,0) to (1,1) using the potential function.

Shortcut for calculating work in a gradient field with a closed curve, where the work is zero.

Explanation of the shortcut for simple closed curves in gradient fields, avoiding line integral computation.

Use of the potential function to calculate work along a segment of the unit circle in a gradient field.

Application of the fundamental theorem of line integrals to find the work along a non-closed curve.

General outline for efficiently finding the integral over a curve of F dot dR in gradient fields.

Process of checking if the vector field is a gradient field for potential shortcuts.

Approach for non-gradient fields, including parameterization and line integral computation.

Introduction to Green's theorem as a shortcut for closed curves, to be covered in the next video.

Conclusion and summary of the video's content on gradient fields and line integrals.

Transcripts

Browse More Related Video

Lec 20: Path independence and conservative fields | MIT 18.02 Multivariable Calculus, Fall 2007

Closed curve line integrals of conservative vector fields | Multivariable Calculus | Khan Academy

Line Integrals of Vector Fields (Introduction)

Lec 19: Vector fields and line integrals in the plane | MIT 18.02 Multivariable Calculus, Fall 2007

Conservative Vector Fields & Potential Functions

Evaluating Line Integrals

Conservative Fields & Path Independence (Vector Fields)

Takeaways

Q & A

What is the difference between a gradient field and a conservative field?

Why is a conservative field also called a gradient field?

What does it mean for a field to be simply connected?

What is the fundamental theorem of line integrals and how does it simplify calculations in a gradient field?

How can you determine if a vector field is a gradient field?

What is the significance of a path-independent vector field?

Why is the work done in a conservative field along a closed curve always zero?

Can you provide an example of a potential function for the vector field F = (y, x)?

How does the fundamental theorem of line integrals apply to a line integral over a portion of the unit circle?

What is the result of the line integral of F = (y, x) over a quarter of the unit circle from θ=0 to θ=π/4?

What is the next topic to be covered after the discussion of gradient fields and the fundamental theorem of line integrals?