Finding Area Using Double Integrals (Rectangular & General Regions)
TLDRThis video tutorial guides viewers through the process of finding the area of a region using double integrals. It explains the concept of breaking down a region into smaller rectangular boxes to approximate the area, and then refining this approximation by considering the limits of integration in two different orders: dx dy and dy dx. The presenter illustrates how to choose the appropriate order based on the shape of the region, using several examples including rectangular areas and regions bounded by curves. The video concludes with a method to determine whether a region is 'X simple' or 'Y simple' to decide the best order for integration.
Takeaways
- 📚 The video is a tutorial on finding the area of a region using double integrals.
- 🔍 It introduces the concept of approximating a region with a grid-like structure of rectangular boxes to estimate the area.
- 📏 The script explains that as the size of the boxes (Delta x and Delta y) decreases, the approximation becomes more accurate, eventually leading to the use of double integrals.
- 🧩 The area of each small rectangular piece is found by multiplying its width and height (Delta x times Delta y).
- 🔄 The video discusses the importance of choosing the order of integration (dx dy or dy dx) based on the shape of the region for easier computation.
- 📈 An example of a rectangular region is used to demonstrate the setup of double integrals in both orders, showing that both yield the same area.
- 📉 The script covers a non-rectangular region defined by x between 0 and 4, and y between 0 and the square root of x, illustrating a more complex setup for double integrals.
- ⚖️ It emphasizes that the choice between dx dy and dy dx should be based on whether the region is 'x simple' or 'y simple', meaning which direction simplifies the integration bounds.
- 📐 The tutorial provides a method to determine if a region is x simple or y simple by testing if the entry and exit points of the region are consistent when drawing lines in one direction.
- 📝 The video concludes with a summary that the setup of double integrals should consider the simplicity in either the x or y direction for efficient computation of the area.
- 👋 The presenter thanks viewers for watching and indicates that further lessons will be provided in subsequent videos.
Q & A
What is the main topic of the video?
-The main topic of the video is finding the area of a region using double integrals.
Why might one choose to use double integrals to find the area of a region?
-Double integrals are used to find the area of a region when the region cannot be easily described by simple geometric shapes or when the region has a complex boundary.
What is the purpose of breaking a region into a grid-like structure when using double integrals?
-Breaking a region into a grid-like structure helps to approximate the area by summing the areas of small rectangular pieces, which can be more easily calculated.
How does the approximation of area using small rectangular boxes relate to the concept of a double integral?
-The approximation using small rectangular boxes is a discrete version of the continuous process of a double integral, where as the size of the boxes approaches zero, the sum of their areas approaches the exact area under the curve defined by the double integral.
What is the significance of choosing the order of integration (dx dy or dy dx) in a double integral?
-The order of integration is significant because it determines the bounds of integration and can affect the complexity of the integral. It should be chosen based on the shape of the region and which variable's bounds are simpler to define.
How does the shape of the region R influence the decision to integrate in the order of dx dy or dy dx?
-The shape of region R influences the decision by indicating which variable's bounds are easier to define. If the region is simpler to describe in terms of one variable changing while the other remains constant, that variable should be integrated first.
What is an example of a region where a double integral is an overkill but is used for demonstration purposes in the video?
-An example given in the video is a rectangular region where x is between 1 & 6 and y is between 2 & 5. While the area of a rectangle can be easily calculated, a double integral is used to demonstrate the method.
What is the process of setting up a double integral for a region bounded by a function and a constant?
-The process involves fixing a value of one variable, drawing through the region in the direction of the other variable to find the bounds, and then integrating with respect to the variable with bounds that are functions or constants.
How does the concept of 'X-simple' or 'Y-simple' regions relate to choosing the order of integration?
-A region is 'X-simple' if the rule for entering and exiting the region when drawing through in the x-direction is consistent regardless of the x-value chosen. Similarly, it is 'Y-simple' if this consistency holds when drawing through in the y-direction. Choosing the order that matches the 'simple' variable can simplify the integration process.
What is the final step in evaluating a double integral to find the area of a region?
-The final step is to evaluate the innermost integral, which will often result in a function of one variable, and then integrate this function with respect to the outer variable within its bounds.
Outlines
📚 Introduction to Finding Area with Double Integrals
The script begins with an introduction to calculating the area of a region using double integrals. The concept of breaking down the region into infinitesimal rectangular boxes to approximate the area is explained. The importance of choosing the correct order of integration, either dx dy or dy dx, based on the shape of the region is highlighted. The process involves considering the limits of integration that correspond to the boundaries of the region, and the script sets the stage for examples that will illustrate the method.
📐 Setting Up Double Integrals for Rectangular Regions
This paragraph delves into the mechanics of setting up double integrals for a simple rectangular region defined by x between 1 and 6, and y between 2 and 5. Two methods of integration order are explored: first integrating with respect to y (dy/dx) and then with respect to x (dx dy). The process involves identifying the correct bounds for integration based on the region's limits. The paragraph demonstrates that both orders yield the same result, 15 square units, for this straightforward example.
🔍 Integrating Non-Rectangular Regions with Double Integrals
The script moves on to more complex regions that are not simple rectangles, such as a region bounded by x between 0 and 4 and y between 0 and the square root of x. The process of setting up the double integral is explained, with a focus on identifying the correct bounds for integration, which in this case involves a variable upper bound for y. The paragraph illustrates the calculation for the area under the curve, demonstrating how to integrate when one of the bounds is a function of the variable of integration.
📉 Double Integrals for Regions with Curved Boundaries
The paragraph discusses how to approach regions with curved boundaries, using the example of a region where x ranges from 0 to 9 and y ranges from the square root of x + 3. It explains the method of setting up the double integral, emphasizing the importance of identifying the correct bounds for both x and y, which may involve solving equations to find intersection points. The paragraph concludes with the calculation of the area for this region.
🔄 Choosing the Order of Integration for Double Integrals
This section of the script addresses the strategy for choosing the order of integration (dx dy or dy dx) based on the simplicity of the region in the x or y direction. It introduces the concepts of 'x-simple' and 'y-simple' regions and provides examples to illustrate how to determine which order of integration is more straightforward. The paragraph emphasizes the importance of consistency in the rules for entering and exiting the region when drawing lines in the chosen direction of integration.
📘 Conclusion on Setting Up Double Integrals
The final paragraph wraps up the script by summarizing the process of setting up double integrals and choosing the appropriate order of integration. It reiterates the importance of analyzing the shape of the region and the simplicity of the integration process in either the x or y direction. The script concludes with a reminder that the choice between dx dy and dy dx can greatly simplify the calculation of the area of a given region.
Mindmap
Keywords
💡Double Integrals
💡Region R
💡Rectangular Boxes
💡Infinitesimal Area
💡Order of Integration
💡Bounds
💡X-simple and Y-simple
💡Area Approximation
💡Integration Bounds
💡Power Rule
Highlights
Introduction to finding the area of a region using double integrals.
Explanation of approximating area with a grid-like structure of rectangular boxes.
Clarification on the concept of Delta a representing the area of a small box in the grid.
The process of refining the approximation by making the boxes smaller.
The transition from Delta x times Delta Y to infinitesimal area elements dx dy.
The importance of choosing the order of integration based on the region's shape.
Demonstration of setting up double integrals for a rectangular region.
Illustration of integrating in both dx dy and dy dx orders for a rectangle.
Verification that both integration orders yield the same area for a rectangle.
Application of double integrals to a region defined by a function and a constant.
Integration strategy for regions with variable boundaries using dy dx order.
Calculation of area for a region with a parabola as the boundary.
Differentiation between X-simple and Y-simple regions for integral setup.
Decision-making process for choosing the order of integration based on simplicity.
Final examples demonstrating the setup of double integrals for both X-simple and Y-simple regions.
Conclusion summarizing the method for setting up double integrals effectively.
Transcripts
Browse More Related Video

Finding Volumes Using Double Integrals| Calculus 3 | Math with Professor V
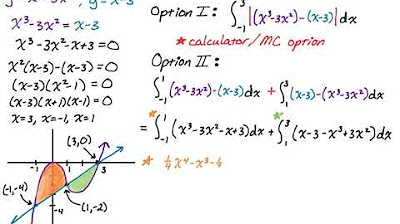
Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

AP Calculus BC Lesson 9.8
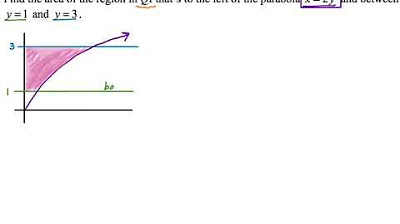
Area Between Curves: Integrating with Respect to y (Example 1)

2022 AP Calculus BC Exam FRQ #5

Area Between Curves: Integrating with Respect to y (Example 2)
5.0 / 5 (0 votes)
Thanks for rating: