Area Between Curves: Integrating with Respect to y (Example 2)
TLDRIn this video, the presenter uses calculus to determine the area between three curves: y = x^3, y = -x, and y = 1. The video begins by identifying the intersection points of these curves, which are essential for defining the region of interest. The presenter then sketches the region, which is bounded by the curves and the x-axis. To calculate the area, the video explains the need to express the right-hand curve (y = x^3) and the left-hand curve (y = -x) as functions of y, resulting in x = y^(1/3) and x = -y, respectively. The integral is set up with y-values as bounds and the functions as x in terms of y. The final integral is calculated from y = 0 to y = 1, integrating the difference between the right and left functions. By applying the power rule in reverse, the presenter finds the area to be 5/4, demonstrating a clear and efficient method for finding the area between curves using integration with respect to y.
Takeaways
- 📐 The video demonstrates how to use calculus to find the area between curves by integrating with respect to y.
- 🔍 The problem involves finding the area between y = x^3, y = -x, and y = 1, which define a region in the plane.
- 🤔 The presenter is confident in visualizing y = x^3 and y = -x, and understands y = 1 is a horizontal line.
- 📈 Intersection points are crucial: (1,1) for y = x^3 and y = 1, (-1,1) for y = -x and y = 1, and the origin (0,0) for y = x^3 and y = -x.
- 🖋️ The region of interest is bounded by y = 1 (top), y = -x (left), y = x^3 (right), and the x-axis (bottom).
- 🟡 The region to be shaded for area calculation does not include parts below the x-axis due to the intersection at the origin.
- 🧮 To set up the integral, the functions y = x^3 and y = -x are rearranged to express x as a function of y: x = y^(1/3) and x = -y, respectively.
- 📉 The lower bound of integration is 0 (y-coordinate of the origin), and the upper bound is 1 (defined by y = 1).
- ✍️ The integral to calculate the area is written as ∫ from 0 to 1 of (y^(1/3) - (-y)) dy.
- 🧑🔬 The integral is simplified using the power rule in reverse, resulting in (3/4)y^(4/3) - (1/2)y^2 evaluated from 0 to 1.
- 🔢 Plugging in the bounds, the area is found by evaluating (3/4)(1)^(4/3) - (1/2)(1)^2, which simplifies to 5/4.
- 🎓 The presenter suggests that integrating with respect to y was more straightforward for this region, as opposed to x, which would have required separate integrals.
Q & A
What is the main topic of the video?
-The video is about using calculus to find the area between curves by integrating with respect to Y.
What are the three curves given in the problem?
-The three curves are y = x^3, y = -x, and y = 1.
How does the video determine the intersection points of the curves?
-The video finds the intersection points by setting y = 1 and solving for x in both x^3 and -x, which gives x = 1 and x = -1, respectively. It also notes that x^3 and -x intersect at the origin (0,0).
What is the region defined by these curves?
-The region is bounded by y = x^3 on the right, y = -x on the left, and y = 1 on the top, with the x-axis as the bottom since the curves intersect at the origin.
How does the video approach the integration with respect to Y?
-The video first sketches the region and then determines the bounds for integration. It rewrites the right and left curves as functions of Y (x = y^(1/3) and x = -y) and integrates from the lower bound 0 to the upper bound 1.
What are the bounds for the integral with respect to Y?
-The lower bound is 0 (the y-coordinate of the origin), and the upper bound is 1 (where y = 1 intersects with x^3 and -x).
How does the video rewrite the integral to make it easier to solve?
-The video uses the reverse power rule to rewrite the integral as (3/4)y^(4/3) - (1/2)y^2 and then integrates this expression from 0 to 1.
What is the final result for the area of the region?
-The area of the region is calculated to be 5/4.
Why might integrating with respect to X be more complicated in this case?
-Integrating with respect to X would require two separate integrals or the calculation of a triangle plus an integral, making it less straightforward than integrating with respect to Y.
What is the significance of the origin in defining the bottom of the region?
-The origin is significant because it is the point where the left and right curves intersect, thus defining the bottom boundary of the region under consideration.
Why does the video mention that the part of the region below the x-axis is not considered?
-The part below the x-axis is not considered because the intersection at the origin (0,0) effectively bounds the region of interest above the x-axis.
What does the video suggest about the choice of variable for integration?
-The video suggests that choosing the variable for integration (Y in this case) that simplifies the calculation can make the problem easier to solve.
Outlines
📐 Calculus and Area Between Curves
The video begins with an introduction to using calculus to calculate the area between curves. The presenter discusses the integration process with respect to Y. The problem at hand is to find the area of the region bounded by y = x^3, y = -x, and y = 1. The presenter outlines the need to understand the shape of each curve and how they intersect to define a region in the plane. The intersection points of y = 1 with y = x^3 and y = -x are determined to be (1, 1), (-1, 1), and the origin (0, 0) for y = x^3 and y = -x. The region to be shaded is then sketched, bounded by the three curves. The presenter proceeds to define the integral's limits and functions, transforming y = x^3 into x = y^(1/3) and y = -x into x = -y to facilitate integration with respect to Y. The integral to calculate the area is set up from 0 to 1, subtracting the left function (-y) from the right function (y^(1/3)). The integration is performed, applying the power rule in reverse, which results in the area of the region being 5/4.
Mindmap
Keywords
💡Calculus
💡Area Between Curves
💡Integration
💡Intersection Points
💡Graphing
💡Region
💡Bounds
💡Function of Y
💡Power Rule
💡Integral Setup
💡Definite Integral
Highlights
The video demonstrates using calculus to find the area between curves.
Integration is performed with respect to Y.
Three curves are given: y=x^3, y=-x, and y=1, which define a region in the plane.
The intersection points of the curves are found to be (1,1), (-1,1), and the origin (0,0).
The region of interest is shaded for clarity, bounded by the three curves.
The bottom of the region is the origin, as the left and right curves intersect at (0,0).
The integral to find the area is set up with the bounds from 0 to 1 on the Y-axis.
The right curve y=x^3 is rewritten as x=y^(1/3) to express x as a function of y.
The left curve y=-x is rewritten as x=-y to express x in terms of y.
The integral expression is formed as the difference between the right and left functions evaluated from 0 to 1 with respect to Y.
The power rule is applied in reverse to integrate the functions with respect to Y.
The integral results in the area being calculated as 3/4 * Y^(4/3) from 0 to 1.
Evaluating the integral at the upper bound (1) and lower bound (0) simplifies the calculation.
The final area of the region is found to be 5/4 square units.
An alternative method using integration with respect to X would require two separate integrals.
The video concludes by emphasizing the efficiency of using Y for integration in this case.
The presenter wishes the viewers good luck and hopes they found the explanation helpful.
Transcripts
Browse More Related Video
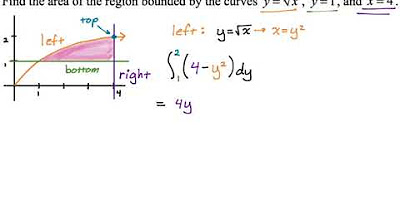
Area Between Curves: Integrating with Respect to y (Example 3)
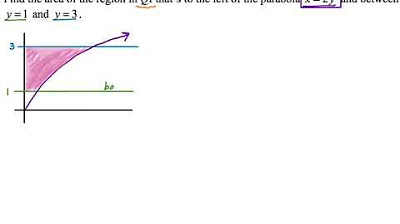
Area Between Curves: Integrating with Respect to y (Example 1)

Area Between y=x^3 and y=3x-2 | MIT 18.01SC Single Variable Calculus, Fall 2010
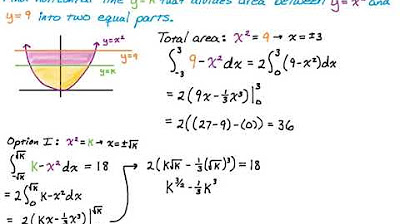
Finding a Horizontal Line (y=k) to Divide a Region into Two Equal Parts Using Calculus
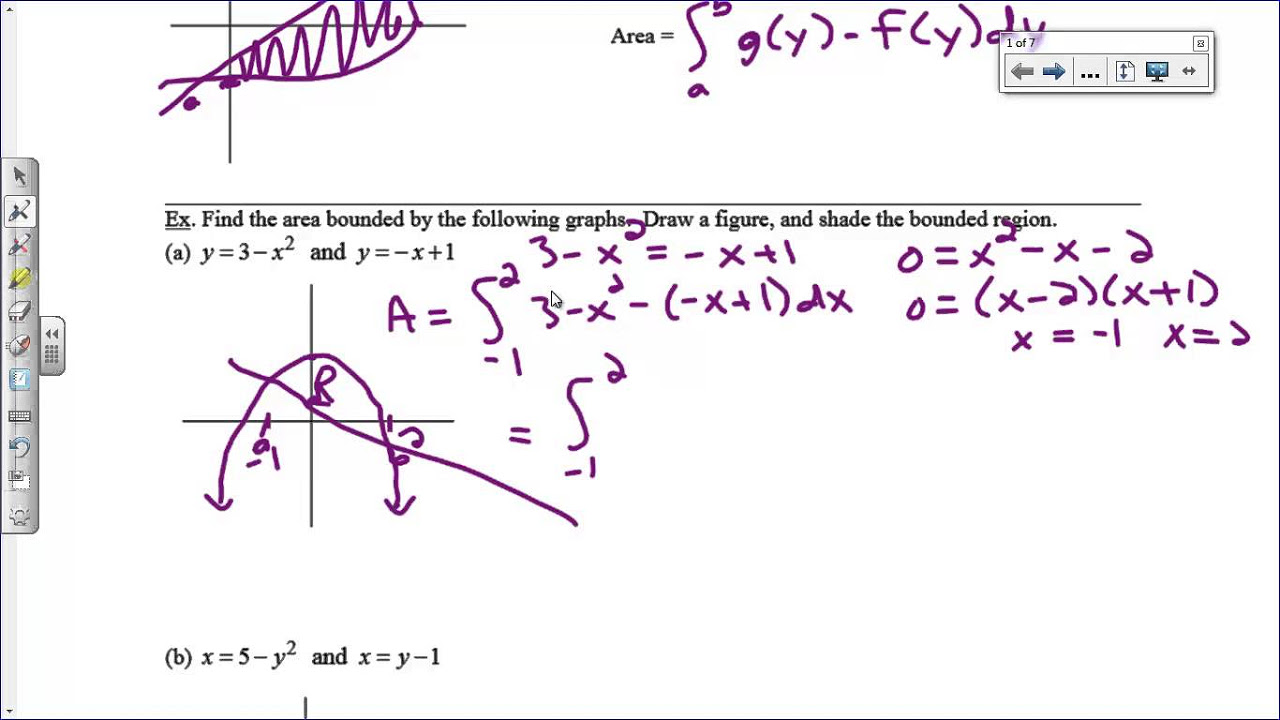
Area of a Region Between Two Curves
Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3
5.0 / 5 (0 votes)
Thanks for rating: