7.2.4 Estimating a Population Mean - Sample Size for a Desired Margin of Error and Confidence Level

Sasha Townsend - Tulsa
30 Oct 202014:43
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script covers the process of determining the necessary sample size to estimate a population mean with a specified degree of accuracy and confidence level. It introduces the formulas for calculating the margin of error when the population standard deviation is known, and then demonstrates how to rearrange these formulas to solve for the sample size. The script also addresses scenarios where the population standard deviation is unknown, suggesting methods such as using the range rule of thumb, calculating a sample standard deviation, or relying on previous studies for estimation. An example is provided to illustrate the calculation for estimating the mean IQ score of attorneys with a 98% confidence level and a margin of error of three points, rounding up to ensure accuracy.

Takeaways
  • πŸ“š The video discusses learning outcome number four of lesson 7.2, focusing on how to determine the necessary sample size to estimate a population mean with a desired degree of accuracy and confidence level.
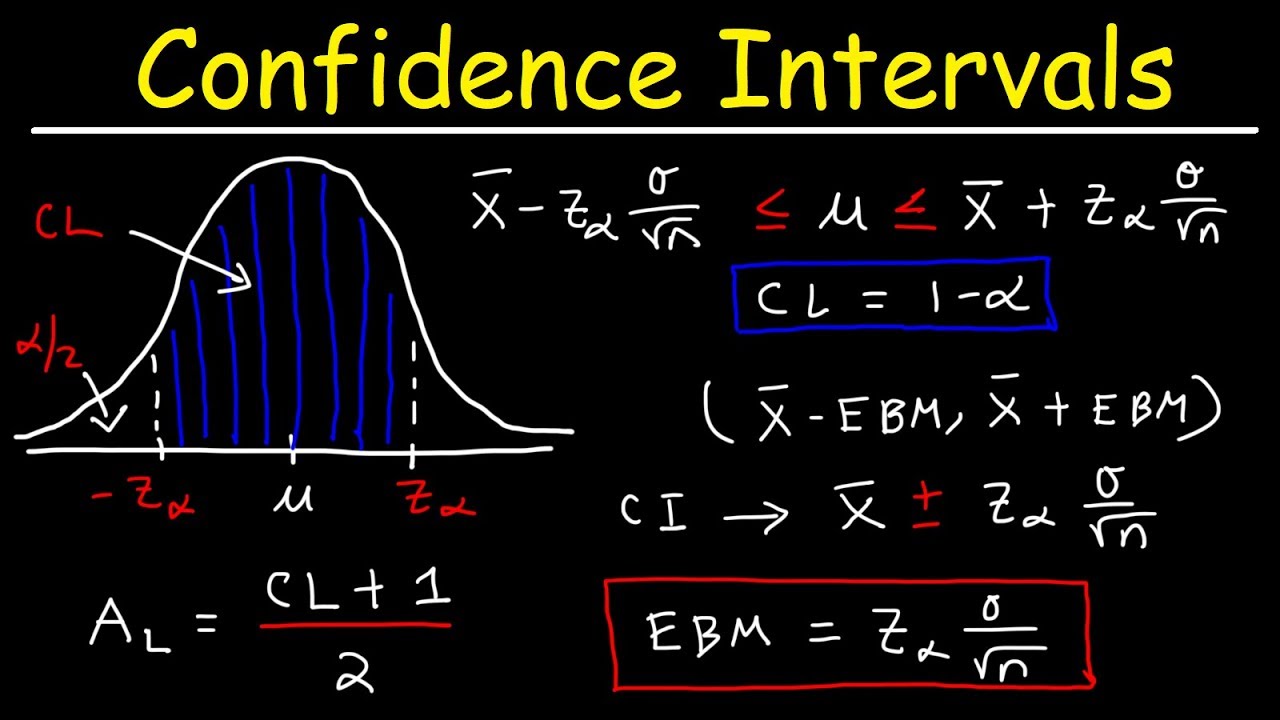
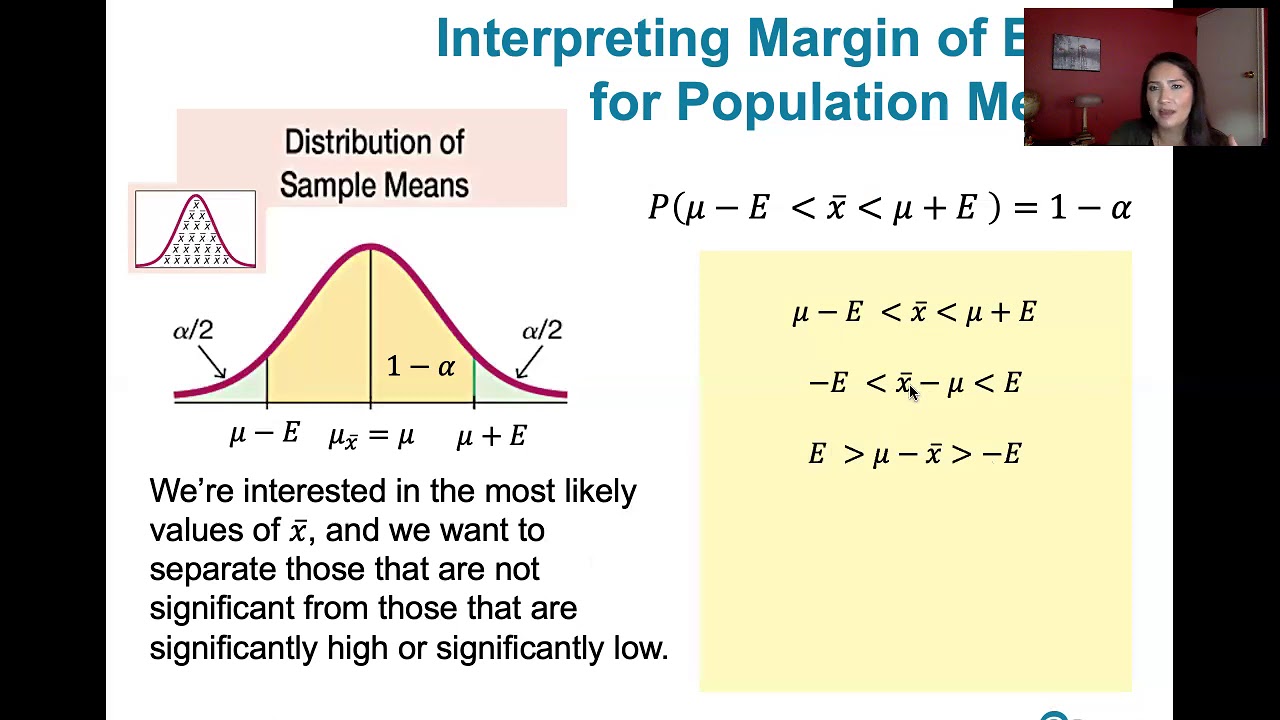
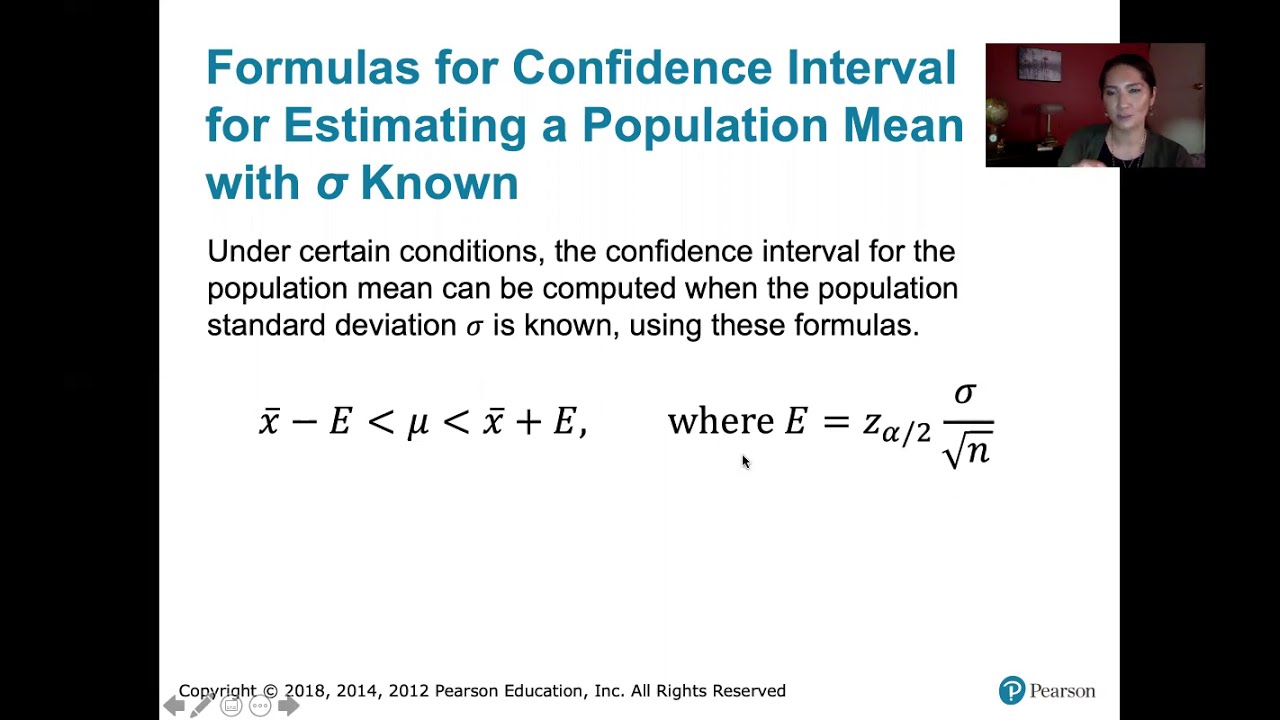
  • πŸ“‰ The script explains the formulas for the confidence interval of a population mean, particularly when the population standard deviation (sigma) is known.
  • πŸ” The margin of error is calculated using the formula involving the z-score (z sub alpha over two), the population standard deviation, and the square root of the sample size.
  • πŸ”’ To find the sample size needed for a given margin of error and confidence level, the formula is rearranged to solve for 'n', the sample size.
  • βš–οΈ The importance of rounding up the calculated sample size to ensure less than the intended margin of error is emphasized.
  • πŸ“ˆ The script reviews the notation for confidence intervals, including symbols for population mean (mu), sample size (n), sample mean (x bar), desired margin of error (e), population standard deviation (sigma), and z-score (z sub alpha over two).
  • πŸ€” The video addresses the common scenario where the population standard deviation is unknown, suggesting methods such as estimating it using the range rule of thumb, computing a sample standard deviation, or using values from previous studies.
  • 🧩 An example is provided to illustrate the process of calculating the required sample size to estimate the mean IQ score of attorneys with a 98% confidence level and a margin of error of three IQ points.
  • πŸ“Š The video demonstrates how to use Excel or a z-table to find the z-score corresponding to a given confidence level, in this case, 98%.
  • πŸ”‘ The final step in the example is to plug the values into the rearranged formula to find the sample size, rounding up to the nearest whole number to ensure accuracy.
  • πŸ”— The script concludes by explaining the confidence interval's significance in estimating population parameters, highlighting that with 98% confidence, the true mean IQ score of attorneys would be within a six-point range based on the sample.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is determining the necessary sample size to estimate a population mean with a desired degree of accuracy and confidence level when the population standard deviation is known.

  • What is the formula for the margin of error when the population standard deviation is known?

    -The margin of error is calculated as \( z_{\alpha/2} \times \frac{\sigma}{\sqrt{n}} \), where \( z_{\alpha/2} \) is the z-score from the standard normal distribution, Οƒ is the population standard deviation, and n is the sample size.

  • How is the sample size formula derived from the margin of error formula?

    -The sample size formula is derived by rearranging the margin of error formula to solve for n, resulting in \( n = \left(\frac{z_{\alpha/2} \times \sigma}{E}\right)^2 \), where E is the desired margin of error.

  • Why should we round up the calculated sample size?

    -We round up the calculated sample size to ensure that the margin of error is not larger than intended. Rounding up reduces the error, which is preferable to having more error than necessary.

  • What is the significance of the z-score in the context of this video?

    -The z-score represents the critical value from the standard normal distribution that corresponds to the desired confidence level and is used to calculate the margin of error and sample size.

  • What is the role of the confidence level in determining the sample size?

    -The confidence level dictates the proportion of the area under the standard normal curve that falls within the confidence interval, which in turn affects the z-score and the required sample size.

  • What are the alternatives if the population standard deviation is unknown?

    -If the population standard deviation is unknown, alternatives include using the range rule of thumb to estimate it, calculating a sample standard deviation from initial data, or using values from previous studies.

  • What is the range rule of thumb for estimating the population standard deviation?

    -The range rule of thumb states that the range (maximum value minus minimum value) divided by four gives a rough estimate of the population standard deviation.

  • How can we find the z-score corresponding to a given confidence level using Excel?

    -In Excel, you can use the function `=NORM.INV(1 - alpha/2)` to find the z-score, where alpha is the significance level corresponding to the (1 - confidence level).

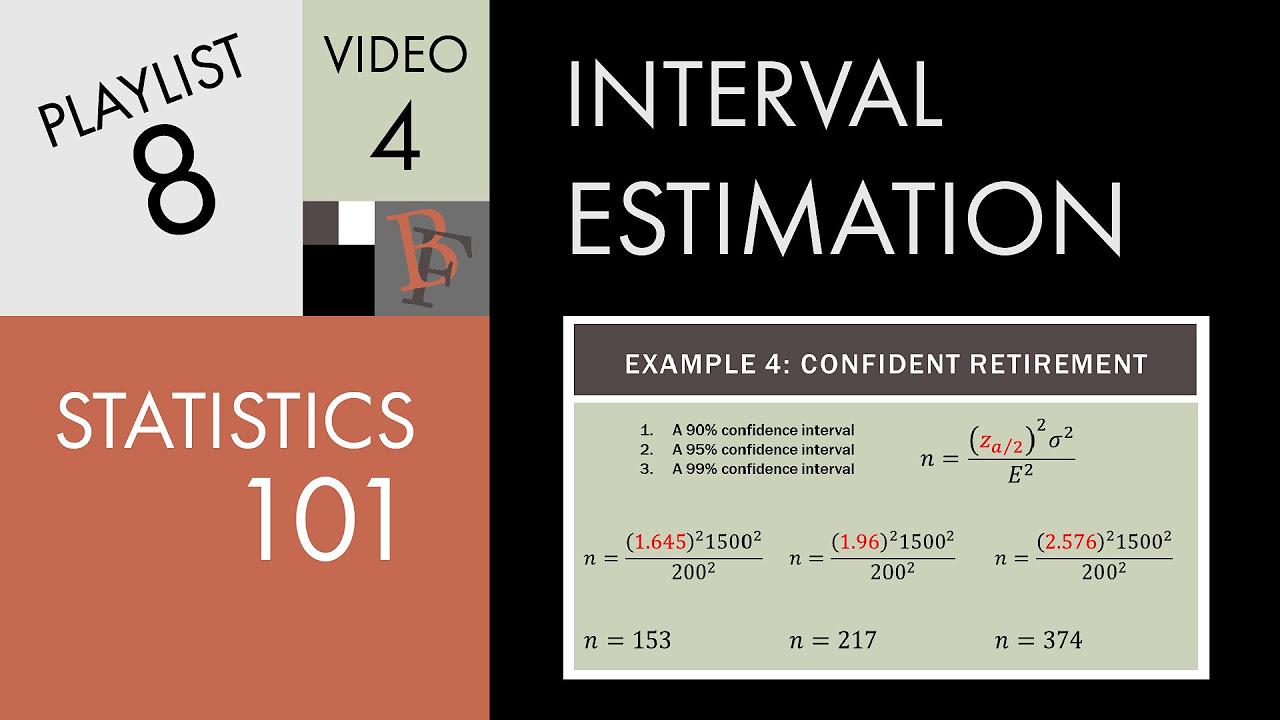
  • Can you provide an example of calculating the required sample size based on the video script?

    -Sure, given a population standard deviation of 15, a desired margin of error of 3 IQ points, and a 98% confidence level, the sample size required would be calculated as \( n = \left(\frac{2.33 \times 15}{3}\right)^2 \), which equals approximately 136, and rounded up to ensure accuracy.

  • What does it mean to have a 98% confidence level in the context of estimating a population mean?

    -A 98% confidence level means that if the sampling process is repeated 100 times, the true population mean would fall within the calculated confidence interval in 98 of those instances.

Outlines
00:00
πŸ“Š Estimating Population Mean with Confidence Intervals

This paragraph introduces the concept of estimating a population mean with a specific degree of accuracy and confidence level. It explains the use of formulas for the confidence interval when the population standard deviation (sigma) is known. The margin of error is calculated using a z-score from the standard normal distribution, the population standard deviation, and the square root of the sample size. The paragraph also discusses how to determine the necessary sample size to achieve a desired margin of error and confidence level by rearranging the error formula and solving for the sample size (n). It emphasizes the importance of rounding up the sample size to ensure the margin of error is not exceeded.

05:01
πŸ” Alternatives When Population Standard Deviation is Unknown

The second paragraph addresses the common scenario where the population standard deviation is not known. It suggests several methods to estimate it, such as using the range rule of thumb (dividing the range by four), calculating a sample standard deviation from initial data collection, or using values from previous studies. The paragraph provides an example of estimating the mean IQ score for attorneys, using a conservative standard deviation of 15, and explains how to calculate the required sample size for a 98% confidence level with a margin of error of three IQ points.

10:01
πŸ“š Calculating the Sample Size with Given Parameters

The final paragraph demonstrates the process of calculating the sample size needed to estimate the mean IQ score of attorneys with a 98% confidence level and a margin of error of three points, using the conservative standard deviation of 15. It involves finding the z-score corresponding to the desired confidence level, plugging in the values into the sample size formula, and rounding up to the nearest whole number. The paragraph concludes with the finding that at least 136 IQ tests are needed to achieve the desired confidence interval, and it explains the implications of this sample size for the confidence level and margin of error.

Mindmap
Keywords
πŸ’‘Sample Size
Sample size refers to the number of observations or data points collected in a study. In the context of the video, it is crucial for estimating a population mean with a desired degree of accuracy and confidence. The script discusses how to calculate the necessary sample size when the population standard deviation is known, which is essential for constructing a confidence interval for the population mean.
πŸ’‘Population Mean
The population mean is the average value of a particular variable for an entire population. The video's main theme revolves around estimating this mean to a certain level of accuracy. The script explains that the sample mean is used as an estimate for the population mean, and the margin of error determines how close this estimate is likely to be to the true population mean.
πŸ’‘Confidence Interval
A confidence interval is a range that is likely to contain the population parameter with a certain level of confidence. The video script describes how to compute the confidence interval for a population mean when the population standard deviation is known, using formulas that incorporate the sample mean, margin of error, and z-scores.
πŸ’‘Margin of Error
The margin of error is the range within which the true population parameter is estimated to lie, with a certain level of confidence. In the script, it is calculated as part of the confidence interval and is determined by the desired level of accuracy and confidence level, as well as the population standard deviation and sample size.
πŸ’‘Z-Score
A z-score represents the number of standard deviations a data point is from the mean in a standard normal distribution. The video explains that z-scores are used to determine the critical value that separates the middle area of the distribution from the tails, which is essential for calculating the margin of error in the confidence interval.
πŸ’‘Population Standard Deviation
The population standard deviation is a measure of the amount of variation or dispersion in a set of values within an entire population. The script discusses the scenario where this value is known, which allows for the computation of the confidence interval for the population mean using specific formulas.
πŸ’‘Sample Mean
The sample mean is the average of the values in a sample used to estimate the population mean. The video script explains that the sample mean, represented as x-bar, is calculated by summing all sample values and dividing by the sample size, n.
πŸ’‘Confidence Level
The confidence level is the probability that the true population parameter lies within the calculated confidence interval. The script mentions a 98% confidence level, which means that if the study is repeated multiple times, the confidence interval would contain the true population mean in 98 out of 100 cases.
πŸ’‘Alpha
Alpha is the significance level used in hypothesis testing and is related to the confidence level. In the script, alpha is the complement of the confidence level (1 - confidence level) and represents the area in the tails of the distribution. It is used to find the z-score that corresponds to the desired confidence level.
πŸ’‘Estimation
Estimation in the context of the video refers to the process of approximating the population mean based on sample data. The script discusses various methods for estimating the population standard deviation when it is not known, such as using the range rule of thumb or computing a sample standard deviation.
Highlights

The video discusses learning outcome number four of lesson 7.2, focusing on estimating a population mean with desired accuracy and confidence.

Introduction of formulas for the confidence interval for estimating a population mean when the population standard deviation is known.

Explanation of the margin of error formula using z-score and population standard deviation divided by the square root of the sample size.

The necessity of sample size calculation for achieving a desired margin of error and confidence level.

Rearranging the error formula to solve for the sample size n, given the margin of error and z-score.

The importance of rounding up the sample size to ensure less than intended error in the estimation.

Review of notation for confidence intervals of population means, including symbols for population mean, sample size, sample mean, margin of error, and z-score.

Discussion on the use of z-scores in relation to sample statistics and confidence levels.

Strategies for estimating the population standard deviation when it is unknown, including the range rule of thumb and using sample standard deviation.

The range rule of thumb states that dividing the range by four gives a rough estimate of the standard deviation.

The option to start sample collection without knowing the population standard deviation and refine the estimate as more data is collected.

Using previous study results to estimate the population standard deviation if known.

An example problem estimating the mean IQ score for attorneys with a given population standard deviation and desired confidence level.

The conservative nature of using the general population standard deviation for a specific group like attorneys.

Calculation of the required sample size for a 98% confidence level with a margin of error of three IQ points.

Explanation of finding the z-score corresponding to a 98% confidence level using Excel or a z-table.

The final calculation resulting in a sample size of at least 136 attorney IQ scores for the desired confidence interval.

The interpretation of the confidence interval and its implications for the reliability of the estimated mean IQ score of attorneys.

Transcripts

Browse More Related Video

7.2.0 Estimating a Population Mean - Lesson Overview, Key Concepts, Learning Outcomes

Statistics 101: Confidence Intervals, Estimating Sample Size Needed

7.1.6 Estimating a Pop. Proportion - The Right Sample Size for a Given Error and Confidence Level

How To Find The Z Score, Confidence Interval, and Margin of Error for a Population Mean

7.2.2 Estimating a Population Mean - Sample Mean, Margin of Error, and Finding Confidence Intervals

7.2.5 Estimating a Population Mean - Confidence Intervals with Known Pop. Standard Deviation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Sample SizePopulation MeanConfidence IntervalStandard DeviationMargin of ErrorStatistical AnalysisEstimation MethodZ-ScoreData CollectionIQ Scores