5.1.4 Discrete Probability Distributions - The Range Rule of Thumb and Significant Values

Sasha Townsend - Tulsa
15 Oct 202005:58
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains the 'range rule of thumb' for determining if a random variable's value is significantly high or low. It emphasizes that values more than two standard deviations from the mean are considered significant. Using a probability distribution, the mean and standard deviation can be calculated to identify these values. The video illustrates this with an example of an X-linked genetic disorder, calculating the mean and standard deviation, and then applying the rule to determine if outcomes of zero or five children inheriting the disorder are statistically significant.

Takeaways
  • 📚 The video discusses the 'range rule of thumb' for determining if a value of a random variable is significantly high or low.
  • 📏 The rule states that values more than two standard deviations from the mean are considered significantly high or low.
  • 📉 Significantly low values are those that are at the mean minus two standard deviations or lower.
  • 📈 Significantly high values are those that are at the mean plus two standard deviations or higher.
  • 🔢 The mean and standard deviation can be calculated using a probability distribution.
  • 📋 The range rule of thumb is not rigid; some might use three standard deviations or a different number.
  • 📊 Values within two standard deviations of the mean are not considered significant in a statistical sense.
  • 🧬 The example given involves a genetic disorder with five males, where the random variable is the number of children who inherit the disorder.
  • 👶 The mean number of children inheriting the disorder was found to be 2.5, with a standard deviation of about 1.1.
  • ✏️ Using the calculated mean and standard deviation, the video shows how to determine if outcomes are significantly high or low.
  • 📝 The outcomes of zero or five children inheriting the disorder are considered significantly low and high, respectively, while one to four are not statistically significant.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the application of the range rule of thumb to determine if a given value of a random variable is significantly high or low, using a probability distribution.

  • What is the range rule of thumb mentioned in the video?

    -The range rule of thumb states that values more than two standard deviations from the mean are considered significantly high or low.

  • What values are considered significantly low according to the range rule of thumb?

    -Significantly low values are those that are the mean minus two standard deviations or lower.

  • What values are considered significantly high according to the range rule of thumb?

    -Significantly high values are those that are the mean plus two standard deviations or higher.

  • What is the significance of values within two standard deviations of the mean?

    -Values within two standard deviations of the mean are not considered significant in a statistical sense.

  • Is the choice of two standard deviations from the mean a rigid rule?

    -No, the choice of two standard deviations is not rigid; it is a preference used in the course and can vary among different sources.

  • What is the random variable in the example involving five males with an X-linked genetic disorder?

    -The random variable is the number of children among the five who inherit the disorder.

  • What was the mean number of children who inherited the disorder in the example?

    -The mean number of children who inherited the disorder was found to be 2.5 children.

  • What was the standard deviation of the number of children who inherited the disorder in the example?

    -The standard deviation was found to be about 1.1 children.

  • How do you calculate significantly low values for the given example?

    -To calculate significantly low values, you subtract twice the standard deviation from the mean, which in this case is 2.5 - 2 * 1.1 = 0.3 or lower.

  • How do you calculate significantly high values for the given example?

    -To calculate significantly high values, you add twice the standard deviation to the mean, which is 2.5 + 2 * 1.1 = 4.7 or higher.

  • What does the video script suggest about the outcomes of zero or five children inheriting the disorder?

    -The script suggests that zero children inheriting the disorder is significantly low, and five children inheriting the disorder is significantly high in a statistical sense.

  • What is the significance of the number of children inheriting the disorder being between one and four?

    -The number of children inheriting the disorder being between one and four is not considered significant in a statistical sense, as it falls within two standard deviations of the mean.

Outlines
00:00
📊 Understanding Statistical Significance with Range Rule of Thumb

This paragraph introduces the concept of determining statistical significance using the range rule of thumb, which involves comparing a given value of a random variable to the mean and standard deviation. It explains that values more than two standard deviations from the mean are considered significantly high or low. The significance is illustrated with a graph, showing the middle range as non-significant and the extremes as significant. The video script also discusses a specific example involving an X-linked genetic disorder, where the random variable is the number of children among five who inherit the disorder. The mean and standard deviation for this example are calculated, and the range rule of thumb is applied to determine the significance of outcomes, such as four or five children inheriting the disorder.

05:05
📈 Applying the Range Rule of Thumb to Analyze Genetic Inheritance Outcomes

The second paragraph continues the discussion on the range rule of thumb, focusing on the outcomes of the genetic disorder example. It clarifies the outcomes where no children or all five children inherit the disorder as significantly low and high, respectively. The paragraph also corrects a previous calculation error, emphasizing the correct values for significantly high outcomes. The summary concludes by visually representing the significance levels on a number line, differentiating between significantly high, low, and non-significant outcomes based on the calculated mean and standard deviations.

Mindmap
Keywords
💡Range Rule of Thumb
The 'Range Rule of Thumb' is a statistical concept used to quickly determine whether a value from a dataset is unusually high or low. In the context of the video, it is defined as values more than two standard deviations from the mean being considered significantly high or low. This rule is central to the video's theme, as it's used to analyze the significance of outcomes in a probability distribution related to a genetic disorder.
💡Standard Deviation
Standard deviation is a measure of the amount of variation or dispersion in a set of values. In the video, it is used to quantify the dispersion of the number of children who might inherit a genetic disorder. The script explains that values more than two standard deviations from the mean are significant, which is a key part of applying the 'Range Rule of Thumb'.
💡Mean
The mean, often referred to as the average, is the sum of all values in a dataset divided by the number of values. In the script, the mean is calculated for the number of children inheriting a genetic disorder, which is 2.5 children. This mean serves as a reference point for determining significant values using the 'Range Rule of Thumb'.
💡Significantly High/Low
The terms 'significantly high' and 'significantly low' are used to describe outcomes that are unusual or unexpected based on a statistical analysis. In the video, any value more than two standard deviations above or below the mean is labeled as such. This concept is crucial for understanding the significance of the outcomes in the genetic disorder example.
💡Probability Distribution
A probability distribution is a statistical description of a random variable that shows the likelihood of different possible outcomes. In the video, the probability distribution is used to list the probabilities of different numbers of children inheriting a disorder, which is essential for understanding the context of the 'Range Rule of Thumb' application.
💡Genetic Disorder
A genetic disorder is a disease caused by mutations in an individual's DNA. In the video, the genetic disorder is an X-linked condition affecting males, and the random variable is the number of children among five who inherit this disorder. This serves as the practical example through which the statistical concepts are explained.
💡Random Variable
A random variable is a variable that can take on different values, each associated with a probability, in a statistical experiment. In the script, the random variable is the number of children among five who inherit the genetic disorder. It is central to the video's discussion of statistical significance.
💡Statistical Significance
Statistical significance refers to the likelihood that a result is not due to random chance. In the video, the script uses the 'Range Rule of Thumb' to determine if the number of children inheriting a disorder is statistically significant, which means it is unlikely to have occurred by chance.
💡X-linked Genetic Disorder
An X-linked genetic disorder is a condition caused by a mutation in one of the X chromosomes. In the video, the disorder is used as a specific example to illustrate the application of the 'Range Rule of Thumb' in determining the significance of the number of children inheriting the condition.
💡Error Correction
The script includes a moment of error correction where the presenter corrects a miscalculation regarding the significantly high value. This demonstrates the importance of accuracy in statistical analysis and serves as a teaching moment within the video.
💡Number Line
The number line is a visual representation of numbers in a sequential order. In the video, the presenter uses a number line to illustrate the calculation of significant values, showing the mean and the points of one and two standard deviations above and below it. This visual aid helps viewers understand the concept of significant values in a more concrete way.
Highlights

The video discusses learning outcome number four from lesson 5.1, focusing on using the range rule of thumb to determine if a value of a random variable is significantly high or low.

The range rule of thumb states that values more than two standard deviations from the mean are significantly high or low.

Significantly low values are defined as the mean minus two standard deviations or lower.

Significantly high values are defined as the mean plus two standard deviations or higher.

The mean and standard deviation can be calculated using a probability distribution to determine if a value is significantly high or low.

Values within two standard deviations of the mean are not considered significant in a statistical sense.

The choice of two standard deviations from the mean is not rigid and can vary based on the preference of the course or instructor.

A graph is used to visually represent the mean, standard deviations, and the range of significantly high and low values.

The example in the video involves a probability distribution of the number of children among five who inherit an X-linked genetic disorder.

The mean number of children inheriting the disorder was previously calculated to be 2.5.

The standard deviation for this scenario was found to be approximately 1.1 children.

Using the range rule of thumb, the video demonstrates how to determine if outcomes of four or five children inheriting the disorder are significantly high.

Calculations for significantly low values involve subtracting two times the standard deviation from the mean.

Significantly high values are calculated by adding two times the standard deviation to the mean.

The video corrects an error in the calculation of significantly high values, adjusting it to 4.7 instead of 5.7.

Values between 0.3 and 4.7 are not considered significantly high or low in this example.

The video visually demonstrates the range of significantly high and low values on a number line.

Outcomes of zero children or five children inheriting the disorder are considered significantly low and high, respectively.

Any other number of children inheriting the disorder, such as one, two, three, or four, is not statistically significant.

Transcripts

Browse More Related Video

[5.1.16] Using the Range Rule of Thumb to find significant values

5.2.4 Binomial Distributions - Mean, St. Dev., Using Range Rule of Thumb to Find Significant Values

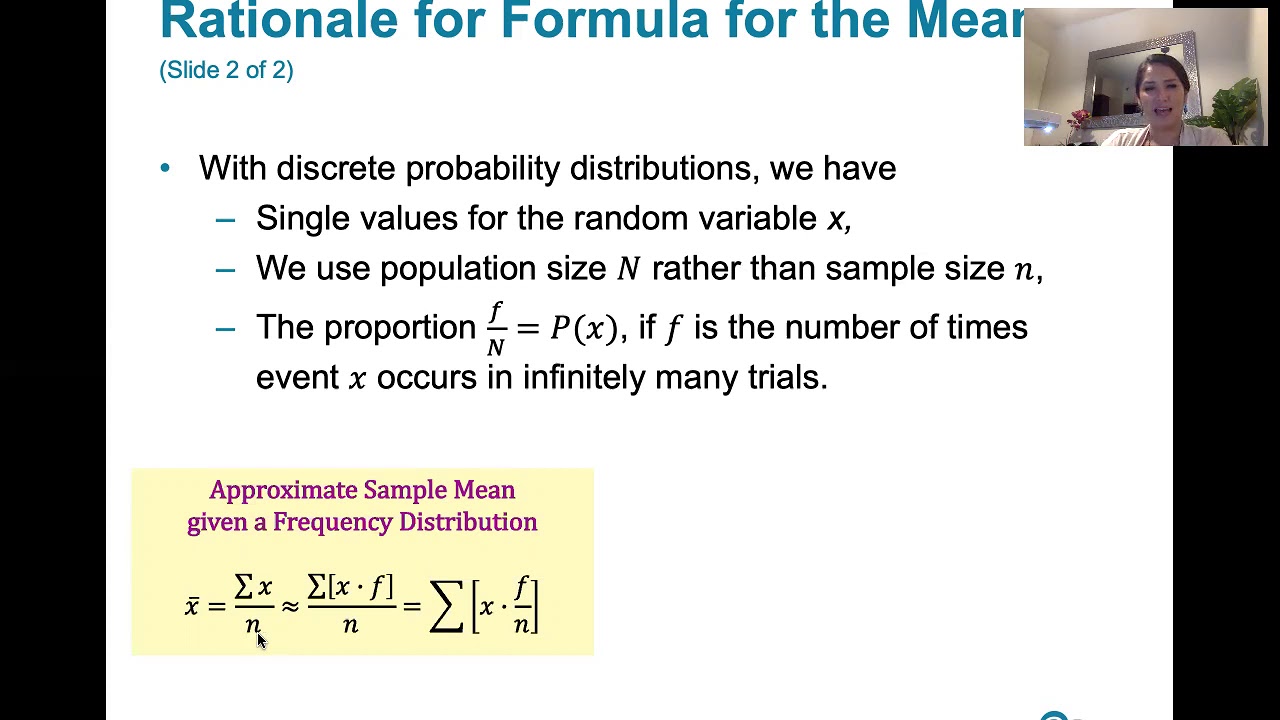
5.1.3 Discrete Probability Distributions - Finding the Mean, Variance, and Standard Deviation

Normal Distribution, Z-Scores & Empirical Rule | Statistics Tutorial #3 | MarinStatsLectures

Empirical Rule of Standard Deviation in Statistics

Standard Deviation - Explained and Visualized

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Statistics LearningRange RuleStandard DeviationsProbability DistributionSignificant ValuesMean CalculationGenetic DisordersStatistical AnalysisData InterpretationEducational Video