4.1.4 Basics of Probability - The Rare Event Rule of Inferential Statistics
TLDRThis video script delves into the rare event rule in inferential statistics, a fundamental principle used to evaluate the validity of assumptions by observing if outcomes significantly deviate from expected probabilities. It uses the example of a gender selection method to illustrate hypothesis testing, where unlikely results lead to the rejection of the null hypothesis. The script emphasizes the significance of the rare event rule in statistical analysis and its application in determining significantly high or low outcomes, often using a 0.05 probability threshold.
Takeaways
- 📚 The Rare Event Rule: In inferential statistics, if an event with a very low probability occurs significantly more or less often than expected, the underlying assumption is likely incorrect.
- 🔍 Hypothesis Testing: The rare event rule is crucial in hypothesis testing, which will be discussed more in the latter part of the semester.
- 👶 Gender Selection Method: A claim is made that a gender selection method increases the likelihood of having a girl, which will be tested using hypothesis testing.
- 🤔 Null Hypothesis: The initial assumption in the gender selection example is that the method has no effect, implying equal chances of having a boy or a girl.
- 🔢 Significance in Numbers: In Study A, 75 girls out of 100 births was considered significant due to its extremely low probability of occurring by chance (0.00003).
- ❌ Rejecting Chance: When the probability of an event is very low and it still occurs, it suggests that the null hypothesis may be wrong, as in the case of Study A.
- 📉 Insignificant Results: In Study B, 55 girls out of 100 had a higher probability (18.4%) of occurring by chance, so the null hypothesis was not rejected.
- 🚫 No Conclusion on Effect: With Study B, the slight difference in gender ratio was not enough to conclude the gender selection method's effectiveness.
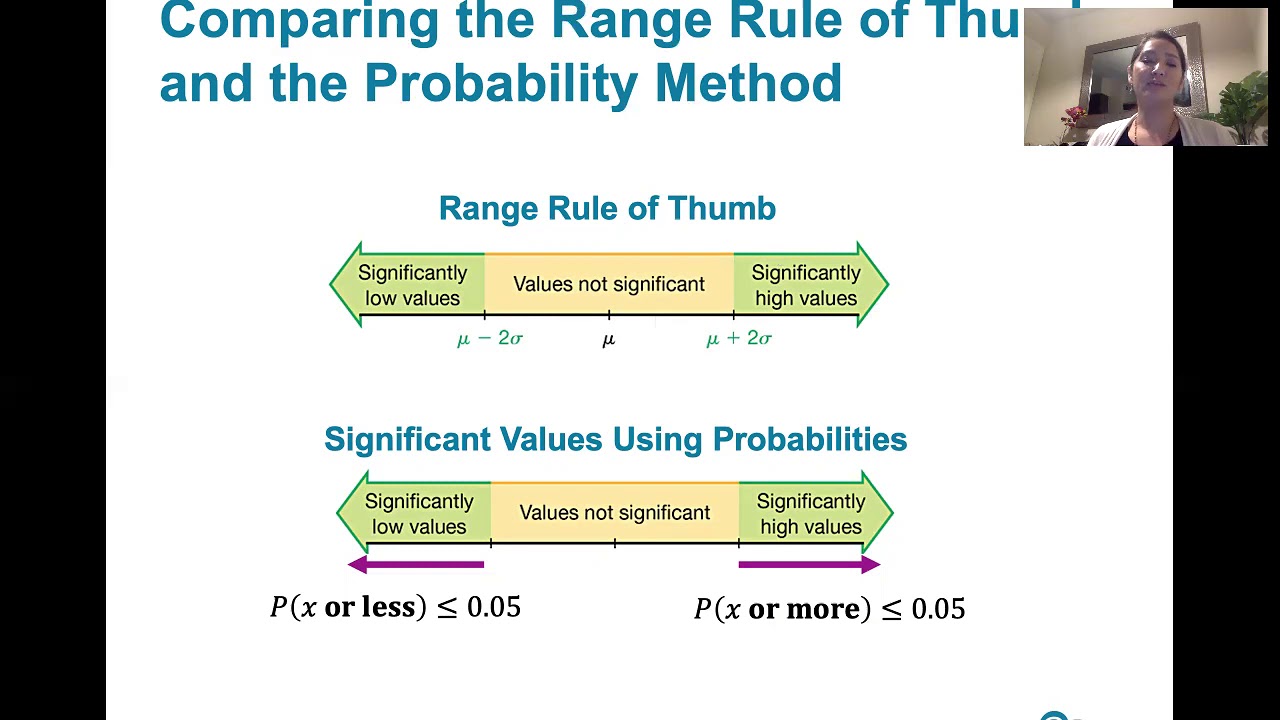
- 📈 Probability and Success: The script discusses how to determine if a number of successes is significantly high or low using a probability threshold, commonly set at 0.05.
- 📊 Statistical Significance: A success count is considered significantly high if the probability of achieving that count or more is less than 0.05, and significantly low if the probability of achieving that count or fewer is less than 0.05.
Q & A
What is the rare event rule in inferential statistics?
-The rare event rule states that if the probability of a particular observed event is very small under a given assumption, and the event occurs significantly more or less often than expected, we can conclude that the assumption is probably not correct.
Why is the rare event rule important in statistics?
-The rare event rule is important because it plays a crucial role in hypothesis testing, allowing statisticians to reject explanations based on very low probabilities when an unlikely event occurs.
What is the significance of the number 0.00003 in the context of the rare event rule?
-The number 0.00003 represents an extremely low probability of an event occurring by chance. If such an event does occur, it suggests that the underlying assumption may be incorrect, leading to the rejection of the null hypothesis.
How does the gender selection method example relate to the rare event rule?
-The gender selection method example illustrates the rare event rule by testing the hypothesis that the method has no effect. If the observed results significantly deviate from the expected 50-50 chance, it suggests the method may be effective, contrary to the initial assumption.
What does it mean to 'reject chance as a reasonable explanation' in the context of hypothesis testing?
-Rejecting chance as a reasonable explanation means that the observed results are so unlikely to have occurred by chance alone that it suggests an alternative explanation, such as the effect of the gender selection method, is more plausible.
What is the significance of the probability value 0.184 in the example of study B?
-The probability value 0.184 indicates that there is an 18.4% chance of obtaining the observed results by chance, which is not considered unlikely enough to reject the null hypothesis that the gender selection method has no effect.
How is the term 'significantly high' defined in the context of inferential statistics?
-A significantly high number of successes is defined as having a probability of x or more successes that is less than or equal to 0.05, indicating that it is unlikely to have occurred by chance.
What is the threshold for considering a number of successes as 'significantly low'?
-A number of successes is considered significantly low if the probability of x successes or fewer is less than or equal to 0.05, suggesting it is unlikely to have occurred by chance.
What is the role of the number 0.05 in statistical hypothesis testing?
-The number 0.05 is commonly used as a threshold for statistical significance. If the probability of observing the results (or more extreme) is less than or equal to 0.05, it is considered statistically significant, leading to the rejection of the null hypothesis.
Can the threshold for significance be changed, and if so, under what circumstances?
-Yes, the threshold for significance can be changed based on the context of the study or the field of research. For example, a 0.01 or 0.1 threshold might be used in different situations to indicate a higher or lower level of confidence in the results.
Outlines
📊 Introduction to the Rare Event Rule in Inferential Statistics
This paragraph introduces the rare event rule, a fundamental principle in inferential statistics used to evaluate the significance of observed outcomes. It explains that if an event with a low probability occurs more or less frequently than expected under a given assumption, this suggests the assumption may be incorrect. The concept is illustrated with a hypothetical gender selection method, where the hypothesis is tested that the method has no effect on the likelihood of having a girl. The example of Study A, with 75 girls out of 100 births, is used to demonstrate the rule, as the probability of such an outcome by chance is extremely low, leading to the rejection of the hypothesis that the method is ineffective.
🔍 Analyzing Study Results with the Rare Event Rule
The second paragraph delves deeper into applying the rare event rule to study results, contrasting the outcomes of two studies. Study A, with its improbable result of 75 girls from 100 births, leads to the rejection of the hypothesis of no effect from the gender selection method, suggesting it is effective. Conversely, Study B, with 55 girls and 45 boys, shows a more expected distribution, as the probability of this outcome occurring by chance is 18.4%, which is not considered rare. This indicates that the gender selection method may not be effective, and the initial assumption of no effect is not rejected. The importance of the rare event rule in hypothesis testing is emphasized, highlighting its role in statistical analysis.
📉 Significance of High and Low Success Rates in Hypothesis Testing
The final paragraph focuses on identifying significantly high or low success rates within the context of hypothesis testing. It explains that a success rate is considered significantly high if the probability of achieving the same or more successes is less than or equal to 0.05, and significantly low if the probability of achieving the same or fewer successes is at the same threshold. The paragraph clarifies the use of 0.05 as a common probability value for determining significance in social sciences, but also notes that this threshold can be adjusted based on the context. The importance of this concept in understanding and interpreting statistical results is stressed, with the expectation that it will be revisited throughout the semester, particularly in the context of hypothesis testing.
Mindmap
Keywords
💡Rare Event Rule
💡Inferential Statistics
💡Hypothesis Testing
💡Assumption
💡Probability
💡Significantly High/Low
💡Gender Selection Method
💡Null Hypothesis
💡Sample Data
💡Significance Level (0.05)
Highlights
Explanation of the rare event rule in inferential statistics.
Definition of the rare event rule: If under a given assumption, the probability of an observed event is very small and it occurs significantly more or less often than expected, the assumption is likely incorrect.
Breaking down the rare event rule into simpler terms for better understanding.
Example of gender selection method to illustrate the rare event rule.
Assumption that the gender selection method has no effect, expecting 50% chance of girls.
Study A: 75 out of 100 births were girls, with a probability of 3 in 10 million.
Conclusion from Study A: Gender selection method is likely effective due to very low probability of the result occurring by chance.
Study B: 55 out of 100 births were girls, with a probability of 18.4%.
Conclusion from Study B: Gender selection method is not necessarily effective since the probability of the result occurring by chance is relatively high.
Importance of rejecting explanations based on very low probabilities in hypothesis testing.
Significantly high number of successes defined as a probability of 0.05 or less for x or more successes.
Significantly low number of successes defined as a probability of 0.05 or less for x or fewer successes.
Usage of 0.05 probability value in social sciences and its significance.
Flexibility in choosing the probability value for defining significantly high or low numbers of successes.
Summary of key points and importance of understanding the rare event rule for hypothesis testing.
Transcripts
Browse More Related Video
5.1.5 Discrete Probability Distributions - Use Probabilities to Determine Significant Values

6.4.3 The Central Limit Theorem - The Rare Event Rule and the Central Limit Theorem

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

Statistical Significance and p-Values Explained Intuitively
8.1.1 Basics of Hypothesis Testing - The Big Picture, Null and Alternative Hypotheses
How to calculate p-values
5.0 / 5 (0 votes)
Thanks for rating: