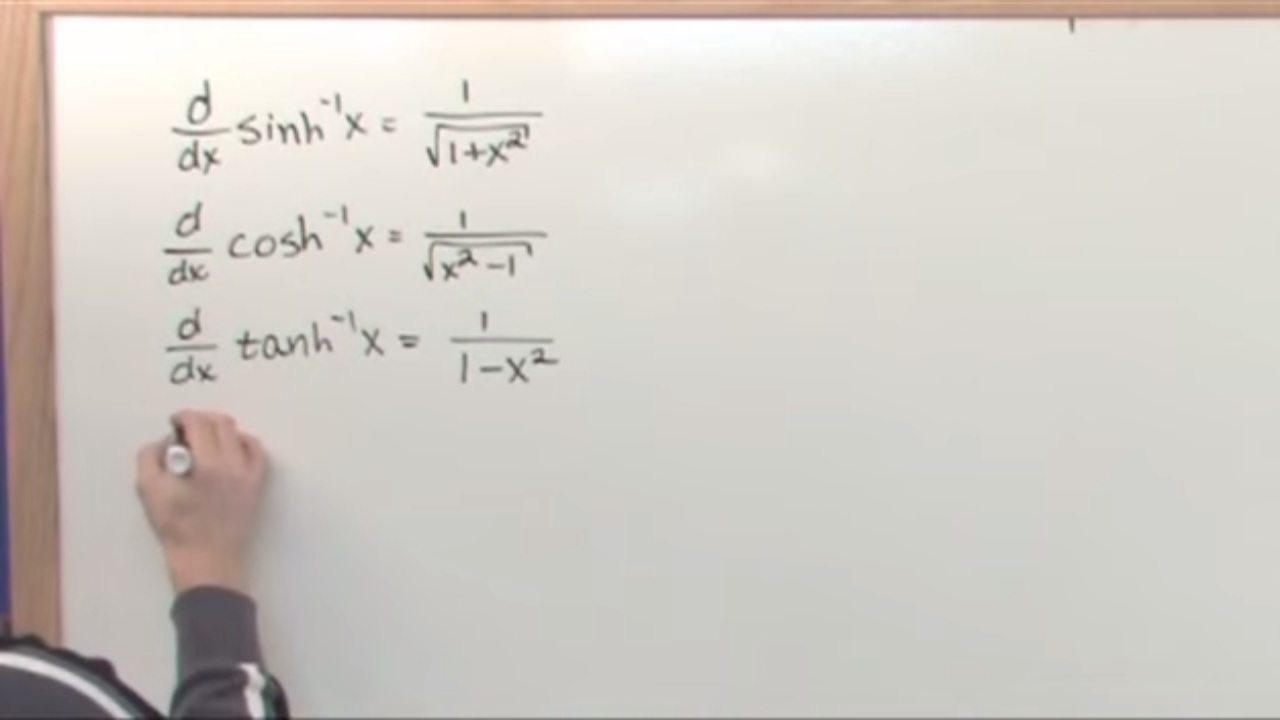Derivatives of Inverse Functions

RH Mathematics
14 Sept 202113:59
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial explains the concept of inverse functions and how to derive them. It begins with defining inverse functions and uses the example of y = x^2 to illustrate finding inverse values. The instructor then covers the geometric interpretation of inverse functions and introduces the formula for the derivative of an inverse function, which is 1/f'(f^(-1)(x)). The explanation is supported by a step-by-step example using a table of values, and the importance of inverse functions being bijections in AP Calculus is highlighted. The video also touches on arc trigonometric derivatives and concludes with examples of finding equations for tangent lines to inverse trigonometric functions.

Takeaways
  • 📚 The video discusses the process of finding the derivative of an inverse function, starting with a refresher on how inverse functions work.
  • 🔍 The inverse image of a value \( f^{-1}(k) \) is defined as the set of all \( x \)'s for which \( f(x) = k \).
  • 📈 A familiar example used is \( y = x^2 \), denoted as \( g(x) \), and the process of finding \( g^{-1}(9) \) is explained geometrically and algebraically.
  • 🤔 The video emphasizes that inverse functions take in \( y \) values and return \( x \) values, which is crucial for understanding how to find the derivative of an inverse function.
  • 📉 Geometrically, finding \( g^{-1}(0) \) involves identifying the \( x \)-value where \( g(x) = 0 \), which is a single point in this case.
  • 🚫 The video clarifies that in the context of AP Calculus, inverse functions will only return one value, even if the original function has multiple solutions.
  • 🔄 The formula for the derivative of an inverse function is \( \frac{d}{dx}(f^{-1}(x)) = \frac{1}{f'(f^{-1}(x))} \), which is derived using the chain rule.
  • 🌐 The video provides an example using a table of values to find \( g(3) \) and \( g'(3) \), demonstrating how to apply the derivative formula for inverse functions.
  • 📌 The concept of \( h \) being the inverse function of \( g \) is introduced, with an example showing how to find the equation of a line tangent to the graph of \( h \).
  • 📚 The video concludes with a discussion on the derivatives of inverse trigonometric functions, emphasizing the need to memorize these formulas for future use.
Q & A

  • What is the definition of an inverse function according to the video?

    -The inverse function, denoted as f inverse of k, is the set of all x's for which f of x equals k.

  • What is the function g(x) used to illustrate the concept of inverse functions in the video?

    -The function g(x) used in the video is y equals x squared.

  • How does the video demonstrate finding g inverse of 9?

    -The video demonstrates finding g inverse of 9 by setting g(x) equal to 9, which leads to x squared equals 9, and then solving for x to get x equals plus or minus 3.

  • What does the video suggest is the geometric interpretation of g inverse of 9?

    -Geometrically, finding g inverse of 9 involves locating the points on the graph of g(x) where the y-value is 9, and then determining the corresponding x-values.

  • What is the result of g inverse of zero according to the video?

    -The result of g inverse of zero is x equals zero, since g(x) equals x squared equals zero.

  • Why does the video state that g inverse of negative four has no real solution?

    -The video states that g inverse of negative four has no real solution because x squared cannot equal a negative number in the real number system.

  • What is the formula for the derivative of an inverse function as presented in the video?

    -The formula for the derivative of an inverse function is the derivative of the inverse at a given x value, which is 1 over f prime of f inverse of x.

  • How does the video explain the proof for the derivative of an inverse function?

    -The proof is based on the chain rule and the property that if f is the original function and f inverse is its inverse, then f of f inverse of x equals x, and their derivatives will also be equal.

  • What is the example used in the video to demonstrate finding the derivative of an inverse function?

    -The example used is finding g prime of 3, where g is the inverse function of f, and it involves using the formula 1 over f prime of f inverse of 3, with f inverse of 3 being 5 and f prime of 5 being negative two.

  • What are the three arc trigonometric derivatives that the video mentions need to be memorized?

    -The video mentions that the derivatives of arc sine, arc cosine, and arc tangent are the three formulas that need to be memorized for inverse trigonometric functions.

  • How does the video approach the task of finding the equation of a tangent line to a function?

    -The video approaches this by finding a point on the function and the slope of the tangent line at that point, then using the point-slope form of a line to write the equation of the tangent.

Outlines
00:00
📚 Understanding Inverse Functions and Derivatives

This paragraph introduces the concept of inverse functions and their derivatives. The instructor explains the definition of an inverse function, using the example of g(x) = x^2, and discusses how to find the inverse function's value for a given y, such as g^(-1)(9). The geometric interpretation of inverse functions is also covered, including how to find the inverse function's value on a graph. The paragraph concludes with the introduction of the formula for the derivative of an inverse function, which is 1/f'(f^(-1)(x)).

05:01
🔍 Deriving Inverse Functions with Examples

The second paragraph delves deeper into the process of deriving inverse functions, starting with a discussion on the chain rule and how it applies to inverse functions. The instructor uses the example of g(x) = x^2 to demonstrate how to find the derivative of the inverse function at a specific point, such as g'(3). The explanation includes the steps to find the original function's inverse value and its derivative, leading to the application of the formula 1/f'(f^(-1)(x)). Additionally, the paragraph touches on the concept of functions being bijections and the implications for inverse functions in the context of AP Calculus.

10:01
📉 Exploring Derivatives of Inverse Trigonometric Functions

The third paragraph focuses on the derivatives of inverse trigonometric functions, presenting three key formulas that students must memorize. The instructor discusses the challenges of differentiating equations with intermingled x's and y's and emphasizes the importance of understanding these derivatives for future problems. The paragraph provides an example of finding the equation of a tangent line to the graph of y = arcsine(2x) at x = 1/4, illustrating the process of finding the point of tangency and the slope using the derivative formula for inverse trigonometric functions. The paragraph concludes with a teaser for a future problem involving the tangent function, encouraging students to practice these concepts.

Mindmap
Keywords
💡Derivative
The derivative in calculus is a measure of how a function changes as its input changes. It is the rate at which the output of a function changes with respect to changes in its input. In the video, the concept of derivatives is central to understanding how to calculate the rate of change for inverse functions, as the script discusses the process of finding the derivative of an inverse function using the formula 1/f'(f^(-1)(x)).
💡Inverse Function
An inverse function is a mathematical function that 'reverses' another function: applying the inverse function to its result will return the original input. The script explains that the inverse image of a value f^(-1)(k) is the set of all x's for which f(x) equals k, and it uses the function y = x^2 as an example to illustrate how to find the inverse function.
💡Square Root
The square root operation is used to find the value that, when multiplied by itself, gives the original number. In the script, the square root is used to solve the equation x^2 = 9, which is part of the process to find the inverse function of y = x^2.
💡Bijection
A bijection is a one-to-one correspondence between the elements of two sets. In the context of the video, the script mentions that AP Calculus typically asks about inverse functions that are at least locally bijections, meaning each input has a unique output and vice versa, which is a requirement for a function to have an inverse.
💡Chain Rule
The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. The script uses the chain rule to derive the formula for the derivative of an inverse function, showing that (f^(-1))'(x) = 1/f'(f^(-1)(x)).
💡Arc Trigonometric Functions
Arc trigonometric functions, such as arcsine, arccosine, and arctangent, are the inverses of the trigonometric sine, cosine, and tangent functions, respectively. The script mentions these functions and their derivatives, which are essential for understanding how to find the derivatives of inverse trigonometric functions.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. The script discusses finding the equation of the tangent line to a curve at a specific point, which involves using the derivative of the function at that point to determine the slope of the tangent line.
💡Table of Values
A table of values is a list of x and y pairs that represent the output of a function for various inputs. The script mentions that in AP Calculus, when evaluating inverse functions, the function will return a single value, and this is often presented in a table of values format.
💡Geometric Interpretation
The geometric interpretation of a mathematical concept helps to visualize it in terms of shapes, lines, and points. In the script, the geometric interpretation is used to explain how to find the inverse function by looking at the graph of the original function and determining where it intersects with certain values.
💡Real Solution
A real solution is a value that is a real number and satisfies the equation in question. The script notes that when solving for the inverse of a function like x^2 = -4, which has no real solutions, the focus is on real-valued functions and their inverses.
Highlights

Introduction to the concept of inverse functions and their definition in the context of a function's inverse image.

Explanation of how to find the inverse of a function using the example of y = x squared.

Geometric interpretation of finding the inverse function, illustrated with the graph of y = x squared.

Clarification on the uniqueness of values returned by inverse functions in AP Calculus.

Discussion on the local bijection property required for a function to have an inverse in the context of AP Calculus.

Derivation of the formula for the derivative of an inverse function using the chain rule.

Illustration of how inverse functions undo each other, leading to the derivation formula.

Example of finding the derivative of an inverse function using a table of values.

Explanation of how to interpret coded language for inverse functions in AP Calculus questions.

Introduction to arc trigonometric derivatives, which are essential for advanced calculus.

Emphasis on memorizing the three arc trigonometric derivative formulas for future use.

Demonstration of finding the equation of a tangent line to the graph of y = arc sine of 2x at a specific point.

Use of the chain rule to find the derivative of a function involving an inverse trigonometric function.

Example of calculating the slope of a tangent line for the function y = r tangent of x divided by 2 at a given x value.

Final example of finding the equation of a tangent line, encouraging students to try it themselves before revealing the answer.

Completion of the tangent line equation example, showcasing the process and final result.

Transcripts

Browse More Related Video

Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

Derivative of Inverse Functions | Calculus 1

Composite and Inverse Functions

Derivative of Inverse Trig Functions via Implicit Differentiation

Introduction to Inverse Functions

AP Calculus AB: Lesson 3.5 Derivatives of Inverse Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Inverse FunctionsDerivative CalculationCalculus TutorialMathematics EducationFunction PropertiesTrigonometric FunctionsChain RuleAP CalculusInverse TrigonometryTangent Lines