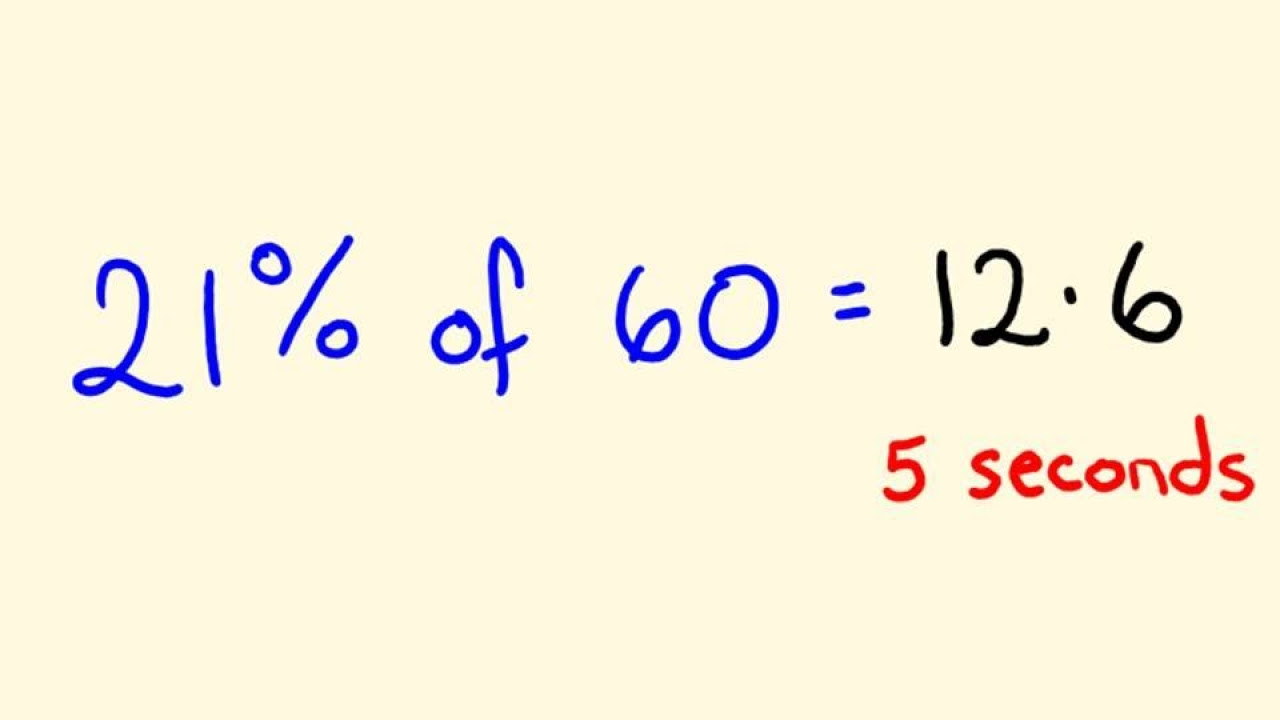Math Antics - What Are Percentages?

mathantics
30 Oct 201208:53
EducationalLearning
32 Likes 10 Comments

TLDRThe Math Antics video script introduces percentages as a special type of fraction with 100 as the denominator. It explains how percentages are used in everyday life for sales tax, discounts, nutrition, and investments. The script clarifies that percentages can be expressed as fractions or decimals and demonstrates the conversion process. With examples, it shows how to convert percentages to decimals by moving the decimal point two places to the left and vice versa, highlighting the significance of percentages in real-world applications.

Takeaways
  • 🧩 Percentages are a fundamental part of everyday life and are used frequently in real-life situations.
  • 📊 A percentage is essentially a fraction with 100 as the denominator, which is why it's represented with the percent sign (%).
  • 📐 The term 'percent' comes from 'per centum' in Latin, meaning 'per 100', which is why percentages are fractions with 100 as the base.
  • 🔢 Percentages can be easily converted to fractions, for example, 15% is the same as 15/100.
  • 📉 Zero percent (0%) is equivalent to zero, and one hundred percent (100%) is equivalent to one whole or 1, representing a 'whole fraction'.
  • 📈 Numbers greater than 100%, such as 126%, are considered improper fractions because the numerator is larger than the denominator.
  • 🔄 Percentages can also be converted into decimal form by moving the decimal point two places to the left and removing the percent sign.
  • 📌 The conversion of percentages to decimals is straightforward because percentages are base-10 fractions, representing hundredths.
  • 📐 Understanding the concept of percentages is crucial as they can be represented in fraction form, decimal form, or with the percent sign.
  • 📘 The script emphasizes the importance of practicing with exercises to solidify the understanding of percentages and their applications.
  • 🌐 For further learning, the Math Antics website is provided as a resource for more information on percentages and related math concepts.
Q & A

  • What is the main topic discussed in the Math Antics video?

    -The main topic discussed in the Math Antics video is percentages, explaining what they are, how they are used in real life, and how they relate to fractions and decimals.

  • Why are percentages considered important in everyday life?

    -Percentages are important in everyday life because they are used to calculate things like sales tax, discounts on sales, nutritional content in food, and potential earnings from investments in the stock market.

  • What is the relationship between percentages and fractions?

    -A percentage is a special type of fraction where the denominator is always 100. This means that a percentage can be represented as a fraction with 100 as the bottom number.

  • How is the percent sign (%) used in representing percentages?

    -The percent sign (%) is used to denote that a number is a percentage. It is placed after the number to indicate that it is a fraction with 100 as the denominator.

  • What does the word 'percent' literally mean?

    -The word 'percent' literally means 'per 100', derived from the prefix 'per' meaning 'for each' or 'for every' and the root 'cent' from Latin meaning 100.

  • How can you convert a percentage to a fraction?

    -To convert a percentage to a fraction, you write the percentage number over 100. For example, 15% is equivalent to 15/100.

  • What is the value of 0% in terms of a fraction?

    -The value of 0% is 0/100, which is considered a 'zero fraction' and its value is zero.

  • What is the significance of 100% in terms of a fraction?

    -100% is equivalent to 100/100, which is known as a 'whole fraction', and its value is one whole or 1.

  • How can you convert a percentage to a decimal?

    -To convert a percentage to a decimal, you move the decimal point two places to the left from its position in the whole number and remove the percent sign. For example, 35% becomes 0.35.

  • What is the decimal equivalent of 4%?

    -The decimal equivalent of 4% is 0.04. You add a zero as a placeholder and then move the decimal point two places to the left.

  • How can you convert a decimal to a percentage?

    -To convert a decimal to a percentage, you move the decimal point two places to the right and add a percent sign. For example, 0.25 becomes 25%.

Outlines
00:00
📚 Introduction to Percentages

This paragraph introduces the concept of percentages as a fundamental part of everyday life, explaining their importance in various real-life scenarios such as calculating sales tax, discounts, nutritional content, and investment returns. It clarifies that percentages are a type of fraction with 100 as the denominator and uses the percent sign (%) for representation. The explanation includes the etymology of 'percent,' linking it to its Latin roots meaning 'per 100.' The paragraph also demonstrates how to convert percentages to their fraction form and highlights special cases like 0% and 100%, which correspond to zero and one, respectively. It also touches on the concept of improper fractions when the numerator exceeds 100.

05:03
🔢 Converting Percentages to Decimals

This paragraph focuses on the conversion of percentages to decimal form, a process made straightforward because percentages are base-10 fractions. It outlines a simple trick for conversion: moving the decimal point two places to the left from its position in the whole number and removing the percent sign. Several examples illustrate this method, including how to handle single-digit percentages by using a zero as a placeholder. The paragraph also explains the representation of 0% and 100% in decimal form, which are 0.00 and 1.00, respectively, and how values exceeding 100% result in decimals greater than one, reflecting their status as improper fractions.

Mindmap
Keywords
💡Percentages
Percentages are a way of expressing a number as a fraction of 100. They are fundamental to the video's theme as they are presented as a real-life application of math. For example, the script mentions using percentages to calculate sales tax, discounts on sales, nutritional content, and investment returns, illustrating their ubiquity in everyday life.
💡Fractions
Fractions are a mathematical representation of parts of a whole, where a numerator represents the part and the denominator represents the total number of equal parts. In the video, it is explained that percentages are a type of fraction with a denominator of 100, which simplifies the understanding of percentages as 'per 100'.
💡Percent Sign (%)
The percent sign is a symbol used to denote that a number is a percentage. The video emphasizes its function as a shorthand for fractions with a denominator of 100, such as '15 percent' being equivalent to '15 over 100'.
💡Sales Tax
Sales tax is a percentage of the purchase price that is added to the cost of goods or services. The script uses sales tax as an example of how percentages are used in real-life financial transactions.
💡Discount
A discount is a reduction in the original price of an item, typically expressed as a percentage. The video mentions discounts to illustrate how percentages are used to calculate the new price after a reduction.
💡Fiber
Fiber refers to the indigestible part of plant food that is important for a healthy diet. In the context of the video, fiber content is given as an example of how percentages can indicate the nutritional value in food, such as the percentage of daily fiber intake in a granola bar.
💡Stock Market
The stock market is a platform where shares of publicly traded companies are bought and sold. The video uses the stock market as an example of how percentages can represent potential gains or losses on investments.
💡Zero Fraction
A zero fraction is a fraction where the numerator is zero, resulting in a value of zero. In the video, 0% is described as a zero fraction, equating to 0 over 100, emphasizing that having zero as the numerator is acceptable.
💡Whole Fraction
A whole fraction is a fraction where the numerator and denominator are equal, resulting in a value of one. The script explains that 100% is a whole fraction, equivalent to 100 over 100, representing a complete or whole value.
💡Improper Fraction
An improper fraction is a fraction where the numerator is greater than the denominator, resulting in a value greater than one. The video uses 126% as an example of an improper fraction, explaining that it is equivalent to 126 over 100.
💡Decimal
A decimal is a way of representing fractions with a denominator of 10, 100, 1000, etc., using a decimal point. The video teaches that percentages can be easily converted to decimals because they are base-10 fractions, with examples such as 15% being 0.15 in decimal form.
Highlights

Introduction to percentages and their importance in everyday life.

Explanation that a percent is a fraction with 100 as the bottom number.

Clarification that percentages are a more compact way to write fractions with 100 as the denominator.

Examples demonstrating the conversion of percentages to fractions, such as 15% being 15 over 100.

Breakdown of the word 'percent' meaning 'per 100' due to its Latin roots.

Discussion on the equivalence of 0% to 0 over 100 and 100% to 100 over 100.

Explanation of improper fractions like 126% being 126 over 100.

Introduction to converting percentages to decimal form by moving the decimal point two places to the left.

Examples of converting percentages to decimals, such as 35% to 0.35 and 75% to 0.75.

Handling single-digit percentages like 4% by using a zero placeholder to convert it to 0.04.

Demonstrating that 1% converts to 0.01 using the same method.

Confirming that 0% equals 0.00 and 100% equals 1.00 in decimal form.

Conversion of values greater than 100%, like 142% to 1.42.

Explanation that percentages can be rewritten as fractions or decimals interchangeably.

Encouragement to practice and understand the basics of percentages before moving on to more advanced topics.

Invitation to learn more and explore further videos and exercises on percentages.

Transcripts

Browse More Related Video

Working With Percentages

Converting Between Fractions, Decimals, and Percentages

Math Antics - Finding A Percent Of A Number

The GED Calculator How to Use it

Basic Math Review

The Percentages You'll See in Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PercentagesFractionsReal-LifeMathematicsConversionDecimalsEducationalTutorialPercentage BasicsMath Antics