Math Antics - Basic Probability

mathantics
15 May 201911:28
EducationalLearning
32 Likes 10 Comments

TLDRIn this Math Antics video, Rob introduces the concept of probability, explaining how it measures the likelihood of events that are uncertain. He uses examples like coin tosses, dice rolls, and spinners to illustrate how probabilities are calculated and how they can be represented as fractions, decimals, or percentages. The video also emphasizes the importance of conducting multiple trials to approach expected probabilities.

Takeaways
  • 😀 Probability is a measure of how likely an event is to occur, often expressed as a fraction, decimal, or percentage.
  • 🎲 In a fair coin toss, the probability of getting heads or tails is equal, each being 1/2 or 50%.
  • 🔢 The Probability Line ranges from 0 (impossible) to 1 (certain), and all probabilities must fall within this range.
  • 🎯 When rolling a standard six-sided die, each number has an equal chance of appearing, making the probability of rolling any specific number 1/6 or 16.7%.
  • 🧩 Conducting multiple trials or experiments helps to approximate the expected probabilities more closely.
  • 🔄 The more trials you perform, the more likely the results will align with the theoretical probabilities.
  • 🎲 Adding up the probabilities of all possible outcomes of an event should always equal 1 (or 100%), as one outcome is certain to occur.
  • 🌈 When using a spinner with multiple equally sized sectors, the probability of landing on a specific number or color is determined by dividing the total probability (1) by the number of sectors.
  • 💎 The probability of an event can be calculated by dividing the number of favorable outcomes by the total number of possible outcomes.
  • 📚 Understanding and applying the concept of probability involves practice and solving various problems to gain proficiency.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is probability, which is about the likelihood of events that are not always certain to happen.

  • Why is probability different from other areas of mathematics?

    -Probability is different because it deals with events that have uncertainty and are not always certain, unlike other areas of mathematics that deal with certainty and predictable outcomes.

  • What is a fair coin toss probability of landing heads or tails?

    -A fair coin toss has a probability of 1/2 or 50% for landing heads and the same probability for landing tails, as both outcomes are equally likely.

  • What is a Probability Line and what does it represent?

    -A Probability Line is a number line that ranges from 0 to 1, representing the likelihood of an event occurring. A probability of 0 means the event is impossible, and a probability of 1 means the event is certain.

  • How can you express probabilities in different forms?

    -Probabilities can be expressed as fractions, decimals, or percentages. For example, a probability of 1/2 can also be expressed as 0.5 or 50%.

  • What is the probability of rolling a 3 on a standard die?

    -The probability of rolling a 3 on a standard die is 1/6 or approximately 16.7%, as there are six equally likely outcomes.

  • What is a trial in the context of probability?

    -A trial in probability is a process with a random outcome, such as tossing a coin, rolling dice, or spinning a spinner.

  • Why might the results of a few trials not be close to the expected probabilities?

    -The results of a few trials might not be close to the expected probabilities because of the unpredictable nature of random events. More trials are needed to approach the expected probabilities.

  • What is the significance of the sum of probabilities for all possible outcomes of a trial?

    -The sum of probabilities for all possible outcomes of a trial must equal 1 or 100%, as it is certain that at least one of the outcomes will occur.

  • How is the probability of spinning a blue sector on a spinner with 16 equally sized sectors calculated?

    -If there are 5 blue sectors out of 16, the probability of spinning a blue is 5/16 or about 31%, which is calculated by dividing the number of favorable outcomes by the total number of possible outcomes.

  • What is the probability of pulling a green marble from a bag containing 3 green, 7 yellow, and 1 white marble?

    -The probability of pulling a green marble is 3/11 or approximately 27%, calculated by dividing the number of green marbles by the total number of marbles.

  • How can you determine if the probabilities of different outcomes are calculated correctly?

    -You can determine if the probabilities are calculated correctly by checking if the sum of the probabilities of all possible outcomes equals 1 or 100%.

Outlines
00:00
🔄 Introduction to Probability

In this introductory paragraph, Rob from Math Antics explains the concept of probability, which deals with the likelihood of events that are not always certain. He contrasts this with the predictability of basic arithmetic operations like addition and multiplication. Rob uses the example of a coin toss to illustrate how probability measures the chance of an event occurring. He explains that with a fair coin, the probability of getting heads or tails is equal, each being one-half. He introduces the Probability Line, a scale from 0 to 1, where 0 means an event is impossible and 1 means it is certain. He also mentions that probabilities can be expressed as fractions, decimals, or percentages.

05:04
🎲 Understanding Dice Rolls and Probability

This paragraph delves deeper into probability with the example of rolling a standard six-sided die. Rob clarifies that while the probability of rolling a specific number like 3 might seem to be 50%, the actual probability is 1/6 or about 16.7%, due to the six possible outcomes. He emphasizes that the probability of any single outcome is the same for all numbers on the die. Rob also discusses the concept of trials and experiments in probability, explaining that conducting more trials increases the likelihood of results aligning with expected probabilities. He demonstrates this by conducting multiple dice rolls and observing the distribution of outcomes.

10:04
🌈 Probabilities with a Spinner and Marbles

In this paragraph, Rob explores the probabilities of events using a spinner with 16 equally sized sectors and a bag of marbles with different colors. He explains how to calculate the probability of landing on a specific number or color by dividing the number of favorable outcomes by the total number of possible outcomes. For example, the probability of spinning a blue sector is 5/16 or about 31%, while spinning a yellow sector is 11/16 or about 69%. Rob also discusses the probability of drawing a marble of a specific color from a bag, demonstrating how to calculate these probabilities using fractions. He concludes by reinforcing the importance of understanding the relationship between the number of favorable outcomes and the total number of outcomes in determining probabilities.

Mindmap
Keywords
💡Probability
Probability is a measure of the likelihood that a particular event will occur. In the video, it is used to describe the chance of outcomes such as flipping a coin, rolling a die, or spinning a spinner. The concept is central to understanding how likely certain events are, and it is illustrated through examples like a fair coin toss having a 50% chance of landing heads or tails.
💡Fair Coin Toss
A fair coin toss is an example of a random event where the outcome (heads or tails) is equally likely. The video uses this to introduce the concept of probability, explaining that the probability of getting heads is one-half, the same as for tails, because a fair coin has two sides and each side is equally likely to show up.
💡Probability Line
The Probability Line is a visual tool used in the video to represent the range of probabilities from 0 (impossible) to 1 (certain). It helps illustrate how different events can have varying levels of likelihood, with the script explaining that a probability of one-half means an event is as likely to happen as not to happen.
💡Dice
Dice are used in the video to demonstrate how probability works with multiple outcomes. A standard die has six sides, each with an equal chance of landing face up. The video explains that the probability of rolling a specific number, like 3, is one-sixth, or about 16.7%, because there are six possible outcomes.
💡Trials
In the context of the video, trials refer to individual instances of an experiment, such as rolling a die or flipping a coin. The video emphasizes that while the outcome of a single trial is random, conducting many trials can help approximate the expected probabilities, illustrating the law of large numbers.
💡Expected Probabilities
Expected probabilities are the theoretical outcomes that would occur if an experiment were repeated a large number of times. The video explains that while individual trials may not reflect these probabilities, conducting more trials increases the likelihood that the results will align with the expected probabilities.
💡Spinner
A spinner is another example used in the video to discuss probability. It has multiple equally sized sectors, and the probability of landing on a specific number or color is determined by the fraction of the total sectors that correspond to that outcome. The video uses a spinner with 16 sectors to illustrate how the probability of landing on a specific number, like 12, is one-sixteenth.
💡Fraction
Fractions are used in the video to express probabilities. The numerator represents the number of favorable outcomes, and the denominator represents the total number of possible outcomes. For example, the probability of flipping heads is expressed as one-half (1/2), and the probability of rolling a 3 on a die is expressed as one-sixth (1/6).
💡Bag of Marbles
The video uses a bag of marbles with different colors to illustrate how to calculate the probability of drawing a marble of a specific color. It shows that the probability of drawing a green marble is 3/11, indicating that there are 3 green marbles out of a total of 11 marbles.
💡Random Outcome
A random outcome is the result of an event where the result cannot be predicted with certainty. The video discusses how trials, such as tossing a coin or rolling a die, have random outcomes, and the probability is used to describe the likelihood of different possible results.
💡Law of Large Numbers
While not explicitly mentioned in the script, the law of large numbers is a concept that underlies the discussion of trials and expected probabilities. It states that as the number of trials increases, the actual ratio of outcomes will converge on the expected theoretical probability. The video demonstrates this by showing that more trials lead to results that are closer to the expected probabilities.
Highlights

Introduction to the concept of probability in contrast to the certainty of traditional math operations.

Explanation of the unpredictability of real-world events like a coin toss.

Understanding that a fair coin toss has an equal probability for heads and tails.

Introduction of the Probability Line ranging from 0 (impossible) to 1 (certain).

Conversion of fractions to decimals and percentages for expressing probabilities.

Probability of rolling a 3 on a die and how it differs from a coin toss due to more outcomes.

Demonstration of dividing the probability evenly among all possible outcomes in a die roll.

Conducting trials to observe the average outcomes in probability.

The importance of conducting more trials to approach expected probabilities.

The rule that the sum of probabilities of all outcomes must equal 1 or 100%.

Using a spinner to illustrate different probabilities with more sectors.

Calculating the probability of spinning a specific color based on the number of colored sectors.

Understanding that the sum of probabilities for different outcomes on a spinner must equal 1.

Calculating the probability of drawing different colored marbles from a bag.

The method of calculating probability using fractions with the number of desired outcomes over total outcomes.

The significance of practice in mastering the concept of basic probability.

Transcripts

Browse More Related Video

What is Probability? (GMAT/GRE/CAT/Bank PO/SSC CGL) | Don't Memorise

Math Antics - What Are Percentages?

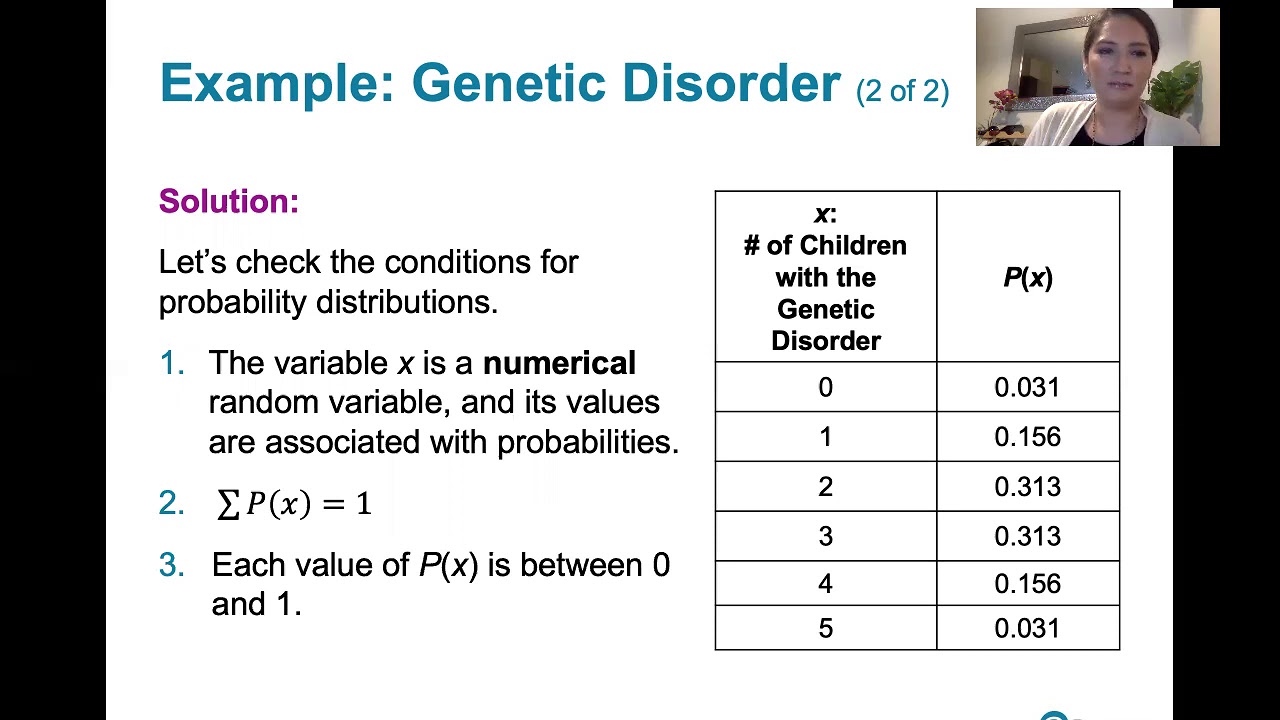
5.1.2 Discrete Probability Distributions - Probability Distributions and Probability Histograms

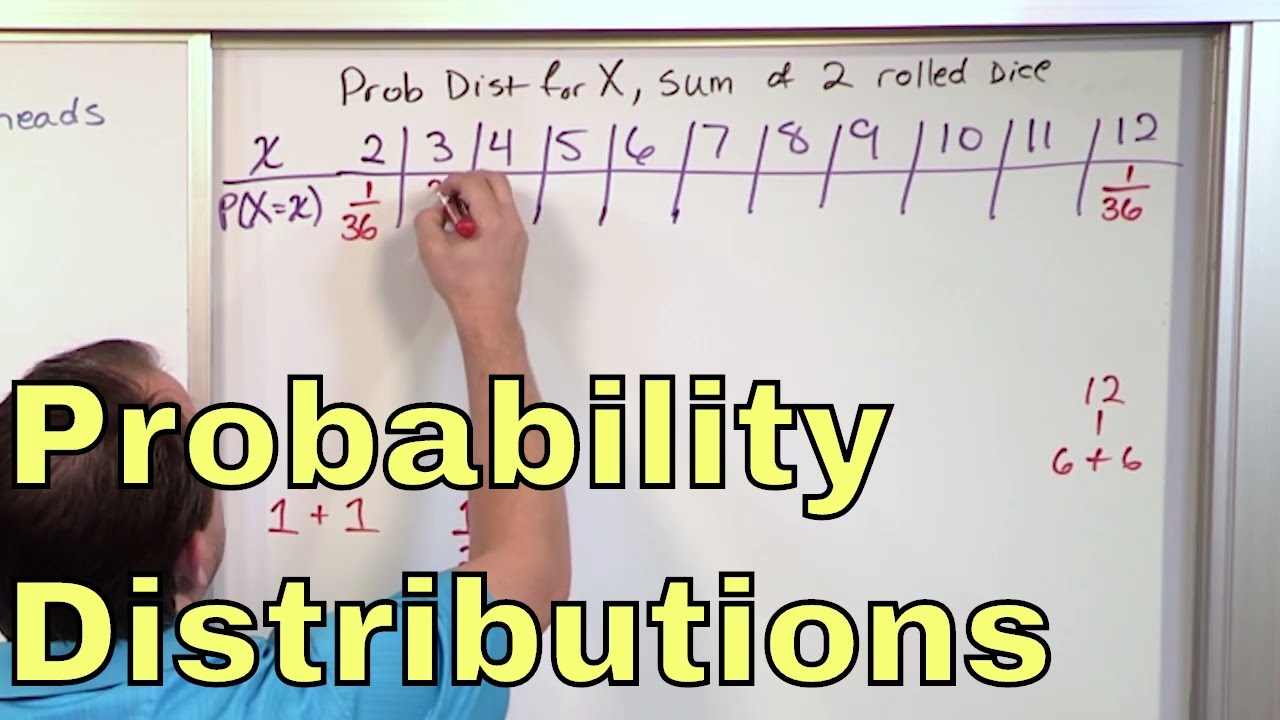
02 - Random Variables and Discrete Probability Distributions

Working With Percentages

Session 40 - Probability Distribution Functions - PDF, PMF & CDF | DSMP 2023

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Probability BasicsMath EducationRandom EventsCoin TossDice RollProbability LineExperiment TrialsSpinner GameMarble BagEducational ContentMath Antics