Lec 15 | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRThis MIT OpenCourseWare lecture introduces the concept of differentials and integrates them into the broader topic of integration. The professor explains differentials as infinitesimals and their notation, highlighting Leibniz's notation for derivatives. The lecture progresses to linear approximations, using differentials to approximate values like the cube root of 64.1. It then delves into antiderivatives and the integral notation, providing examples of integrating functions like sin(x) and x^a. The professor also discusses the uniqueness of antiderivatives and the method of substitution for more complex integrals. The session wraps up with advanced guessing for integration and emphasizes the importance of practice to solidify understanding of differentiation and integration.
Takeaways
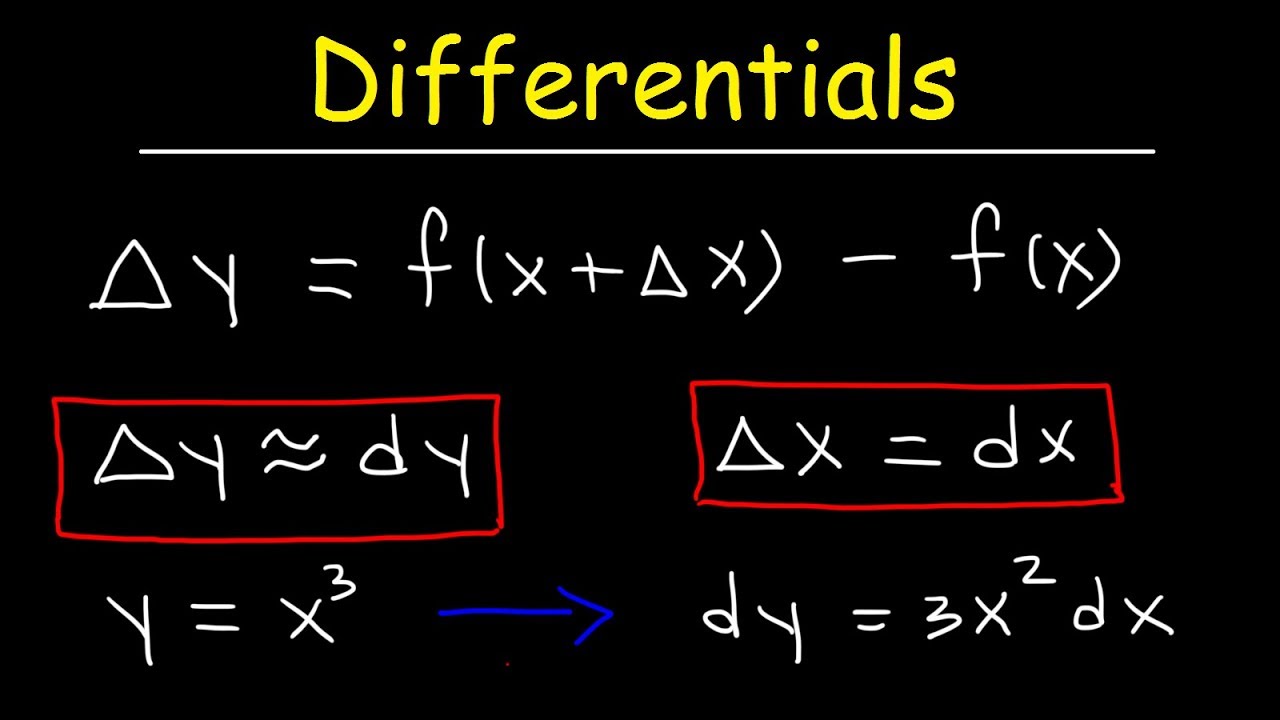
- 📚 The lecture introduces the concept of differentials as a foundational aspect of integration, denoted as dy = f'(x) dx for a function y = f(x).
- 🔢 Differentials are associated with the Leibniz interpretation of derivatives, which represents them as ratios of infinitesimals, a concept made intuitive by Leibniz and widely used in calculus.
- 📈 The importance of notations in mathematical development is highlighted, with Leibniz's notation having a significant impact on the progress of calculus, particularly in Britain.
- 📉 The lecture demonstrates the use of differentials in linear approximations, replacing delta x and delta y with dx and dy, respectively, to simplify notation and thought process.
- 🧮 An example of linear approximation is given using the function x^(1/3), illustrating the calculation of an approximate value for 64.1^(1/3) using differentials.
- 🆕 The concept of antiderivatives is introduced, which are functions that represent the integral of another function, denoted by the integral sign ∫.
- 📚 The indefinite integral is explained as an antiderivative with an arbitrary constant added, reflecting the ambiguity inherent in integration.
- 📝 Several standard integral formulas are derived and discussed, such as the integrals of sin x, x^a, and sec^2 x, emphasizing the need to remember differentiation formulas for integration.
- 🔄 The method of substitution is introduced as a technique for integrating more complex functions, demonstrated with examples and the use of differentials to simplify the process.
- ❗ The uniqueness of antiderivatives is emphasized, stating that if two functions have the same derivative, they differ by a constant, which is a fundamental principle in calculus.
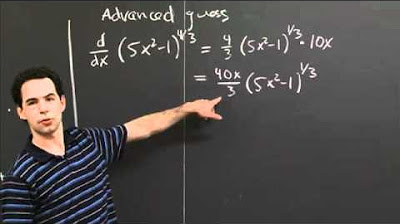
- 🎯 Advanced guessing is suggested as a method for quickly identifying integrals, where experience allows one to predict the antiderivative based on the form of the integrand.
Q & A
What is the main topic introduced in the lecture after discussing the mean value theorem?
-The main topic introduced in the lecture is integration, which will occupy the rest of the course.
What is the notation for the differential of a function y = f(x)?
-The differential of y is denoted as dy, and it is defined as f'(x) dx.
How does the Leibniz interpretation of derivatives relate to differentials?
-The Leibniz interpretation of derivatives views them as a ratio of differentials, also known as infinitesimals.
What impact did the adoption of Leibniz's notation have on the development of calculus in Britain compared to the continent?
-The adoption of Leibniz's notation on the continent led to more rapid development in calculus compared to Britain, where Newton's notation was dominant, resulting in Britain falling behind by one or two hundred years.
What is the purpose of using the differential notation dx to replace delta x?
-The purpose of using dx to replace delta x is to simplify notation and represent a small change in x more intuitively within the context of differentials.
Can you provide an example of linear approximation using the new differential notation?
-An example given in the lecture is approximating 64.1 to the 1/3 power using the differential notation, where dy = 1/3 * 64^(-2/3) dx, and calculating it to be approximately 4.002.
What is the significance of the integral sign in the context of antiderivatives?
-The integral sign represents the process of integrating a function to find its antiderivative, which is a function whose derivative is the original function.
Why is the antiderivative of a function considered indefinite?
-The antiderivative is considered indefinite because it can differ by a constant value, meaning there is an ambiguity up to a constant when finding the antiderivative of a function.
What is the method of substitution used for when integrating?
-The method of substitution is used for integrating complex functions by defining a new function that simplifies the integral, allowing for easier computation.
How does the concept of 'advanced guessing' assist in solving integrals?
-Advanced guessing is a technique where, based on experience and pattern recognition, one can anticipate the form of the antiderivative and verify it by differentiation, streamlining the integration process.
What is the uniqueness theorem for antiderivatives and what does it imply?
-The uniqueness theorem for antiderivatives states that if two functions have the same derivative, then they are equal up to a constant. This means that while antiderivatives are unique up to a constant, they represent the same family of functions.
Can you give an example of how two seemingly different integral solutions can actually be the same?
-The example provided in the lecture is the integral of sin x cos x dx, which can be solved as 1/2 sin^2 x + c_1 or -1/2 cos^2 x + c_2. The two solutions are the same because the constants c_1 and c_2 differ by 1/2.
Outlines
📚 Introduction to Integration and Differentials
The professor begins by transitioning from theoretical aspects of calculus, specifically the mean value theorem, to the practical application of integration. A new notation is introduced: differentials, denoted as 'dy' for a function 'y = f(x)', which is defined as 'f'(x) dx. This notation is attributed to Leibniz and is considered more effective than Newton's notation, with historical implications suggesting it contributed to the British lag in calculus development. The concept of infinitesimals is touched upon, and the differential dy is likened to the change in y, or delta y, with dx replacing delta x. The lecture aims to familiarize students with this notation, which will be prevalent throughout the course.
🔍 Linear Approximations and Differential Notation
The professor illustrates the use of differentials with linear approximations, a concept previously covered using delta x and delta y. By introducing 'dx' as a small change in x and 'dy' as the corresponding change in y, the lecture simplifies the notation. An example calculation is performed to approximate 64.1^(1/3) using the function x^(1/3) and its differential, resulting in an approximate value of 4.002. The lecture emphasizes that while the notation has changed, the underlying mathematical principles remain consistent, highlighting the efficiency of Leibniz's notation in mathematical communication.
📈 Exploring Antiderivatives and Integral Notation
The lecture delves into the concept of antiderivatives, introducing the integral sign and the process of indefinite integration. The professor explains that the integral of a function g(x) with respect to dx results in an antiderivative, G(x), which is a function whose derivative is g(x). Several examples of integrals are provided, including the integral of sin x dx, which is -cos x + c, and the integral of x^a dx, which is (x^(a+1))/(a+1) + c, with a notable exception when a = -1. The lecture also addresses the ambiguity of antiderivatives due to the addition of an arbitrary constant 'c', emphasizing that this is the only source of ambiguity.
📘 Understanding the Indefinite Integral and Special Cases
The professor continues to explore the concept of indefinite integrals, providing examples and highlighting special cases. The integral of dx/x is discussed, leading to the natural logarithm function, ln x, plus an arbitrary constant. The importance of considering both positive and negative values of x is noted, with the derivative of ln|x| for negative x being 1/x. Additional examples include the integral of sec^2 x dx, which is tan x + c, and the integral of √(1 - x^2) dx, which is sin^(-1) x + c. The lecture reinforces the idea that the antiderivative is unique only up to an arbitrary constant, a fundamental principle in calculus.
🔢 The Fundamental Theorem of Calculus and Its Implications
The professor emphasizes the importance of the fundamental theorem of calculus, which states that if the derivative of one function equals the derivative of another, then the two functions are identical up to an arbitrary constant. This theorem is crucial for the validity of calculus, as it ensures that the rate of change of a function determines the function itself, up to a constant. The lecture provides a proof for this theorem, highlighting its foundational role in calculus and its implications for the study of differential equations.
🧩 Advanced Integration Techniques: Substitution and Advanced Guessing
The professor introduces advanced techniques for integration, focusing on the method of substitution, which is particularly suited for complex integrals. An example is given where the integral of x^3 times (x^4 + 2)^5 dx is simplified by letting u = x^4 + 2, thus transforming the integral into a more manageable form. The lecture also introduces 'advanced guessing', a technique where one can predict the antiderivative based on experience and knowledge of differentiation formulas. Examples include integrals of e^(6x) dx and x e^(-x^2) dx, where the correct antiderivative is guessed and verified by differentiation.
🎯 The Uniqueness of Antiderivatives and Trigonometric Identities
The lecture discusses the uniqueness of antiderivatives and the use of trigonometric identities to verify different forms of the same integral. The professor presents the integral of sin x cos x dx and shows that it can be represented as either 1/2 sin^2 x + c or -1/2 cos^2 x + c, highlighting that the two forms are equivalent due to the Pythagorean identity sin^2 x + cos^2 x = 1. The importance of understanding that different-looking formulas can represent the same family of functions, differing only by a constant, is emphasized.
📝 Concluding with Logarithmic Integration and Upcoming Topics
In the final part of the lecture, the professor tackles an integral involving a natural logarithm, ln x, by using the substitution method. The integral of 1/ln x dx is simplified by letting u = ln x, resulting in a straightforward integral of 1/u du, which integrates to ln u + c. The professor then converts back to the original variable, yielding ln(ln x) + c. The lecture concludes with a mention of upcoming topics, including differential equations and a review for an upcoming test, with a handout to be provided detailing the test contents.
Mindmap
Keywords
💡Differentials
💡Integration
💡Leibniz Interpretation
💡Linear Approximation
💡Antiderivative
💡Integral Sign
💡Substitution Method
💡Differentiation Formulas
💡Uniqueness of Antiderivatives
💡Advanced Guessing
Highlights
Introduction to integration and differentials as a new notation.
The differential of y, denoted as dy, is defined as f'(x) dx.
Differentials are used to represent infinitesimals in the Leibniz interpretation of derivatives.
The effectiveness of Leibniz's notation contributed to the advancement of calculus on the continent.
Illustration of linear approximations using differentials to replace delta x and delta y.
Example calculation: Approximating 64.1 to the 1/3 power using differentials.
The concept of antiderivative and its notation introduced.
Integration of standard functions like sin x and x^a, and the importance of constants in antiderivatives.
The uniqueness of antiderivatives up to a constant and its significance in calculus.
Method of substitution for integration, tailored for differential notation.
Integration example using substitution: x^3 (x^4 + 2)^5 dx.
Advanced guessing technique for integration to foresee the result of integration.
Example of advanced guessing in integration: e^(6x) dx.
Differentiation between various antiderivatives and their underlying constants.
Integration of trigonometric functions and the use of trigonometric identities.
Substitution method applied to integrate ln x and the resulting antiderivative.
Upcoming topics on differential equations and review for the test.
Transcripts
Browse More Related Video

Unit II: Lec 2 | MIT Calculus Revisited: Single Variable Calculus

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems
Differentials and Derivatives - Local Linearization
Antidifferentiation by substitution | MIT 18.01SC Single Variable Calculus, Fall 2010

BusCalc 13.2 Integration by Substitution
Integration Using u-Substitution
5.0 / 5 (0 votes)
Thanks for rating: