Logarithms
TLDRThe video script guides viewers through solving a complex logarithmic expression: log base 16 of 27, times log base 36 of 32, times log base 9 of 216. It introduces the change of base formula, allowing conversion of any base to a common base, typically base 10. The script then demonstrates simplifying the expression by converting numbers into their prime factors and applying logarithmic properties to reduce the expression to simpler terms. The final step involves canceling out common logarithms and multiplying the remaining coefficients, resulting in the simplified answer of 2 13/16. This educational content effectively teaches the application of logarithmic properties and simplification techniques.
Takeaways
- 📚 The problem involves calculating the value of a logarithmic expression: log base 16 of 27 times log base 36 of 32 times log base 9 of 216.
- 🔍 The change of base formula is introduced: log base a of b equals log b divided by log a, with a new base c, which can be any number.
- 📐 The script demonstrates the change of base formula with an example: log base 3 of 4 can be rewritten as log 4 divided by log 3 using base 10.
- ✅ The formula is applied to each part of the expression: log base 16 of 27 becomes log 27 / log 16, and similarly for the other parts.
- 🔢 The script suggests converting larger numbers into smaller numbers by expressing them as powers of smaller bases: e.g., 27 as 3^3, 16 as 2^4, 32 as 2^5, etc.
- 💡 Another logarithmic property is explained: exponents can be moved in front of the log, such as log (5^3) becoming 3 log 5.
- 📉 The expression is simplified by converting exponents to coefficients and then canceling out common logs: 3 log 3 / 4 log 2 * 5 log 2 / 2 * log 6 * 3 log 6 / 2 log 3.
- 🧩 After canceling, the remaining expression is simplified to a multiplication of coefficients: 3/4 * 5/2 * 3/2.
- 📈 The coefficients are multiplied to get the final numerical answer: 9 * 45 / 16, which simplifies to 405 / 16.
- 📝 The final answer is converted to a mixed number: 2 and 13/16, which is the result of the original logarithmic expression.
Q & A
What is the original expression given in the video script?
-The original expression given is log base 16 of 27, times log base 36 of 32, times log base 9 of 216.
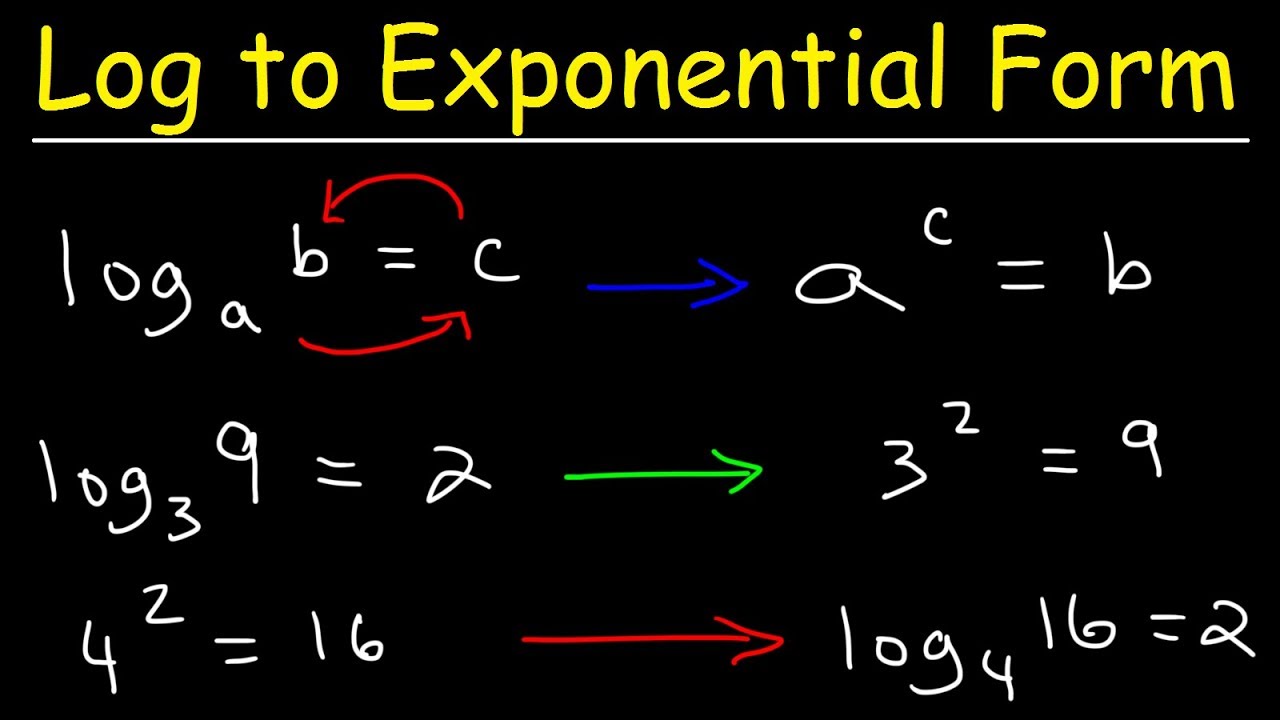
What is the change of base formula in logarithms?
-The change of base formula states that log base a of b is equal to log b divided by log a, where the new base is c (which can be any number).
Why is base 10 often used when applying the change of base formula?
-Base 10 is often used because if no base number is specified, it's always assumed to be base ten, and log base ten of a number is the same as the number itself.
How can the expression log base 16 of 27 be rewritten using the change of base formula?
-It can be rewritten as log 27 divided by log 16.
What property of logarithms allows you to move the exponent to the front?
-The property is that log base a of b^c is equal to c times log base a of b.
How can the number 27 be expressed as a power of a smaller base?
-27 can be expressed as 3 raised to the third power (3^3).
What is the value of 32 when expressed as a power of 2?
-32 is 2 raised to the fifth power (2^5).
What is the simplified form of the expression after applying the change of base formula and converting larger numbers into smaller numbers?
-The simplified form is (3 log 3 / 4 log 2) * (5 log 2 / 2 log 6) * (3 log 6 / 2 log 3).
What logarithms can be cancelled out in the simplified expression?
-Logarithms that can be cancelled out are log 3, log 2, and log 6.
What is the final answer for the original expression after simplification?
-The final answer is 2 13/16, which is a mixed number obtained by simplifying the improper fraction 45/16.
Outlines
📚 Logarithmic Expression Simplification
This paragraph introduces a complex logarithmic expression and sets the stage for a step-by-step simplification process. It begins by posing a question regarding the value of a specific logarithmic expression involving different bases and numbers. The video encourages viewers to attempt the problem before revealing the solution. The speaker then introduces the change of base formula, which is essential for simplifying the expression. The formula is explained through an example, emphasizing that the new base can be any number, with base ten being a common choice due to its convenience. The paragraph concludes with the application of the change of base formula to the given expression, transforming it into a more manageable form.
🔍 Simplifying Logarithms Using Properties
In this paragraph, the speaker continues the simplification process by converting the numbers within the logarithmic expression into smaller, more manageable numbers using exponentiation. For example, 27 is rewritten as 3 cubed, and 16 as 2 to the fourth power. The paragraph also introduces another logarithmic property that allows the exponent to be brought in front of the logarithm, transforming the expression further. The speaker then simplifies the expression by canceling out common logarithmic terms, leaving only the coefficients. The final step involves multiplying these coefficients to find the numerical value of the expression, which is then converted into a mixed number for clarity. The paragraph concludes with the final answer to the original problem, demonstrating the utility of logarithmic properties in simplifying complex expressions.
Mindmap
Keywords
💡Logarithm
💡Change of Base Formula
💡Base
💡Exponentiation
💡Logarithmic Properties
💡Coefficients
💡Simplification
💡Mixed Number
💡Multiplication and Division
💡Power of a Number
Highlights
Introduction of a mathematical problem involving logarithms with different bases.
Explanation of the change of base formula for logarithms.
Demonstration of converting a logarithmic expression using the change of base formula.
Use of base 10 as the default logarithmic base.
Conversion of numbers into powers of smaller numbers for simplification.
Application of logarithmic properties to simplify the expression.
Transformation of exponents into coefficients in logarithmic expressions.
Cancellation of common logarithmic terms to simplify the expression.
Multiplication of coefficients to find the numerical value of the expression.
Conversion of an improper fraction to a mixed number.
Final answer presentation as a mixed number.
Emphasis on the practical application of logarithmic properties.
Illustration of step-by-step problem-solving in logarithms.
Highlighting the importance of choosing an appropriate base for simplification.
Encouragement for viewers to pause and work on the problem independently.
Explanation of how to use the change of base formula and logarithmic properties to simplify expressions.
Conclusion and thanks for watching, summarizing the educational content.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: