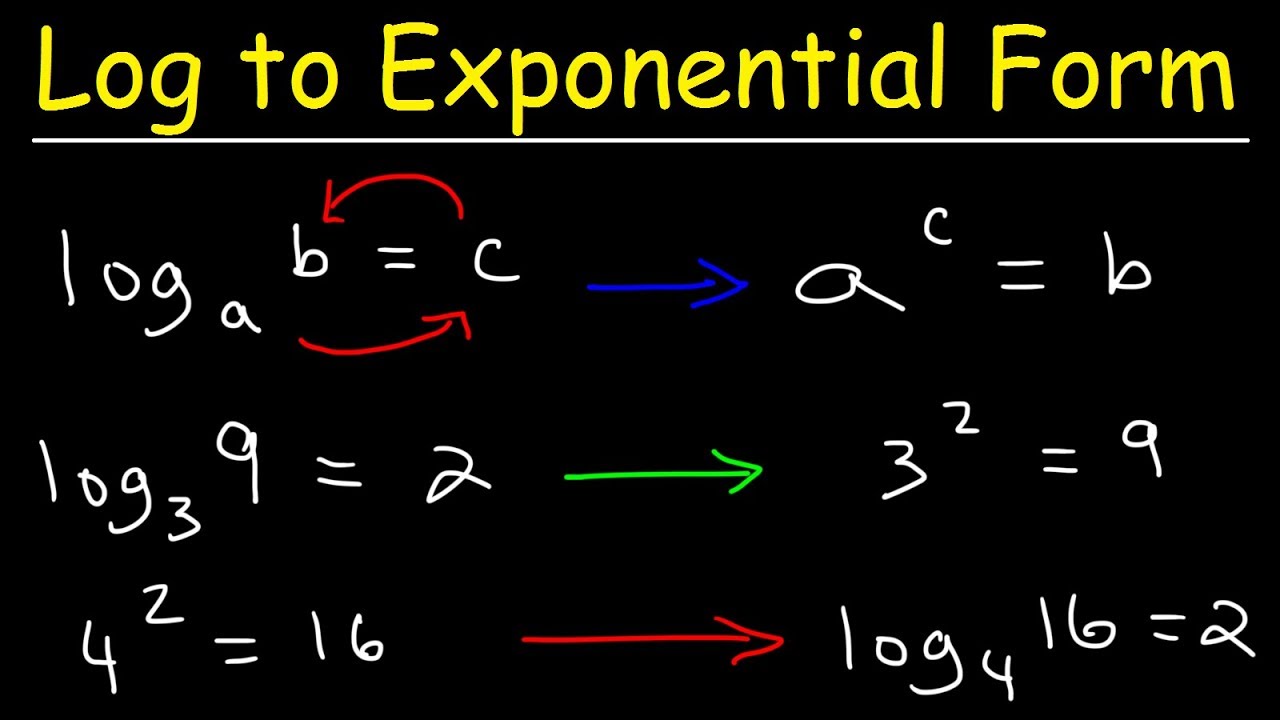
Exponential Logarithmic Equations
TLDRThis video script guides viewers through solving a logarithmic equation step by step. It begins by introducing a complex equation involving logarithms with different bases and exponents. The presenter simplifies the equation using logarithmic properties, such as changing the base and exponent manipulation. They demonstrate how to simplify expressions like 4 raised to the log base 4 of 5 to 5 and how to handle the equation when bases don't match, by changing 16 into base 4. The script then walks through the process of expanding and factoring the equation, leading to two potential solutions for x. It concludes by testing the solutions, discarding x=0 due to the presence of a logarithm with a negative argument, and confirming that x=3 is the correct solution, as it satisfies the original equation.
Takeaways
- 📚 The equation to solve is \(16^{\log_4(x-2)} + 10\log_{10}(x+1) - 4\log_4(5) = 0\).
- 🔍 An important logarithmic property is that \(a^{\log_a(b)} = b\), which simplifies calculations.
- 👉 Simplifying \(4^{\log_4(5)}\) results in \(5\) because of the logarithmic property mentioned above.
- 🔢 When the base of a logarithm is not specified, it is assumed to be base ten, so \(\log_{10}(x+1)\) simplifies to \(x+1\).
- 🧩 The number 16 can be rewritten as \(4^2\) to match the base of the logarithm in the equation.
- 📐 The expression \(4^2\) raised to the power of \(\log_4(x-2)\) can be simplified by changing the base to match the exponent, resulting in \(x-2\).
- 🔗 Understanding exponent rules helps in simplifying expressions like \(x^{12}\) to \((x^4)^3\) or \((x^3)^4\).
- ✂️ By swapping exponents and bases, the expression can be rewritten as \(4^{\log_4(x-2)^2}\), which simplifies to \(x-2\) squared.
- 📉 The equation simplifies to \(x^2 - 3x - 1 = 0\) after expanding and combining like terms.
- 🔑 Factoring out the greatest common factor gives \(x(x-3) = 0\), leading to potential solutions of \(x = 0\) or \(x = 3\).
- ❌ The solution \(x = 0\) is not valid because it would result in a logarithm of a negative number, which is undefined.
- ✔️ Upon testing, \(x = 3\) is confirmed as the correct solution because it satisfies the original equation.
Q & A
What is the original equation presented in the script?
-The original equation is \(16^{\log_4(x - 2)} + 10\log_{10}(x + 1) - 4\log_4(5) = 0\).
Why is \(7^{\log_7(9)}\) equal to 9?
-It is equal to 9 because by the property of logarithms, the base raised to the power of its logarithm is the argument itself. So, \(7^{\log_7(9)} = 9\).
How can we simplify \(4^{\log_4(5)}\)?
-We can simplify it to 5, because by the property of logarithms, the base raised to the power of its logarithm is the argument, so \(4^{\log_4(5)} = 5\).
What is the simplified form of \(10\log_{10}(x + 1)\)?
-The simplified form is \(x + 1\), since \(\log_{10}(x + 1)\) is simply \(x + 1\) when the base is 10.
How can we express 16 in terms of base 4?
-We can express 16 as \(4^2\), because four squared (4 * 4) equals sixteen.
What is the property of exponents that allows us to change \((4^2)^{\log_4(x - 2)}\) to \(4^{\log_4(x - 2) \cdot 2}\)?
-The property is that when raising a power to another power, you multiply the exponents, so \(a^{(m \cdot n)} = (a^m)^n\).
How do we simplify the expression \(x - 2\) squared?
-We can expand it as \((x - 2)(x - 2) = x^2 - 4x + 4\).
What is the greatest common factor (GCF) of \(x^2 - 4x + 4\) and \(x + 1\)?
-The GCF is \(x\), as we can factor out \(x\) from both terms to get \(x(x - 3)\).
Why is x = 0 not a valid solution to the equation?
-x = 0 is not valid because it would result in a logarithm of a negative number, which is undefined.
How do we verify that x = 3 is a correct solution?
-By substituting x = 3 back into the original equation and checking if both sides are equal. If they are, then it is a correct solution.
What is the final solution for the equation after verifying the possible values of x?
-The final solution is x = 3, as it is the only value that satisfies the original equation without resulting in any undefined operations.
What is the significance of changing the base of logarithms in this problem?
-Changing the base of logarithms helps simplify the equation by making the coefficients and the operations more manageable, and it can also help in applying properties of logarithms more effectively.
Outlines
📚 Logarithm Equation Simplification
This paragraph introduces a complex logarithmic equation and provides a step-by-step guide on how to simplify it. The equation involves logarithms with different bases and exponents. The speaker begins by explaining a fundamental logarithm property, where the base raised to the power of the logarithm equals the argument of the logarithm. This is illustrated with the example of 7 raised to the log base 7 of 9, which simplifies to 9. The speaker then applies this property to simplify parts of the given equation, such as reducing 4 raised to the log base 4 of 5 to just 5. The base 10 logarithm is also simplified to x plus 1. The process continues with converting 16 into base 4 and simplifying the equation further by manipulating exponents and bases, eventually leading to a quadratic expression in terms of x.
🔍 Solving the Simplified Logarithmic Equation
In this paragraph, the focus is on solving the simplified equation derived from the previous step. The equation is factored to isolate x, resulting in two potential solutions: x equals zero or three. However, the solution x equals zero is discarded because it would result in a logarithm of a negative number, which is undefined. The remaining solution, x equals three, is tested by substituting it back into the original equation. The calculations confirm that this solution satisfies the equation, as the left side equals the right side, validating that x equals three is the correct answer to the problem.
Mindmap
Keywords
💡Equation
💡Logarithm
💡Base
💡Exponent
💡Simplification
💡Change of Base Formula
💡Property of Logarithms
💡Factoring
💡Greatest Common Factor (GCF)
💡Solution
💡Validation
Highlights
The equation to be solved is 16^(log4(x-2)) + 10*log(x) + 1 - 4*log4(5) = 0.
A property of logarithms is introduced: log_b(a^c) = c*log_b(a).
Demonstration that 7^(log7(9)) simplifies to 9 using logarithm properties.
Explanation of the change of base formula for logarithms.
Simplification of 4^(log4(5)) to 5 using logarithm properties.
Clarification that if no base is shown, it is assumed to be base ten.
Transformation of 16 to 4^2 to match the base of the logarithm.
Exposition on raising a power to another power and how exponents are multiplied in such cases.
Rearrangement of the equation to simplify 4^2^(log4(x-2)) to 4^(log4(x-2))^2.
Cancellation of the base 4 in the expression 4^(log4(x-2)) resulting in (x-2).
Expansion of (x-2)^2 and simplification to x^2 - 3x + 1 - 5.
Factoring out the greatest common factor x from the quadratic expression.
Setting each factor equal to zero to find potential solutions for x.
Elimination of x = 0 as a solution due to the presence of a logarithm.
Verification of x = 3 as a valid solution by substituting back into the original equation.
Final confirmation that x = 3 satisfies the original equation, making it the correct answer.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: