Discrete and continuous random variables | Probability and Statistics | Khan Academy
TLDRThis video script delves into the concept of random variables, distinguishing between discrete and continuous types. Discrete variables take on distinct values, such as the outcome of a coin toss (0 or 1), while continuous variables can assume any value within a range, like the mass of an animal at a zoo. Examples illustrate the difference: a student's birth year is discrete, but the exact winning time of a 100-meter dash is continuous. The script clarifies that discrete variables can have infinite but countable values, whereas continuous variables have uncountable values in an interval, highlighting the fundamental distinction between the two.
Takeaways
- 📚 Random variables come in two types: discrete and continuous.
- 🔢 Discrete random variables can take on distinct or separate values that can be counted.
- 🔍 Continuous random variables can take on any value within a range, which can be infinite.
- 🎯 An example of a discrete random variable is the outcome of a fair coin flip, which can be either heads (1) or tails (0).
- 🐘 An example of a continuous random variable is the mass of a randomly selected animal from the New Orleans zoo, which can vary infinitely.
- 📊 The distinction between discrete and continuous is based on whether the values can be counted or listed.
- 📅 The year a random student was born is a discrete random variable because it can only take on specific years.
- 🌏 The number of ants born in the universe tomorrow is a discrete random variable because it can theoretically be counted.
- ⏱ The exact winning time of the men's 100-meter dash at the 2016 Olympics is a continuous random variable due to the infinite decimal precision.
- ⌛ If the winning time is rounded to the nearest hundredth, it becomes a discrete random variable because the possible values are countable.
- 🔑 Understanding the difference between discrete and continuous random variables is crucial for statistical analysis and probability theory.
Q & A
What are the two types of random variables discussed in the script?
-The two types of random variables discussed in the script are discrete random variables and continuous random variables.
What is the defining characteristic of discrete random variables?
-Discrete random variables are characterized by the ability to take on distinct or separate values that can be counted.
How does the script define continuous random variables?
-Continuous random variables are defined as those that can take on any value in a range, which could even be infinite, such as any value in an interval.
What is an example of a discrete random variable given in the script?
-An example of a discrete random variable given in the script is a random variable X that equals 1 if a fair coin lands heads and 0 if it lands tails.
What is an example of a continuous random variable mentioned in the script?
-An example of a continuous random variable mentioned in the script is Y, which represents the mass of a random animal selected at the New Orleans zoo.
Why is the mass of a random animal at the zoo considered a continuous random variable?
-The mass of a random animal at the zoo is considered a continuous random variable because it can take on any value within a range, and there are an infinite number of possible values between any two given values (e.g., between 0.01 and 0.02).
What is another example of a discrete random variable discussed in the script?
-Another example of a discrete random variable discussed in the script is the year that a random student in the class was born, as it can take on specific discrete years like 1992, 1985, or 2001.
How does the script illustrate the concept of a random variable with infinite potential values?
-The script illustrates the concept of a random variable with infinite potential values by discussing the number of ants born tomorrow in the universe, which can be counted and thus is a discrete random variable.
What is the tricky part about determining if the exact winning time of the men's 100-meter dash at the Olympics is a discrete or continuous random variable?
-The tricky part is that while the Olympics measure times to the nearest hundredth of a second, the exact winning time of the men's 100-meter dash could theoretically take on any value within a range, making it a continuous random variable.
How does rounding the winning time of the men's 100-meter dash to the nearest hundredth change the type of random variable it represents?
-Rounding the winning time to the nearest hundredth changes it from a continuous random variable to a discrete one, as it now takes on specific, countable values like 9.56, 9.57, or 9.58 seconds.
What is a key takeaway from the script regarding the difference between discrete and continuous random variables?
-A key takeaway from the script is that discrete random variables can be counted and listed, while continuous random variables cannot due to their infinite and uncountable number of possible values within a range.
Outlines
🎲 Introduction to Discrete and Continuous Random Variables
The video script begins by explaining the concept of random variables and their two main types: discrete and continuous. Discrete random variables are characterized by taking on distinct or separate values, which can be counted. Examples given include a fair coin toss resulting in heads (1) or tails (0), clearly a discrete variable due to its limited outcomes. Continuous random variables, on the other hand, can take any value within a range, potentially infinite, such as the mass of an animal at a zoo, which can vary infinitely and is not limited to distinct values.
📊 Understanding Discrete Random Variables with Examples
This paragraph delves deeper into the concept of discrete random variables, using the year a student was born as an example. It emphasizes that discrete variables can be finite or infinite in number, but the key is that they are countable. The script contrasts this with continuous variables, which cannot be counted because they can take on an infinite number of values within a range, as illustrated with the example of the mass of animals at the zoo. The number of ants born in the universe the next day is also presented as a discrete variable because it can theoretically be counted, even though the number could be extremely large.
🏅 The Nature of Continuous Random Variables and Their Distinction
The final paragraph focuses on continuous random variables, using the exact winning time of the men's 100-meter dash at the Olympics as an example. It explains that the exact time can be any value within a range and is therefore continuous. However, if the winning time were rounded to the nearest hundredth of a second, it would become discrete, as the number of possible outcomes would be limited and countable. This distinction highlights the importance of how variables are defined and measured in determining whether they are discrete or continuous.
Mindmap
Keywords
💡Random Variables
💡Discrete Random Variables
💡Continuous Random Variables
💡Distinct Values
💡Range
💡Countable Values
💡Mass
💡Year of Birth
💡Winning Time
💡Rounding
Highlights
Random variables come in two varieties: discrete and continuous.
Discrete random variables can take on distinct or separate values.
Continuous random variables can take on any value in a range, potentially infinite.
The meaning of 'discrete' in English language is used to define discrete random variables.
Examples of random variables are given to illustrate discrete and continuous types.
A random variable X defined as 1 for heads and 0 for tails of a fair coin is discrete.
Random variable Y as the mass of a random animal from the New Orleans zoo is continuous.
Exact mass of an object can take on any set of values, making it continuous.
Random variable Y defined as the year a random student was born is discrete.
Discrete random variables can have an infinite but countable number of values.
Continuous random variables cannot have their values listed or counted.
Random variable Z as the number of ants born tomorrow in the universe is discrete.
The exact winning time for the men's 100-meter dash at the 2016 Olympics is continuous.
Rounding the winning time to the nearest hundredth makes it a discrete random variable.
The distinction between discrete and continuous random variables is based on countability of values.
Understanding the nature of random variables is crucial for statistical analysis and modeling.
Transcripts
Browse More Related Video
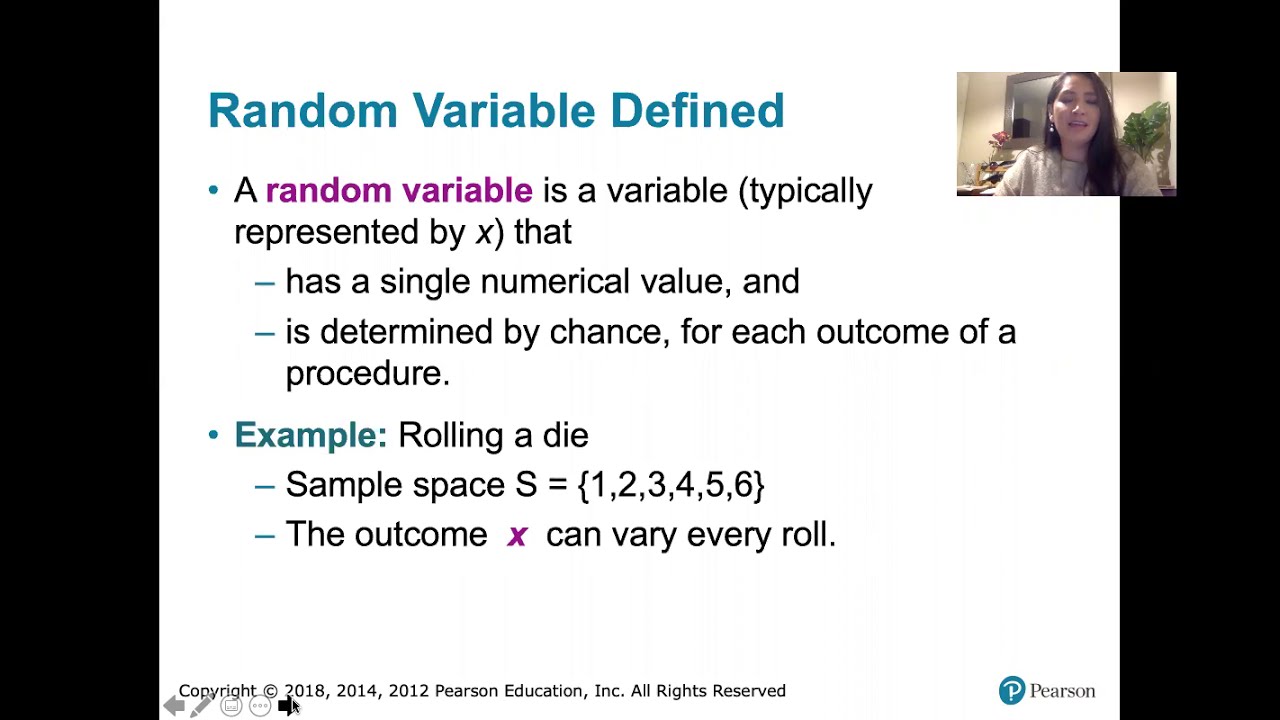
5.1.1 Discrete Probability Distributions - Discrete and Continuous Random Variables

Introduction to Discrete Random Variables and Discrete Probability Distributions
Probability density functions | Probability and Statistics | Khan Academy
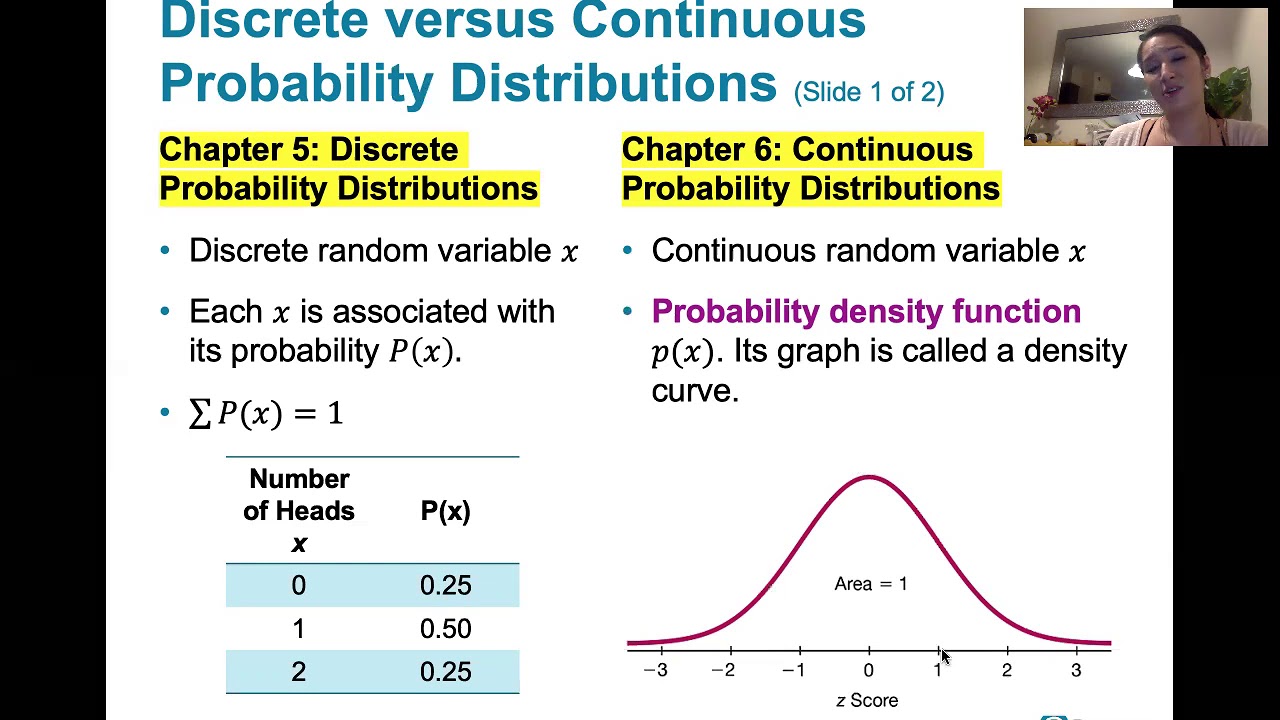
6.1.1 The Standard Normal Distribution - Discrete and Continuous Probability Distributions

Types of Data: Categorical vs Numerical Data
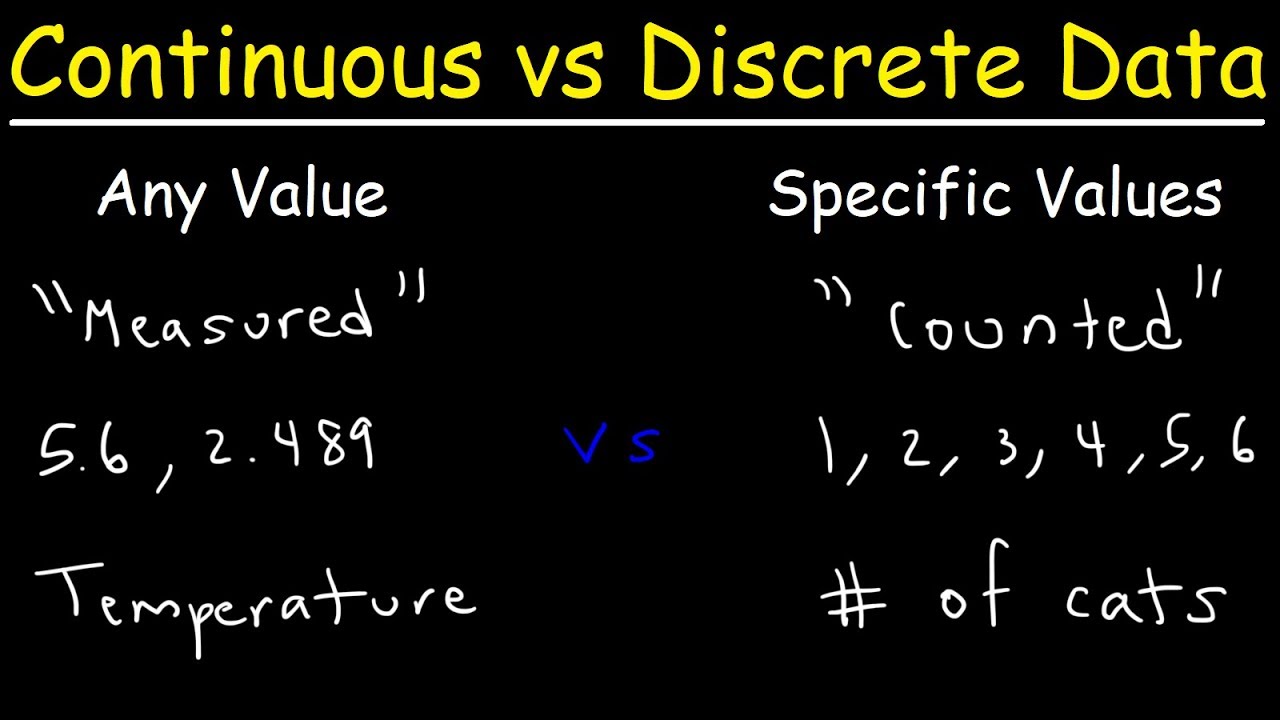
Continuous vs Discrete Data
5.0 / 5 (0 votes)
Thanks for rating: