Critical values for Confidence Intervals

MrDaveblev
10 Nov 201109:11
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial focuses on calculating critical values using the Z-alpha notation, specifically Z-alpha/2, for constructing confidence intervals. The instructor explains the concept of finding middle numbers that represent a certain percentage of data, using the normal distribution's symmetry. The process involves identifying the area to the left and right of the data's mean, which corresponds to the tails of the distribution. The inverse normal distribution function is highlighted as a key tool for converting areas into Z-scores. The video demonstrates step-by-step calculations for different confidence levels, such as 96% and 87%, and emphasizes the importance of correctly interpreting and applying the results, including handling the sign of the critical values.

Takeaways
  • πŸ“š The video discusses homework problems related to Z-scores and specifically Z_alpha/2 notation.
  • πŸ“Š The script explains the concept of a confidence interval and how it represents middle numbers in a data set.
  • πŸ” To find the middle 96% of data, the script describes a visual method of cutting off the 96% and identifying the corresponding Z-score.
  • πŸ“ The script mentions that the inverse normal distribution is used to find a Z-score when given a percentage or probability.
  • πŸ“ˆ The area to the left of a Z-score is calculated by subtracting the middle percentage from 1 and dividing by 2 to find the tail area.
  • πŸ”’ The script provides a step-by-step process to calculate the critical value for a given alpha level using the inverse normal distribution.
  • βš–οΈ The importance of symmetry in the normal distribution is highlighted, where the tails each contain 2% of the remaining 4% after accounting for the middle 96%.
  • πŸ“ The script clarifies the difference between finding the critical value for alpha/2 and alpha, with specific examples given for alpha = 0.05.
  • πŸ‘‰ The process involves using the inverse normal function on a calculator and carefully considering the sign of the result based on the context.
  • πŸ”„ The script repeats the process for different percentages (96%, 87%, and 86%) to demonstrate the method's application across various scenarios.
  • πŸ“‰ The final step is to ensure correct rounding of the calculated Z-scores and to be mindful of the context in which they are used (positive or negative).
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is about calculating critical values using the Z-alpha notation, specifically focusing on Z-alpha/2, in the context of creating confidence intervals.

  • What is a confidence interval and why is it important?

    -A confidence interval is a range of values, derived from a statistical model, that is likely to contain the value of an unknown parameter. It is important because it provides a measure of uncertainty around an estimate and is commonly used in inferential statistics.

  • What does the notation Z-alpha/2 represent?

    -The notation Z-alpha/2 represents the critical value from the standard normal distribution that corresponds to the middle of the specified confidence level, with alpha/2 in each tail of the distribution.

  • How do you find the critical value for a given confidence level?

    -To find the critical value for a given confidence level, you use the inverse normal distribution function, which takes the area to the left of the Z-score and returns the Z-score itself.

  • What is the significance of the 96% confidence interval mentioned in the script?

    -The 96% confidence interval mentioned in the script signifies that the interval will contain the true population parameter with a probability of 96%, leaving 4% in the tails of the distribution.

  • How is the area to the left of a Z-score related to the confidence interval?

    -The area to the left of a Z-score represents the probability that a random variable from a normal distribution will fall below that Z-score. In the context of a confidence interval, this area corresponds to the confidence level of the interval.

  • What is the difference between using alpha and alpha/2 in the context of critical values?

    -Alpha represents the total area in both tails outside the confidence interval, while alpha/2 is the area in just one tail. When calculating critical values, using alpha/2 is appropriate because it focuses on one side of the distribution.

  • How does the inverse normal distribution function help in finding critical values?

    -The inverse normal distribution function helps in finding critical values by allowing you to input the area to the left (or right) of the desired Z-score and it returns the actual Z-score that corresponds to that area.

  • What is the process for calculating the critical value when the confidence level is 96%?

    -To calculate the critical value for a 96% confidence interval, you would input an area to the left of 0.02 (since 96% is in the middle, leaving 4% in the tails, and half of that is 2%) into the inverse normal distribution function.

  • Can you provide an example of how to calculate the critical value for a 95% confidence interval?

    -To calculate the critical value for a 95% confidence interval, you would use the inverse normal distribution function and input an area to the left of 0.025 (since 5% is in the tails, and half of that is 2.5%).

  • What is the importance of considering symmetry when finding critical values?

    -Considering symmetry is important because the normal distribution is symmetric around the mean. This means that the critical value for the lower tail can be found by taking the negative of the critical value for the upper tail, simplifying the calculation process.

  • How does the script guide the viewer in understanding the process of finding critical values?

    -The script guides the viewer by providing a step-by-step explanation of how to use the inverse normal distribution function to find critical values for different confidence levels, including examples and the reasoning behind each step.

Outlines
00:00
πŸ“š Understanding Confidence Intervals and Z-Scores

This paragraph discusses the concept of confidence intervals in statistics, specifically focusing on the Z-score notation Z_alpha/2. The speaker explains that a confidence interval requires a starting and stopping number, which represent the middle numbers of the data set. The example given is finding the numbers that correspond to the middle 96% of the data. The speaker uses a visual aid to illustrate that 96% of the data falls between two values, with the remaining 4% split equally into two tails of 2% each. The task is to find the Z-score that corresponds to the 2% in the tails using the inverse normal distribution. The speaker provides a step-by-step guide on how to calculate this using the inverse normal function on a calculator, emphasizing the importance of considering the symmetry of the distribution and correctly interpreting the results, including taking the positive version of the Z-score when necessary.

05:00
πŸ” Calculating Critical Values with Z-Score Notation

In this paragraph, the speaker continues the discussion on calculating critical values using Z-score notation, focusing on different scenarios where the confidence level and alpha values are given or need to be derived. The speaker explains how to find the critical value Z_alpha/2 when given a specific confidence level, such as 96% or 87%, by using the inverse normal distribution and calculating the area to the left of the Z-score. The process involves converting the given percentage to an alpha value, which is the remaining percentage outside the confidence interval, and then dividing by two to find the tail area. The speaker demonstrates how to input these values into a calculator to obtain the Z-score and emphasizes the importance of taking the absolute value and considering the symmetry of the distribution when interpreting the results. The paragraph also covers the case where the alpha value is directly given, such as 0.05, and how to calculate the corresponding Z-score without the need to subtract from one.

Mindmap
Keywords
πŸ’‘Confidence Interval
A confidence interval is a range of values, derived from a data set, that is likely to contain the true value of an unknown parameter. In the context of the video, the instructor is discussing how to calculate a confidence interval using Z-scores, which are critical values that help determine the boundaries of this interval. The video mentions finding numbers that correspond to the middle 96% of data, which is part of constructing such an interval.
πŸ’‘Z-score (Z alpha)
A Z-score, also referred to as 'Z alpha' in the video, is a measure of how many standard deviations an element is from the mean. It is used in statistics to standardize scores. The video script discusses finding a Z-score that corresponds to a certain percentage of the data, which is crucial for determining the critical values needed for a confidence interval.
πŸ’‘Critical Value
Critical values are the threshold values that determine the limits of a confidence interval. The video explains how to find these values using the Z-score notation and the inverse normal distribution. The script provides examples of calculating critical values for different confidence levels, such as 96% and 87%.
πŸ’‘Inverse Normal Distribution
The inverse normal distribution is used to find Z-scores from given probabilities. It's a tool that helps in determining the Z-score when the area to the left (or right) of the score is known. In the video, the instructor explains using the inverse normal to find the Z-score that corresponds to the middle 96% of the data.
πŸ’‘Area to the Left
In the context of the video, 'area to the left' refers to the proportion of data that lies to the left of a certain Z-score on the standard normal distribution curve. The script mentions calculating the area to the left as part of the process to find the critical value for a confidence interval.
πŸ’‘Alpha (Ξ±)
Alpha is a measure used in hypothesis testing to determine the probability of making a Type I error, or the likelihood of rejecting a true null hypothesis. In the video, 'alpha over two' is used to find the critical value for the upper and lower bounds of a confidence interval. The script provides examples of how to calculate 'alpha over two' for different alpha values.
πŸ’‘Symmetric Distribution
A symmetric distribution is one where the left and right sides are mirror images of each other. The video script refers to the normal distribution as being symmetric, which is why the tails on either side of the mean each contain 2% of the data when the middle 96% is accounted for.
πŸ’‘Percentage in the Middle
The 'percentage in the middle' is the proportion of data that falls within a specified range, typically around the mean, in a distribution. In the video, the instructor is calculating confidence intervals, which require identifying the Z-score that corresponds to the middle 96% or 87% of the data.
πŸ’‘Rounding
Rounding is the process of adjusting a number to a specified level of precision. In the context of the video, the instructor emphasizes the importance of rounding the calculated critical values to two decimal places to ensure accuracy in the confidence interval.
πŸ’‘Calculator
A calculator is an electronic device used to perform mathematical calculations. The video script mentions using a calculator to input values and perform calculations related to finding critical values and Z-scores for constructing confidence intervals.
Highlights

Introduction to the homework set covering weeks 12, 13, and 14 with a focus on Z alpha notation, specifically Z alpha over two.

Explanation of confidence intervals and the need for a starting and stopping number to represent middle numbers.

Visual aid provided for understanding the concept of cutting off 96% of the data for the first problem.

Clarification on how to find the number that cuts off 96% of the data using Z-scores.

Introduction of the inverse normal distribution and its role in finding critical values.

Detailed explanation of how to calculate the area to the left of a Z-score for a given percentage.

Method to find the critical value for a 96% confidence interval using the inverse normal distribution.

Use of a calculator to find the critical value by plugging in the area left over in the tail.

Conversion of the area to the left to a decimal for calculator input.

Explanation of the symmetry in the normal distribution and its application in finding the critical value.

Process of finding the critical value for a different confidence interval, specifically 87%.

Comparison of methods for finding critical values with different confidence levels.

Instructions on how to handle the negative sign when finding the positive version of the critical value.

Different approach for problem number 2 where alpha is given directly as 0.05.

Calculation of the critical value for alpha equals 0.05 using the inverse normal distribution.

Emphasis on the importance of correct rounding when finding critical values.

Final summary of the process for finding critical values with Z alpha over two notation.

Transcripts

Browse More Related Video

Find the z-score given the confidence level

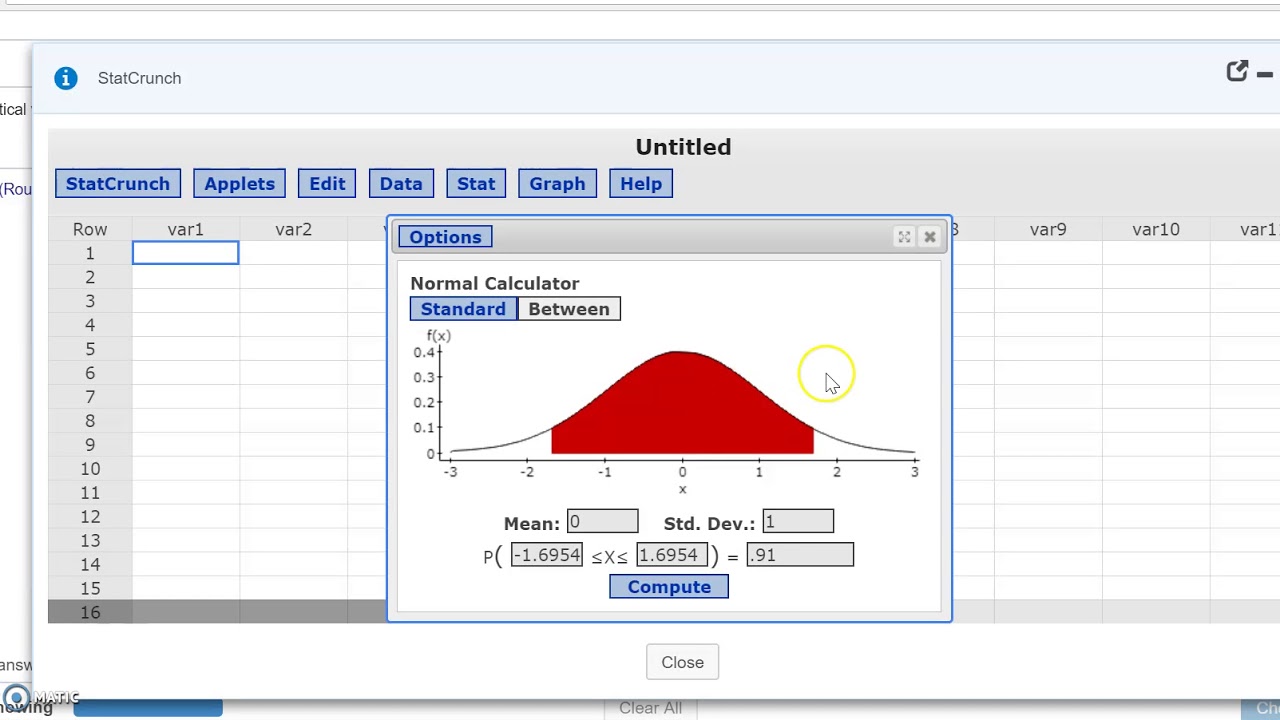
Find Critical Value Za/2 with Statcrunch

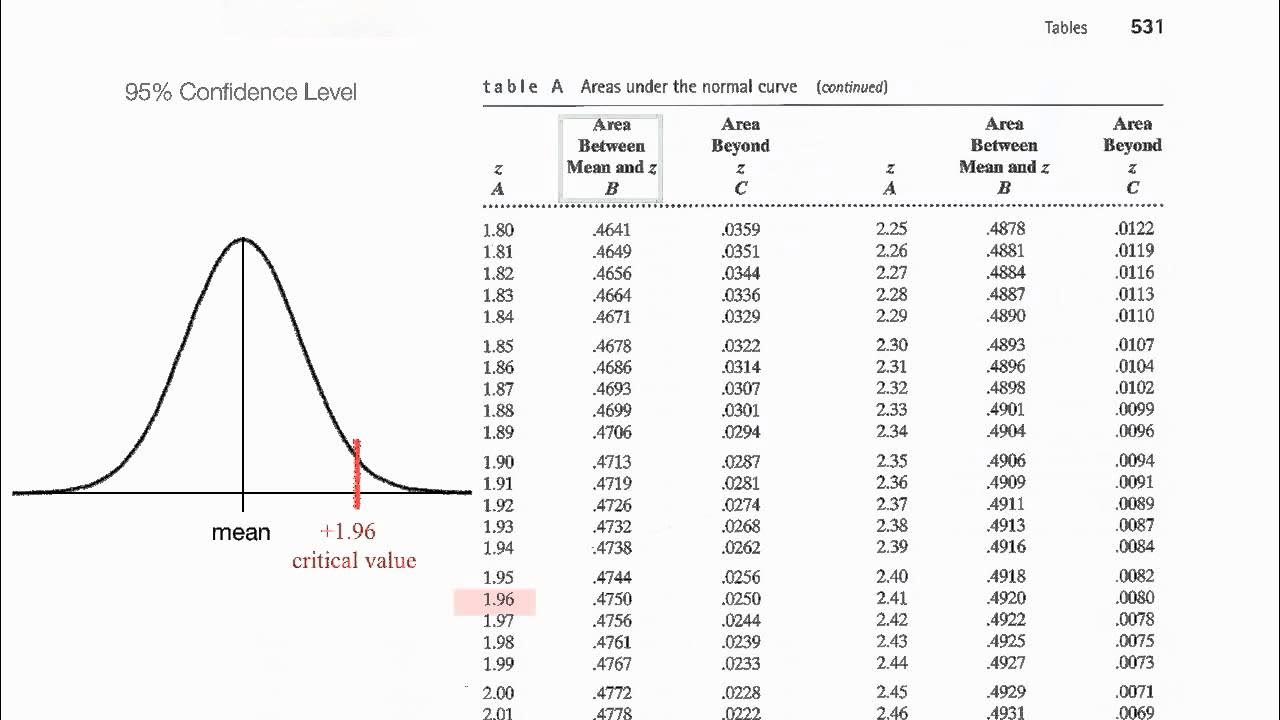
Normalized Table: Understand z scores, p values, critical values

6.1.5 Standard Normal Distribution - z scores Corresponding to Areas. Percentiles. Critical Values.

7.1.3 Estimating a Population Proportion - Critical Values, Rationale and How to Compute Them

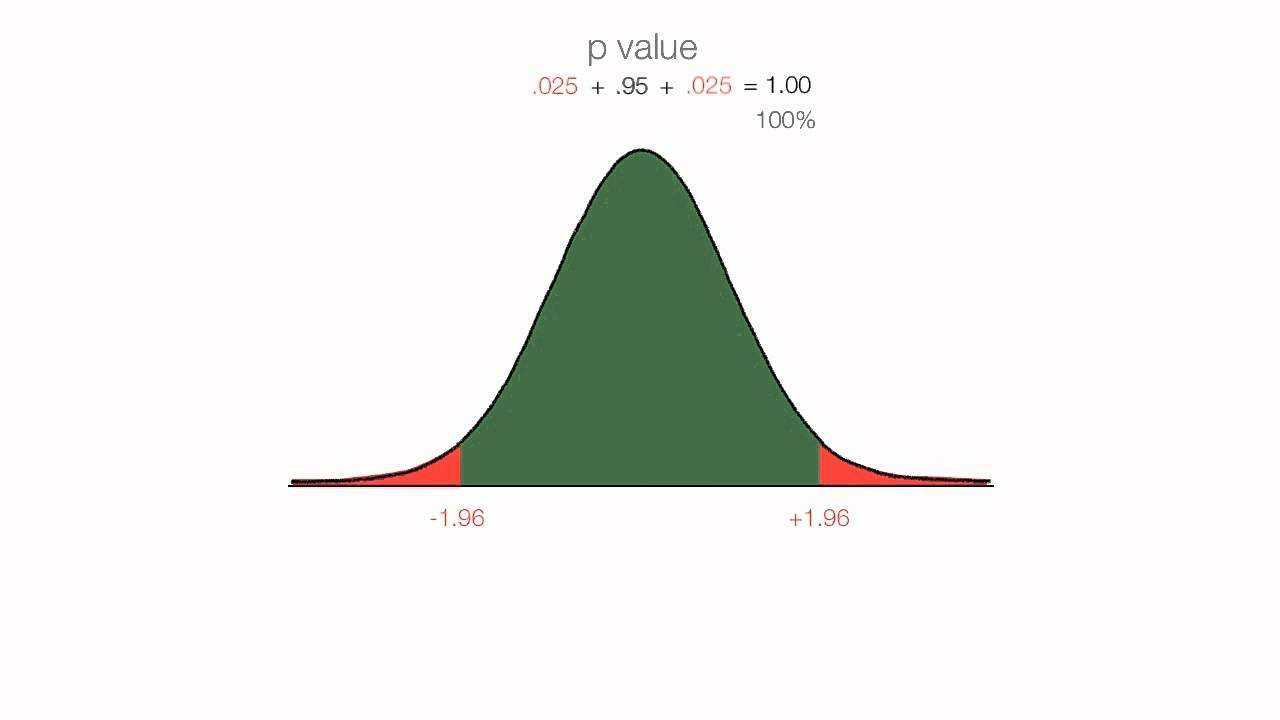
P Values, z Scores, Alpha, Critical Values

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
StatisticsConfidence IntervalsZ-scoresInverse NormalCritical ValuesCalculationEducationalHomework HelpTutorialData Analysis