Free-Body Diagrams
TLDRIn this AP Physics essentials video, Mr. Andersen explores the concept of free-body diagrams, which are diagrams depicting all the forces acting on an isolated body. He begins with a thought-provoking question about weight changes on an angled platform, then demonstrates how a free-body diagram can explain the phenomenon. The video continues with examples of various scenarios involving a car, illustrating how to correctly draw and interpret free-body diagrams to understand forces like gravity, normal force, traction, friction, and air resistance. Andersen emphasizes the importance of including only the body and external forces, avoiding common mistakes. This educational video aims to teach students how to create and use free-body diagrams to qualitatively and quantitatively solve physics problems.
Takeaways
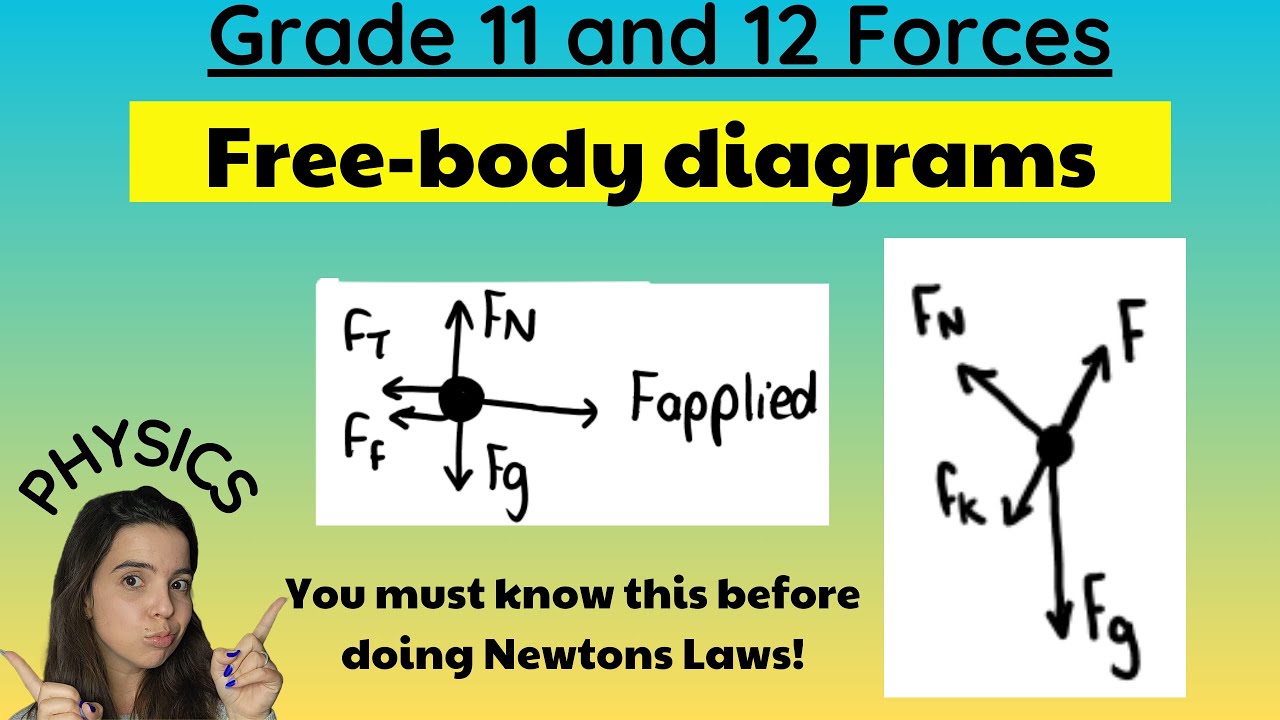
- 📐 **Free-Body Diagrams**: A diagram that represents all the forces acting on an object in a specific environment.
- 🧐 **Weight on an Angled Platform**: When a platform is angled, the weight reading decreases due to the change in the normal force and the component of gravitational force.
- 🎯 **Gravity and Normal Force**: Gravity acts vertically downward, while the normal force is the support force from the surface, acting perpendicular to the surface.
- 📈 **Breaking Down Forces**: Forces can be broken down into components parallel and perpendicular to the surface the object is on.
- 🚫 **Avoiding Common Mistakes**: Do not include internal forces or forces exerted by the body itself in a free-body diagram.
- 🔄 **Newton's Third Law**: For every action, there is an equal and opposite reaction; the ground pushes back with a traction force when the car pushes against it.
- 🔄 **Friction and Air Resistance**: These forces act opposite to the direction of motion and are important to consider in a free-body diagram.
- 📉 **No Normal Force in Free Fall**: During free fall, there is no normal force acting on the object, which is why the acceleration is downward due to gravity alone.
- 🚗 **Acceleration Direction**: The direction of acceleration is determined by the net vector sum of all forces acting on the object.
- 🔢 **Setting Up Coordinate Systems**: It's beneficial to align one axis of the coordinate system with the direction of acceleration for clarity.
- 📚 **Practical Application**: Free-body diagrams can be used both qualitatively and quantitatively to analyze and solve problems in physics.
Q & A
What is the purpose of a free-body diagram?
-A free-body diagram is used to represent all the external forces acting on an object when it is isolated from its environment. It helps in visualizing and analyzing the forces that affect the motion of the object.
What would happen to your weight if you stood on a scale and angled the platform?
-Your weight would appear to decrease as the platform is angled. This is because the normal force, which balances the component of your weight perpendicular to the platform, becomes less than your actual weight when measured vertically.
How can a free-body diagram help explain the change in weight when standing on an angled platform?
-A free-body diagram can break down your weight into two components: one perpendicular to the platform (normal force) and one parallel to the platform (force into the platform). The latter is smaller than the actual weight, which is why the scale reads a lower weight.
What is the normal force and how does it relate to the object's motion?
-The normal force is the force exerted by a surface that supports an object, acting perpendicular to the surface. It is equal and opposite to the component of the object's weight perpendicular to the surface and prevents the object from sinking into the surface.
Why is it important to set up a coordinate system when drawing a free-body diagram?
-Setting up a coordinate system helps in determining the direction of acceleration and simplifies the analysis of forces. It is beneficial to align one of the axes (either x or y) parallel to the acceleration of the object.
What are the common mistakes to avoid when drawing a free-body diagram?
-Common mistakes include including other bodies, internal forces, or forces exerted by the body itself. A free-body diagram should only include the object of interest and the external forces acting on it.
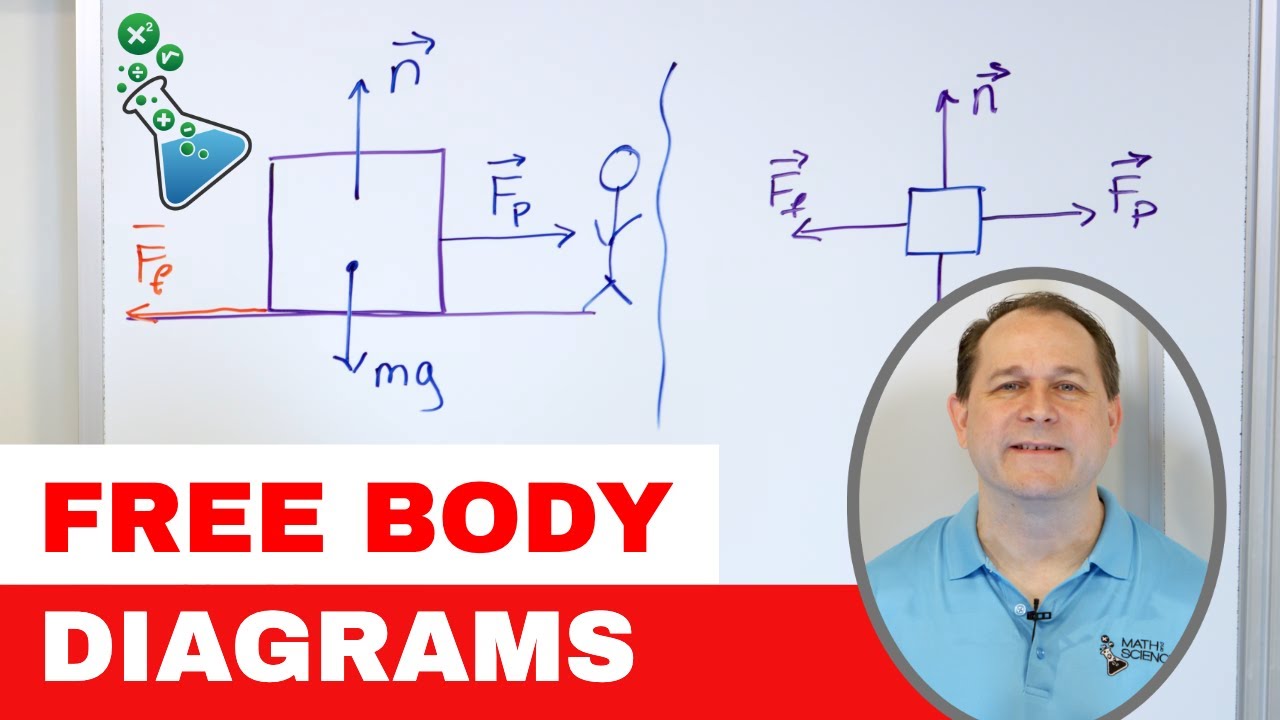
What forces act on a stationary car sitting on a horizontal platform?
-The forces acting on a stationary car on a horizontal platform are gravity (acting vertically downward) and the normal force (acting vertically upward from the platform).
How does the traction force affect a car that is accelerating to the right?
-The traction force is the force exerted by the ground on the car, pushing it to the right. This force, along with the direction of other forces, determines the car's acceleration.
What is the role of frictional forces and air resistance in the motion of a car?
-Frictional forces and air resistance oppose the motion of the car. They act in the opposite direction to the car's movement and can reduce the car's speed or cause it to decelerate.
Why is there no normal force acting on a car that is falling to Earth?
-When a car is in free fall, there is no surface beneath it to exert a normal force. The only force acting on the car is gravity, which causes it to accelerate downward.
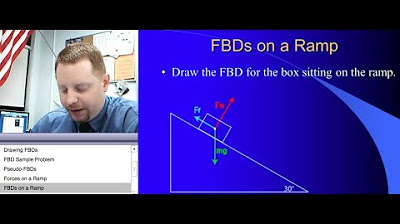
How does the angle of a ramp affect the forces acting on a car accelerating down it?
-The angle of a ramp affects the direction and magnitude of the forces acting on a car. The normal force acts perpendicular to the ramp, while gravity and frictional forces (including air resistance) combine to cause the car to accelerate down the ramp.
What is the significance of the tensional force in a scenario where a car is hooked up and motionless?
-The tensional force holds the car in place when it is motionless. It balances the component of gravity acting down the slope, ensuring that there is no net acceleration and the car remains stationary.
Outlines
📚 Introduction to Free-Body Diagrams
In this segment, Mr. Andersen introduces the concept of free-body diagrams, which are diagrams that depict all the forces acting on an object isolated from its environment. He begins with a thought experiment involving a person standing on a scale at different angles to illustrate how weight can appear to change due to the platform's angle. This leads to a discussion about the importance of understanding the forces at play, such as gravity and the normal force, through the use of a free-body diagram. The paragraph emphasizes the need to label all external forces correctly and to avoid including internal forces or other bodies in the diagram. The example of a car on a stationary platform is used to demonstrate how to properly set up a free-body diagram, highlighting the forces of gravity and the normal force, and the importance of aligning the coordinate system with the object's acceleration.
🚗 Application of Free-Body Diagrams in Various Scenarios
This paragraph delves into applying free-body diagrams to different physical situations involving a car. Mr. Andersen guides the viewer through various scenarios, such as a car accelerating to the right, a car falling to earth, and a car at the peak of its trajectory. In each case, he explains the forces involved, including gravity, normal force, traction, friction, and air resistance, and how they contribute to the car's acceleration. The importance of setting up a coordinate system that aligns with the direction of acceleration is reiterated, and viewers are encouraged to pause the video and attempt to draw the free-body diagrams themselves before checking their work against the provided examples. The paragraph concludes with a scenario of a car at rest on an incline, illustrating the forces of gravity, normal force, and tension, and emphasizing the utility of free-body diagrams in both qualitative and quantitative problem-solving.
Mindmap
Keywords
💡Free-body diagrams
💡Weight
💡Normal force
💡Gravity
💡Acceleration
💡Coordinate system
💡Traction
💡Frictional forces
💡Air resistance
💡Tensional force
Highlights
The video is about free-body diagrams, which are diagrams of a body removed from its environment with all the forces acting on it.
A thought problem is presented where the weight of an object is explored by standing on a scale on a slanted platform.
The phenomenon of weight changing with the angle of the platform is explained using free-body diagrams.
The concept of normal force, which is the force exerted by the platform holding the object in place, is introduced.
The video emphasizes the importance of setting up a coordinate system parallel to the object's acceleration for free-body diagrams.
Common mistakes in drawing free-body diagrams, such as including other bodies or internal forces, are highlighted.
The video provides an example of drawing a free-body diagram for a car not moving on a stationary platform.
The normal force is explained as always acting perpendicular to the object on which it is sitting, such as a car on a platform.
The video discusses the forces acting on a car accelerating to the right, including traction and air resistance.
The importance of including only body and external forces in free-body diagrams is emphasized.
A car falling to earth is discussed, with the explanation of gravity and the absence of a normal force causing acceleration down.
The video describes the free-body diagram for a car with air resistance, leading to a net acceleration down.
A car accelerating down a ramp is discussed, with the normal force acting down the platform and a net acceleration due to frictional forces and air resistance.
A car at the top of a ramp is explained, with the normal force acting at an angle and no net acceleration.
The video concludes with a car at rest, with gravity acting down and a normal force at an angle, resulting in no net acceleration.
The practical application of free-body diagrams is discussed, emphasizing their use in solving problems both qualitatively and quantitatively.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: