High School Physics - Free Body Diagrams

Dan Fullerton
6 Feb 201310:41
EducationalLearning
32 Likes 10 Comments

TLDRThe video script provides an insightful explanation of free body diagrams, a fundamental tool in physics for analyzing the forces acting on an object. It introduces the concept with clarity, using relatable examples such as an elephant falling off a tightrope and a glass of soda on a table. The importance of correctly identifying and labeling forces like gravity, normal force, and friction is emphasized. The video also touches on pseudo free body diagrams for situations where forces do not align with the axes, highlighting the method of breaking down forces into components. The content is engaging, informative, and well-structured, catering to users seeking to enhance their understanding of this essential physics topic.

Takeaways
  • 📚 Free body diagrams are a tool used to visualize and analyze all the forces acting on a single object.
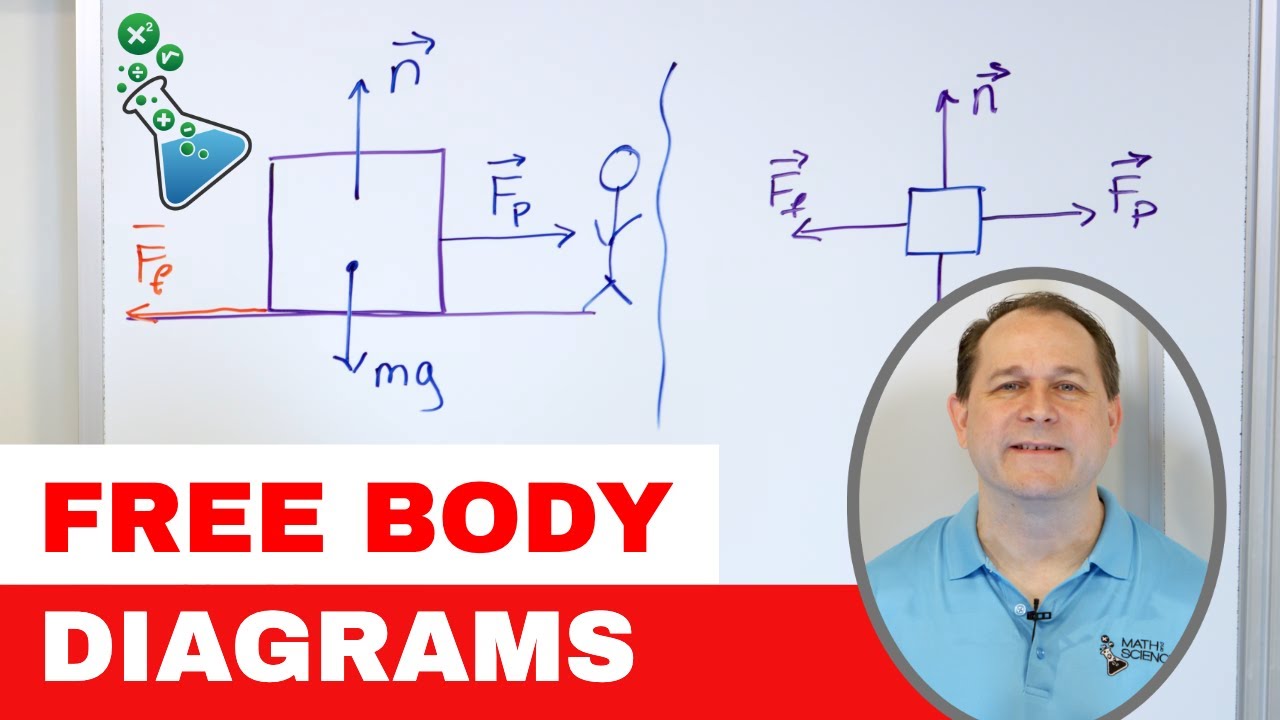
  • 🎯 To create a free body diagram, start by drawing the object of interest as a dot and then label and draw all the external forces acting on it.
  • 📈 When drawing forces, it's common to choose a coordinate system with the direction of the object's motion as one of the positive axes.
  • 🐘 Example: An elephant falling off a tightrope is subject to the force of gravity (mg), which can be represented as the mass of the elephant times the gravitational field strength.
  • 🌬️ Including air resistance in the analysis introduces an additional force that opposes the motion of the falling elephant, acting in the opposite direction of gravity.
  • 🥤 Another example: A glass of soda on a table is subject to the force of gravity (mg) pulling it down and a normal force (Fn) from the table pushing it up.
  • 🔍 To determine if an object is in equilibrium, ensure that the sum of all forces acting on it is balanced, resulting in a net force of zero.
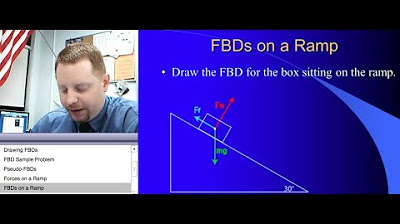
  • 🏗️ When dealing with objects on inclined planes, consider the force of gravity, normal force, and friction force, and how they relate to the object's motion or potential motion.
  • 📐 Pseudo free body diagrams are used when the forces do not align with the chosen axes, breaking forces into components that do align with the axes.
  • 🚫 When preparing a free body diagram for an AP class, always draw the forces first and then a separate diagram for the components if needed, not just the pseudo free body diagram.
  • 🔄 Remember to never label a force as 'centripetal' on a free body diagram, as it is a resultant effect of other forces, such as tension or friction.
Q & A

  • What is the primary purpose of a free body diagram?

    -The primary purpose of a free body diagram is to visually represent all the forces acting on a single object, which helps in analyzing a physical situation more easily.

  • How is an object typically represented in a free body diagram?

    -An object is typically represented as a dot in a free body diagram, indicating that no advanced artwork skills are needed to create these diagrams.

  • What are the steps to create a basic free body diagram?

    -To create a basic free body diagram, start by choosing the object of interest and drawing it as a dot. Then, label and draw all the external forces acting on that object. Finally, sketch a coordinate system with the object's motion direction as one of the positive axes.

  • How is the force of gravity typically denoted in a free body diagram?

    -The force of gravity is typically denoted as mg, where m is the mass of the object and g is the gravitational field strength. This notation helps avoid confusion with other meanings of the symbol W, such as weight, work, or watts.

  • What is a pseudo free body diagram and when is it used?

    -A pseudo free body diagram is used when the forces acting on an object do not align with the chosen axes. It involves breaking those forces into components that do align with the axes, making the analysis easier.

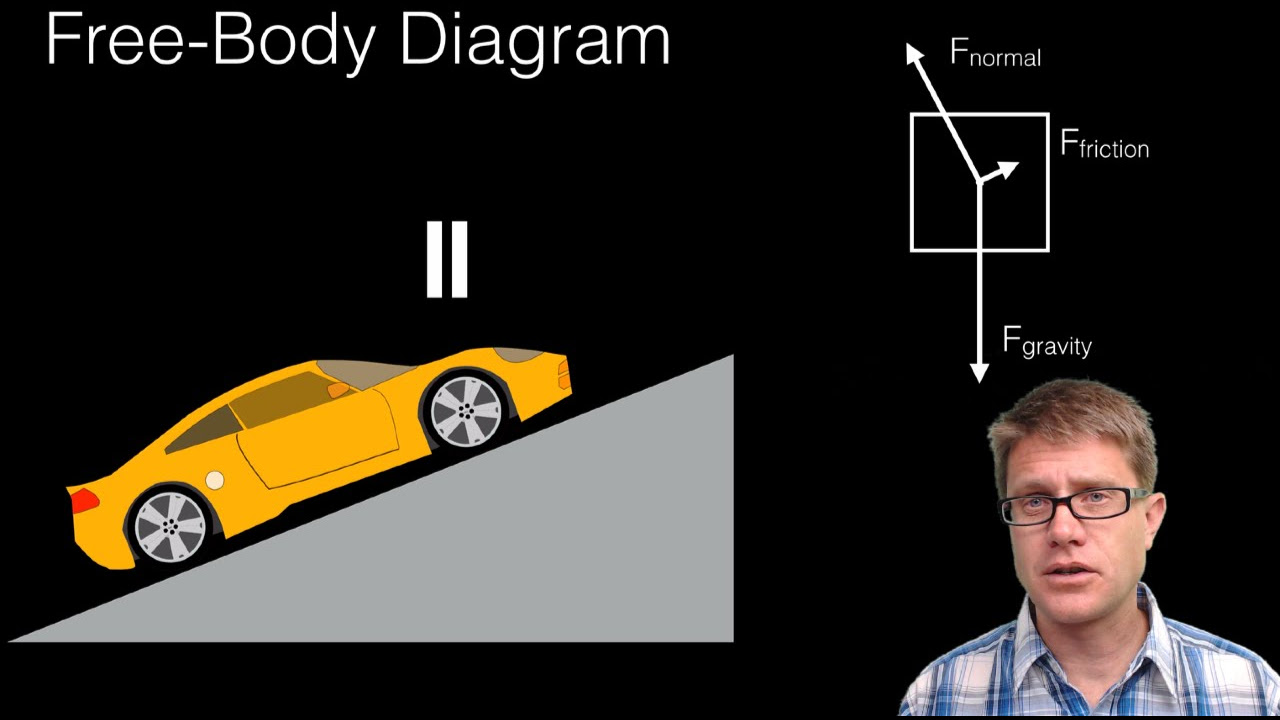
  • The normal force is the force exerted by a surface that supports an object. It is represented in a free body diagram as an upward force perpendicular to the surface, often denoted as FN or n.

    -null

  • How can you determine if an object is in equilibrium according to a free body diagram?

    -An object is in equilibrium if the sum of all forces acting on it is zero, meaning there is no net force. In a free body diagram, this would be represented by balanced forces in both the vertical and horizontal directions.

  • What is the force of friction and how does it relate to an object's motion?

    -The force of friction is a resistive force that opposes the motion of an object. It acts in the opposite direction to the motion or potential motion of the object and is represented in a free body diagram as an arrow with an appropriate label, such as f or Ff.

  • How can you analyze a situation involving an object on an inclined plane using a free body diagram?

    -To analyze an object on an inclined plane using a free body diagram, you would represent the force of gravity acting downward, the normal force perpendicular to the surface, and the force of friction acting up the ramp if the object is not sliding down. These forces must be balanced for the object to remain stationary on the ramp.

  • What is the significance of breaking forces into components in a pseudo free body diagram?

    -Breaking forces into components in a pseudo free body diagram allows for a more straightforward application of Newton's second law and other physical principles. It helps in resolving forces along the coordinate axes, simplifying calculations and analysis.

  • Why is it important to avoid labeling forces as centripetal on a free body diagram?

    -It is important to avoid labeling forces as centripetal on a free body diagram because centripetal force is not an actual force but rather the result of other forces such as tension or friction acting towards the center of a circular path. Labeling a force as centripetal could lead to confusion and incorrect analysis.

Outlines
00:00
📚 Introduction to Free Body Diagrams

This paragraph introduces the concept of free body diagrams, which are visual tools used to represent all the forces acting on a single object, facilitating the analysis of physical situations. Mr. Fullerton explains the basic steps to create a free body diagram, including selecting the object of interest, drawing it as a dot, labeling all external forces, and sketching a coordinate system with the object's motion direction as one of the positive axes. An example is provided using an elephant falling off a tightrope to illustrate how to draw a simple free body diagram, neglecting air resistance, and focusing on the force of gravity acting on the elephant.

05:00
📐 Analyzing Forces and Pseudo Free Body Diagrams

In this paragraph, Mr. Fullerton delves deeper into the analysis of forces using free body diagrams. He discusses how to handle situations where forces do not align with the chosen axes by introducing the concept of pseudo free body diagrams, which involve breaking down forces into components that do align with the axes. The paragraph provides examples, including an elephant with air resistance and a glass of soda on a table, to demonstrate the labeling of forces such as gravity, normal force, and air resistance. It also explains how to apply these concepts to problems involving equilibrium, where all forces on an object are balanced, and how to identify the correct free body diagram among given options.

10:01
🔍 Advanced Applications and Tips for Free Body Diagrams

The final paragraph focuses on the advanced application of free body diagrams and offers valuable tips. Mr. Fullerton explains how to deal with forces that do not align with the axes by creating a pseudo free body diagram, breaking down the forces into components along the axes using geometry and trigonometry. The example of a box on a ramp is used to illustrate this process, highlighting the identification of forces such as gravity, normal force, and friction. The paragraph concludes with a reminder to never label forces as centripetal on a free body diagram, as centripetal force is actually caused by another force like tension or friction. Additionally, viewers are directed to aplusphysics.com for further assistance, and Mr. Fullerton wishes everyone a great day.

Mindmap
Keywords
💡Free Body Diagrams
Free Body Diagrams are graphical representations that illustrate all the forces acting on a single object, simplifying the analysis of physical situations. In the video, Mr. Fullerton uses the example of an elephant falling off a tightrope to demonstrate how to draw and interpret such diagrams, highlighting their importance in understanding the forces at play in a scenario, such as gravity and air resistance.
💡Forces
Forces are interactions that, when unbalanced, can change the motion of an object. In the context of the video, various forces like gravity, air resistance, and normal force are discussed. For instance, gravity pulls the elephant down when it falls, while air resistance acts in opposition to the elephant's motion through the air.
💡Gravity
Gravity is the force that attracts two bodies towards each other, often exemplified by the Earth's pull on objects. In the video, gravity is the primary force acting on the elephant as it falls, represented as mg (mass times the gravitational field strength), and is directed downwards.
💡Pseudo Free Body Diagrams
Pseudo Free Body Diagrams are a variation of standard Free Body Diagrams where forces not aligned with the chosen axes are broken down into components. This technique is introduced in the video when discussing a box on a ramp, where gravity's force is resolved into components parallel and perpendicular to the ramp's surface to facilitate analysis.
💡Components
In the context of forces, components refer to the individual parts of a force that align with specific axes. The video explains how to resolve the force of gravity into two components when dealing with inclined planes, such as a ramp, which helps in applying Newton's laws for analysis.
💡Normal Force
The normal force is the reaction force exerted by a surface on an object in contact with it, perpendicular to the surface. In the video, the normal force is what prevents the glass of soda from passing through the table, acting upwards to balance the force of gravity acting downwards on the glass.
💡Friction
Friction is a force that opposes the relative motion or tendency of such motion of two surfaces in contact. The video discusses friction as the force that prevents the box from sliding down the ramp, acting upwards along the ramp to counteract the component of gravity parallel to the ramp's surface.
💡Equilibrium
Equilibrium refers to a state where the net force on an object is zero, meaning all forces acting on the object are balanced. The video uses the example of a box in equilibrium to illustrate how the upward forces balance the downward forces, resulting in no acceleration or movement of the box.
💡Analysis
Analysis in the context of the video involves breaking down physical situations to understand the forces at play. Mr. Fullerton emphasizes the importance of analysis when using Free Body Diagrams to determine the effects of various forces, such as gravity and friction, on an object's motion.
💡Newton's Laws
Newton's Laws are fundamental principles that describe the relationship between a body and the forces acting upon it, and its motion in response to those forces. Although not explicitly detailed in the video, the mention of applying Newton's second law implies that the analysis of forces using Free Body Diagrams is to determine the resultant motion according to these laws.
💡Centripetal Force
Centripetal force is the force that keeps an object moving in a circular path, directed towards the center of the circle. While not a main focus of the video, Mr. Fullerton cautions against labeling forces as centripetal on Free Body Diagrams, as it is not a force in itself but rather the result of other forces like tension or friction acting towards the center of the circular path.
Highlights

Free body diagrams are a tool to show all forces acting on an object and simplify physical situation analysis.

A free body diagram represents the object of interest as a dot, simplifying the drawing process.

External forces acting on the object are drawn and labeled on the diagram.

A coordinate system is sketched, typically aligning the object's motion with one of the positive axes.

The force of gravity is represented as mg (mass times gravitational field strength) for simplicity and to avoid confusion with other symbols.

Air resistance is a force that opposes the motion of an object and acts in the opposite direction of the object's movement.

The normal force is the reaction force from the surface on which an object rests, always perpendicular to the surface.

Free body diagrams can be used to analyze equilibrium situations where all forces on an object are balanced.

In a balanced situation, the net force on the object is zero, both vertically and horizontally.

When forces do not align with the axes, a pseudo free body diagram can be used to break these forces into components.

For AP classes, it is important to draw the forces first and then make a separate diagram for the components to avoid losing points.

The component of gravity parallel to the direction of motion is calculated as mg sine theta, and the perpendicular component as mg cosine theta.

Free body diagrams show forces, not components of forces, except in a pseudo free body diagram.

Avoid labeling forces on a free body diagram as centripetal force, as it is an effect of other forces such as tension or friction.

The lecture provides practical examples, such as an elephant falling off a tightrope and a glass of soda on a table, to illustrate the application of free body diagrams.

For objects on inclined planes, the force of friction opposes the motion of the object and acts in the opposite direction of the intended motion.

In a pseudo free body diagram, forces are decomposed into components that line up with the coordinate axes, facilitating the application of Newton's second law.

The lecture emphasizes the importance of correctly labeling and representing forces in free body diagrams for accurate physical analysis.

Transcripts

Browse More Related Video

High School Physics - Ramps and Inclines

Free-Body Diagrams

2022 Live Review 2 | AP Physics 1 | Understanding Forces and Newton’s Laws

Breaking down forces for free body diagrams | AP Physics 1 | Khan Academy

AP® Physics 1: Forces and Newton's Laws (Unit 2)

Master Free-Body Diagrams for Physics Problems - [1-5-18]

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationForce AnalysisFree Body DiagramsMechanicsProblem SolvingTeaching MethodLearn PhysicsConcept ClarificationMotional AnalysisFrictional Force