What the Second Derivative Tells Us
TLDRThis educational video script delves into the significance of the second derivative in calculus, explaining its role as the rate of change of the first derivative. The presenter uses the example of y=2x^2 to illustrate how the first derivative (2x) indicates the function's increasing or decreasing nature. The second derivative's positivity or negativity reveals the concavity of a graph—concave up when positive and concave down when negative. The script also touches on points of inflection, where the second derivative equals zero, and their relation to changes in concavity. It briefly mentions the third derivative, humorously referred to as the 'jerk,' but focuses on the importance of the first and second derivatives in understanding a function's behavior.
Takeaways
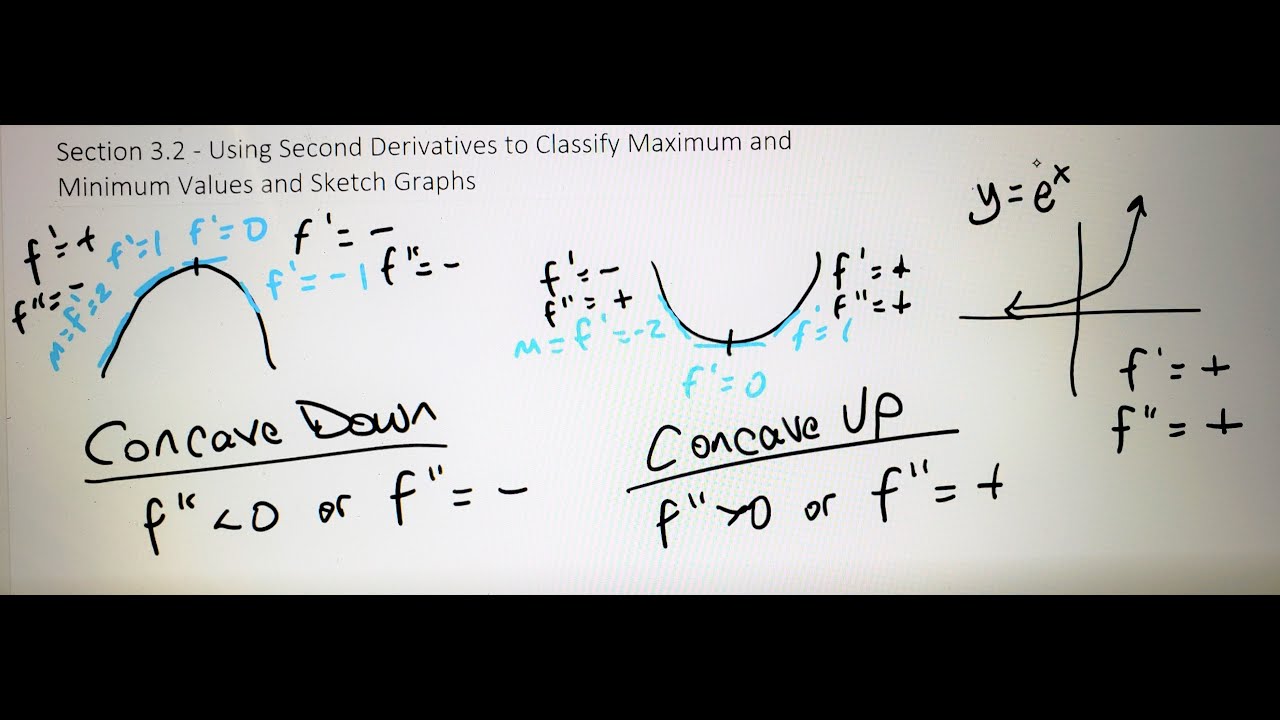
- 📚 The second derivative represents the rate of change of the first derivative, providing insight into the concavity of a graph.
- 📈 In the example of y = 2x^2, the first derivative (2x) indicates the function's increasing or decreasing nature based on the sign of x.
- 📉 The slopes of the tangent lines to the graph change from large negative to large positive, suggesting the concavity of the graph.
- 🔍 A positive second derivative indicates that the slopes of the tangent lines are always increasing, which corresponds to a graph that is concave up.
- 🔎 Conversely, a negative second derivative means the graph is concave down, as the slopes of the tangent lines are decreasing.
- 🤔 The second derivative being zero at a point is known as a point of inflection, where the concavity of the graph may change.
- 📊 For the function y = x^3 - 9x, the second derivative (6x) is positive when x > 0, indicating concave up, and negative when x < 0, indicating concave down.
- 🔑 The point of inflection is where the second derivative equals zero, and it often, but not always, coincides with a change in concavity.
- 🤷♂️ There are exceptions to the rules about the first and second derivatives indicating maximum or minimum points without additional analysis.
- 🚗 The third derivative, mentioned only briefly, is sometimes referred to as the 'jerk' and indicates points of significant change in the graph's curvature, though it's not a primary focus in this script.
- 📚 The script emphasizes the importance of the first and second derivatives for analyzing the behavior of a function, particularly in relation to concavity and points of inflection.
Q & A
What does the second derivative tell us?
-The second derivative is the rate of change of the first derivative and it describes the concavity of the graph.
What example is used to explain the second derivative?
-The function y = 2x^2 is used to explain the second derivative.
How does the first derivative behave for y = 2x^2?
-The first derivative, which is 2x, is negative when x is negative (indicating a decreasing slope) and positive when x is positive (indicating an increasing slope).
What is indicated by the tangent lines as they move across the graph of y = 2x^2?
-The tangent lines become less steep as they approach the vertex, flatten at the vertex where the derivative is zero, and then become steeper again as they move away.
What does it mean if the second derivative is always positive?
-If the second derivative is always positive, it means the graph is concave up, indicating that the slope values are increasing.
What happens when the second derivative is negative?
-When the second derivative is negative, the graph is concave down, meaning the slope values are decreasing.
What is a point of inflection?
-A point of inflection is where the second derivative equals zero, indicating a change in the concavity of the graph.
What function is used to illustrate the point of inflection?
-The function y = x^3 - 9x is used to illustrate the point of inflection.
How is the concavity determined for the function y = x^3 - 9x?
-The concavity is determined by the second derivative, which is 6x. The graph is concave up when x is positive and concave down when x is negative.
What is the significance of the third derivative?
-The third derivative, mentioned briefly, is referred to as the 'jerk' point, where the car would jerk the most. However, its practical importance is not emphasized in this script.
Outlines
📚 Understanding the Second Derivative
This paragraph delves into the concept of the second derivative as the rate of change of the first derivative. It uses the example of the function y = 2x^2, a basic parabola, to illustrate how the first derivative (2x) indicates whether the function is increasing or decreasing. The explanation continues with how the second derivative, which is always positive for this function, indicates that the slope of the tangent lines is continually increasing, suggesting the graph is concave up. The paragraph also touches on the concept of points of inflection, where the second derivative equals zero, and briefly mentions the third derivative, humorously referring to it as the 'jerk' point.
📉 Analyzing Concavity and Points of Inflection
The second paragraph focuses on the implications of the second derivative for the concavity of a graph. It explains that a positive second derivative indicates the graph is concave up, while a negative second derivative suggests the graph is concave down. Using the function y = x^3 - 9x as an example, the paragraph demonstrates how the second derivative (6x) can be positive or negative depending on the value of x, leading to changes in concavity. The point where the second derivative is zero, x = 0 in this case, is identified as the point of inflection, where the graph changes concavity. The paragraph also discusses the relationship between the first and second derivatives at points of inflection and the potential for exceptions to the general rules.
Mindmap
Keywords
💡Second Derivative
💡First Derivative
💡Concavity
💡Point of Inflection
💡Tangent Line
💡Rate of Change
💡Parabola
💡Maximum and Minimum
💡Derivative Test
💡Graph
💡Jerk
Highlights
The second derivative represents the rate of change of the first derivative.
Example function y=2x^2 is used to explain the concept of the second derivative.
The first derivative, 2x, indicates the function's increasing and decreasing behavior.
The vertex of the parabola is where the first derivative is zero.
Tangent lines' slopes change from negative to positive as the function transitions from decreasing to increasing.
The second derivative being always positive indicates upward concavity.
A positive second derivative implies that the slope of the tangent lines is always increasing.
Concave up shape occurs when the second derivative is positive.
Concave down shape is indicated when the second derivative is negative.
The point of inflection is where the second derivative is zero.
The point of inflection marks a change in concavity.
The function y=x^3-9x is used to illustrate the point of inflection.
The second derivative of y=x^3-9x is 6x, which determines concavity.
Concavity changes at the origin in the example of y=x^3-9x.
The relationship between first and second derivatives at points of zero values.
Exceptions to the rules of concavity and points of inflection are acknowledged.
The concept of the 'jerk' point, where the third derivative is zero, is mentioned.
The importance of first and second derivatives over the third in the context of the video.
Transcripts
Browse More Related Video
Math 11 - Section 3.2

AP Calculus AB: Lesson 2.4 The Second Derivative

Calculus 1: Concavity Examples

What the Second Derivative Tells You about a Graph

Concavity introduction | Using derivatives to analyze functions | AP Calculus AB | Khan Academy

MATH1325 Lecture 10 2 - Concavity & Points of Inflection
5.0 / 5 (0 votes)
Thanks for rating: