Transforming Standard Form to General Form of Parabola | @ProfD

Prof D
5 Apr 202111:07
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, viewers are guided through the process of converting the standard form of a parabola's equation into its general form. The presenter introduces two standard forms and their respective general forms, then provides six step-by-step examples to illustrate the transformation. Each example includes distributing terms, transposing them to one side of the equation, and combining like terms to achieve the general form. The video concludes with an invitation for viewers to ask questions in the comments section, ensuring an interactive learning experience.

Takeaways
  • 📚 The video is an educational tutorial on converting the standard form of a parabola's equation to its general form.
  • 📐 It introduces two standard forms of parabola equations and their corresponding general forms, focusing on the structure of the equations.
  • 🔍 The first standard form is \(y - k = 4p(x - h)^2\) and the corresponding general form is \(y^2 + dx + ey + f = 0\).
  • 🔍 The second standard form is \(x - h = 4p(y - k)^2\) with the corresponding general form being \(x^2 + dx + ey + f = 0\).
  • 📝 The video provides step-by-step examples to demonstrate the transformation process from standard to general form.
  • 📈 Example 1 involves converting \(x^2 = 8y + 56\) to the general form, resulting in \(x^2 - 8y - 56 = 0\).
  • 📉 Example 2 shows the transformation of \(y^2 = -8(x + 7)\) to \(y^2 + 8x + 56 = 0\).
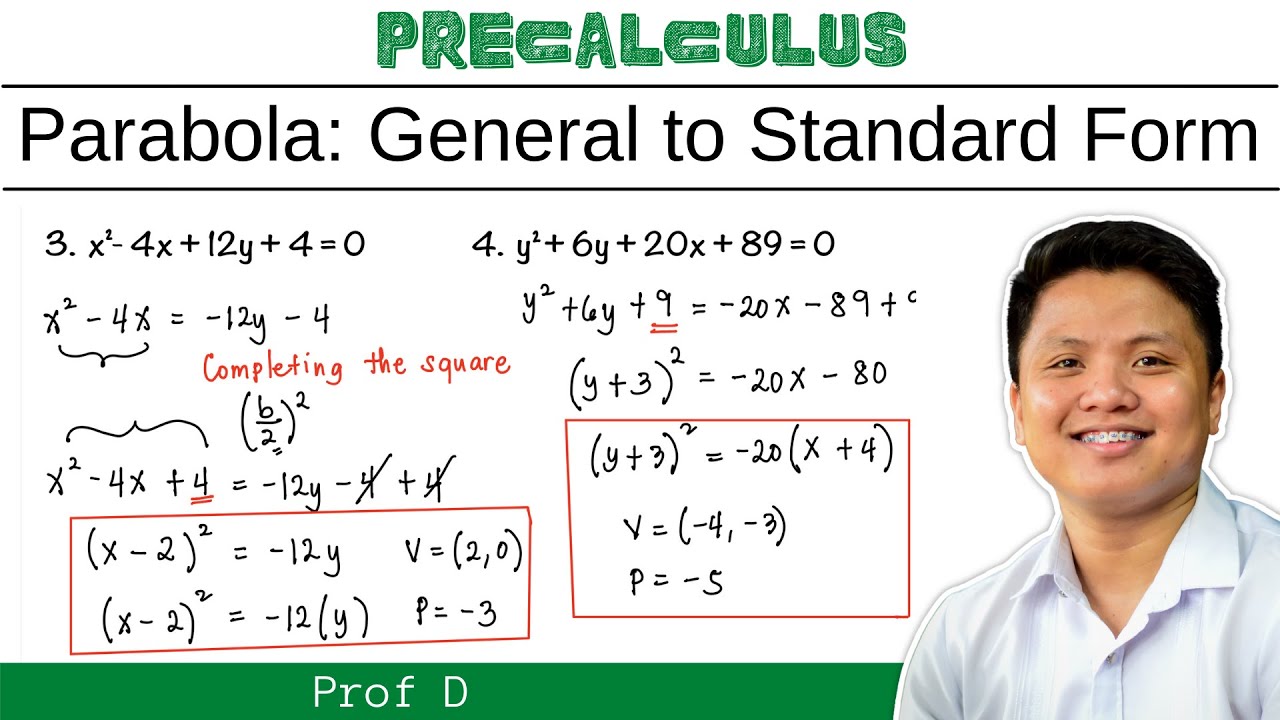
  • 📊 Example 3 explains how to convert \((x - 2)^2 = -12y\) into the general form, yielding \(x^2 - 4x + 12y + 4 = 0\).
  • ✅ Example 4 demonstrates squaring the binomial \(y + 3\) and converting it to \(y^2 + 20x + 6y + 89 = 0\).
  • 📌 Example 5 involves squaring \(y - 4\) and transforming \(y^2 - 10x - 8y + 36 = 0\).
  • 📍 The final example, number 6, squares \(x + 5\) and results in the equation \(x^2 + 10x + 4y + 17 = 0\).
  • 👨‍🏫 The video is hosted by Prof D, who encourages viewers to ask questions or seek clarifications in the comments section.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is how to transform the standard form of the equation of a parabola to its general form.

  • What are the two standard forms of the equation of a parabola mentioned in the video?

    -The two standard forms are: 1) (y - k)^2 = 4p(x - h) and 2) (x - h)^2 = 4p(y - k).

  • What is the general form of the parabola equation corresponding to the standard form (y - k)^2 = 4p(x - h)?

    -The general form corresponding to (y - k)^2 = 4p(x - h) is y^2 + dx + ey + f = 0.

  • What is the general form of the parabola equation corresponding to the standard form (x - h)^2 = 4p(y - k)?

    -The general form corresponding to (x - h)^2 = 4p(y - k) is x^2 + dx + ey + f = 0.

  • How do you transform the equation x^2 = 8(y + 7) into its general form?

    -First, distribute the terms to get x^2 = 8y + 56. Then, transpose the terms to the left side to get x^2 - 8y - 56 = 0.

  • What are the values of d, e, and f in the general form of the equation x^2 - 8y - 56 = 0?

    -In the equation x^2 - 8y - 56 = 0, the values are d = 0, e = -8, and f = -56.

  • How do you transform the equation y^2 = -8(x + 7) into its general form?

    -First, distribute the terms to get y^2 = -8x - 56. Then, transpose the terms to the left side to get y^2 + 8x + 56 = 0.

  • What are the values of d, e, and f in the general form of the equation y^2 + 8x + 56 = 0?

    -In the equation y^2 + 8x + 56 = 0, the values are d = 8, e = 0, and f = 56.

  • How do you transform the equation (x - 2)^2 = -12y into its general form?

    -First, expand the binomial to get x^2 - 4x + 4. Then, transpose -12y to the left side to get x^2 - 4x + 12y + 4 = 0.

  • What are the values of d, e, and f in the general form of the equation x^2 - 4x + 12y + 4 = 0?

    -In the equation x^2 - 4x + 12y + 4 = 0, the values are d = -4, e = 12, and f = 4.

  • How do you transform the equation (y + 3)^2 = -20(x + 4) into its general form?

    -First, expand the binomial to get y^2 + 6y + 9. Then, distribute -20 to get -20x - 80. Transpose the terms to the left side to get y^2 + 6y + 20x + 89 = 0.

  • What are the values of d, e, and f in the general form of the equation y^2 + 6y + 20x + 89 = 0?

    -In the equation y^2 + 6y + 20x + 89 = 0, the values are d = 20, e = 6, and f = 89.

  • How do you transform the equation (y - 4)^2 = 10(x - 2) into its general form?

    -First, expand the binomial to get y^2 - 8y + 16. Then, distribute 10 to get 10x - 20. Transpose the terms to the left side to get y^2 - 10x - 8y + 36 = 0.

  • What are the values of d, e, and f in the general form of the equation y^2 - 10x - 8y + 36 = 0?

    -In the equation y^2 - 10x - 8y + 36 = 0, the values are d = -10, e = -8, and f = 36.

  • How do you transform the equation (x + 5)^2 = -4(y - 2) into its general form?

    -First, expand the binomial to get x^2 + 10x + 25. Then, distribute -4 to get -4y + 8. Transpose the terms to the left side to get x^2 + 10x + 4y + 17 = 0.

  • What are the values of d, e, and f in the general form of the equation x^2 + 10x + 4y + 17 = 0?

    -In the equation x^2 + 10x + 4y + 17 = 0, the values are d = 10, e = 4, and f = 17.

Outlines
00:00
📚 Introduction to Parabola Transformation

In this educational video, the instructor begins by welcoming the viewers back to the channel and introducing the topic of transforming the standard form of a parabola's equation into its general form. Two standard forms are discussed: one with 'y - k' squared and the other with 'x - h' squared. The instructor then outlines the general form of a parabola's equation, which includes terms like 'y^2 + dx + ey + f = 0' and 'x^2 + dx + ey + f = 0'. The video proceeds with several examples to demonstrate the transformation process.

05:02
🔍 Detailed Steps for Transforming Parabola Equations

The instructor provides a step-by-step guide on transforming standard form equations of parabolas into their general form. The process involves distributing terms, transposing variables, and combining like terms to achieve the general form 'ax^2 + by + c = 0'. Each example is meticulously explained, with the instructor emphasizing the importance of correctly identifying coefficients for 'x', 'y', and the constant term 'c'. The examples range from simple quadratic equations to more complex scenarios involving binomials squared and terms multiplied by variables.

10:05
📘 Final Examples and Conclusion

The final segment of the video script includes additional examples that further illustrate the transformation process. The instructor demonstrates how to handle equations with binomials squared and terms that require distribution and transposition. Each example is carefully worked through, leading to the general form of the parabola's equation. The video concludes with an invitation for viewers to ask questions or seek clarifications in the comments section and a farewell message from the instructor, signaling the end of the lesson.

Mindmap
Keywords
💡Standard Form
The term 'Standard Form' in the context of the video refers to one of the two common representations of the equation of a parabola. It is a specific way to express the equation where the variable terms are set up in a particular pattern. In the script, the standard forms are given as 'y - k = 4p(x - h)' and 'x - h = 4p(y - k)', which are used as starting points to transform into the general form of a parabola's equation.
💡General Form
The 'General Form' is the target equation format that the video aims to achieve from the standard form. It is a more universal representation of a quadratic equation, typically written as 'Ax^2 + Bx + Cy + Dy + E = 0', where A, B, C, D, and E are coefficients. The video demonstrates how to rearrange and combine terms from the standard form equations to fit this general structure.
💡Parabola
A 'Parabola' is a type of conic section, which is a curve generated by the intersection of a plane with a double cone. In the video, the focus is on transforming the equations that represent parabolas. Parabolas have various applications in mathematics and physics, and understanding their equations is essential for solving related problems.
💡Coefficient
A 'Coefficient' is a numerical factor that multiplies a variable in an algebraic expression. In the context of the video, coefficients are the numbers that multiply the variables x and y in the equations of parabolas. The script discusses how to manipulate these coefficients to transform the standard form into the general form.
💡Distribute
To 'Distribute' in algebra means to multiply a term by each term inside a parenthesis and then add the results. In the script, the term 'distribute' is used when transforming the standard form equations, such as when '8 times (x + 7)' is expanded to '8x + 56' in one of the examples.
💡Square
The term 'Square' in the video script refers to the mathematical operation of squaring a number or a variable, which means multiplying the number or variable by itself. Squaring is used in the transformation process, as seen in the example '(x - 2)^2' which expands to 'x^2 - 4x + 4'.
💡Binomial
A 'Binomial' is an algebraic expression with two terms, typically in the form of 'a + b' or 'a - b'. In the video, binomials are squared or squared and then manipulated to fit into the general form of a parabola's equation. For example, '(x - 2)^2' is a binomial that is squared to transform the standard form equation.
💡Combine Like Terms
To 'Combine Like Terms' is to add or subtract terms in an algebraic expression that have the same variables raised to the same power. In the script, this process is used to simplify the equations after expanding binomials and distributing terms, such as combining '8y' and '6y' to get '14y' in the general form equation.
💡Transposing
Transposing in the context of algebra involves moving terms from one side of an equation to the other, typically by changing their signs. In the video, transposing is mentioned when moving terms like '10x' from the right side to the left side of the equation, changing it to '-10x' to achieve the general form.
💡Example
The 'Example' keyword in the script refers to the specific mathematical problems provided to illustrate the process of transforming standard form equations into the general form. Each example demonstrates a step-by-step approach, applying the concepts of distribution, squaring, and combining like terms to reach the desired equation format.
Highlights

Introduction to transforming standard form of parabola equations to general form.

Two standard forms of parabola equations are presented: y-k squared equals 4p(x-h) and x-h squared equals 4p(y-k).

General form equations are y^2 + dx + e*y + f = 0 and x^2 + dx + ey + f = 0 corresponding to the two standard forms.

Example one: Transforming x^2 = 8*y + 56 into general form x^2 - 8y - 56 = 0.

Example two: Transforming y^2 = -8(x + 7) into general form y^2 + 8x + 56 = 0.

Example three: Transforming (x - 2)^2 = -12y into general form x^2 - 4x + 12y + 4 = 0.

Example four: Transforming (y + 3)^2 = -20(x + 4) into general form y^2 + 20x + 6y + 89 = 0.

Example five: Transforming (y - 4)^2 = 10(x - 2) into general form y^2 - 10x - 8y + 36 = 0.

Example six: Transforming (x + 5)^2 = -4(y - 2) into general form x^2 + 10x + 4y + 17 = 0.

Explanation of the process to distribute and transpose terms in the equation.

Demonstration of how to handle binomial expansion in the transformation process.

Clarification on how to combine like terms to reach the general form equation.

Instruction on how to identify and set coefficients d, e, and f in the general form equation.

Emphasis on the importance of setting the equation to equal zero in the general form.

Illustration of the step-by-step method to transform each example equation.

Highlighting the need to adjust the signs of terms when transposing to the left side of the equation.

Providing a clear and structured approach to solving the transformation of parabola equations.

Encouragement for viewers to ask questions or seek clarifications in the comment section.

Closing remarks and sign-off by Prof D, indicating the end of the video.

Transcripts

Browse More Related Video

Transforming General Form to Standard Form of Parabola | @ProfD

How to find the equation of Hyperbola given its graph

Writing Equation of A Parabola in Standard and General Form | @ProfD

CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

STANDARD EQUATION OF ELLIPSE TO GENERAL FORM | PRE-CALCULUS

STANDARD EQUATION OF PARABOLA TO GENERAL FORM

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsEducationalAlgebraParabolaEquationsGeneral FormStandard FormTeachingTutorialMath Help