STANDARD EQUATION OF ELLIPSE TO GENERAL FORM | PRE-CALCULUS

WOW MATH
14 Sept 202113:13
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video by Markham Walmart focuses on transforming the standard equation of an ellipse into its general form. The instructor walks through three examples, demonstrating how to manipulate the equation by finding the least common denominator (LCD), multiplying both sides by the LCD, and then simplifying to achieve the general form. The process involves expanding squared binomials, combining like terms, and adjusting coefficients to match the general equation format. The video aims to help viewers understand the mathematical concepts and steps involved in converting ellipse equations, making complex topics more accessible.

Takeaways
  • 📚 The video is a lesson on transforming the standard equation of an ellipse to its general form.
  • 🔍 The process involves finding the least common denominator (LCD) and multiplying both sides of the equation by it to eliminate fractions.
  • 📐 The first example provided is an equation \( \frac{x + 3}{5} + \frac{y - 1}{4} = 1 \), which is transformed into general form by multiplying through by 100.
  • 🧩 In the first example, the equation is expanded to show the terms \( x^2, 6x, 9 \) for \( (x + 3)^2 \) and \( y^2, -2y, 1 \) for \( (y - 1)^2 \).
  • 🔢 The general form of the first equation is derived as \( 4x^2 + 24x + 25y^2 - 50y + 36 = 100 \).
  • ✅ The second example given is \( \frac{x^2}{4} + \frac{y - 2}{16} = 1 \), simplified by multiplying by 16 to get \( 4x^2 + y^2 - 4y + 4 = 16 \).
  • 📉 The third example starts with \( \frac{(x - 2)^2}{25} + \frac{(y - 5)^2}{144} = 1 \) and is transformed by multiplying by 3600.
  • 📘 The final equation from the third example is \( 144x^2 - 576x + 25y^2 - 250y + 576 + 625 = 3600 \), which simplifies to the general form.
  • 👨‍🏫 The instructor emphasizes the importance of combining like terms and correctly placing constants and variables in the general form equation.
  • 👍 The video concludes with an encouragement to like, subscribe, and hit the bell for more educational content on the Walmart Math channel.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is transforming the standard equation of an ellipse to its general form.

  • What is the first example equation given in the video?

    -The first example equation is \((x + 3)^2 / 25 + (y - 1)^2 / 4 = 1\).

  • What is the least common denominator (LCD) for the first example?

    -The least common denominator (LCD) for the first example is 100, which is derived from multiplying the denominators 25 and 4.

  • How does the video explain the process of transforming the equation to general form?

    -The video explains by multiplying both sides of the equation by the LCD, expanding the squared terms, and then combining like terms to form the general equation.

  • What is the general form of the first example equation after transformation?

    -The general form of the first example equation is \(4x^2 + 24x + 36 + 25y^2 - 50y + 25 = 100\).

  • What is the second example equation discussed in the video?

    -The second example equation is \(x^2 / 4 + (y - 2)^2 / 16 = 1\).

  • Why is there no need to multiply by the LCD in the second example?

    -There is no need to multiply by the LCD in the second example because the denominators are already powers of 2, and the LCD is already the larger denominator, which is 16.

  • What is the final step in transforming the second example to general form?

    -The final step is to combine like terms and move the constant term to the right side of the equation, resulting in \(4x^2 + y^2 - 4y + 4 = 16\).

  • What is the third example equation provided in the video?

    -The third example equation is \((x - 2)^2 / 25 + (y - 5)^2 / 144 = 1\).

  • What is the least common multiple (LCM) used for the third example?

    -The least common multiple (LCM) used for the third example is 3600, which is the product of the denominators 25 and 144.

  • What is the general form of the third example equation after transformation?

    -The general form of the third example equation is \(144x^2 - 576x + 576 + 25y^2 - 250y + 625 = 3600\).

  • What is the final result of the third example equation after simplification?

    -After simplification, the final result of the third example equation is \(144x^2 + 25y^2 - 576x - 250y + 191 = 0\).

  • What does the instructor suggest at the end of the video?

    -The instructor suggests liking, subscribing, and hitting the bell button for more video tutorials.

Outlines
00:00
📚 Transforming Ellipse Equations to General Form

This paragraph introduces the topic of converting the standard equation of an ellipse to its general form. The speaker begins by explaining the process using an example equation, where they identify the coefficients a, b, c, and d, and the LCD (Least Common Denominator). They then proceed to demonstrate the algebraic manipulation required to transform the equation, including squaring terms and combining like terms. The goal is to reach the general form of the ellipse equation, which is a more complex representation that includes squared terms and linear terms for both x and y variables.

05:09
🔍 Detailed Algebraic Steps for Ellipse Transformation

In this paragraph, the speaker continues the algebraic process of transforming the standard form of an ellipse equation into the general form. They provide a step-by-step guide on how to multiply both sides of the equation by the LCD to eliminate the denominators. The speaker then squares the binomials and combines like terms to simplify the equation. They also show how to rearrange the terms to isolate the variable terms on one side and the constant term on the other, resulting in the general form of the ellipse equation.

10:16
📘 Additional Examples of Ellipse Equation Transformation

The final paragraph presents additional examples of transforming ellipse equations into their general form. The speaker uses two more equations to illustrate the process, explaining the calculation of the LCD and the subsequent algebraic steps. They show how to multiply through by the LCD, expand the squared terms, and combine like terms to achieve the general form. The speaker emphasizes the importance of correctly identifying coefficients and performing algebraic manipulations to reach the desired form. The video concludes with an encouragement for viewers to like, subscribe, and stay tuned for more educational content.

Mindmap
Keywords
💡Transforming
Transforming in the context of the video refers to the mathematical process of converting an equation from one form to another. Specifically, it is the act of changing the standard equation of an ellipse into its general form. This is a fundamental concept in algebra and geometry, as it allows for a deeper understanding of the properties and behavior of the ellipse. For instance, the script mentions 'transforming standard equation of ellipse to general form' to illustrate the main mathematical operation being discussed.
💡Ellipse
An ellipse is a geometric shape defined as the set of all points for which the sum of the distances to two fixed points (the foci) is constant. It is a conic section, like a circle or a parabola. In the video, the ellipse is the primary subject, with the focus on how to represent its equation in different forms. The script uses the term to introduce the mathematical concept that the lesson is based upon, such as in 'discussing transforming, standard equation of ellipse to general form'.
💡Standard Equation
The standard equation of an ellipse is a specific form that describes the ellipse's position and orientation in a coordinate plane. It is typically written with the variable terms squared and divided by constants that relate to the ellipse's semi-major and semi-minor axes. The video script refers to this form when it discusses 'transforming standard equation of ellipse to general form,' indicating that the lesson will cover the transition from this more simplified representation to a more general one.
💡General Form
The general form of an equation is a more flexible representation that includes all the terms of the equation without specific constraints on their arrangement. In the context of the video, converting an ellipse's equation to its general form allows for a broader analysis and application of the ellipse's properties. The script exemplifies this by showing the process of 'transforming standard equation to general form,' which is a key step in the mathematical procedure being taught.
💡Vertex Form
Vertex form is a specific representation of a conic section's equation that highlights the vertex of the conic. For ellipses, the vertex form is particularly useful as it clearly shows the center of the ellipse and its orientation. Although not explicitly defined in the script, the term is implied when discussing the transformation of equations, suggesting that the standard form is a precursor to the vertex form, which is an intermediate step before reaching the general form.
💡Quantity
In the script, 'quantity' is used to denote a mathematical expression that is part of a larger equation. It is a term that encapsulates the variables and coefficients within it. For example, 'the quantity of x plus three, squared' is used to describe the expression (x + 3)^2, which is a component of the ellipse's equation in the video's examples.
💡LCD (Least Common Denominator)
The least common denominator is a concept in algebra that refers to the smallest multiple that is shared by all the denominators in a set of fractions. In the context of the video, the LCD is used to clear the fractions from the equation by multiplying both sides by this number, which simplifies the process of transforming the equation. The script mentions 'LCD' in the process of transforming the standard form to the general form, such as 'so, LCD 25 and four'.
💡Squaring
Squaring, in mathematics, refers to the operation of multiplying a number by itself. In the video, squaring is used to transform terms within the equation, such as 'x plus three, squared' which is written as (x + 3)^2. This operation is a part of the algebraic manipulation required to convert the equation from its standard form to the general form.
💡Combining Like Terms
Combining like terms is a fundamental algebraic process where terms in an equation that have the same variable raised to the same power are added or subtracted together. The video script refers to this process when simplifying the expanded form of the equation, such as 'combine similar terms so 3x plus 3x that is 6x', which is an essential step in reaching the general form of the ellipse's equation.
💡Constant Term
A constant term in an algebraic expression is a term that does not contain any variables. It is a fixed number that remains unchanged regardless of the values of the variables. In the video, the constant term is part of the final simplified equation, such as 'so you make variable x x ethereum 24x, and then you may variable in the y that is negative 50 y, so you e that is constant so san manger, and some constant terminal 36, plus 25 minus 100 the answer is negative, 39, equals zero'.
Highlights

Introduction to transforming the standard equation of an ellipse to its general form.

Explanation of the standard form of an ellipse equation.

Demonstration of transforming the equation using example number one.

Clarification on identifying LCD (Least Common Denominator) and its role in the transformation.

Multiplication of both sides of the equation by 100 to eliminate the denominator.

Expansion of the squared binomials (x+3)^2 and (y-1)^2.

Combining like terms to simplify the equation.

Transformation of the equation into the general form.

Presentation of the completed general form equation for the first example.

Introduction to the second example with a different ellipse equation.

Explanation of when LCD is not necessary for transformation.

Multiplication of the equation by 16 to transform it into the general form.

Simplification of the equation by combining like terms.

Finalization of the general form for the second example.

Introduction of the third example with yet another ellipse equation.

Calculation of the LCD as 3600 for the third example.

Multiplication of the equation by 3600 to transform it into the general form.

Expansion and simplification of the squared binomials for the third example.

Final general form equation for the third example presented.

Conclusion of the video with a summary of the process.

Invitation to like, subscribe, and hit the bell button for more video tutorials.

Transcripts

Browse More Related Video

STANDARD EQUATION OF AN ELLIPSE IN A GENERAL FORM

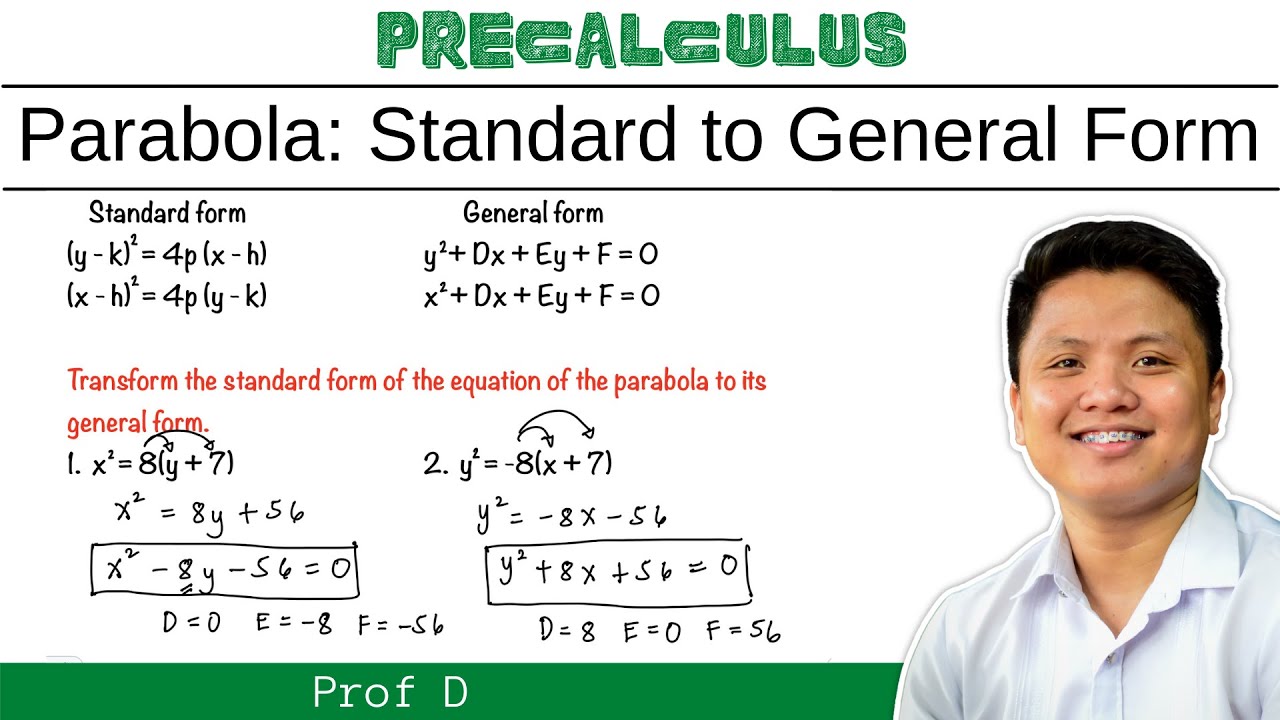
Transforming Standard Form to General Form of Parabola | @ProfD

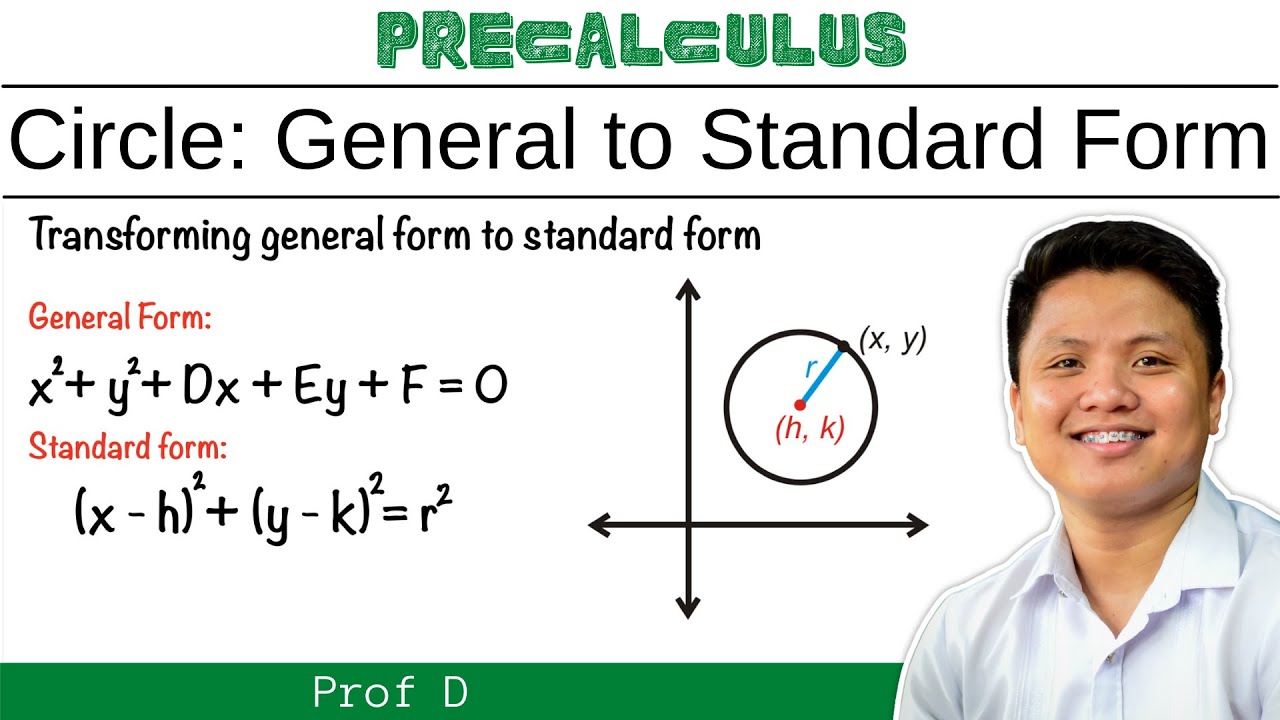
CIRCLE | TRANSFORMING GENERAL FORM TO STANDARD FORM | PROF D

PART 2: CIRCLES || PRE - CALCULUS

STANDARD EQUATION OF PARABOLA TO GENERAL FORM

GENERAL EQUATION OF THE HYPERBOLA IN STANDARD FORM || PRE-CALCULUS

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Ellipse EquationsMathematics TutorialStandard FormGeneral FormEducational ContentTransform EquationsGeometry LessonsMath ClassWalmart ChannelMathematics Learning