Functions and Graphs | Precalculus

The Organic Chemistry Tutor
27 Apr 202115:03
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive review of functions and their graphical representations through a series of problems. It begins with evaluating a quadratic function at a specific point, followed by solving for x when a function equals a constant. The script then delves into the vertical line test to identify functions from given graphs, calculates the value of a function from a graph, and discusses intervals of increase, decrease, and constancy. It also identifies the location and value of relative extrema, evaluates a piecewise function, and determines the domain and range of a graph. The script concludes with a method to find the difference quotient of a function, illustrating the process with a square root function. The video is designed to be interactive, encouraging viewers to pause and solve problems independently for a deeper understanding.

Takeaways
  • 📚 The video is a multiple-choice review focusing on functions and their graphs, starting with evaluating a quadratic function at a specific point.
  • 🔍 To evaluate a function at a specific point, replace the variable with the given value and perform the calculation.
  • 🧩 When solving for x where f(x) equals a constant, manipulate the equation to isolate x, considering the absolute value and solving for two possible x values.
  • 📉 The vertical line test is used to determine if a graph represents a function; a graph passes the test if no vertical line intersects it more than once.
  • 📈 The value of a function at a specific x can be found by looking at the corresponding y value on the graph.
  • 📊 To determine intervals of increase, decrease, and constancy for a function, analyze the graph's behavior and express these intervals using union notation.
  • 🏔 Identifying the location of a relative maximum or minimum on a graph involves locating the x-coordinate where the function reaches its peak or valley.
  • 📐 For piecewise functions, select the appropriate function segment based on the value of x and then evaluate it.
  • 📏 The domain of a graph is expressed using interval notation, including all possible x-values, while the range includes all possible y-values.
  • 🔑 The difference quotient is calculated using the formula (f(x+h) - f(x))/h, and simplification often involves multiplying by the conjugate to eliminate the square roots.
Q & A

  • What is the value of f(3) for the function f(x) = x^2 - 5x + 7?

    -To find f(3), substitute x with 3 in the function: f(3) = 3^2 - 5*3 + 7 = 9 - 15 + 7 = 1. So, f(3) equals 1, which corresponds to answer choice C.

  • If f(x) = 10, what is the possible value of x for the given quadratic function?

    -To solve for x when f(x) = 10, set the function equal to 10 and solve the resulting quadratic equation. After adding 8 to both sides and dividing by 2, we get x - 5 = ±9, which gives x = 14 or x = -4. Since -4 is listed, the correct answer is B.

  • How can you determine if a graph represents a function?

    -A graph represents a function if it passes the vertical line test, meaning a vertical line drawn anywhere through the graph will intersect it at no more than one point. Choices A, C, and D fail this test, while choice B passes, indicating it is a function.

  • What is the value of f(-1) according to the graph provided in the script?

    -To find f(-1), locate the point on the graph where x is -1. The corresponding y-value is 2, so f(-1) is equal to 2, which corresponds to answer choice D.

  • If f(x) = 3, what could be the possible values of x based on the graph?

    -When f(x) = 3, we look for the x-values where y equals 3 on the graph. The x-values are -2 and approximately 5, but since -2 is listed in the answer choices, x can be -2.

  • What are the intervals where the function f(x) = x^2 - 5x + 7 is increasing, decreasing, and constant?

    -The function is increasing before x = -2 and from x = 3 to infinity. It is decreasing between x = -2 and x = 3. The function is constant between x = -1 and x = 2.

  • Where is the relative maximum of f(x) located on the graph?

    -The relative maximum, which looks like a hill, is located at x = -2 on the graph, so the correct answer is B.

  • What is the relative minimum value of f(x) on the graph?

    -The relative minimum, which looks like a valley, is located at the y-coordinate of -2 on the graph, so the correct answer is B.

  • What is the value of f(4) for the piecewise function provided?

    -Since 4 is greater than 2, we use the first part of the piecewise function: f(4) = 4^2 + 4 = 16 + 4 = 20. Therefore, the correct answer is D.

  • How do you determine the domain and range of the graph shown in the script?

    -The domain includes all the x-values the graph represents, expressed in interval notation as (-5, -2) U [2, ∞). The range includes all the y-values, expressed as [-5, -3] U [1, ∞).

  • What is the difference quotient for the function f(x) = √(x + 2)?

    -The difference quotient is given by (f(x + h) - f(x))/h. For f(x) = √(x + 2), simplifying the expression yields 1/(√(x + h + 2) + √(x + 2)).

Outlines
00:00
📚 Evaluating Functions and Solving for x

This paragraph introduces a multiple-choice review of functions and graphs. It begins with a problem involving the function f(x) = x^2 - 5x + 7, where the task is to evaluate f(3). The solution involves substituting x with 3, which results in f(3) = 1, corresponding to answer choice C. The paragraph continues with a problem where f(x) = 10, and the goal is to solve for x, leading to two possible solutions, x = 14 or x = -4, with the latter being the correct answer as it's listed in the choices. The explanation emphasizes the importance of pausing the video to work through the problems independently for a more beneficial learning experience.

05:01
📈 Understanding Graphs and Functions

The second paragraph delves into identifying functions through the vertical line test. It explains that a graph represents a function if a vertical line intersects it at no more than one point. The paragraph evaluates multiple answer choices, eliminating those that fail the vertical line test due to intersecting at multiple points. The correct function, represented by answer choice B, touches the vertical line at only one point. The paragraph also covers determining the value of f(-1) from a given graph, identifying intervals of increase, decrease, and constancy in a function's behavior, and locating the relative maximum of a function, which is found at x = -2.

10:02
📉 Determining Function Behavior and Values

This paragraph focuses on identifying intervals where a function is increasing, decreasing, or constant, and it provides a method to express these intervals using union symbols and interval notation. It also explains how to find the relative minimum value of a function, which is associated with a specific y-coordinate on the graph. The paragraph includes a piecewise function example to illustrate how to determine the value of f(4), and it concludes with a discussion on how to express the domain and range of a graph using interval notation and inequalities.

🔍 Calculating the Difference Quotient

The final paragraph explains how to find the difference quotient of a given function using the formula f(x+h) - f(x) / h. It provides a step-by-step guide to simplify the expression, which involves substituting x with x+h, multiplying the numerator and denominator by the conjugate of the numerator, and then simplifying the resulting expression. The paragraph demonstrates the process of canceling terms and arriving at the final simplified form of the difference quotient, which is 1 / (sqrt(x + h + 2) + sqrt(x + 2)).

Mindmap
Keywords
💡Function
A function in mathematics is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. In the video, the function is used to describe the relationship between variables, such as f(x) = x^2 - 5x + 7, where x is the input and f(x) is the output. The script discusses evaluating the function at specific points, like f(3), and solving for x when f(x) equals a given value.
💡Graph
A graph is a visual representation of data, typically with a set of vertices and a set of edges connecting these vertices. In the context of the video, graphs are used to represent functions, where the x-axis represents the input values and the y-axis represents the output values. The script reviews how to interpret graphs to find function values, determine intervals of increase and decrease, and identify relative maxima and minima.
💡Multiple Choice
Multiple choice is a type of question that provides several options from which the respondent must choose the correct answer. The video script uses multiple choice questions to review concepts related to functions and graphs, allowing the viewer to select the correct answer from given options, such as identifying the correct value of x when f(x) = 10 or determining which graph represents a function.
💡Evaluate
To evaluate a function means to calculate its value at a specific point. In the video, the process of evaluating a function is demonstrated when the script describes how to find f(3) by substituting x with 3 in the function f(x) = x^2 - 5x + 7, resulting in f(3) = 1.
💡Absolute Value
The absolute value of a number is its distance from zero on a number line, regardless of direction. In the video, the concept of absolute value is used when solving for x in the equation 9 = |x - 5|, which leads to two possible solutions, x = 14 and x = -4, as the absolute value eliminates the negative aspect of the equation.
💡Vertical Line Test
The vertical line test is a method used to determine if a curve represents a function. According to the script, a curve passes the vertical line test if, when a vertical line is drawn anywhere through the curve, it intersects the curve at no more than one point. The video uses this test to identify which of the given graphs represent functions.
💡Piecewise Function
A piecewise function is a function that is defined by multiple pieces, each with its own formula. In the video, an example of a piecewise function is given where different formulas are used depending on the value of x, such as f(x) = x^2 + 4 for x > 2 and another formula for x ≤ 2. The script explains how to determine which piece to use when evaluating the function at a specific value of x.
💡Domain
The domain of a function is the set of all possible input values (x-values) for which the function is defined. In the video, the script discusses how to determine the domain of a graph, which is expressed in interval notation, indicating the range of x-values for which the graph is valid.
💡Range
The range of a function is the set of all possible output values (y-values) that result from applying the function to its domain. The video script explains how to find the range of a graph, which is depicted by the y-values that the graph reaches, and it is also expressed in interval notation.
💡Difference Quotient
The difference quotient is a mathematical formula used to estimate the slope of the tangent line to a curve at a given point. In the video, the script provides the formula for the difference quotient, f(x + h) - f(x) / h, and demonstrates how to apply it to a given function to find the rate of change at a specific point.
💡Relative Maximum and Minimum
A relative maximum or minimum is a point on a graph where the function changes from increasing to decreasing (relative maximum) or decreasing to increasing (relative minimum). The video script explains how to identify these points on a graph, which are characterized by a 'peak' or 'valley' shape, and provides an example of finding the x-value and y-value associated with a relative maximum or minimum.
💡Interval Notation
Interval notation is a way to express a set of numbers, particularly in the context of a function's domain and range. It uses parentheses and brackets to indicate whether the endpoints of an interval are included or excluded. The video script uses interval notation to describe the x-values and y-values that the function covers, such as (-∞, -2) ∪ [3, ∞) for the domain and [-5, -3] ∪ [1, ∞) for the range.
Highlights

Review of functions and graphs through multiple choice questions

Evaluating a quadratic function by substituting x with a specific value

Solving for x when a function equals a constant value

Using the vertical line test to determine if a graph represents a function

Identifying the value of a function from a graph

Finding x values that correspond to a specific y value on a graph

Analyzing intervals of increasing, decreasing, and constant functions

Locating the position of a relative maximum on a graph

Determining the value of a relative minimum from a graph

Applying piecewise functions to find the value of a function

Expressing the domain and range of a graph using interval notation

Calculating the difference quotient of a function

Simplifying expressions using conjugates to cancel terms

Final expression for the difference quotient

Understanding the concept of absolute value in equations

Determining the intervals where a function is increasing, decreasing, or constant

Transcripts

Browse More Related Video

Business Calculus - Math 1329 - Section 4.3 - Derivatives of Exponential and Logarithmic Functions

Calculus AB Homework Day 1 - Review 1: Functions

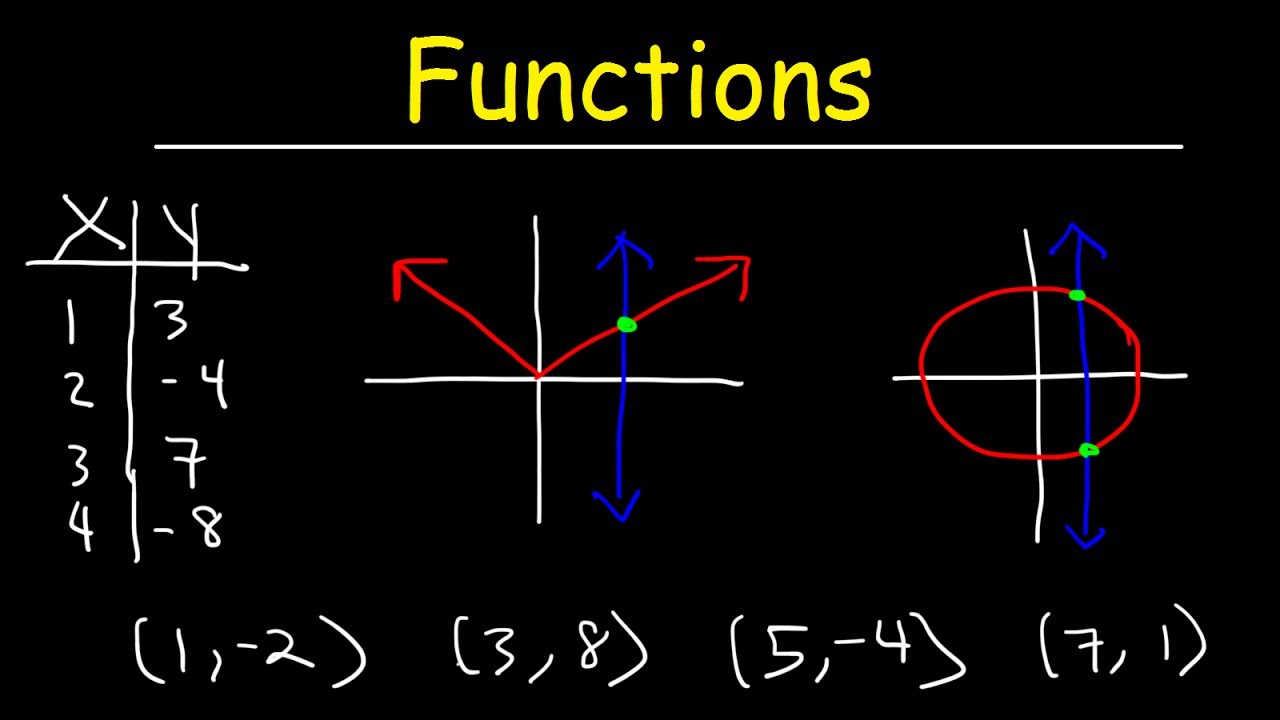
Functions - Vertical Line Test, Ordered Pairs, Tables, Domain and Range

Using the Definition of Continuity in Calculus

Linear Approximations | Using Tangent Lines to Approximate Functions

How to Graph Piecewise Functions (Precalculus - College Algebra 12)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Functions ReviewGraph AnalysisMathematics EducationMultiple ChoiceProblem SolvingEducational ContentAlgebra ConceptsVideo TutorialInteractive LearningMath Strategies